Given: 2x+13=1 Prove: x = 1 Select from the drop-down menus to correctly complete the proof. Statement Reason 2x+13=1 Given 2x+1=3 Choose... 2x = 2 Choose... x = 1 Choose...
Answers
The value of x from the equation is 1
Given the equation 2x + 1 = 3
We are to find the value of x as shown:
Step 1: Subtract 1 from both sides
2x + 1 - 1 = 3 - 1 (Subtraction property of equality)
2x = 2
Step 2: Divide both sides by 2:
2x/2 = 2/2 (Division property of equality)
x = 1
Hence the value of x from the equation is 1
Learn more here: https://brainly.com/question/2159099
x=1
explanation: k12
..................................
Related Questions
HELP ASAP ! Find the slope of the line.
A. \(\frac{1}{3}\)
B. 3
C. -\(\frac{1}{3}\)
D. -3
![HELP ASAP ! Find the slope of the line.A. [tex]\frac{1}{3}[/tex]B. 3C. -[tex]\frac{1}{3}[/tex]D. -3](https://i5t5.c14.e2-1.dev/h-images-qa/contents/attachments/h6eYF6yOZLxchry8dccTxP2FcEaZ3FeC.jpeg)
Answers
Answer:
A. \(\frac{1}{3}\)
Step-by-step explanation:
Slope = rise/run or (y2 - y1) / (x2 - x1)
Pick 2 points (0,2) (3,3)
We see the y increase by 1 and the x increase by 3, so the slope is
m = 1/3
So, the answer is A. \(\frac{1}{3}\)
Estimate 235 divided by 6
Answers
if you have a population mean and standard deviation of 7 and 3, respectively, what is the z-value when x is 4?
Answers
The z - value of given data is -1. A data point's z-value is a measurement of how many standard deviations the data point is from the population mean.
When x is 4, what is the z-value?The z-value for a given data point (x) can be calculated using the following formula: z = (x - ) /, where and are the population mean and standard deviation, respectively. In this case, the sum of 7 and 3 is 3. Consequently, we can enter the values into the formula with x = 4 to get the following outcome: z = (4 - 7) / 3 = -1
The data point x = 4 is therefore one standard deviation from the population mean. A standard score with a z-value of -1 can be used to compare a data point to other population values and make distributional inferences.
Learn more about standard deviation here:
brainly.com/question/23907081
#SPJ4
Follow this link to view Juan's work. Critique Juan's work by justifying correct solutions and by explaining any errors he made. For any errors made, provide and explain a correct response. Be sure to explain each key aspect of the graph.
and y’all please no bitly answers. i’ve been looking everywhere for an answer so please if you answer give the correct answer. :)

Answers
Answer:
Explain more
Step-by-step explanation:
Go to Wyzant for expert
*************MUST ANSWER!***************
Sebastian has a points card for a movie theater.
-He earns 85 points just for signing up.
-He earns 2.5 points for each visit to the movie theater.
-He needs 115 points for a free movie ticket.
Write and solve an Equation which can be used to determine v, the number of visits Sebastian must make to earn a free movie ticket.

Answers
Answer:
Look Down
Step-by-step explanation:
85+2.5v=115
2.5v=30
v=12
Find the amount that results from the given investment, \( \$ 50 \) invested at \( 10 \% \) compounded continuously after a period of 4 years After 4 years, the investment results in \( \$ \) (Round t
Answers
To find the amount that results from investing $50 at 10% interest compounded continuously after a period of 4 years, we can use the formula for continuous compound interest:
�=�⋅���
A=P⋅ert
where: A = Amount (result) P = Principal (initial investment) r = Interest rate t = Time in years e = Euler's number, approximately 2.71828
Plugging in the given values: P = $50 r = 10% = 0.10 t = 4 years
We can calculate the amount as follows:
�=$50⋅�0.10⋅4
A=$50⋅e
0.10⋅4
Using a calculator or a software, we can evaluate the exponential function to find the amount:
�≈$50⋅�0.40≈$73.23
A≈$50⋅e
0.40
≈$73.23
So, after 4 years, the investment results in approximately $73.23.
The investment results in approximately $73.23 after 4 years at compound interest.
To know more about compound interest, visit :
brainly.com/question/13155407
#SPJ11
The class average on a math test was 80.3 and the standard deviation was 5.7.
What percentage of the class scored above a 86?
Answers
Answer:
= 15.866%
Step-by-step explanation:
The class average on a math test was 80.3 and the standard deviation was 5.7.
What percentage of the class scored above a 86?
We solve using z score formula
z = (x-μ)/σ, where
x is the raw score = 86
μ is the population mean = 80.3
σ is the population standard deviation = 5.7
Hence:
z = 86 - 80.3/5.7
z = 1
Probability value from Z-Table:
P(x<86) = 0.84134
P(x>86) = 1 - P(x<86) = 0.15866
Converting to Percentage
= 0.15866 × 100
= 15.866%
Therefore, the percentage of the class scored above a 86 is 15.866%
for what value of x and y the ordered pairs (x+3,y-2) (y-1,2x-3)
Answers
u spammed in my question :(
Nine hundred sixty seven divided by four with remander
Answers
Answer:
240
Step-by-step explanation: If this helped pls give this Brainliest answer.
Answer:
967÷4= 241 r 3
Step-by-step explanation:
Can someone please help me with this?

Answers
Answer:.35,38% 3/5
Step-by-step explanation:Hope this helped
Answer: 0.35, 38% , 3/5
Step-by-step explanation:
3/5= 0.6= 0.60
38%= 0.38
0.35
Noir drove from the Dead Sea up to Amman, and her altitude increased at a constant rate. When she began driving her altitude was 400 meters below sea level. When she arrived in Amman 2 hours later, her altitude was 1000 meters above sea level. Let y represent noir’s altitude in meters relative to sea level after x hours.
Answers
Answer:
y(x) = -400 m + (700 m/hr)x
Step-by-step explanation:
Let y = 0 represent sea level. Noir's initial altitude was -400 m. In two hours she went from -400 m to +1000 m, a positive change of 1400 m. Thus, the rate of ascent was m = slope = (change in altitude) / (change in time), or
rate of ascent = 1400/2 m/hr = 700 m/hr
Thus, an equation describing her altitude relative to sea level after x hours is
y(x) = -400 m + (700 m/hr)x, where x is the number of hours of driving completed. This formula correctly predicts the altitude after two hours:
y(2) = -400 m + (700 m/hr)(2 hr) = 1000 m above sea level.
toms water bottle can hold up to 32 ounces of water. each day he drinks more than 2 full bottles of water. which inequality correctly describes the number of ounces of water, w, that tom drinks each day?
Answers
The inequality that correctly describes the number of ounces of water, w, that tom drinks each day is d. 64<w.
Amount of water that the bottle can hold = 32 ounces
Bottles of water consumed = 2
A mathematical comparison and representation of the connection between two expressions is known as an inequality. It can be regarded as a generalisation of an equation and is denoted by signs such as <, > and =.
Tom consumes more than two complete bottles of water every day. Since each bottle can carry up to 32 ounces of water, we can describe Tom's daily water consumption as -
= w > 2 × 32
Therefore,
Simplifying the right side of the inequality:
w > 64
Complete Question:
Toms water bottle can hold up to 32 ounces of water. each day he drinks more than 2 full bottles of water. which inequality correctly describes the number of ounces of water, w, that tom drinks each day?
a. w>2
b. 32<w
c. w = 64
d. 64<w
Read more about inequality on:
https://brainly.com/question/24372553
#SPJ4
5!, called 5 _______ is the product of all positive integers from _______ down through _______. by definition, 0!_______.
Answers
Answer:
120
Step-by-step explanation:
5!, called 5 factorial is the product of all positive intergers from 5 down though 1. by definition, 0! is 0.
Factorials are basically the number times itself-1 the 2 the 3 until it times it by itself-(itself-1). n!=n*(n-1)*n(n-2).....*n-(n-1).
For example 2!=2*1=2 and 6!=6*5*4*3*2*1=720
keep in mind that I am not an expert so the blanks I filled in might be wrong and the explanation might have errors
5!, called "5 factorial," is the product of all positive integers from 5 down through 1. By definition, 0! is equal to 1.
Factorial is a mathematical operation denoted by an exclamation mark (!). It represents the product of all positive integers from a given number down to 1. In the case of 5!, it is calculated as 5 × 4 × 3 × 2 × 1, resulting in the value of 120.
The exclamation mark notation allows us to represent the factorial of a number concisely. For example, 5! represents the factorial of 5. It is important to note that 0! is defined to be equal to 1. This is a special case in factorial calculations. While it may seem counterintuitive, it is defined this way to maintain consistency and enable certain mathematical calculations and formulas.
Therefore, 5! is the product of all positive integers from 5 down through 1, equaling 120, and 0! is defined to be 1.
Learn more about Factorial here:
https://brainly.com/question/30136880
#SPJ11
PLEASE HELP, GIVING BRAINLIEST!!!!
Triangle LMP ≅ triangle SMQ
A. This is SAS
B. This is SSS

Answers
Answer:
sas
Step-by-step explanation:
Answer:
Step-by-step explanation:
There is no third fact. Either LM must equal MS or P and Q are both right angles. You can't assume that is true. It must be part of the givens. Even LP is perpendicular to PQ QS is perpendicular to PQ would do it. But as it stands, there is no solution.
If P and Q are both right angles, the proof would be ASA which is not a choice.
If LM =MS (as a given), then it would be SAS
Since neither of these are possible, there is no answer.
Figure ABCD is a parallelogram.
What is the value of x?
1. 6
2.7
3.8
4.9
Please help fast!!!

Answers
Answer:
x = 7
Step-by-step explanation:
Parallel sides have to be the same length
5x+3 = 38
Subtract 3 from each side
5x+3-3 = 38-3
5x = 35
Divide by 5
5x/5 = 35/5
x = 7
3x
2x
x + 30
The diagram shows a triangle.
The sizes of the angles, in degrees, are
3x
2x
x + 30
Work out the value of x
Answers
Answer:
x = 25°
Step-by-step explanation:
3x + 2x + x + 30° = 180°
6x + 30° = 180°
6x = 150°
x =25°
=> 3x = 25 * 3 = 75°
=> 2x = 25 * 2 = 50°
=> x + 30° = 25° + 30° = 55°
thank for t
he answer
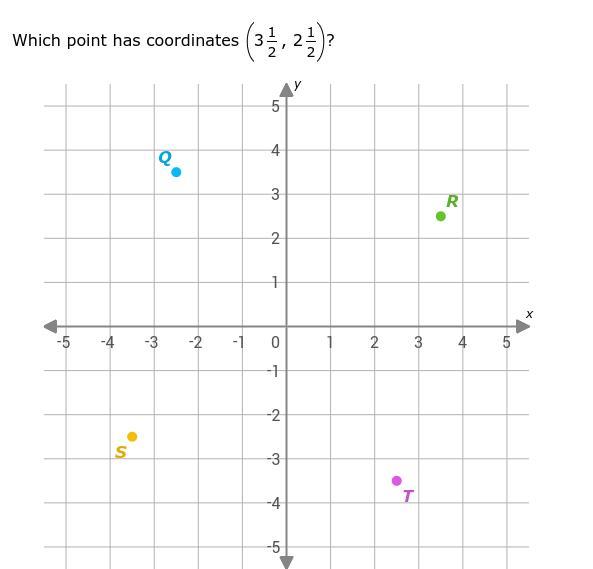
Answers
Answer:
R
Step-by-step explanation:
Look at the X value, 3.5, and draw a line from 3.5 up on the X axis.
Look at the Y value, 2.5, and draw a horizontal line on the y axis. Your point is at R.
Given the equation y = 3(2)x

Answers
Regarding the exponential function y = 3(2)^x, we have that:
We know that the graph has a y-intercept at (0,3), because the a-value is of 3.We know that the graph models exponential growth, because the b-value is of 2.The numeric value of the function at x = 3 is given as follows: 24.What is the exponential function?An exponential function is defined as follows:
y = ab^(x/n).
In which the parameters are defined as follows:
a is the initial value.b is the rate of change.n is the time needed for the rate of change.The function for this problem is given as follows:
y = 3(2)^x.
Hence the parameters are given as follows:
a = 3 -> y-intercept at (0,3).b = 2 > 1, hence exponential growth.At x = 3, the numeric value of the function is obtained as follows:
y = 3 x 2^3
y = 3 x 8
y = 24.
More can be learned about exponential functions at https://brainly.com/question/30113628
#SPJ1
help plz i will give all my point's

Answers
Answer:
B the answer is B I believe so
Total cookies sold were: 69
Gabby sold: 35
We don’t know how many Ross sold so his cookies are: B (until calculated)
Equation:
35+B=69
Option C is the answer.
We want to calculate the total amount of cookies and in order to do so, we must add 35 and B to calculate 69.
I can give you a more in depth explanation if you want just leave a comment! (Also no need to give any points). I also tutor, so if you need any math help I can leave my email in the comments.
Roger wants to compare values across categories using vertical rectangles. which of the following charts must roger use?
Line chart
Clustered column chart
Pie chart Stacked
column chart
Answers
To compare values across categories using vertical rectangles, Roger should use:
Clustered Column Chart.
Roger must use a Clustered Column Chart to compare values across categories using vertical rectangles. This type of chart represents data with vertical bars, making it easy to compare values across different categories. By visually comparing the heights of the columns, you can quickly identify which category has the highest or lowest value, as well as observe the relative differences between the categories.
In contrast, a line chart is more suitable for showing trends over time, a pie chart is used to represent proportions or percentages of a whole, and a stacked column chart is used to show the composition of a whole across different categories.
To learn more about a line charts visit : https://brainly.com/question/26233943
#SPJ11
A survey of 25 randomly selected customers found the ages shown (in years). The mean is 30.96 years and the standard deviation is 9.54 years. a) Construct a 90% confidence interval for the mean age of all customers, assuming that the assumptions and conditions for the confidence interval have been mat. b) How large is the margin of error? c) How would the confidence interval change if you had assumed that the population standard deviation was known to be 10.0 yeans?
Answers
To calculate the 90% confidence interval of the population mean age, we can use the following formula: 90% Confidence Interval = sample mean ± margin of error where margin of error = critical value * standard errorLet us calculate the critical value and standard error first.
For a 90% confidence interval, the level of significance is α = 0.10 (10% of probability is distributed between two tails of the normal distribution curve). The corresponding critical values can be obtained from the normal distribution table. Since the sample size is n = 25, we can use a t-distribution with (n - 1) = 24 degrees of freedom to calculate the standard error. The formula for the standard error is: standard error = standard deviation / sqrt(sample size)Substituting the given values:
standard error = 9.54 / sqrt(25) = 1.908
Critical value at α/2 = 0.05 level of significance with 24 degrees of freedom = ±1.711We can calculate the margin of error by multiplying the critical value by the standard error:
margin of error = 1.711 * 1.908 = 3.267
Therefore, the 90% confidence interval for the mean age of all customers is:
90% CI = 30.96 ± 3.267 = (27.693, 34.227)
The margin of error for a 90% confidence interval is 3.267. This means that if we repeatedly drew random samples of 25 customers from the population and calculated their mean age, about 90% of the confidence intervals that we constructed using the sample data would contain the true population mean age. The margin of error is influenced by the sample size and the level of confidence. As the sample size increases, the margin of error decreases, and vice versa. As the level of confidence increases, the margin of error increases, and vice versa. If we assumed that the population standard deviation was known to be 10.0 years, we can use the normal distribution instead of the t-distribution to calculate the critical value. The formula for the critical value is: critical value = zα/2 where zα/2 is the z-score for the desired level of significance α/2. For a 90% confidence interval, α/2 = 0.05 and the corresponding z-score is 1.645 (obtained from the normal distribution table). The formula for the margin of error is:
margin of error = zα/2 * standard error = 1.645 * 9.54 / sqrt(25) = 3.047
The 90% confidence interval for the mean age of all customers, assuming a known population standard deviation of 10.0 years, is:
90% CI = 30.96 ± 3.047 = (27.913, 34.007)
Thus, the 90% confidence interval for the mean age of all customers is (27.693, 34.227) with a margin of error of 3.267. If we had assumed that the population standard deviation was known to be 10.0 years, the 90% confidence interval would be (27.913, 34.007) with a margin of error of 3.047.
To learn more about t-distribution visit:
brainly.com/question/32675925
#SPJ11
Simplify the expression (3xy-3/5x-2y2)2
Answers
6x3y5
—————
5
Step-by-step explanation:
q8
an È 2n2+31 If it is applied the Limit Comparison test for n=1 V5+n5 than lim n-00 bn
Answers
To apply the Limit Comparison Test for the series\(Σ(2n^2 + 3)/(5 + n^5)\) as n approaches infinity, we can compare it with the series\(Σ(1/n^3).\)
First, we need to find the limit of the ratio of the two series as n approaches infinity:
\(lim(n- > ∞) [(2n^2 + 3)/(5 + n^5)] / (1/n^3)\)
Next, we can divide the numerator and denominator by the highest power of n:
\(lim(n- > ∞) [2 + (3/n^2)] / (1/n^5)\)
Taking the limit as n approaches infinity, the second term (3/n^2) approaches zero, and the expression simplifies to:
l\(im(n- > ∞) [2] / (1/n^5) = 2 * n^5\)
Therefore, if the series\(Σ(1/n^3)\) converges, then the series \(Σ(2n^2 + 3)/(5 + n^5)\) also converges. And if the series Σ(1/n^3) diverges, then the series \(Σ(2n^2 + 3)/(5 + n^5)\) also diverges.
learn more about:- Limit Comparison Test here
https://brainly.com/question/31362838
#SPJ11
the difference between an actual score and a predicted score is called a(n) ____________.
Answers
The difference between an actual score and a predicted score is called a residual.
In various fields such as statistics, econometrics, and data analysis, the term "residual" refers to the difference between the observed or actual value of a variable and the value predicted by a model or estimation method. It represents the unexplained or leftover variation in the data after considering the model's predictions.
In the context of regression analysis, the predicted score is obtained by fitting a regression model to the data and using it to estimate the expected value of the dependent variable. The difference between the actual value and the predicted value for a specific observation is the residual for that observation.
Residuals play a crucial role in assessing the goodness of fit of a regression model. By examining the pattern and properties of residuals, such as their mean, variance, and distribution, analysts can evaluate how well the model captures the underlying relationships in the data and identify any systematic deviations or outliers.
Overall, residuals provide valuable information for understanding the accuracy and precision of predictions made by a model and can be used to refine and improve the model's performance.
Learn more about variable at: brainly.com/question/15078630
#SPJ11
Do not assume that any of the angles are equal. Show your work.
1. if ∠ 3 is 45 degrees. Find ∠9.
2. if ∠2 = 72 degrees, and ∠4 = 57 degrees, find ∠3.
3. if ∠7 = 120 degrees, and ∠5 = 40 degrees. Find ∠1.
4. if ∠8 = 145 degrees, and ∠1 = 37 degrees, find ∠2.
5. if ∠2 = 72 degrees, and ∠4 = 57 degrees, find ∠3.
6. ∠6 = 139 degrees, and ∠2 = 68 degrees. Find ∠7.

Answers
Answer:
∠2 = 60°, ∠3 = 120°, ∠4 = 60°, ∠5 = 120°, ∠6 = 60°, ∠7 = 120° and ∠8 = 60°
Step-by-step explanation:
l II m and p is their transversal and 1 = 120°
∠1 + ∠2 = 180 °(Straight line)
120° + ∠2 = 180° > ∠2 = 180° - 120° = 60°
∠2 = 60°
But ∠1 = ∠3 (Vertically opposite angles)
∠3 = ∠1 = 120°
Similarly ∠4 = ∠2
(Vertically opposite angles)
∠4 = 60°
∠5 = ∠1 (Corresponding angles)
∠5 = 120°
Similarly ∠6 = ∠2 (Corresponding angles)
∠6 = 60°
∠7 = ∠5 (Vertically opposite angles)
∠7 = 120°
And ∠8 = ∠6 (Vertically opposite angles)
∠8 = 60°
Hence ∠2 = 60°, ∠3 = 120°, ∠4 = 60°, ∠5 = 120°, ∠6 = 60°, ∠7 = 120° and ∠8 = 60°
AE is an angle bisector of BAC.
If mBAE = x + 30 and mEAC = 3x - 10,
determine the value of x.

Answers
Answer:
It’s (20) hope this helps
Step-by-step explanation:
The value of x is 20.
What is a Triangle?Polygon which has three sides and three vertices is called as triangle.
Sum of all the angles of the triangle is 180°.
Given, line AE is an angle bisector of BAC.
In the triangle ABC:
Line AE bisects ∠BAC into two equal angle.
That means, ∠BAE = ∠EAC
And the angle measure is given.
So, mBAE = mEAC,
x +30 = 3x - 10
3x-x = 30+10
2x = 40
x = 20
Therefore, the value of x is 20.
To learn more about the triangle;
https://brainly.com/question/2773823
#SPJ5
A student calculated the slope of the line graphed below to
be 2.
Explain the mistake and give the correct slope.

Answers
The slope of a linear function is calculated as the change in y divided by the change in x, instead of the change in x divided by the change in y, as the student did, hence the correct slope is given as follows:
1/2 = 0.5.
How to define a linear function?The slope-intercept equation for a linear function is presented as follows:
y = mx + b
In which:
m is the slope.b is the intercept.From the graph, when x increases by 2, y increases by 1, hence the slope m of the linear function is given as follows:
m = 1/2
m = 0.5.
More can be learned about linear functions at https://brainly.com/question/15602982
#SPJ1
In a 30-60-90 triangle, the smallest side measures inches 6v10 inches. Which is the exact measure for the largest side? a. 12v10 inches b. 2v30 inches c. 3v10 inches a. 6v5 inches
Answers
The exact measurements for the largest side is 12√10 inches. Hence option a is the correct option.
What is the right triangle?
A right triangle or right-angled triangle, or more formally an orthogonal triangle, formerly known as a rectangle triangle, is a triangle with one right angle, i.e. two perpendicular sides. Trigonometry is founded on the relationship between the sides and other angles of a right triangle.
Given that the angles of a triangle are 30-60-90.
The smallest angle is always opposite the shortest side, and vice versa. The longest side is also opposite the largest angle, and vice versa.
The opposite side of angle 30 is the shortest side of the triangle.
The opposite side of angle 90 is the longest side of the triangle.
Sine law:
a/sin A = b/ sin B =c/sin C
a is the opposite side of angle A, b is the opposite side of angle B, c is the opposite side of angle C.
Assume that the length of the longest side is a.
Applying sine law in the given triangle:
6√10/sin 30 = a/sin 90
6√10/(1/2)= a/1
a = 12√10
To learn more about sine law, click on the below link:
https://brainly.com/question/9339489
#SPJ4
Find the generating function of the sequence {an}n≥0 determined by an = an−1 + 6an−1 with initial conditions a0 = 1, a1 = 3. You need to find the closed form of the generating function, but you don’t need find the closed form of the coefficients.
Answers
The generating function for the sequence {an} is given by a(x) = (1 + 2x) / (1 - x - 6x^2). It captures the terms of the sequence {an} as coefficients of the powers of x.
To find the generating function of the sequence {an}, we can use the properties of generating functions and solve the given recurrence relation.
The given recurrence relation is: an = an-1 + 6an-2
We are also given the initial conditions: a0 = 1 and a1 = 3.
To find the generating function, we define the generating function A(x) as:
a(x) = a0 + a1x + a2x² + a3x³ + ...
Multiplying the recurrence relation by x^n and summing over all values of n, we get:
∑(an × xⁿ) = ∑(an-1 × xⁿ) + 6∑(an-2 × xⁿ)
Now, let's express each summation in terms of the generating function a(x):
a(x) - a0 - a1x = x(A(x) - a0) + 6x²ᵃ⁽ˣ⁾
Simplifying and rearranging the terms, we have:
a(x)(1 - x - 6x²) = a0 + (a1 - a0)x
Using the given initial conditions, we have:
a(x)(1 - x - 6x²) = 1 + 2x
Now, we can solve for A(x) by dividing both sides by (1 - x - 6x^2²):
a(x) = (1 + 2x) / (1 - x - 6x²)
Therefore, the generating function for the given sequence is a(x) = (1 + 2x) / (1 - x - 6x²).
Read more on Functions here: https://brainly.com/question/29890699
#SPJ11
What is an equation of the vertical line that passes through (−2, −9)?
x=?
Answers
Answer:
x = -2
Step-by-step explanation: