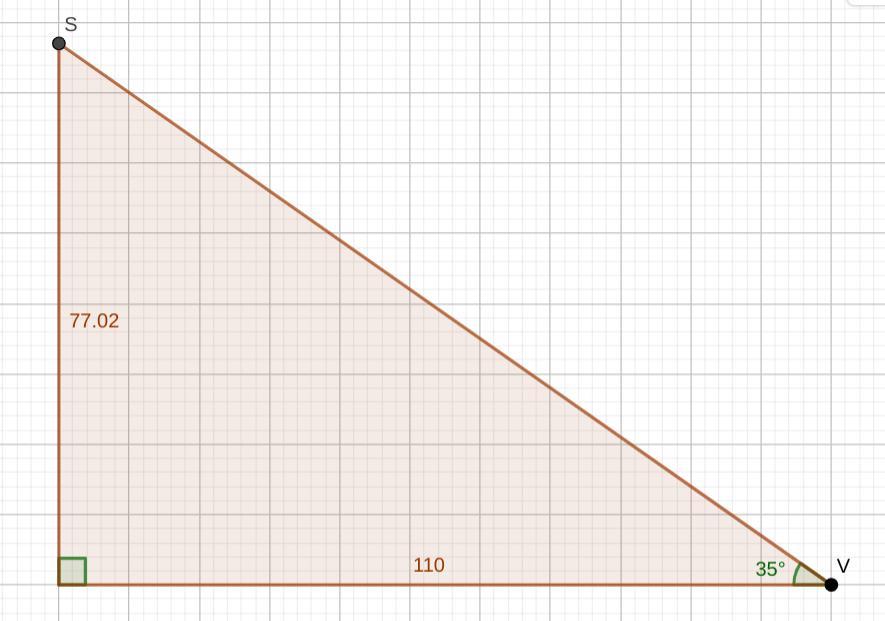
From a distance of 110 m, the angle of elevation to the top spire of a tower is 35°.
How high is the tower to the nearest metre

The volume of the helium-filled balloon at 0.855 atm and 10.0 °C will be approximately 42.81 L, calculated using the ideal gas law equation.

To compute this problem, we can use the ideal gas law, which states that PV = nRT, where P is the pressure, V is the volume, n is the number of moles, R is the gas constant, and T is the temperature in Kelvin.

First, we need to convert the initial temperature of 25 °C to Kelvin:

T1 = 25 + 273.15 = 298.15 K

Next, we can rearrange the ideal gas law equation to solve for V2:

V2 = (P1 * V1 * T2) / (P2 * T1)

We have:

P1 = 1.08 atm (initial pressure)

V1 = 50.0 L (initial volume)

P2 = 0.855 atm (final pressure)

T2 = 10.0 °C (final temperature)

Converting the final temperature to Kelvin:

T2 = 10 + 273.15 = 283.15 K

Substituting the values into the equation:

V2 = (1.08 * 50.0 * 283.15) / (0.855 * 298.15)

V2 ≈ 42.81 L

Therefore, the volume of the helium-filled balloon at 0.855 atm and 10.0 °C will be approximately 42.81 L.

To know more about ideal gas law refer here:

https://brainly.com/question/30458409#

#SPJ11

Answer: FALSE

Step-by-step explanation:

                                   1500 decagram = 15 kg

                                      1 decagram = 0.01 kg

                                       1500 decagram = 1500/0.01 kg

                                                                   = 1500/100 kg

                                        1500 decagram = 15kg  

Answer:

If you entered the function correctly there is not a range  

Step-by-step explanation:

Answer: {y|-♾<y<0}

Step-by-step explanation: i’m taking this summer school class

Answer:

y=-2x+6

\

Step-by-step explanation:

Answer:

2.38 yds^3

Step-by-step explanation:

Volume of a box = width x length x height

Now we multiply!!

? = 1 x 1.7 x 1.4

1 x 1.7 x 1.4 = 2.38

Have an amazing day!!

Please rate and mark brainliest!!

\( \huge\fbox\orange{ANSWER} \)

\( \mathsf \purple{v = l \times w \times h}\)

\( \mathsf \pink{v = 1.7 \times 1 \times 1.4}\)

\( \mathsf \purple{v = 2.38 {yd}^{3} }\)

2.38 cubic yards

The function in variable w giving the cost C (in dollars) of constructing the box is C(w) = 30w² + 270/w. The result is obtained by using the formula of volume and area of the box.

We have a rectangular storage container without a lid.

The formula of volume of the box is

V = l × w × h

Where

So, the height is

10 = 2w × w × h

10 = 2w² × h

h = 10/2w²

h = 5/w²

To find the total cost, calculate the area of base and sides of the box!

See the picture in the attachment!

The base area is

A₁ = 2w × w = 2w² m²

The sides area is

A₂ = 2(2wh + wh)

A₂ = 2(3wh)

A₂ = 6wh

A₂ = 6w(5/w²)

A₂ = 30/w m²

The total cost is

C = $15(2w²) + $9(30/w)

C = $30w² + $270/w

The function of the total cost is

C(w) = 30w² + 270/w

Hence, the function of constructing the box is C(w) = 30w² + 270/w.

Learn more about function of area here:

brainly.com/question/28698395

#SPJ4

Refer to the image for the graph of the lines.

The common form of the equation of a line is y = mx + c, where m is the slope of the line and c is a constant.

We need to draw any line with a slope m = 2, and

another line with a slope m = -2.

Disclaimer: Let us assume that the constant c = 0.

Then the equation to the line with a slope of "positive" 2 is given by

y = 2x

Then the equation to the line with a slope of "negative" 2 is given by

y = -2x

Refer to the attached image for the graph of the lines with the slope of "positive" 2 and "negative" 2.

f: green line indicates a line with a slope of "positive" 2.

g: blue line indicates a line with a slope of "negative" 2.

Learn more at:

https://brainly.com/question/28360812

#SPJ9

The transitive closure of {a,b,c,d,e} is option A which is {(a, c), (b, d), (c, a), (d, b), (e, d)}.

In mathematics, the transitive closure of a paired connection R on a set X is the littlest connection on X that contains R and is transitive. For limited sets, "smallest" can be taken in its standard sense, of having the least related matches; for boundless sets, it is the unique minimal transitive superset of R. Hence the transitive closure of {a,b,c,d,e} is option A which is {(a, c), (b, d), (c, a), (d, b), (e, d)}.

The reflexive closure of {a,b,c,d,e} is {(a,a),(a,e),(b,a),(b,b),(b,d),(c,c),(c,d),(d,a),(d,c),(d,d),(e,a),(e,b),(e,c),(e,e)}

as reflexive closure is the union of relation R and the identity relation on set so{ (a, a),(b,b),(c,c),(d,d),(e,e)} U {R} will result in {(a, a),(a,e),(b, a),(b,b),(b,d),(c,c),(c,d),(d, a),(d,c),(d,d),(e, a),(e,b),(e,c),(e,e)}

Symmetric closure will be the union of relation R and the inverse of relation R so the symmetric closure will be

{(a,e),(b,a),(a,b),(b,d),(d,b),(c,d),(d,c),(d,a),(a,d),(e,b),(b,e),(e,c),(c,e),(e,e)}.

To learn more about closures and their properties,

https://brainly.com/question/19340450

#SPJ4

Answer: my best guess is… (a, b, c, d, e}

Step-by-step explanation: I hope this helps<3

Yes, there are experts here in data forecasting methods who can help you with the topics you've mentioned including time series (holts, holts winter), naive method, regression, acf, pacf, arima, stl method and multivariate time series.

Below are brief explanations of each of these terms:

To know more about data forecasting, visit

https://brainly.com/question/28431229

#SPJ11

Answer:

Elena could tell without finishing by simplifying both sides. The next step would have been 30x +24x = 30x+24. This would have been simplified down to x=1

Step-by-step explanation:

She got to the last line 36x + 18 = 36x + 18 when she stopped and said the equation has infinitely many solutions.

The allocation of weights to the important variables that produce the calculation's optimum is referred to as a direct consequence.

The equation is given as,

12x +6(4x + 3) = 6(6x + 4) - 6

Simplify the equation, then we have

12x +6(4x + 3) = 6(6x + 4) - 6

12x + 24x + 18 = 36x + 24 - 6

36x + 18 = 36x + 18

She got to the last line 36x + 18 = 36x + 18 when she stopped and said the equation has infinitely many solutions.

More about the solution of the equation link is given below.

https://brainly.com/question/545403

#SPJ2

The number of distinct telephone numbers possible given the restriction is 800,000.

Given that :

For the first digit, there are 8 options available (digits 0-6 and 9, excluding 7 and 8).

For the remaining five digits (second to sixth), there are 10 options available for each digit (digits 0-9).

Therefore, the total number of distinct telephone numbers possible can be calculated by multiplying the number of options for each digit:

Total number of distinct telephone numbers = 8 * 10 * 10 * 10 * 10 * 10 = 8 * 10⁵ = 800,000

Hence, there are 800,000 distinct telephone numbers possible in this country.

Learn more on combination: https://brainly.com/question/4658834

#SPJ4

Answer:

106

Step-by-step explanation:

Interior angles add up to 360 degrees.

360-74-74(angles across are the same)=212

212/2=106 degrees

Answer:

x = 106°

Step-by-step explanation:

adjacent angles in this parallelogram are supplementary (add to 180°)

The sequence converges to a limit of 1.

To determine whether the sequence converges or diverges, and to find the limit if it converges, let's examine the sequence given by the formula:

an = (2n^2 - 5n) / (2n^2 + 1)

To check for convergence, we can take the limit of the sequence as n approaches infinity.

lim(n→∞) (2n^2 - 5n) / (2n^2 + 1)

Let's simplify the expression by dividing every term by n^2:

lim(n→∞) (2 - 5/n) / (2 + 1/n^2)

As n approaches infinity, both 5/n and 1/n^2 go to zero, so we have:

lim(n→∞) (2 - 0) / (2 + 0)

lim(n→∞) 2/2To determine whether the sequence converges or diverges, and to find the limit if it converges, let's examine the sequence given by the formula:

an = (2n^2 - 5n) / (2n^2 + 1)

To check for convergence, we can take the limit of the sequence as n approaches infinity.

lim(n→∞) (2n^2 - 5n) / (2n^2 + 1)

Let's simplify the expression by dividing every term by n^2:

lim(n→∞) (2 - 5/n) / (2 + 1/n^2)

As n approaches infinity, both 5/n and 1/n^2 go to zero, so we have:

lim(n→∞) (2 - 0) / (2 + 0)

lim(n→∞) 2/2

The limit simplifies to 1. Therefore, the sequence converges, and the limit of the sequence is 1.

Learn more about converges

brainly.com/question/15415793

#SPJ11

(a) Without more information, we cannot determine the initial number of insects. (b) The differential equation satisfied by P(t) is: dP/dt = kP, where k is the growth rate of the insect population.

(c) To find the time it takes for the population to double, we can use the formula:

2P(0) = P(0)e^(kt)

where P(0) is the initial population size. Solving for t, we get:

t = ln(2)/k

(d) Without more information, we cannot determine the time at which the population will equal a certain value.
Hi! To answer your question, I need the specific function P(t). However, I can provide you with a general framework to answer each part of your question once you have the function.

(a) To find the initial number of insects, evaluate P(t) at t=0:
P(0) = [Insert the function with t=0]

(b) To find the differential equation satisfied by P(t), differentiate P(t) with respect to t:
dP(t)/dt = [Insert the derivative of the function]

(c) To find the time at which the population doubles, first determine the initial population, P(0), then solve for t when P(t) is twice that value:
2*P(0) = P(t)
Solve for t: [Insert the solution for t]

(d) To find the time at which the population equals a specific value (let's call it N), set P(t) equal to N and solve for t:
N = P(t)
Solve for t: [Insert the solution for t]

Once you have the specific function P(t), you can follow these steps to find the answers to each part of your question.

Learn more about population here : brainly.com/question/27991860

#SPJ11

Answer:

Option (4)

Step-by-step explanation:

Equation of a line passing through a point \((x_1,y_1)\) and with slope 'm' is,

y - y₁ = m(x - x₁)

Given → Slope 'm' = -2

Point through which the line is passing is (-1, -2)

x₁ = -1 and y₁ = -2

Therefore, equation will be,

y + 2 = -2(x + 1)

y = -2x - 2 - 2

y = -2x - 4

Option (4) is the correct option.

The average rate of change of f(x) = 2^x over the interval [1, 3] is 3.

To find the average rate of change of f(x)=2^x over the x interval [1,3], we use the formula: (f(3) - f(1)) / (3 - 1).

To calculate the average rate of change of a function over a specific interval, we use the following formula:

Average Rate of Change = (f(b) - f(a)) / (b - a),

where f(a) represents the value of the function at the lower limit of the interval (a) and f(b) represents the value of the function at the upper limit of the interval (b).

In this case, the function is f(x) = 2^x and the interval is [1, 3].

To find the average rate of change of f(x) over the interval [1, 3], we substitute the values into the formula:

Average Rate of Change = (f(3) - f(1)) / (3 - 1).

Now, let's calculate f(3) and f(1):

f(3) = 2^3 = 8,
f(1) = 2^1 = 2.

Substituting these values into the formula:

Average Rate of Change = (8 - 2) / (3 - 1) = 6 / 2 = 3.

Therefore, the average rate of change of f(x) = 2^x over the interval [1, 3] is 3.

Know more about average rate of change here:

https://brainly.com/question/13235160

#SPJ11

Answer:

So the sequence of the five numbers are;

10,26,74,218,650

Step-by-step explanation:

In this question, we are interested in building a sequence of 5 numbers given the initial term.

Okay, to build this sequence, we will need to look at the rule that guides the building of the new sequence:

The rule is that the next term is 4 less than 3 times the previous term.

Okay, let’s call the next term y, while the previous term is x.

Following what was specified in the question;

y = 3x -4

Recall; the next term(y) is 4 less (-4) than 3 times (3 multiplied by) the previous term (x)

So let’s start with the initial term of 10.

The next term will be;

y = 3(10) -4 = 30-4 = 26

The next term after 26 will be ;

y = 3(26) -4 = 78-4 = 74

The next term after 74 will be;

y = 3(74) -4 = 222-4 = 218

The next term after 218 will be;

y = 3(218) -4 = 650

Answer:

B

Step-by-step explanation:

hope this helps with the work

Answer:

$1,849

Step-by-step explanation:

7,396/4=1,849

Answer: The simplest form of 2114 is 32.

Answer: g < 5

5 is the amount of gigabytes

Oliver can use up to 5 gigabytes while staying within his budget of $60 per month.

The inequality representing the number of gigabytes Oliver can use while staying within his budget of $60 per month is:

3g + 45 ≤ 60.

where,

"g" represents the number of gigabytes used.

$3 is the cost per gigabyte.

$45 is the flat monthly cost.

$60 is the maximum budget.

Oliver pays a flat cost of $45 per month, which is represented by the constant term 45 in the inequality.

He also pays $3 per gigabyte, so the cost for the gigabytes used is 3g, where "g" represents the number of gigabytes.

The total cost (3g + 45) must be less than or equal to $60, as he wants to keep his bill under $60 per month.

Hence, the inequality is 3g + 45 ≤ 60.

Now, solve for g:

45 + 3g ≤ 60

Subtract 45 from both sides:

3g ≤ 15

Divide both sides by 3:

g ≤ 5

So, Oliver can use up to 5 gigabytes while staying within his budget of $60 per month.

To learn more on inequality click:

https://brainly.com/question/28841069

#SPJ3

The area of the hexagon is 498.6 units

A regular polygon is a type of polygon with thesame sides and angles. The area of a polygon is given as ;

A = n× s × a/2

where n is the number of sides

a is the apothem and

s is the side length

Side length = apothem × 2tan (180/n)

apothem = 12

s = 12 × 2tan 30

s = 12×1.154

s = 13.85

A = 6 × 13.85 × 12/2

= 498.6 units²

therefore the area of the hexagon is 498.6 units²

learn more about area of polygon from

https://brainly.com/question/22640000

#SPJ1

(a) 95% confidence interval for the difference between female and male times is (11.954, 255.591).

(b) The assumptions and conditions for the two-sample t-test are met, so we can use the results of the test and confidence interval.

a) To construct a 95% confidence interval for the difference between female and male times, we can use a two-sample t-test. Let's denote the mean time for female swimmers as μf and the mean time for male swimmers as μm. We want to test the null hypothesis that there is no difference between the two means (i.e., μf - μm = 0) against the alternative hypothesis that there is a difference (i.e., μf - μm ≠ 0).

The formula for the two-sample t-test is:

t = (Xf - Xm - 0) / [sqrt((s^2f / nf) + (s^2m / nm))]

where Xf and Xm are the sample means for female and male swimmers, sf and sm are the sample standard deviations for female and male swimmers, and nf and nm are the sample sizes for female and male swimmers, respectively.

Using the data from the plots, we get:

Xf = 917.5, sf = 348.0137, nf = 15

Xm = 783.7273, sm = 276.0625, nm = 28

Plugging in these values, we get:

t = (917.5 - 783.7273 - 0) / [sqrt((348.0137^2 / 15) + (276.0625^2 / 28))] = 2.4895

Using a t-distribution with (15+28-2) = 41 degrees of freedom and a 95% confidence level, we can look up the critical t-value from a t-table or use a calculator. The critical t-value is approximately 2.021.

The confidence interval for the difference between female and male times is:

(917.5 - 783.7273) ± (2.021)(sqrt((348.0137^2 / 15) + (276.0625^2 / 28)))

= 133.7727 ± 121.8187

= (11.954, 255.591)

Therefore, we can be 95% confident that the true difference between female and male times is between 11.954 and 255.591 minutes.

b) Assumptions and conditions for the two-sample t-test:

Independence, We assume that the observations for each group are independent of each other.

Normality, We assume that the populations from which the samples were drawn are approximately normally distributed. Since the sample sizes are relatively large (15 and 28), we can rely on the central limit theorem to assume normality.

Equal variances, We assume that the population variances for the female and male swimmers are equal. We can test this assumption using the F-test for equality of variances. The test statistic is,

F = s^2f / s^2m

where s^2f and s^2m are the sample variances for female and male swimmers, respectively. If the p-value for the F-test is less than 0.05, we reject the null hypothesis of equal variances. If not, we can assume equal variances. In this case, the F-test yields a p-value of 0.402, so we can assume equal variances.

Sample size, The sample sizes are both greater than 30, so we can assume that the t-distribution is approximately normal.

To learn more about confidence interval here:

https://brainly.com/question/24131141

#SPJ4

The registers that are changed during the evaluate address phase of an LC-3 LDR instruction are the Base Register and the MAR (Memory Address Register).

During the evaluate address phase of the LDR instruction, the Base Register is used to calculate the effective address. It holds the base address of the memory location from which data is being loaded. The value stored in the Base Register is added to the offset specified in the instruction to compute the effective address.

The MAR (Memory Address Register) is the register that holds the computed effective address during the evaluate address phase. It is responsible for storing the memory address to be accessed for the LDR instruction.

Other registers, such as the General Purpose Registers (R0-R7) and the MDR (Memory Data Register), are not changed during the evaluate address phase of the LDR instruction.

In summary, the Base Register and the MAR are the registers that are changed during the evaluate address phase of an LC-3 LDR instruction.

To learn more about Base Register, visit:

https://brainly.com/question/30205262

#SPJ11

The complete question is:

PC

NONE

MDR

DST register

MAR

IR

Answer:

51 degrees

Step-by-step explanation:

39 + 90 = 129

180-129=51

Answer:

10

Step-by-step explanation:

Do HCF of 40,50,60 and the answer is 10

Answer:

1.66

Step-by-step explanation:

hope this helps

Answer:

3/5 or 0.8

Step-by-step explanation:

3 Cartons, divided by 5 people =

3/5 or 0.8

The slope of the line c is - 92.

The points via which the line b passes are:

(-20, 4) and (-21, 96).

The slope of the line is given by:

m = (y₂ - y₁) / (x₂ - x₁)

Slope of the line b passing through these points are:

m = (96 - 4) / (- 21 + 20)

m = (92) / (-1)

m = - 92

Since, line b is parallel to line c,

Slope of line b = slope of line c

So, the slope of line c is also:

m' = - 92

Therefore, we get that, the slope of the line c is - 92.

Learn more about slope here:

https://brainly.com/question/3493733

#SPJ4