find the slope
(8, 10), (-7, 14)
Answers
Answer:
4/-15 is the slope
Step-by-step explanation:
Related Questions
The selling price of a fan excluding VAT is Rs 1250. It is given that the VAT rate is 15 %. Find the price of the fan inclusive of VAT.
Answers
Answer:
Rs 1,437.5
Step-by-step explanation:
VAT = 15% = 15/100 × Rs 1250 = Rs 187.5
the price inclusive of VAT = Rs 1,250 + Rs 187.5
= Rs 1,437.5
Graph the given data set and describe what kind of model best describes the data. Then write a function that models
the data.
X y
-2,-1
-1,-2
0,-1
1,2
2,7
Answers
The data appears to follow a quadratic curve, and a quadratic model would best describe the data. The quadratic function that models the data is y = 2x^2 - x - 1.
To graph the given data set, we can plot the points on a coordinate plane as follows:
|
8 |
7 | ●
6 |
5 |
4 |
3 |
2 | ●
1 | ●
0 | ●
-1 | ●
-2 | ●
|_____________
-2 -1 0 1 2
From the graph, we can see that the data appears to follow a quadratic curve. Therefore, a quadratic model would best describe the data.
To write a quadratic function that models the data, we can use the standard form of a quadratic equation:
y = ax^2 + bx + c
where a, b, and c are constants to be determined.
To find the values of a, b, and c, we can use the data points and solve the resulting system of equations
-1 = 4a - 2b + c
-2 = a - 2b + c
-1 = c
2 = 4a + 2b + c
7 = 4a + 8b + c
Solving the system of equations, we get:
a = 2
b = -1
c = -1
Therefore, the function that models the data is:
y = 2x^2 - x - 1
suppose that $f(x)$ is a polynomial that has degree $6$ and $g(x)$ is a polynomial that has degree $3$. if $h(x)$ is also a polynomial such that $f(g(x)) g(h(x)) h(f(x))$ is a polynomial of degree $36$, then what is the degree of the polynomial $h$?
Answers
The resulting polynomial will have a degree of is \($g(h(x))$\)a polynomial that results from substituting \($h(x)$ into $g(x)$.\)\($(\text{degree of } h(x)) \times 6$.\)
To determine the degree of the polynomial $h(x)$, we need to analyze the degree of the composite polynomial \($f(g(x))g(h(x))h(f(x))$.\)
Let's break down the composite polynomial:
$f(g(x))$ is a polynomial that results from substituting $g(x)$ into $f(x)$. Since $g(x)$ is a polynomial of degree $3$ when substituted into $f(x)$ of degree $6$, the resulting polynomial will have a degree of \($6 \times 3 = 18$.\)
$g(h(x))$ is a polynomial that results from substituting $h(x)$ into $g(x)$. Since $h(x)$ is a polynomial of unknown degree when substituted into $g(x)$ of degree $3$, the resulting polynomial will have a degree of \($3 \times (\text{degree of } h(x))$.\)
$h(f(x))$ is a polynomial that results from substituting $f(x)$ into $h(x)$. Since $f(x)$ is a polynomial of degree $6$ when substituted into $h(x)$ of unknown degree, The resulting polynomial will have a degree of
\($(\text{degree of } h(x)) \times 6$.\)
To know more about degree of the polynomial:- brainly.com/question/31437595
#SPJ11
The spacecraft is designed to leave the surface of Mars with the first stage of its propulsion system and be put into Martian orbit. Then, the second stage is used to boost the spacecraft from Martian orbit into an interplanetary trajectory and return to Earth. If the spacecraft is in Martian orbit at an altitude of 384 km, what is the velocity required to escape the gravitational attraction of Mars. Give your answer in km/s. Note that the velocity direction and magnitude required to actually return to Earth may be different.
Answers
The velocity required to escape the gravitational attraction of Mars is 5.03 km/s.
To calculate the velocity required to escape the gravitational attraction of Mars, we need to find the escape velocity at the given altitude. The formula for escape velocity is:
ve = sqrt((2 * G * M) / r)
where ve is the escape velocity, G is the gravitational constant (approximately 6.674 × 10^-20 km³/kg·s²), M is the mass of Mars (approximately 6.417 × 10^23 kg), and r is the distance from the center of Mars to the spacecraft.
Given that the spacecraft is at an altitude of 384 km, we first need to add this to the radius of Mars (approximately 3,390 km) to get the distance r:
r = 3,390 km + 384 km = 3,774 km
Now we can plug these values into the escape velocity formula:
ve = sqrt((2 * 6.674 × 10^-20 km³/kg·s² * 6.417 × 10^23 kg) / 3,774 km)
ve ≈ 5.03 km/s
So, the velocity required to escape the gravitational attraction of Mars at an altitude of 384 km is approximately 5.03 km/s. Keep in mind that the velocity direction and magnitude required to actually return to Earth may be different.
For more such questions on Escape velocity.
https://brainly.com/question/14297933#
#SPJ11
Plz help !!!! Failing class plz help!!

Answers
Answer:
that is alot I would help you but I don't know how to do it
Step-by-step explanation:
Help me please!!!!!!!!!!!!!

Answers
1/3
it is going to be smaller since its a dilation so its obviously neither of the whole numbers, and its not half of the originial one, its a little bit larger than half, its 1/3
option a is correct
I will give u brainliest and 5 star and thanks if its correct

Answers
Answer:
The answer is D.5(9a-2b)
Step-by-step explanation:
Answer:
D
Step-by-step explanation:
5x9a -5x2b= 45a - 10b
so that is the answer
43. Which of the following statement is true? a. The t distribution is positively skewed b. The t distribution is negatively skewed c. The t distribution is symmetrical d. All the values of the t distribution are positive
Answers
Therefore, the correct statement is that the t distribution is Symmetrical (option c).
The correct statement is:
c. The t distribution is symmetrical.
The t distribution, also known as the Student's t distribution, is a probability distribution that is used in statistical inference when the sample size is small or when the population standard deviation is unknown. It is similar to the normal distribution but has slightly thicker tails.
The t distribution is symmetrical, meaning it has a bell-shaped curve that is centered around its mean. The symmetry of the t distribution is a fundamental property that allows for the calculation of confidence intervals and hypothesis tests in statistical analysis.
Unlike the normal distribution, the t distribution has more probability in the tails, which means it has fatter tails. This indicates that extreme values are more likely to occur in the t distribution compared to the normal distribution when dealing with small sample sizes. As the sample size increases, the t distribution approaches the shape of the normal distribution.
Regarding options a, b, and d:
a. The t distribution is not positively skewed. Skewness refers to the asymmetry of a distribution, and the t distribution is symmetric.
b. The t distribution is not negatively skewed. Again, skewness does not apply to the t distribution as it is symmetric.
d. All the values of the t distribution are not positive. The t distribution takes both positive and negative values since it represents the distribution of t-statistics, which can be positive or negative depending on the sample data and the hypothesis being tested.
Therefore, the correct statement is that the t distribution is symmetrical (option c).
For more questions on Symmetrical .
https://brainly.com/question/29545496
#SPJ8
the base of a solid is a right trianglewhose base side has length a and whose perpendicular side has length 1/2a. a. Find the volume of the solid if cross sections perpendicular to the base of the triangle are semicircles.
Answers
The volume of the solid is (1/24)\(\pi a^{3}\)
To find the volume of the solid, we can consider the cross sections perpendicular to the base. Since these cross sections are semicircles, we can use integration to calculate the volume.
Let's denote the variable for the perpendicular side of the triangle as y, which ranges from 0 to (1/2)a. For each y, the corresponding cross section is a semicircle with radius y. The area of each cross section is (1/2)πy^2.
To find the volume, we integrate the area of the cross sections over the range of y:
V = ∫[(1/2)\(\pi y^{2}\)]dy from 0 to (1/2)a
Evaluating the integral, we get:
V = (1/24)\(\pi a^{3}\)
learn more about sections perpendicular here:
https://brainly.com/question/28981602
#SPJ11
Which is not a combination?
(why am i so stuck on this omg)
A. choosing 3 toppings for your pizza
B. lining 3 students up in a row
C. choosing 2 desserts from a tray of 10
D. choosing 5 students to represent a class of 30
Answers
Answer: B
Step-by-step explanation: because
There are two coins in a bin. When one of them is flipped it lands on heads with probability 0.6 and when the other is flipped, it lands on heads with probability 0.3. One of these coins is to be chosen at random and then flipped. a) What is the probability that the coin lands on heads? b) The coin lands on heads. What is the probability that the chosen coin was the one that lands on heads with probability 0.6?
Answers
The probability that the coin lands on heads if one of them is flipped and lands on heads with probability 0.6 is 0.6 × 1/2 + 0.3 × 1/2 = 0.45. Therefore, the probability that the coin lands on heads is 0.45.
a) Let A be the event that the chosen coin is the one that lands on heads with probability 0.6 and B be the event that the coin lands on heads. Then, the required probability is P(A | B) = P(A and B) / P(B) .
Here, P(A and B) = probability that the chosen coin is the one that lands on heads with probability 0.6 and it actually lands on heads.
Since the probability that the coin lands on heads are 0.45 and the probability that the chosen coin is the one that lands on heads with a probability of 0.6 is 1/2, we have P(A and B) = 0.6 × 1/2 = 0.3. The probability that the coin lands on heads is 0.45.
So, P(B) = probability that the coin lands on heads = 0.45.P(A | B) = P(A and B) / P(B) = 0.3 / 0.45 = 2/3.
Hence, the probability that the chosen coin was the one that lands on heads is 0.6 if the coin lands on heads are 2/3.To learn more about “probability” refer to the: https://brainly.com/question/13604758
#SPJ11
Evaluate the following expression.
(-6)^-2
Answers
Answer:
1/36
Step-by-step explanation:
\((-6)^{-2}\) can be written as:
\(\frac{1}{(-6)^{2} }\) (this is done by the law of indices \(a^{-m}=\frac{1}{a^{m} }\) )
then by simplifying the above expression, we get:
\(\frac{1}{(-6 * -6)} =\frac{1}{36}\)
So the final answer is 1/36 ( \(\frac{1}{36}\) )
Pls help urgent !!!!

Answers
Answer:
I would say B
Calculate the SPEED of a
sprinter who ran 200 meters
in 20 seconds.
Answers
Speed = distance / time
Speed = 200 meters / 20 seconds
Speed = 10 meters / second
1 meter per second = 3.6 km per hour
10 meters per second x 3.6 = 36 km per hour
Answer: 36 kilometers per hour
Step-by-step explanation: Average speed = total distance / total time.
Which statement is true about the polynomial
–10m4n3 + 8m2n6 + 3m4n3 – 2m2n6 – 6m2n6 after it has been fully simplified?
Answers
Answer:
Below.
Step-by-step explanation:
–10m4n3 + 8m2n6 + 3m4n3 – 2m2n6 – 6m2n6
= 8m2n6 – 2m2n6 – 6m2n6 – 10m4n3 + 3m4n3
= 8m2n6 - 8m2n6 – 7m4n3
= –7m4n3.
It is a mononomial.
6.7 problem 7
Suppose P=f(t) is the population (in thousands) of town t years after 1990, and that f(7)=15 and f(12)=25,
(a) Find a formula for f(t) assuming f is exponential in the form ab^t. Use 5 decimal places for a & b: P=f(t)=
(b) Find a formula for f−1(P)=
(c) Evaluate f(45)=
(d) f−1(45)=
Write out sentences to explain the practical meaning of your answers to parts (c) and (d). Consider the seven numbered statements in the list below:
The town's population in 2045 is f(45) people.
The town's population has grown by f(45) people over a 45 year period.
The town's population in 2035 is f(45) people.
The town's population will reach 45,000 people in f−1(45) years after 1990.
The town's population will reach 45,000 people in f−1(45) years from now.
The town's population will reach 45 people in f−1(45) years after 1990.
The town's population in 2035 is f(45) thousand people.
(e) Which statement above explains the meaning of your answer to (c)? (enter the number 1-7 of the correct statement).
(f) Which statement above explains the meaning of your answer to (d)? (enter the number 1-7 of the correct statement).
Answers
So the corresponding answers to these questions are:
\(A) a= 7.33657 \\ b= 1.10757\\B)f(t)^{-1} = log (7.33657)+ t*log(1.10757)\\C)728.10886\\D) 2.86219\)
For the letter a, it will be necessary to calculate the values of a and b, therefore:
\(f(t)=a*b^{t} \\f(7)=15 \\ a*b^{7}=15\\a=\frac{15}{b^{7}} \\f(12)=25\\a= \frac{25}{b^{12}}\\\frac{15}{b^{7}} =\frac{25}{b^{12}}=> b^{5}= 1.66667=> b= 1.10757\\a=\frac{15}{b^{7}}=> a=7.33657\)
So for the letter B we will do the logarithm so we will have:
\(P=f(t)=ab^{t}=(7.33657)(1.10757)^{t} \\f(t)^{-1} = log (7.33657)+ t*log(1.10757)\)
For the letter C we will use the formula given in the statement just substituting the value of t=45:
\(f(t)=ab^{t} \\f(45)=(7.33657)(1.10757)^{45} = 728.10886\)
The formula calculated on the letter B will be useful for the letter D only having to substitute t=45, therefore:
\(f(45)^{-1} = log (7.33657)+ 45*log(1.10757)\\f(45)^{-1} = 2.86219\)
Learn more: brainly.com/question/20838017
9-4(5-x)=3(x-1)-2
what kind of math is this like the name
Answers
Answer:
x = -3
Topics are listed below.
Step-by-step explanation:
Topics of this question include:
Solving equations with variablesDistributive propertyBalancing EquationsSolve:
9 - 4(5 - x) = 3(x - 1) -29 - 20 + x = 3x - 3 - 2-11 + x = 3x - 5-2x = 6x = -3-Chetan K
Answer:
The value of x is 6.
Step-by-step explanation:
Concept :
Here, we will use the below following steps to find a solution using the transposition method:
Step 1 :- we will Identify the variables and constants in the given simple equation.Step 2 :- then we Simplify the equation in LHS and RHS.Step 3 :- Transpose or shift the term on the other side to solve the equation further simplest.Step 4 :- Simplify the equation using arithmetic operation as required that is mentioned in rule 1 or rule 2 of linear equations.Step 5 :- Then the result will be the solution for the given linear equation.\(\begin{gathered}\end{gathered}\)
Finding the value of x.
\(\begin{gathered}\quad\implies{\tt{9 - 4(5 - x)=3(x - 1) - 2 }}\\\\\quad\implies{\tt{9 - (4 \times 5 - 4\times x)=(3 \times x - 3 \times 1) - 2}}\\\\\quad\implies{\tt{9 - (20 - 4x)=(3x - 3) - 2}}\\\\\quad\implies{\tt{9 - 20- 4x=3x - 3- 2}} \\\\\quad\implies{\tt{- 11 - 4x=3x - 5}}\\\\\implies{\tt{- 4x + 3x = - 11 + 5}} \\ \\\implies{\tt{-x = - 6}}\\\\ \implies{\tt{ \cancel{-}\: x = \cancel{-} 6}}\\\\\quad\implies{\tt{x = 6}}\end{gathered}\)
Hence, The value of x is 6.
\(\rule{300}{2.5}\)
How I can answer this question, NO LINKS, if you answer correctly I will give u brainliest!

Answers
Answer:
20
Step-by-step explanation:
\(\frac{-8*10^7}{-4*10^6}\\=2*10\\=20\)
The probability that Tom wins a tennis match is 0.6. What is the probability that Tom loses a tennis match?
Answers
Answer:
0.4
Step-by-step explanation:
1-0.6=0.4???????????????
A coffee company wants a new flavor of Cajun coffee. How many pounds of coffee worth $7 a pound should be added to 30 pounds of coffee worth $2 a pound to get a mixture worth $5 a pound?
Answers
The amount of coffee worth $7 a pound that should be added is given by A = 45 pounds
What is an Equation?Equations are mathematical statements with two algebraic expressions flanking the equals (=) sign on either side.
It demonstrates the equality of the relationship between the expressions printed on the left and right sides.
Coefficients, variables, operators, constants, terms, expressions, and the equal to sign are some of the components of an equation. The "=" sign and terms on both sides must always be present when writing an equation.
Given data ,
Let the equation be represented as A
Now , the value of A is
Let the amount of coffee worth $7 a pound that should be added be A
And , the amount of coffee worth $ 2 is = 30 pounds
Let the cost per pound for the mixture is = $ 5
The total amount of mixture = 5 ( 30 + A )
On simplifying the equation , we get
2 ( 30 ) + 7 ( A ) = 5 ( 30 + A )
60 + 7A = 150 + 5A
Subtracting 5A on both sides , we get
60 + 2A = 150
Subtracting 60 on both sides , we get
2A = 90
Divide by 2 on both sides , we get
A = 45 pounds
Hence , the amount of coffee is 45 pounds
To learn more about equations click :
https://brainly.com/question/19297665
#SPJ9
Define a function in Scheme that
returns True if a matrix (list of lists) is symmetric and returns
False otherwise.
Answers
Here's a Scheme function that checks whether a matrix is symmetric or not:
```scheme
(define (is-symmetric-matrix matrix)
(define (get-element matrix i j)
(if (null? matrix)
#f
(if (= i 0)
(if (null? (car matrix))
#f
(if (= j 0)
(car (car matrix))
(get-element (cdr matrix) i (- j 1))))
(get-element (cdr matrix) (- i 1) j))))
(define (is-matrix-symmetric-helper matrix i j)
(if (null? matrix)
#t
(if (equal? (get-element matrix i j)
(get-element matrix j i))
(is-matrix-symmetric-helper matrix i (+ j 1))
#f)))
(if (null? matrix)
#t
(is-matrix-symmetric-helper matrix 0 0)))
```
The function `is-symmetric-matrix` takes a matrix as an input, which is represented as a list of lists. It uses a helper function called `is-matrix-symmetric-helper` to compare each element of the matrix with its corresponding element in the transposed position. The `get-element` function is used to retrieve the element at position `(i, j)` in the matrix.
The `is-matrix-symmetric-helper` function recursively iterates over the matrix, comparing each element with its transposed element. If any pair of corresponding elements is found to be different, it immediately returns `#f` (False), indicating that the matrix is not symmetric. If it reaches the end of the matrix without finding any differences, it returns `#t` (True), indicating that the matrix is symmetric.
Finally, the main `is-symmetric-matrix` function first checks if the matrix is empty. If it is, it immediately returns `#t` since an empty matrix is considered symmetric. Otherwise, it calls the helper function with the initial indices `(0, 0)` and returns its result.
Learn more about Scheme function: https://brainly.com/question/33167627
#SPJ11
Please could you solve this!!!!
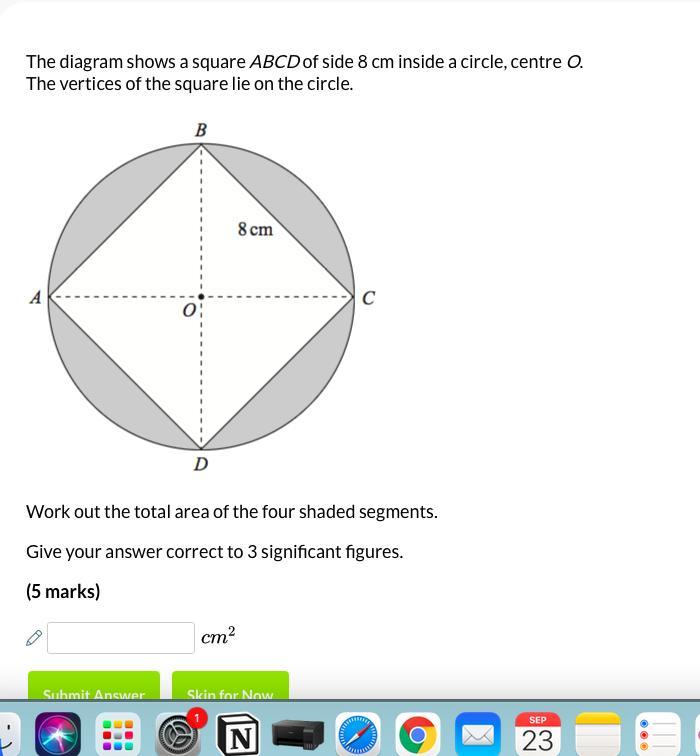
Answers
Answer: no clue
Step-by-step explanation:
DECORATING Steve and Abby purchased a set of vases to place on a 12-foot long mantel above their fireplace. They want to place one vase 1/4 of the distance from one end of the mantel and the other vase 3/4 of the distance from the same end. What is the shortest distance from the end of the mantel should each vase be placed?
Answers
Answer:
Shortest distance of first mantel should be 3 ft from left end and shortest distance of second mantel should be 3 ft from right end
Step-by-step explanation:
Length of the mantel = 12 ft
Since first vase is 1/4 of the distance from one end of the mantel, then let's choose left end;.
Distance of first vase from left end = 1/4 × 12 = 3 ft
Also, distance of second vase from left end = 3/4 × 12 = 9 ft
This second vase is 9 ft from left end. Which means it is 3 ft from right end since the mantel is 12 ft in length.
Thus,shortest distance of first mantel should be 3 ft from left end and shortest distance of second mantel should be 3 ft from right end.
Let W be the subspace spanned by u, and u,. Write y as the sum of a vector in W and a vector orthogonal to W. -11 [1] 4, 4, = 1 , u2 = 1 y= 1 The sum is y=y+z, where y= D is in Wand z= is orthogonal to W
Answers
we have y = [-1, -1, -1] + [-10, 5, 5], where [-1, -1, -1] is in W and [-10, 5, 5] is orthogonal to W.
To write vector y as the sum of a vector in W and a vector orthogonal to W, we need to find the projection of y onto W and then subtract it from y to obtain the orthogonal component.
Let's first find the projection of y onto W. The projection of a vector onto a subspace is given by the formula:
proj_W(y) = (y⋅u / ||u||^2) * u
where ⋅ represents the dot product and ||u|| represents the norm (magnitude) of vector u.
Given the information, we have:
y = [-11, 4, 4]
u = [1, 1, 1]
First, calculate the dot product of y and u:
y⋅u = (-11)(1) + (4)(1) + (4)(1) = -11 + 4 + 4 = -3
Next, calculate the norm squared of u:
||u||^2 = (1^2) + (1^2) + (1^2) = 1 + 1 + 1 = 3
Now, substitute these values into the projection formula:
proj_W(y) = (-3 / 3) * [1, 1, 1]
= [-1, -1, -1]
The projection of y onto W is [-1, -1, -1].
To obtain the orthogonal component z, we subtract the projection from y:
z = y - proj_W(y)
= [-11, 4, 4] - [-1, -1, -1]
= [-11 + 1, 4 + 1, 4 + 1]
= [-10, 5, 5]
Know more about vector here:
https://brainly.com/question/29740341
#SPJ11
Please answer. I will give brainliest

Answers
Answer:
16.5f
Step-by-step explanation:
just know that’s right
the national survey of student engagement found that 24% of seniors report that they often or very often went to class without completing readings or assignments.12 assume that the sample size of seniors is 276,000. (a) find the margin of error for 99% confidence.
Answers
Margin of error for 99% confidence interval is 0.0021
What is confidence interval?
he range of values that, should you repeat your experiment or resample the population in the same manner, you would anticipate your estimate to fall within a particular percentage of the time.
Main Body:
Given :
99% Confidence Interval for p
p = 0.24,n = 276000
Significance level = α = 1− confidence = 1 − 0.99 = 0.01
Critical z-value = zα/2 = z0.01/2 = z0.005 = 2.58 (closest value From z table)
Standard e
or of p : SE =
√p× (1 − p)n=√0.24 × 0.76276000
≈ 0.0008129
E = zα/2 ×√p× (1 − p)n
= 2.58 × 0.0008129 ≈ 0.002097
Hence ,Margin of Error or, E = 0.00210
To know more about confidence interval click on the link below
https://brainly.com/question/17097944
#SPJ4
use a technique of integration or a substitution to find an explicit solution of the given differential equation. ( x x) dy dx = y y
Answers
To find an explicit solution of the given differential equation, we can use separation of variables.
The explicit solution of the differential equation (x^2)dy/dx = (y^2) can be found by rearranging the equation and separating the variables:
\[ \frac{dy}{y^2} = \frac{dx}{x^2} \]
Integrating both sides, we get:
\[ \int \frac{1}{y^2} \, dy = \int \frac{1}{x^2} \, dx \]
This simplifies to:
\[ -\frac{1}{y} = -\frac{1}{x} + C \]
where C is the constant of integration.
To solve the given differential equation (x^2)dy/dx = (y^2), we use the technique of separation of variables. This involves rearranging the equation to separate the variables y and x on different sides of the equation.
We start by dividing both sides of the equation by (y^2):
\[ \frac{dy}{y^2} = \frac{dx}{x^2} \]
Next, we integrate both sides of the equation with respect to their respective variables. The integral of 1/y^2 with respect to y gives us -1/y, and the integral of 1/x^2 with respect to x gives us -1/x. Adding the constant of integration C to account for the antiderivative, we have:
\[ -\frac{1}{y} = -\frac{1}{x} + C \]
This equation represents the general solution to the given differential equation. The constant of integration C can be determined by applying initial conditions if they are given.
know more about explicit solution :brainly.com/question/31684625
#SPJ11
a theater has 30 rows with 27 seats in the first row, 31 in the second row, 35 in the third row, and so forth. How many seats are in the theater
Answers
Answer:
Question relates to a Arithmetic Sequence {30,32,34...} where d = 2
and the terms are the enumber of seats in each row.
S%5Bn%5D=%28n%2F2%29%282%2Aa%5B1%5D%2B+%28n-1%29d%29 |Sum of the seats in the 26 rows.
S%5B26%5D=%2826%2F2%29%282%2A30%2B+%2825%292%29 = 1430 seats in the ALL 26 rows
Step-by-step explanation:
3x+2 < 2x-5 for x=8
How do I do this
Answers
Answer:
3x+2 is not less than 2x-5
Step-by-step explanation:
3(8)+2<2(8)-5; 24+2 < 16-5; 26 < 9
At 6am, the temperature started
at -2°F. At 10am the temperature
increased by 8°. The last recorded
temperature of the day at 4pm
was 11°F. What was the recorded
temperature at 10am?
Answers
Answer:
6°
Step-by-step explanation:
It started at -2°f and increased b 8° so 2 of those degrees get you back to 0°f and the remains is 6 so 6°
Hope this helped :)