find the codomain of f(x) = (x+3) / (x-1)
Answers
Solution
- The question tells us to determine the codomain of the following rational function:
\(f(x)=\frac{x+3}{x-1}\)- The codomain of the function is the possible values that f(x) can take.
; From the function, we can see that:
\(\begin{gathered} f(x)=\frac{x+3}{x-1} \\ \text{when }x=1 \\ f(1)=\frac{1+3}{1-1}=\frac{4}{0} \\ \\ \text{Thus, f(1) leads to an undefined value. As such,} \\ x\ne1 \end{gathered}\)- Thus, we can conclude that the codomain is:
\(undefined\)Related Questions
PLEASE HELP !! ILL GIVE BRAINLIEST *EXTRA POINTS*..
IM GIVING 40 POINTS !! DONT SKIP :((.

Answers
Answer:
m=-3/2
b=3
equation f(x) = -3/2x +3
Proportional
negative slope
Step-by-step explanation:
Parallelogram I is a scaled copy of parallelogram H.
What scale factor takes parallelogram H to parallelogram I?
Answers
1 ÷ 3 is the scale factor that takes parallelogram H to parallelogram I.
If Parallelogram I is a scaled replica of Parallelogram H, then perhaps the figure in which every length in the initial estimate is multiplied by the exact number.
Scale factor = size of new shape ÷ size of the old shape.
The magnification ratio of a parallelogram I is significantly 3 times that of parallelogram H this ratio could be only assumed when the data is not given.
The polygon will be reduced if the magnification ratio would be between 0 and 1. The picture will be magnified if the zoom ratio is larger than one.
The scale factor refers to the ratio of the old thing's scale to the new object's scale. Although the size of these ratios varies, they are useful.
Use negative magnification to enlarge the picture. Whenever the scale factor is negative, the value diverges from the scale.
Read more about scaled copy at
https://brainly.com/question/465048?referrer=searchResults
#SPJ1
The complete question is -
Parallelogram I is a scaled copy of parallelogram H. What scale factor takes parallelogram H to parallelogram I in the image?
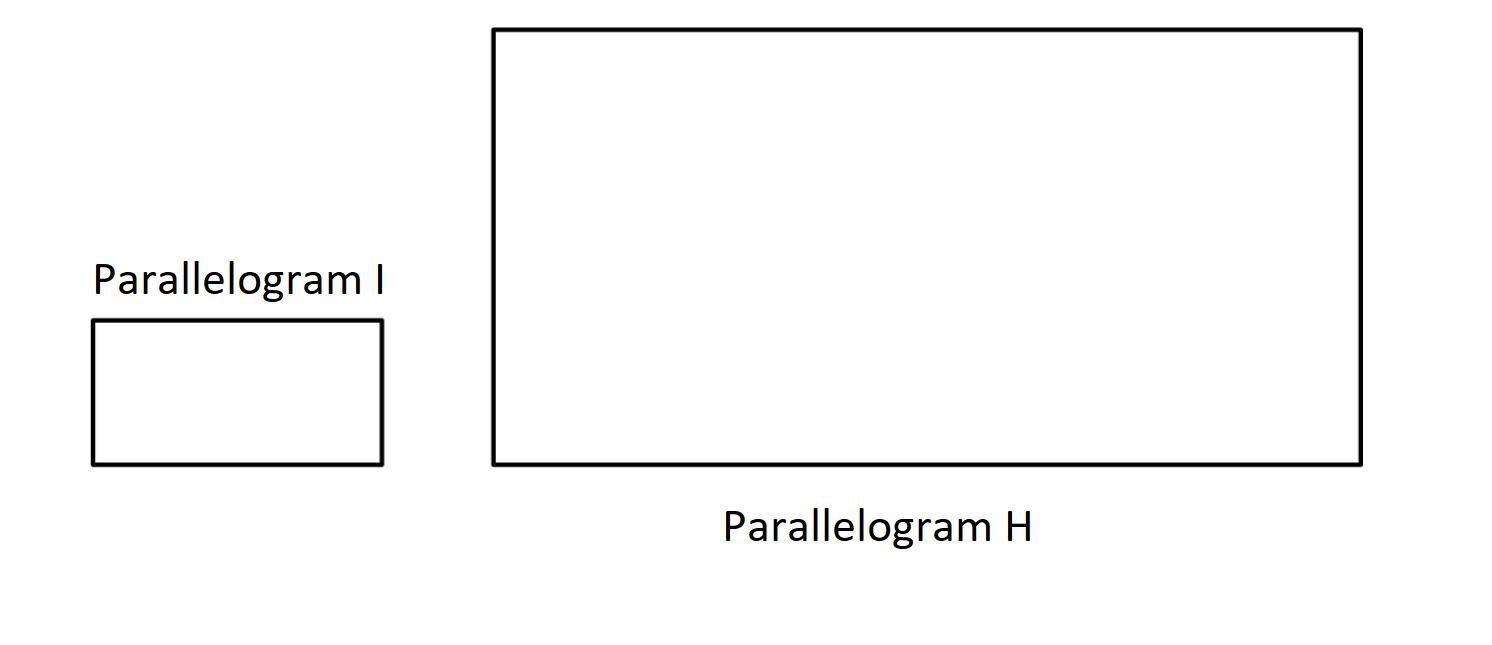
The scaling factor that transforms parallelogram H into parallelogram I is 1/3.
If Parallelogram I is a scaled-down version of Parallelogram H, then it might be the result of multiplying each length in the initial estimate by a precise value.
Scale factor is equal to new shape's size minus old shape's size.
A parallelogram I's magnification ratio is noticeably three times greater than a parallelogram H's. Only in cases where no data is provided may this ratio be assumed.
If the magnification ratio is in the range between 0 and 1, the polygon will be smaller. If the zoom ratio exceeds one, the image will be enlarged. The scale factor is the ratio of the scale of the old thing to the scale of the new thing.These ratios are helpful despite the fact that their sizes differ.
Enlarge the image by applying negative magnification. The value deviates from the scale whenever the scale factor is negative.
To know more about scaled copy refer
https://brainly.com/question/28747162
please help!! need it fast, will give brainliest!! and pls show work !!
Find the measure of angle AEB

Answers
Answer:
An acute angle
Step-by-step explanation:
An acute angle is smaller than an obtuse ad right angle.
hope this helps and hope it was right 'cause I really don't know what you meant. :)
Three friends play a game. Jamila has 4-1/2
more points than Carter. Carter has 7 1/2 more
points than Aisha. Jamila has 26 points. Write
and solve an equation to find the number of
points Aisha has. Show your work.
Answers
The equation is written as
x + 11 = 26Aisha has 15 points
How to write the equationLet's use the given information to set up an equation to represent the relationship between the points each friend has.
Let x be the number of points Aisha has.
Then, according to the problem statement, we know that:
Carter has 7 1/2 more points than Aisha, so he has
x + 7 1/2 points.
Jamila has 4 - 1/2 more points than Carter, so she has
x + 7 1/2 + 4 - 1/2 points,
which simplifies to x + 11 points.
Jamila has 26 points, so we can set x + 11 equal to 26 and solve for x:
x + 11 = 26
x = 26 - 11
x = 15
Therefore, Aisha has 15 points.
Learn more about word problem at
https://brainly.com/question/21405634
#SPJ1
How do I find GBA and show all the work

Answers
Answer:
Angle ACB = 44°
There are two ways to solve it. Both are right
Solution number 1
From triangle ABC
angle BAC = 180°-(102° +44°) = 36°
Because BG is parallel with AC
Then angle GBA = angle BAC = 34°Another solution
The sum of angles in the shape AGBC = 360°
So angle GBC = 360 - (90 + 90 + 44 + 102) = 34°The sum of the squared differences of scores from their mean is zero.
True or False?
Answers
Answer:
False
Step-by-step explanation:
We have several terms that we can take reference to in this true or false situation. The first is Central Tendency which measures for locating a single score that's most representative or descriptive of all scores in a distribution.
Given text:
The sum of the squared differences of scores from their mean is zero.
The main term within this sentence is the word mean. This term is the sum of a set divided by the total (#) of scores summed.
In order to determine whether this is true or false, we must list what is referred to as the 5 characteristics of the term Mean.
1. Changing an existing score will change the mean.
2.Adding a new score or removing an existing score will change the mean, unless that value equals the mean.
3. Adding, subtracting, multiplying, or dividing each score in a distribution by a constant will cause the mean to change by that constant.
4. The sum of the differences of scores from their mean is zero.
5. The sum of the squared differences of scores from their mean is minimal.
After taking closer analysis, there has been clarification within the characteristics of the mean confirming that the answer is indeed false.
#SPJ1
Find and prove an inequality relating 100n and n^{3} .
Answers
An inequality relating 100n and n³ is 100n ≥ n³ for n ≤ 10 and 100n ≤ n³ for n ≥ 10.
What is inequality?An inequality is comparison of two values, showing if one is less than, greater than, or simply not equal to another value.
Since 100n and n³ for n = 1, 2, 3, . . . 9, 10, 11 are 100, 200, 300, . . . 900, 1000, 1100 and 1, 8, 27, . . . 729, 1000, 1331 respectively.
Therefore, an inequality relating 100n and n³ will be 100n ≥ n³ for n ≤ 10 and 100n ≤ n³ for n ≥ 10.
Induction hypothesis:
Suppose 100n ≤ k³ for some positive integer k ≥ 10.
We need to show that 100( k + 1 ) ≤ ( k + 1 )³ = k³ + 3k² +3k + 1.
Note 100( k + 1 ) = 100k + 100 ≤ k³ + 100
≤ k³ + 3k² (∵ k ≥ 10 )
≤ k³ + 3k² + 3k
≤ k³ + 3k²+3k + 1
So 100( k + 1 ) ≤ ( k + 1 )³, which is true.
Hence by the principle of mathematical induction, 100n ≤ k³ for every integer k ≥ 10.
Know more about inequality here:
https://brainly.com/question/20383699
#SPJ1
Please show how to factor this 3x^2 -10x-8. \(3x^{2}-10x-8\)
Answers
Answer:
(x-4)(3x+2)
Step-by-step explanation:
Answer:
\((3x+2)(x-4)\)
Step-by-step explanation:
Something to keep in mind is that the factored expression will be in either the form of \((ax-b)(x-c)\) or \((ax-b)(cx-d)\). In either case, the final term will only be the product of -b and -c in the first case or -b and -d in the second case. This means that the term without an x will not be affected by the coefficient of x.
We have the following expression
\(3x^2-10x-8\)
The first thing we can do is list out the factors of -8
1 and -8
-1 and 8
2 and -4
-2 and 4
These are all of the pairs that could be factors of -8.
Normally, the next step would be to look for which of these factors would add together to give you the coefficient of x, but as there is a 3\(x^2\), this is complicated a little more.
Instead, we have to look for 3 multiplied by one of the factors and then added to the other factor. In the case of this problem, the result must be -10.
At this point, you just have to begin testing out which ones will work.
Let us look at the factors 2 and -4
\(2(3)-4=2\\\\2-4(3)=-10\)
We can see that while the first one didn't work, the second one resulted in -10, which is what we wanted.
Now that we know what the factors are, let us write them in the form\((ax-b)(x-c)\)
Normally, it does matter which number you pair with each x, but in this case, it does matter. As we had to multiply the -4 by 3, it must be in the pair that is opposite to the pair with the 3x.
Knowing this, we can construct out factor
\((3x+2)(x-4)\)
(Sorry that this explanation was quite lengthy)
what is the value of the expression below (8^1/2)^2
Answers
Answer:
8
Step-by-step explanation:
The square of a number square rooted is the number itself.
A bakery sells mocha cupcakes and vanilla cupcakes. It sold 160 cupcakes in a day, and 65% of them were vanilla. How many of the cupcakes sold that day were mocha?
Answers
0.65x160= 104 vanilla
160-104=56 mocha
The formula of calculating the gradient of a line is
Answers
Answer:
To calculate the gradient of a straight line we choose two points on the line itself. From these two points we calculate: The difference in height (y co-ordinates) ÷ The difference in width (x co-ordinates). If the answer is a positive value then the line is uphill in direction.
Determine the equation of the circle graphed below.
y
10
8
6
o
2
-10
-8
-6
4
-2
4
6
8
10
-2
-6
-10

Answers
Answer:
(x-7)²+(y-4)²=3²Step-by-step explanation:
to understand thisyou need to know about:circle equationPEMDAStips and formulas:circle equation standard form: (x-a)²+(y-b)²=r²given:(a,b)=(7,4)r=3let's solve:substitute the values of a,b and r :(x-7)²+(y-4)²=3³and
we are done

The equation of a circle is a measure of its center and its radius.
The equation of the circle is: \(x^2 + y^2 - 14x -8y + 56 = 0\)
The general equation of a circle is \((x - a)^2 + (y - b)^2 + r^2\). Where:
\((a,b) \to\) center
\(r \to\) radius
From the given figure, we have:
\(r = 3\)
\((a,b) = (7,4)\)
So, the equation of the circle is:
\((x - a)^2 + (y - b)^2 + r^2\)
\((x - 7)^2 + (y - 4)^2 = 3^2\)
\((x - 7)^2 + (y - 4)^2 = 9\)
Open brackets
\(x^2 - 14x + 49 + y^2 -8y + 16 = 9\)
Collect like terms
\(x^2 + y^2 - 14x -8y + 49 + 16 - 9 = 0\)
\(x^2 + y^2 - 14x -8y + 56 = 0\)
Hence, the equation of the given circle is: \(x^2 + y^2 - 14x -8y + 56 = 0\)
Read more about equations of circles at:
https://brainly.com/question/23988015
50 Points!!!!!! Triangle ABC is given.
Angle A is labeled two x degrees. Angle B is labeled forty degrees. Angle C is labeled three x degrees.
What is the measure, in degrees, of ∠A?
Enter your answer in the box.

Answers
All 3 angles add up to 180 degrees.
so 40 + 2x + 3x = 180
40 + 5x = 180
5x = 140
x = 28
So angle A = 2x = 2(28) = 56 degrees.
Answer: 56°
Step-by-step explanation:
We know that a triangle's angles add up to 180 degrees. We will create an equation to help us solve for x using this information.
Given:
2x + 3x + 40 = 180
Combine like terms:
5x + 40 = 180
Subtract 40 from both sides of the equation:
5x = 140
Divide both sides of the equation by 5:
x = 28
Next, we will substitute this value of x into the expression for ∠A.
∠A = 2x = 2(28) = 56°
Plz I will give brainliest
Answers
Answer:
I'm so confused
Step-by-step explanation:
did you forget to add a picture
The vertices of triangle ABC are given: A (0;-1) B(12; -10) C(10;4)
Find:
a) the equation of the median and bisector drawn from vertex A;
b) the equation of the altitude from vertex B;
c) the point of intersection of the median and height;
d) the coordinates of the center of mass (the point of intersection of the medians).
Answers
verified
Verified by Toppr
Median bisects the line.
D≡(
2
−3+5
,
2
−9−8
)≡(1,
2
−17
)
E≡(−
2
1+5
,
2
6−8
)≡(2,−1)
F≡(−
2
1−3
,
2
6−9
)≡(−2,
2
−3
)
∴ equation of AD:(y−y
1
)=(
x
2
−x
1
y
2
−y
1
)
AD
(x−x
1
)
⇒(y−6)=
⎝
⎜
⎜
⎛
1+1
2
−17
−6
⎠
⎟
⎟
⎞
(x+1)⇒(y−6)=−
4
29
(x+1)
⇒
4
29
x+y−6+
4
29
=0
AD⇒29x+4y+5=0
equation of BE:(y+9)=(−
2+3
1+9
)
BE
(x+3)
⇒(y+9)=
5
8
(x+3)⇒5y+45=8x+24
BE⇒8x−5y−21=0
Equation of CF:(y+8)=
⎝
⎜
⎜
⎛
−2−5
−
2
3
+8
⎠
⎟
⎟
⎞
CF
(x−5)
⇒(y+8)=−
14
13
(x−5)⇒14y+112=−13x+65
CF:13x+14y+47=0
Given the following probabilities, determine whether the events A and B are
independent or dependent.
P(A) = 2/3
P(B) = 1/4
P(A and B)= 2/7
Answers
If P(A)= \(\frac{2}{3}\), P(B) = \(\frac{1}{4}\) and P(A and B)= \(\frac{2}{7}\), then the events A and B are dependent.
By comparing the probability of events A and B intersection (P(A and B)) to the product of their individual probabilities (P(A) * P(B)), we can find out whether the events are independent or dependent.
Condition of independent events,
If P(A) * P(B) = P(A and B)
Condition of dependent events,
If P(A) * P(B) ≠ P(A and B)
Given,
P(A) = \(\frac{2}{3}\)
P(B) = \(\frac{1}{4}\)
P(A and B) = \(\frac{2}{7}\)
Calculating P(A) * P(B) and compare it to P(A and B):
P(A) * P(B) = \(\frac{2}{3}\) * \(\frac{1}{4}\) = \(\frac{2}{12}\) = \(\frac{1}{6}\)
Since P(A and B) ≠ P(A) * P(B), therefore events A and B are dependent.
To know more about dependent and independent events,
https://brainly.com/question/1374659
https://brainly.com/question/30239562
PLEASE HELP!! a bag contains 10 marbles. four of them are red, three blue, two white, and one yellow. A mare ke drawn at random. What is the probability that it is blue?

Answers
Answer:
The probability that one marble drawn at random is not blue is 70%
Suppose you want to have $400,000 for retirement in 25 years. Your account earns 4% interest.
a) How much would you need to deposit in the account each month?
$
b) How much interest will you earn?
$
Answers
\(~~~~~~~~~~~~\stackrel{\textit{payments at the beginning of the period}}{\textit{Future Value of an annuity due}} \\\\ A=pmt\left[ \cfrac{\left( 1+\frac{r}{n} \right)^{nt}-1}{\frac{r}{n}} \right]\left(1+\frac{r}{n}\right)\)
\(\qquad \begin{cases} A=\textit{accumulated amount}\dotfill&\$400000 \\ pmt=\textit{periodic payments}\\ r=rate\to 4\%\to \frac{4}{100}\dotfill &0.04\\ n= \begin{array}{llll} \textit{times it compounds per year}\\ \textit{monthly, thus twelve} \end{array}\dotfill &12\\ t=years\dotfill &25 \end{cases}\)
\(400000=pmt\left[ \cfrac{\left( 1+\frac{0.04}{12} \right)^{12 \cdot 25}-1}{\frac{0.04}{12}} \right]\left(1+\frac{0.04}{12}\right)\)
\(\cfrac{400000}{\left[ \frac{\left( 1+\frac{0.04}{12} \right)^{12 \cdot 25}-1}{\frac{0.04}{12}} \right]\left(1+\frac{0.04}{12}\right)}=pmt\implies \cfrac{400000}{\left[ \frac{\left( \frac{301}{300} \right)^{300}-1}{\frac{1}{300}} \right]\left(\frac{301}{300}\right)}=pmt \\\\\\ \cfrac{400000}{515.84}\approx pmt\implies {\Large \begin{array}{llll} 775.43\approx pmt \end{array}}\)
how much will it be in interest alone?
well, for 25 years every month you'd have been putting down that much, so we can just subtract what you put it from the 400,000 and what's left is the interest earned
\(400000~~ - ~~(25)(12)(775.43) ~~ \approx ~~ \text{\LARGE 167317}\)
Identify all the two-dimensional figures that are cross-sections for each of the three-dimensional solids.
Cube
pentagon
square
triangle
rectangle
octogon
hexagon
trapezoid
Answers
Answer:
square, triangle, trapezoid,rectangle
The Two-dimensional figures are square, triangle, rectangle, and trapezoid.
What are two-dimensional figures?Two-dimensional shapes do not have any thickness and can be measured on only two faces. These can be drawn on a sheet of paper with the constitution of two axises only.
The Two-dimensional figures that are cross-sections for each of the three-dimensional solids are:
squaretrianglerectangletrapezoidLearn more about two-dimensional, here:
https://brainly.com/question/1211190
#SPJ2
Ms. Sanchez's guests drank 534534 gallons of pink lemonade in 1414 hour. What was the average rate in gallons per hour which her guests drank the pink lemonade?
Answers
Scores on the GRE (graduate record examination) are normally distributed with a mean of 553 and a standard deviation of 112. Use the 68-95-99.7 rule to find
the percentage of people taking the test who score between 421 and 645
The percentages of people taking the test who scores between 421 and 645 is__%
Answers
The percentages of people taking the test who scores between 421 and 645 is: 68 %
How to use the empirical rule of statistics?The empirical rule, also referred to as the three-sigma rule or the 68-95-99.7 rule, is defined as a statistical rule which states that almost all observed data for a normal distribution will fall within three standard deviations (denoted by σ) of the mean or average (denoted by µ).
We are given:
Sample mean: x' = 553
standard deviation; s = 112
Thus:
x' - ns = 421
x' + ns = 645
Where n is the number of standard deviations from the mean. Thus:
553 - 112s = 421
112s = 553 - 421
112s = 132
s = 132/112
s ≈ 1
So 1 standard deviation from the means indicates a percentage of 68%
Read more about empirical rule of statistics at: https://brainly.com/question/10093236
#SPJ1
PLEASE HELP???!!! Look at the attachment below

Answers
Answer:
7. 5
6. 0
Step-by-step explanation:
Answer:
See attachedStep-by-step explanation:
Using the numbers in the Venn diagram we get answers:
Those have a dog and a cat: 5 + 7 = 12Those have a dog but don't have a cat: 5Those don't have a dog: 6Those don't have a dog or cat: 0
Here are the heights (in inches) of 12 students in a seminar. 71, 67, 62, 60, 70, 64, 68, 72, 58, 63, 60, 66 What is the percentage of these students who are shorter than 65 inches? 1% X 5
Answers
25% of the students in the seminar are shorter than 65 inches.
To find the percentage of students who are shorter than 65 inches, we first need to find the number of students whose height is less than 65 inches:
There are three students who are shorter than 65 inches: 62, 60, and 58.
Therefore, the percentage of students who are shorter than 65 inches is:
(3 students / 12 students) × 100% = 25%
Note that the value given for 1% × 5 does not appear to be relevant to this question, and is not necessary for the calculation of the percentage of students who are shorter than 65 inches.
For such more questions inches
https://brainly.com/question/876758
#SPJ8
Sally made a profit of $2500 after selling stocks for $19000 after 2.5 years. What was her average annual percentage gain?
13.25%
6.06%
3.78%
Answers
Sally's average annual percentage gain is approximately 5.26%.
To calculate Sally's average annual percentage gain, we can use the formula:
Average Annual Percentage Gain = (Profit / Initial Investment) * (1 / Time) * 100
Profit = $2500
Initial Investment = $19000
Time = 2.5 years
Substituting the values into the formula:
Average Annual Percentage Gain = (2500 / 19000) * (1 / 2.5) * 100
= (0.1316) * (0.4) * 100
= 5.26
Therefore, Sally's average annual percentage gain is approximately 5.26%.
for such more question on average
https://brainly.com/question/130657
#SPJ8
Sean arrives at quater to 7 dinner starts at 6:30 is sean early or late for dinner
Answers
Answer:
Sean is late for dinner.
Step-by-step explanation:
A quarter to 7 is 6:45 so he is 15 minutes late to the dinner.
If Sean arrives at quarter to 7 (which means 6:45), he is 15 minutes late for dinner, as dinner started at 6:30.
What is time estimation?When we say that Sean arrived at quarter to 7, it means that he arrived at 6:45, because a quarter of an hour is equivalent to 15 minutes. We can then compare this time to the time that dinner started, which is 6:30.
If Sean arrived at or before 6:30, he would be considered early for dinner. However, since he arrived at 6:45, he is 15 minutes late for dinner. This means that dinner has already started and he has missed the beginning of the meal.
In general, it is considered polite and respectful to arrive on time for a dinner invitation or any other social event. If you are going to be late, it's always a good idea to let your host know so they can adjust their plans accordingly.
To know more about time estimation follow
https://brainly.com/question/8476295
#SPJ2
2
5. Many people believe that criminals who plead guilty tend to get lighter sentences than those
who are convicted in trials. The accompanying table summarizes randomly selected sample
data for defendants in burglary cases in a specific city. All of the subjects had prior prison
sentences. Use a 0.05 significance level to find the critical value needed to test the claim that
the sentence (sent to prison or not sent to prison) is independent of the plea.
Sent to prison
Not sent to prison
Guilty Plea
392
564
Not-Guilty Plea
58
14
(1 point)09.488
03.841
042.557
05.991
Answers
Answer:
Is 03.841
Step-by-step explanation:
To find the critical value needed to test the claim that the sentence is independent of the plea, we need to perform a chi-square test of independence. The critical value is based on the significance level (α) and the degrees of freedom.
In this case, the given significance level is 0.05. Since the table represents a 2x2 contingency table (two categories for plea and two categories for sentence), the degrees of freedom (df) can be calculated as (number of rows - 1) * (number of columns - 1) = (2 - 1) * (2 - 1) = 1.
To find the critical value at a significance level of 0.05 with 1 degree of freedom, we consult a chi-square distribution table or use statistical software.
The critical value for a chi-square test with 1 degree of freedom and a significance level of 0.05 is approximately 3.841.
Therefore, the correct answer is 03.841.
Franklin deposited $75 in a no-interest bank account when he opened it. After that, he deposits $50 per month in the account. Assume he makes no other withdrawals or deposits. The equation below can be used to find m , the number of months it will take him to save a total of $175.
75 + ?m = 175
What number should he use as the coefficient of m?
50
75
125
175
Answers
Answer:
50
Step-by-step explanation:
The coefficient is amount of money in m (months)
The 50 is the amount of money
They are depositing $50 per month, 50m
Therefore, the coefficient is 50.
Answer:
50
Step-by-step explanation:
Find the derivative of the function using the definition of derivative. State the domain of the function and the domain of its derivative. 19. f(x) = 3x - 8
23. f(x) = x² - 2x³
25. g(x) = √9 - x
27. G(t) = 1 - 2t/3+1
Answers
The derivatives of the functions are now listed:
19) f'(x) = 3
23) f'(x) = 2 · x - 6 · x²
25) g'(x) = - 1 / [2 ·√(9 - x)]
27) g'(t) = - 2 / 3
How to find the derivative of a function
In this case we have four functions, whose first derivatives must be found by means of derivative rule. Now we present the result of each expression along with the corresponding derivative rules:
19) f(x) = 3 · x - 8 → f'(x) = 3 Differentiation for the addition of two functions / Derivative of a power / Derivative of the product of a power and a constant / Derivative of a constant
23) f(x) = x² - 2 · x³ → f'(x) = 2 · x - 6 · x² Differentiation for the subtraction of two functions / Derivative of a power / Derivative of the product of a power and a constant
25) g(x) = √(9 - x) → g'(x) = - 1 / [2 ·√(9 - x)] Differentiation of the square root / Chain rule
27) g(t) = 1 - 2 · t / 3 + 1 → g'(t) = - 2 / 3 Differentiation for the addition of two functions / Derivative of a power / Derivative of the product of a power and a constant / Derivative of a constant
To learn more on derivative rules: https://brainly.com/question/25324584
#SPJ1
At the local Theatre of the Arts, tickets cost $4 for children and $5 for adults. In the opening Saturday night of a play, the theater made $540. The second day was a matinee and the prices were lower for children at $3 and the same price as Saturday for adults. They made $440 at the matinee.
A) Write a system of equations in standard form that represents the prices at the Theatre on Saturday and the second day.
B) Rewrite the system of equations in slope-intercept form. What are the y-intercepts of both equations?
Answers
A. The system of equations in standard form is: 4x + 5y = 540 and 3x + 5y = 440.
B. The y-intercept of the equation representing the prices on Saturday night is 108, and the y-intercept of the equation representing the prices at the matinee on the second day is 88.
A) Let's define the variables:
Let x represent the number of children attending.
Let y represent the number of adults attending.
On Saturday night:
The equation for the revenue generated on Saturday night is:
4x + 5y = 540 (since children's tickets cost $4 and adults' tickets cost $5, and the total revenue is $540).
Matinee on the second day:
The equation for the revenue generated at the matinee is:
3x + 5y = 440 (since children's tickets cost $3 and adults' tickets still cost $5, and the total revenue is $440).
Therefore, the system of equations in standard form is:
4x + 5y = 540
3x + 5y = 440
B) Let's rewrite the system of equations in slope-intercept form:
On Saturday night:
4x + 5y = 540
Rearranging the equation, we get:
5y = -4x + 540
Dividing both sides by 5, we get:
y = (-4/5)x + 108
The y-intercept of this equation is 108.
Matinee on the second day:
3x + 5y = 440
Rearranging the equation, we get:
5y = -3x + 440
Dividing both sides by 5, we get:
y = (-3/5)x + 88
The y-intercept of this equation is 88.
For such more question on equation:
https://brainly.com/question/17145398
#SPJ8
What is the quotient?
3
4
Answers
Answer:
0.75
Step-by-step explanation:
it makes sense that 3 is the Dividend, 4 is the Divisor, and you want to know the Quotient. Thus, the answer is:
3 ÷ 4 = 0.75