find the area of the part of the surface z=x^2 (\sqrt{3})y z=x 2 ( 3 )y that lies above the triangle with vertices (0,0),(1,0)(0,0),(1,0), and (1,2)(1,2).
Answers
The given surface is z = x²√3y + x2(3)y. The triangle has vertices at (0,0), (1,0), and (1,2).Let's graph the surface and unitary triangle:
Graph of surface z = x²√3y + x2(3)yGraph of triangle with vertices (0,0), (1,0), and (1,2)From the graph, we can see that the surface intersects the triangle along the lines x = 0, y = 0, and y = 2 - x. Therefore, we can set up a double integral for the area of the part of the surface that lies above the triangle:∬R z = x²√3y + x2(3)y dA, where R is the region enclosed by the triangle.
Using the limits of integration, the integral becomes∫₀¹ ∫₀^(2-x) x²√3y + x2(3)y dy dxThe inner integral with respect to y is∫₀^(2-x) x²√3y + x2(3)y dy = [x²(√3/2)y² + x²y³]₀^(2-x)= x²(√3/2)(2-x)² + x²(2-x)³= x²(2 - x)²(√3/2 + 2x)The outer integral with respect to x is∫₀¹ x²(2 - x)²(√3/2 + 2x) dxWe can expand the (2 - x)² term, and then use polynomial integration to evaluate the integral:∫₀¹ x²(2 - x)²(√3/2 + 2x) dx= ∫₀¹ (√3/2)x²(2 - x)² dx + ∫₀¹ 4x²(2 - x)³ dx= (√3/2) ∫₀¹ x²(4 - 4x + x²) dx + 4 ∫₀¹ x²(8 - 12x + 6x² - x³) dx= (√3/2) [4/3 - 2 + 1/3] + 4 [8/3 - 6/2 + 3/3 - 1/4]= (8/3)√3 - (14/3) ≈ 0.7714Therefore, the area of the part of the surface z = x²√3y + x2(3)y that lies above the triangle with vertices (0,0), (1,0), and (1,2) is approximately 0.7714.
To know more about unitary method visit:
https://brainly.com/question/28276953
#SPJ11
Related Questions
[(p implies q)and(q implies r)]implies(p implies r) in descrete mathematics problem
Answers
The statement [(p implies q) and (q implies r)] implies (p implies r) in discrete mathematics.
In discrete mathematics, implication is represented by the conditional operator (=>). The given statement [(p implies q) and (q implies r)] can be written as (p => q) ∧ (q => r). We want to prove that this statement implies (p => r).
To demonstrate this, we need to show that whenever the statement [(p => q) ∧ (q => r)] is true, the implication (p => r) is also true. This can be done using a truth table or logical reasoning.
If (p => q) and (q => r) are both true, it means that whenever p is true, q must also be true, and whenever q is true, r must also be true. In this case, if p is true, then q is true, and as q is true, r must also be true. Therefore, (p => r) is true.
Hence, we can conclude that the statement [(p implies q) and (q implies r)] implies (p implies r) in discrete mathematics.
Learn more about discrete mathematics click here :brainly.com/question/27247288
#SPJ11
Draw the Region, the axis of revolution, specify the method, state the formula, solve
Answers
The volume of the solid of revolution created by rotating the function f(x) = x^2 about the x-axis between x=0 and x=2 is approximately 20.106 cubic units.
Figure out the axis of revolution and specify the method?The axis of revolution is a line about which a two-dimensional shape is rotated to create a three-dimensional solid. The method for finding the formula to solve for the volume of a solid of revolution depends on the shape being rotated and the axis of revolution.
For example, if we want to find the volume of a solid of revolution created by rotating a function f(x) about the x-axis between the limits of integration a and b, we can use the following formula:
V = π∫[a,b] (f(x))^2 dx
This formula is derived from the shell method, which involves breaking the solid into thin cylindrical shells, finding the volume of each shell, and adding them up. The formula is then the integral of the volume of each shell.
To solve this integral, we can use various methods such as integration by substitution or integration by parts. Once we have found the antiderivative of the integrand, we can evaluate the definite integral using the limits of integration a and b.
For example, if we have the function f(x) = x^2 and we want to find the volume of the solid of revolution created by rotating this function about the x-axis between x=0 and x=2, we can use the formula:
V = π∫[0,2] (x^2)^2 dx
Simplifying this expression, we get:
V = π∫[0,2] x^4 dx
Integrating this expression with respect to x, we get:
V = π[(1/5)x^5] [0,2]
Evaluating this expression at the limits of integration, we get:
V = π[(1/5)(2^5 - 0)]
V = π(32/5)
Therefore, the volume of the solid of revolution created by rotating the function f(x) = x^2 about the x-axis between x=0 and x=2 is approximately 20.106 cubic units.
Learn more about Axis of revolution
brainly.com/question/28649256
#SPJ11
Todd and Agnes are making desserts. Todd buys peaches and a carton of vanilla yogurt. Agnes buys the same number of pieces of fruit. Is there a situation in which they pay the same amount for their purchases?
Answers
Answer:
8 fruits
Step-by-step explanation:
Todd and agnes are making desserts. todd buys
peaches and a carton of vanilla yogurt. agnes
buys apples and a jar of honey. they bought
the same number of pieces of fruit. is there a
situation in which they pay the same amount for
their purchases? explain.
apples
peaches=
$1.25 for peaches
$1.00 for apples
yogurt
honey=
$4 for yogurt
$6 for honey
Todd:
Peaches + yogurt
1.25x + 4
Agnes:
1.00x + 6
Where,
x = number of pieces of fruit
Equate the cost of Todd and Agnes
1.25x + 4 = 1.00x + 6
1.25x - 1.00x = 6 - 4
0.25x = 2
x = 2/0.25
= 8
x = 8 fruits
The situation in which they both pay the same amount for their purchases is when they buy 8 fruits each
Jacob would like to purchase a coat and hat for a ski trip. The coat is $62.75, and the hat is $14.25. If the sales tax rate is 8%, then what will be the amount of tax on Jacob’s purchase? help please
Answers
The amount of sales tax on Jacob’s purchase is $6.16
What is sales tax?
It is a tax which is charged by the government to raise money so it can provide public services. The tax is based on a certain percent of the price. Example: My State charges 4% sales tax. I want to buy a top advertised for $40. So the sales Tax is $40 × 4% = $1.6.
Jacob would like to purchase a coat and hat for a ski trip. The coat is $62.75, and the hat is $14.25.
So the total cost of coat and hat is $(62.75+14.25)
= $ 77
Sales tax is 8%
It means in $100 the sales tax is $8
In $100 the sales tax is $8
In $1 the sales tax is 8/100
In $77 the sales tax is (8×77)/100
= $6.16
Hence, the amount of sales tax on Jacob’s purchase is $6.16.
To know more about sales tax
https://brainly.com/question/30109497
#SPJ1
40% of the people at Tim's Halloween party dressed up as superheroes. If 14 people dressed as superheroes, how many people were at Tim's Halloween party?
Answers
Can you provide the solution for this exercise?
Let u(w) = −(b − w)c. What restrictions on w, b, and c are required to ensure that u(w) is strictly increasing and strictly concave? Show that under those restrictions, u(w) displays increasing absolute risk aversion.
Answers
under the restrictions that c is negative to ensure strict concavity, the utility function u(w) = -(b - w)c displays increasing absolute risk aversion.
To ensure that u(w) is strictly increasing, we need the derivative of u(w) with respect to w to be positive for all values of w. Taking the derivative, we have du(w)/dw = -c. For u(w) to be strictly increasing, -c must be positive, which implies c must be negative.
To ensure that u(w) is strictly concave, we need the second derivative of u(w) with respect to w to be negative for all values of w. Taking the second derivative, we have d²u(w)/dw² = 0. Since the second derivative is constant and negative, u(w) is strictly concave.
Now, let's examine the concept of increasing absolute risk aversion. If a utility function u(w) exhibits increasing absolute risk aversion, it means that as wealth (w) increases, the individual becomes more risk-averse.
In the given utility function u(w) = -(b - w)c, when c is negative (as required for strict concavity), the absolute risk aversion increases as wealth (w) increases. This is because the negative sign implies that the utility function is concave, indicating that the individual becomes more risk-averse as wealth increases.
Learn more about utility function here:
https://brainly.com/question/31498128
#SPJ11
The diagram below shows the side view of a ramp used to help load and unload a moving van. Which measurement is closest to the length of the ramp in feet?

Answers
Measurement is closest to the length of the ramp in feet is 33 feet
Let AB = 26 ft and BC = 19.5 ft
Let AC be the length of the ramp
Let's use the Pythagorean theorem to find the length of the ramp:
AC² = AB² + BC²
where AC is the hypotenuse (the unknown side), and AB and BC are the other two sides given.
Substituting the given values, we get:
AC² = 26² + 19.5²
AC² = 676 + 380.25
AC² = 1056.25
AC = \(\sqrt{1056.25}\)
AC = 32.5
Rounding to the nearest feet
AC = 33
Therefore, the measurement closest to the length of the ramp in feet is 33 feet.
Learn more about Pythagorean theorem here
https://brainly.com/question/14930619
#SPJ1
Determine the value of the variable that makes each equation true.

Answers
Answer:
-9
-11
-4
Step-by-step explanation:
the sophists were principally concerned with mathematics, and formulated the so-called pythagorean theorem.
Answers
The statement "the sophists were principally concerned with mathematics, and formulated the so-called Pythagorean theorem" is not entirely accurate.
While the sophists were a group of philosophers who were known for their expertise in various subjects, including mathematics, they did not formulate the Pythagorean theorem. This theorem is named after Pythagoras, a philosopher and mathematician who lived in ancient Greece.The sophists were more concerned with rhetoric and the art of persuasion.
They were skilled at using language and arguments to influence others, and they often taught these skills to others for a fee. While they may have had some knowledge of mathematics, their primary focus was not on this subject.In fact, the Pythagorean theorem is traditionally attributed to Pythagoras and his followers, who were known as the Pythagoreans.
This theorem states that in a right-angled triangle, the square of the length of the hypotenuse (the side opposite the right angle) is equal to the sum of the squares of the lengths of the other two sides. This is a fundamental concept in geometry, and it has many practical applications in fields such as engineering and physics.In conclusion, while the sophists may have had some knowledge of mathematics, their primary focus was on rhetoric and persuasion. The Pythagorean theorem is traditionally attributed to Pythagoras and his followers, not the sophists.
To know more about Pythagoras visit:
brainly.com/question/17179659
#SPJ11
1,120 people attend a show at a theatre.
3/8 of the seats were taken by children.
The rest of the seats are taken by adults.
An adult ticket costs £28 in total, the theatre receives £24,220 in ticket sales.
How much does a child's ticket cost?
Answers
Answer:
£11
Step-by-step explanation:
first lets find the number of children's = 3/8 * 1120 =420 children's
then find number of adults = 1120 -420 = 700 adults
if adult costs, £28
28 * 700 = £19600
lets subtract the total cost - total adult ticket cost
= £24,220 - £19600
= £4620......this is the total children's ticket cost
there are 420 children ---> £4620
1 children --> £4620/420 = £11
A pool measuring 12 meters by 22 meters is surrounded by a path of uniform width, as shown in the figure. If the area of the pool and the path combined is 936 square meters. What is the width of the path?
Answers
The width of the path is 1 meter. This makes the length of the pool including the path 14 meters and the width 24 meters,
Let's denote the width of the path as x. Then the length of the pool including the path is 12 + 2x meters and the width is 22 + 2x meters. The total area of the pool and the path combined is given as 936 square meters, so we can set up an equation:
(12 + 2x)(22 + 2x) = 936
Simplifying this equation, we get:
4x^2 + 68x - 72 = 0
Factoring this quadratic equation, we get:
(x + 9)(4x - 8) = 0
Since the width of the path cannot be negative, we can only consider the positive root, which is: x = 2
Therefore, the width of the path is 2 meters.
But wait, this is not the final answer! We need to remember that the width of the path is uniform, which means it is the same on all sides of the pool.
If the width of the path is 2 meters, then the length of the pool including the path is 16 meters and the width is 26 meters. This gives a total area of: 16 x 26 = 416 square meters
which is clearly less than 936 square meters. Therefore, we made a mistake in our calculation and the width of the path cannot be 2 meters.
Let's go back to our quadratic equation and solve for x again: 4x^2 + 68x - 72 = 0
Dividing both sides by 4, we get:
x^2 + 17x - 18 = 0
Factoring this quadratic equation, we get:
(x + 18)(x - 1) = 0
Again, we can only consider the positive root, which is:
x = 1
Therefore, the width of the path is 1 meter. This makes the length of the pool including the path 14 meters and the width 24 meters, giving a total area of: 14 x 24 = 336 + 600 = 936 square meters, which confirms that our solution is correct.
To know more about length click here
brainly.com/question/30625256
#SPJ11
The swiss mathematician leonhard euler and the french mathematician rene descartes both discovered a pattern in the numbers of edges, vertices, and faces of polyhedrons. solve problems 810.
Answers
Answer:
I am stuck on this two
Step-by-step explanation:
rectangle, 6 8 14 12
Just read and answer me

Answers
Answer:
the picture isnt showing up on mines
Question 3 of 10
Which of the following must be true for an expression to be a difference of
two squares?
a. all variables are raised to an even power
b. there are only two terms
c. both terms have negative coefficients
OA. a, b, and c
B. a and b
OC. a and c
D. b and c
Answers
B is true: you cannot have a trinomial, only binomial or two terms
C is FALSE: you cannot take a square root of a negative and get a real number
B. a and b
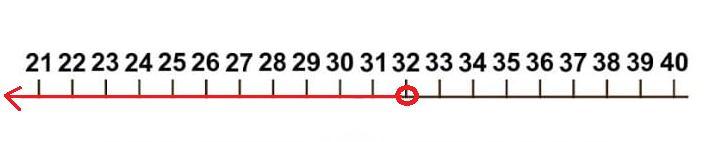
The chef filled a big pot with 3. 7 cups of water and filled a smaller pot with 7. 3 cups of water. Which pot has more water?
Answers
Using a unitary method, we have identified that the smaller pot has more water.
To compare the amount of water in the two pots, we need to find a common unit of measurement. In this case, both pots are measured in cups, so we can simply compare the number of cups in each pot. The chef filled the larger pot with 3.7 cups of water, and the smaller pot with 7.3 cups of water.
Now, we can use a unitary method to compare the two quantities. Let's assume that one cup is our unit of measurement. To find out how many cups of water are in the smaller pot, we can divide 7.3 by 1 cup. This gives us 7.3 cups of water. To find out how many cups of water are in the larger pot, we can divide 3.7 by 1 cup. This gives us 3.7 cups of water.
As we can see, the smaller pot has more water than the larger pot.
To know more about unitary method here
https://brainly.com/question/28276953
#SPJ4
I NEED HELP WITH THIS QUESTION PLEASE ? :(

Answers
Answer:
x=42
Step-by-step explanation:
X + 40
Х
Solve for x and find the measure of each angle using the space below.

Answers
Answer:
X = 70
Step-by-step explanation:
180 - 40 = 140
140/2 = 70
Please mark as brainliest if answer is right
Have a great day, be safe and healthy
Thank u
XD
the circumference of head sizes of newborns is approximately normal with mean 13.5 inches and standard deviation of 0.75 inches. what percent of babies have a head circumference greater than 14 inches?
Answers
A 24.8% of babies have a head circumference greater than 14 inches.
What is Circumference?The circumference of a circle is the length of the curved boundary or the perimeter of the circular shape. It is a measurement of the distance around the circle and is equal to the arc length of the circle if it were opened up and straightened out into a line segment. The circumference of a circle is related to its diameter by the formula: C = π * d
To calculate the percentage of babies with a head circumference greater than 14 inches, we need to find the area under the normal distribution curve to the right of 14 inches.
First, we need to convert 14 inches to the standard units (z-score). We can do that using the following formula:
z = (14 - 13.5) / 0.75 = 0.67
Next, we can use a standard normal distribution table to find the area to the right of z = 0.67. The value from the table is approximately 0.752.
Therefore, approximately 75.2% of babies have a head circumference less than or equal to 14 inches, and 24.8% of babies have a head circumference greater than 14 inches.
To know more about Circumference visit,
https://brainly.com/question/1513769
#SPJ1
PLS HELP WILL MARK BRAIN THINGY

Answers
The vertices of the image of the figure LMNO following a 90° rotation about the origin are; L'(3, 5), M'(6, 6), N'(3, 4), O'(6, 4), indicating that the corresponding segment L'O' and M'N' are on the lines x = 6 and x = 3, the correct option is option B.
B. x = 6 and x = 3
What is a rotation transformation?A rotation is the circular motion about a specified center of rotation of the points on the figure of the preimage.
The coordinates of the vertices of the figure LMNO are; L(-5, 3), M(-6, 6), N(-4, 6), O(-4, 3)
The transformation of the figure LMNO = 90° clockwise rotation about the origin.
The coordinate of the image of the point (x, y) following a rotation of 90° about the origin is the point (y, -x).
Therefore, the coordinates of the image of the figure LMNO following a rotation of 90° about the origin are found as follows;
L(-5, 3) \(\underset{\longrightarrow}{R_{90^{\circ}\ clockwise}}\) L'(3, 5)
M(-6, 6) \(\underset{\longrightarrow}{R_{90^{\circ}\ clockwise}}\) M'(6, 6)
N(-4, 6) \(\underset{\longrightarrow}{R_{90^{\circ}\ clockwise}}\) N'(6, 4)
O(-4, 3) \(\underset{\longrightarrow}{R_{90^{\circ}\ clockwise}}\) O'(3, 4)
The points L' and O' are colinear with the line x = 3, and the points M' and N' are colinear with the line x = 6
Therefore, the corresponding segment to the segment LO, L'O' lie on the the line x = 3, and the corresponding segment to the segment MN, M'N' lie on the line x = 6
The correct option is B. x = 6, and x = 3
Learn more about rotation transformation here: https://brainly.com/question/18406100
#SPJ1
If the sales tax rate is 7% in New York, then how much sales tax would you pay in New York for a $34 pair of
pants?
Please I need help fast
Answers
Answer:
you would pay 2.38 sales tax for a 34 dollar pair of pants
Step-by-step explanation:
Explain how to compute the exact value of each of the following definite integrals using the Fundamental Theorem of Calculus. Leave all answers in exact form, with no decimal approxi- mations. (a) 2x3+6x-7)dx (b) 6 cosxdx (c) 10edx
Answers
The exact value of the definite integral ∫(2x³ + 6x - 7)dx over any interval [a, b] is (1/2) * (b⁴ - a⁴ + 3(b² - a²) - 7(b - a). This expression represents the difference between the antiderivative of the integrand evaluated at the upper limit (b) and the lower limit (a). It provides a precise value without any decimal approximations.
To compute the definite integral ∫(2x³ + 6x - 7)dx using the Fundamental Theorem of Calculus, we have to:
1: Find the antiderivative of the integrand.
Compute the antiderivative (also known as the indefinite integral) of each term in the integrand separately. Recall the power rule for integration:
∫x^n dx = (1/(n + 1)) * x^(n + 1) + C,
where C is the constant of integration.
For the given integral, we have:
∫2x³dx = (2/(3 + 1)) * x^(3 + 1) + C = (1/2) * x⁴ + C₁,
∫6x dx = (6/(1 + 1)) * x^(1 + 1) + C = 3x²+ C₂,
∫(-7) dx = (-7x) + C₃.
2: Evaluate the antiderivative at the upper and lower limits.
Plug in the limits of integration into the antiderivative and subtract the value at the lower limit from the value at the upper limit. In this case, let's assume we are integrating over the interval [a, b].
∫[a, b] (2x³ + 6x - 7)dx = [(1/2) * x⁴ + C₁] evaluated from a to b
+ [3x²+ C₂] evaluated from a to b
- [7x + C₃] evaluated from a to b
Evaluate each term separately:
(1/2) * b⁴ + C₁ - [(1/2) * a⁴+ C₁]
+ 3b²+ C₂ - [3a² C₂]
- (7b + C₃) + (7a + C₃)
Simplify the expression:
(1/2) * (b⁴ a⁴ + 3(b² - a²) - (7b - 7a)
= (1/2) * (b⁴ - a⁴) + 3(b² - a²) - 7(b - a)
This is the exact value of the definite integral of (2x³+ 6x - 7)dx over the interval [a, b].
To know more about definite integral refer here:
https://brainly.com/question/29685762#
#SPJ11
a circular table has chairs around it. there are people who are currently seated at this table in such a way that if one more person decides to sit down, then he or she must sit adjacent to someone. what is the smallest possible value for ?
Answers
A circular table is surrounded by chairs. There are individuals who are currently seated at this table in such a way that if one more individual decides to sit down, then they must sit adjacent to someone. The smallest possible value for the number of people currently seated at the table is 3.
This is because in order for one more person to sit adjacent to someone, there must already be at least 3 people seated at the table. This is because if there were only 2 people seated at the table, the one more person would not have a "someone" adjacent to them to sit next to.
You can learn more about seating arrangements at
https://brainly.com/question/27935318
#SPJ4
Solve m/8<4 graph the solution

Answers
Answer:
Rearrange the equation by subtracting what is to the right of the greater than sign from both sides of the inequality :
m/8-(-4)<0
m
Simplify —
8
m
— - -4 < 0
2.1 Subtracting a whole from a fraction
Rewrite the whole as a fraction using 8 as the denominator :
-4 -4 • 8
-4 = —— = ——————
1 8 8
Step-by-step explanation:
the answer is m<-32
The solution of inequality m/8 < 4 is,
⇒ m = 32
Which is shown in graph of image.
What is mean by Inequality?A relation by which we can compare two or more mathematical expression is called an inequality.
We have to given that;
The expression of the inequality is,
⇒ m/ 8 < 4
Now, We can simply the expression as;
⇒ m/ 8 < 4
⇒ m/ 8 - 4 < 0
⇒ (m - 32)/8 < 0
⇒ m - 32 < 0
⇒ m < 32
Therefore, The solution of inequality m/8 < 4 is,
⇒ m = 32
Which is shown in graph of image.
Learn more about the inequality visit:
https://brainly.com/question/25944814
#SPJ3
rectangle QRST has coordinates Q(-8,5), R(-8,7), S(-5,7), and T(-5,5). dilate the rectangle by a scale factor of 4 with a center of dilation at the origin. write a rule to describe this transformation and use the rule to determine the coordinates of rectangle Q'R'S'T'
Answers
The rule to describe the transformation, dilation by a scale factor of 4 with center of dilation at the origin is (x,y)→(4x,4y) and the coordinates of rectangle Q'R'S'T' is are Q'(-32,20) , R'(-32,28) , S'(-20,28) , T'(-20,20) .
In the question ,
a rectangle QRST with coordinates Q(-8,5), R(-8,7), S(-5,7), and T(-5,5) is given ,
and to dilate the rectangle by a scale factor of 4 with center of dilation at the origin , we multiply coordinates of the original shape with the dilation factor
so the rule to describe the dilation will be
(x,y)→(4x,4y)
Using this rule to find the new coordinates of the rectangle Q'R'S'T' .
When Q = (-8,5)
Q' = (4*(-8),4*5)
Q' = (-32,20)
When R = (-8,7)
R' = (4*(-8),4*7)
R' = (-32,28)
When S = (-5,7)
S' = (4*(-5),4*7)
S' = (-20,28)
When T= (-5,5)
T' = (4*(-5),4*5)
T' = (-20,20)
So the coordinates of the rectangle Q'R'S'T' is Q' = (-32,20) , R' = (-32,28) , S' = (-20,28) , T' = (-20,20) .
Therefore , the rule to describe the transformation, dilation by a sale factor of 4 with center of dilation at the origin is (x,y)→(4x,4y) and the coordinates of rectangle Q'R'S'T' are Q'(-32,20) , R'(-32,28) , S'(-20,28) , T'(-20,20) .
Learn more about Transformation here
https://brainly.com/question/16938631
#SPJ1
Ms. Fry started walking as her New Year's resolution. She calculated that she can walk ½ mile in ¼ of an hour. What is her walking rate in miles per hour?
Answers
Answer:
the walking rate miles per hour is 2 miles per hour
Step-by-step explanation:
The computation of the walking rate miles per hour is shown below;
Given that
She can walk half mile in one-fourth of an hour
= Miles ÷ hours
= (1÷ 2) ÷ (1 ÷4)
= 0.50 ÷ 0.25
= 2 miles per hour
Hence, the walking rate miles per hour is 2 miles per hour
The same is considered
Determine which ordered pair is part of the solution set for the inequality.
3x + y ≥ 6
(4, 3), (-2, 4), (-5, -3), (3, -3)
Answers
Explanation:You just replace the values but first you start by sending the 3x to the other side
PLEASE HELP! ILL MARK BRAINLIEST!!

Answers
A student earned a grade of 80%mon a math test that had 20 problems. How many problems on this test did the student answer correctly (Round to the nearest whole number)
Answers
Answer:
16
Step-by-step explanation:
20*80/100 use this formula to find it
How many cuboids of length 10 m, width 3 m and height 2 m can be formed from a
cuboid of 18 m length, 15 m width and 20 m height?
Answers
Answer:
Given that l=20cm, b=12cm, h=9cm
∴T.S.A=2(lb+bh+lh)
=2[(20×12)+(12×9)+(20×9)]
=2(240+108+180)
=2×528
∴T.S.A=1056cm
2
Answer:
simply divide the volume of both cuboids like lbh/lbh simply you will get your ans.
In triangle xyz, m∠y = 38.25° and m∠z = 74.3°. determine the measure of the exterior angle to ∠x. 152.05° 112.55° 98.60° 62.05°
Answers
Applying the exterior angle theorem, the measure of the exterior angle to angle X is: B 112.55°.
How to Apply the Exterior Angle Theorem?In other to find the measure of the exterior angle to angle X, we have to recall the theorem known as the exterior angle theorem, which states that the measure of an exterior angle is equal to the two interior remote angles that are opposite to it.
In triangle XYZ, angle Y and Z are the remote interior angles. We are given the following:
Measure of angle Y = 38.25°
Measure of angle Z = 74.3°
Therefore:
The measure of the exterior angle to angle X = measure of angle Y + measure of angle Z
Substitute
The measure of the exterior angle to angle X = 38.25° + 74.3°
The measure of the exterior angle to angle X = 112.55°
Learn more about exterior angles theorem on:
https://brainly.com/question/11356657
#SPJ1
