Example Given the supply function P=10+ vg Find the price elasticity of supply. (a) Averaged along an arc between Q=100 and Q=105 (b) At the point Q=100.
Answers
(a) Averaged along an arc between Q=100 and Q=105:
The price elasticity of supply is approximately equal to 4.88% divided by (5v / (20 + 205v) * 100), where v is a parameter from the supply function P = 10 + vg.
(b) At the point Q=100:
The price elasticity of supply is equal to 100 multiplied by (v / (10 + 100v)), where v is a parameter from the supply function P = 10 + vg.
To calculate the price elasticity of supply, we need to use the following formula:
Elasticity of Supply = (% Change in Quantity Supplied) / (% Change in Price)
(a) Averaged along an arc between Q=100 and Q=105:
First, let's calculate the initial quantity supplied and price at Q=100:
P = 10 + v * 100
P = 10 + 100v (Equation 1)
Next, let's calculate the final quantity supplied and price at Q=105:
P = 10 + v * 105
P = 10 + 105v (Equation 2)
Now, let's find the percentage change in quantity supplied:
% Change in Quantity Supplied = (Q2 - Q1) / [(Q1 + Q2) / 2] * 100
% Change in Quantity Supplied = (105 - 100) / [(100 + 105) / 2] * 100
% Change in Quantity Supplied = 5 / 102.5 * 100
% Change in Quantity Supplied ≈ 4.88%
Next, let's find the percentage change in price:
% Change in Price = (P2 - P1) / [(P1 + P2) / 2] * 100
% Change in Price = [(10 + 105v) - (10 + 100v)] / [(10 + 100v + 10 + 105v) / 2] * 100
% Change in Price = (105v - 100v) / (20 + 205v) * 100
% Change in Price = 5v / (20 + 205v) * 100
Now, we can calculate the price elasticity of supply using the formula:
Elasticity of Supply = (% Change in Quantity Supplied) / (% Change in Price)
Elasticity of Supply ≈ (4.88% / (5v / (20 + 205v) * 100)
(b) At the point Q=100:
Using Equation 1, we have:
P = 10 + 100v
Now, let's find the derivative of P with respect to v:
dP/dv = 100
The price elasticity of supply at Q=100 is equal to the derivative of P with respect to v multiplied by v divided by P:
Elasticity of Supply = (dP/dv) * (v / P)
Elasticity of Supply = (100) * (v / (10 + 100v))
To learn more about price elasticity of supply visit : https://brainly.com/question/8729849
#SPJ11
Related Questions
Twenty-two more than a number is 47
Answers
Answer:
the number is 25
Step-by-step explanation:
If the number is n, then twenty-two more is n + 22
which equals 47, so n+ 22 = 47
n = 47 - 22 = 25
The distribution of the amount of money in savings accounts for University of Miami students has an average of 1,100 dollars and a standard deviation of 1000 dollars. Suppose that we take a random sample of 24 University of Miami students and ask them how much they have in their savings account. The sampling distribution of the sample mean amount of money in a savings account is
A. approximately Normal, with a mean of 1100 and a standard error of 204.12 B. not approximately normal C. Approximately Normal with an unknown mean and standard error D. approximately Normal, with a mean of 1100 and a standard error of 1000
Answers
A. approximately Normal, with a mean of 1100 and a standard error of 204.12.
This is because the Central Limit Theorem (CLT) states that the sampling distribution of the sample mean tends towards a normal distribution with a mean equal to the population mean and a standard error equal to the population standard deviation divided by the square root of the sample size.
In this case, the sample size is 24, so the standard error is 1000/sqrt(24) = 204.12. Therefore, the sampling distribution of the sample mean is approximately normal with a mean of 1100 and a standard error of 204.12.
To know more about standard error:
https://brainly.com/question/13179711
#SPJ4
ANSWER THIS RIGHT NOW PLEASE

Answers
The perimeter of rectangle A is k times the perimeter of rectangle B. Therefore, option C is the correct answer.
Here, we have,
Given that, rectangle A has a length and width that are k times the length and width of rectangle B.
We have,
The perimeter of a rectangle is the total distance of its outer boundary. It is twice the sum of its length and width and it is calculated with the help of the formula: Perimeter = 2(length + width).
Let the length of a rectangle A is L and the width of a rectangle A is W.
Let the length of a rectangle B is KL and the width of a rectangle A is KW.
Now, Perimeter of a rectangle A
= 2(L+W)
Perimeter of a rectangle B
= 2(KL+KW)
= 2K(L+W)
The perimeter of rectangle A is k times the perimeter of rectangle B. Therefore, option C is the correct answer.
To learn more about the perimeter of a rectangle visit:
brainly.com/question/15287805.
#SPJ1
complete question:
If rectangle A has a length and width that are k times the length and width of rectangle B, which statement is true?
A. the perimeter of rectangle A is 2k times the perimeter of rectangle B.
B. the perimeter of rectangle A is k^2 times the perimeter of rectangle B.
C. the perimeter of rectangle A is k times the perimeter of rectangle B.
D. the perimeter of rectangle A is k^3 times the perimeter of rectangle B.
answer this and get 100 points
write a percent equation that can be used to solve the following problem 38 what's the percent of 74
A. 38=p*74
B.74=p*38
C p*74=0.38
D p=38*74
Answers
Option B, which represents the correct Percent equation, is the correct answer.
The correct answer is option B.
To find the percent of a number, we need to divide the amount we want to find the percentage of by the total or whole amount, and then multiply by 100 to express it as a percentage.
In this problem, we want to find the percent of 74 that is 38. Let's use p to represent the unknown percentage.
The percent equation for this problem is:
38 = p/100 * 74
We divide 38 by 100 to express it as a decimal, and then multiply by 74 to get the amount that corresponds to the percentage we want to find.
Simplifying this equation, we get:
0.38 * 74 = p
So the percent of 74 that is 38 is approximately 28.12%.
Therefore, option B, which represents the correct percent equation, is the correct answer.
To know more about Percent equation.
https://brainly.com/question/24877689
#SPJ11
A 9-kilogram bag of rice costs $12.96. What is the unit price?
Answers
Answer:
$1.44
Step-by-step explanation:
9kg costs $12.96
1kg costs ($12.96*1kg)÷9kg
=$1.44
find dy/dx by implicit differentiation. y sin(x2) = x sin(y2)
Answers
The derivative dy/dx of the equation ysin(x^2) = xsin(y^2) is given by (sin(y^2) - ycos(x^2)2x) / (sin(x^2) - 2yxcos(y^2)).
In the given equation, y and x are both variables, and y is implicitly defined as a function of x. To find dy/dx, we differentiate each term using the chain rule and product rule as necessary.
Differentiating the left-hand side of the equation, we apply the product rule to ysin(x^2). The derivative of ysin(x^2) with respect to x is dy/dxsin(x^2) + ycos(x^2)*2x.
Differentiating the right-hand side of the equation, we apply the product rule to xsin(y^2). The derivative of xsin(y^2) with respect to x is sin(y^2) + x*cos(y^2)2ydy/dx.
Now we have two expression for the derivative of the left and right sides of the equation. To isolate dy/dx, we can rearrange the terms and solve for it.
Taking the derivative of ysin(x^2) = xsin(y^2) with respect to x using implicit differentiation yields:
dy/dxsin(x^2) + ycos(x^2)2x = sin(y^2) + xcos(y^2)2ydy/dx.
By rearranging the terms, we can solve for dy/dx:
dy/dx * (sin(x^2) - 2yxcos(y^2)) = sin(y^2) - y*cos(x^2)*2x.
Finally, we can obtain the value of dy/dx by dividing both sides by (sin(x^2) - 2yxcos(y^2)):
dy/dx = (sin(y^2) - ycos(x^2)2x) / (sin(x^2) - 2yxcos(y^2)).
Learn more about implicit differentiation here brainly.com/question/31568657
#SPJ11
PLEASE HELP IM TAKING A TEST!!
Which of these graphs represent a reaction that is exothermic and why
A. Graph 1, because the reactants have lower potential energy than the products
B.Graph 2, because the products have lower potential energy than the reactants
C.Graph 1, because the products have lower potential energy than the reactants
D.Graph 2, because the reactants have lower potential energy than the products

Answers
Answer:
maybe answer is D
HOPE IT HELPED
Find the equation of the sphere that passes throught a(1,2,-3), with center b (2,4,0).
Answers
The equation of sphere is (x -1 )^{2} + (y - 2 )^{2} + (z+3 )^{2} = 14.
According to the statement
we have to find the equation of the sphere which passes through the a(1,2,-3), with center b (2,4,0).
So, For this purpose, we know that the
Sphere is In analytical geometry, if “r” is the radius, (x, y, z) is the locus of all points and \((x_{0} , y_{0} , z_{0})\)
is the center of a sphere, then the equation of a sphere is given by: \((x -x_{0} )^{2} + (y - y_{0} )^{2} + (z-z_{0} )^{2} = r^{2}\).
So, Here from given equation
The sphere that passes through a(1,2,-3), with center b (2,4,0).
here the locus point is a(1,2,-3).
The radius of sphere is
\(Radius = \sqrt{(1-2)^{2} + (2-4)^{2} + (-3-0)^{2} } \\Radius = \sqrt{(1 + 4 +9 }\)
So, radius is square root 14.
And then the equation of sphere become
\((x -1 )^{2} + (y - 2 )^{2} + (z+3 )^{2} = 14\).
So, The equation of sphere is (x -1 )^{2} + (y - 2 )^{2} + (z+3 )^{2} = 14.
Learn more about Sphere here
https://brainly.com/question/1293273
#SPJ4
I need help!
Answers Choices:
6
-6
1/6
-1/6

Answers
x2 = 2
y1 = 6
y2 = 12
(y2 - y1) / (x2 - x1)
(12 - 6) / (2 - 1)
6 / 1
So the answer is 6
6•1=6
6•2=12
6•4=18
6•4=24
Calculus (yet again)!
The water level of a certain body of water is changing at a rate of \(W(t) = \frac{1}{2}cos(3 - \frac{t}{2})\) inches per hour, where t represents hours since 12 a.m.
What is the average hourly number of inches that the water level changes for this lake in one day?
I'm pretty sure that the number of inches that the water changes in one day would be represented by \(\int\limits^2_0 {W(t)} \, dt\) (where the number on top of the integral is a 24), but how would I set up an integral for the average hourly number of inches? Thank you so much!
Answers
Answer:
The average hourly number of inches that the water level changes per day is about 0.0231 inches per hour.
Step-by-step explanation:
The water level of a certain body of water is changing at a rate represented by the function:
\(\displaystyle W(t) = \frac{1}{2}\cos \left( 3- \frac{t}{2}\right)\)
Where W is measured in inches per hour and t represents hours since 12 A.M.
And we want to determine the average hourly number of inches that the water level changes for the lake in one day.
Recall that the average value of a function is given by:
\(\displaystyle f_\text{avg} = \frac{1}{b-a} \int_{a}^{b} f(x) \, dx\)
Hence, the find the average hourly number of inches that the water level changes in one day, we simply need to find the average value of W from t = 0 to t = 24.
Substitute:
\(\displaystyle W(t)_{\text{avg}} = \frac{1}{(24)-(0)} \int_{0}^{24} \frac{1}{2}\cos \left(3 - \frac{t}{2}\right) \, dt\)
Simplify:
\(\displaystyle W(t)_{\text{avg}} = \frac{1}{24} \int_{0}^{24} \frac{1}{2}\cos \left(3 - \frac{t}{2}\right) \, dt\)
Evaluate using u-substitution. We can let:
\(\displaystyle u = 3 - \frac{t}{2} \Rightarrow du = -\frac{1}{2}\, dt\Rightarrow -2\, du = dt\)
Hence:
\(\displaystyle W(t)_{\text{avg}} = -\frac{1}{24} \int_{3}^{-9} \cos u \, du\)
Evaluate:
\(\displaystyle \begin{aligned} W(t)_{\text{avg}} &= \frac{1}{24}\int_{-9}^{3} \cos u\, du \\ \\ &= \frac{1}{24}\left(\sin u\Big|_{-9}^{3}\right) \\ \\ &= \frac{1}{24}\left(\sin3 - \sin -9\right) \\ \\ &=\frac{1}{24}\left(\sin 3 + \sin 9\right) \\ \\ &\approx0.0231 \end{aligned}\)
In conclusion, the average hourly number of inches that the water level changes per day is about 0.0231 inches per hour.
Part A: Cassie hiked 4 tenths of a 4.7-mile trail. How many miles did Cassle have left to hike?
Part B: Dana was 1.6 miles ahead of Cassie. How many miles did Dana hike already?
Answers
The distance left to hike by Cassius is equal to 1.44 miles . Dana hiked 3.5986 miles
We are given that Cassie hiked 4 tenths of a 4.7-mile trail and Dana was 1.6 miles ahead of Cassie.
Since Cassius walked 6/10th of the trail of 3.6 miles.
Part of the trail remaining = 1 - 6/10 = 4/10 = 2/5
Distance remaining will be 2/5 of 3.6 miles
= 1.44 miles.
Hence distance left to hike by Cassius = 1.44 miles
b. Total distance by which Dana was ahead of Cassius = 1.6miles.
Distance left to hike by Dana= 1.44 miles - 1.6 miles = 0.0014 miles.
so, the distance hiked by Dana= 3.6 miles - 0.0014 miles = 3.5986 miles.
Dana hiked 3.5986 miles.
Learn more about the unitary method, please visit the link given below;
https://brainly.com/question/23423168
#SPJ1
factor out the common factor: -48y - 6x
i get how to do it but i'm not sure whether to put the negative in the greatest common factor or in the binomial. so basically -6(8y+x) or 6(-8y-x).
Answers
Answer:
-6 (x + 8y)
Step-by-step explanation:
Factor -48y -6x
-6x-48y
Answer : -6 (x+8y)
A survey was given to a random sample of 400 residents of a town to determine whether they support a new plan to raise taxes in order to increase education spending. Of those surveyed, 168 respondents said they were in favor of the plan. Determine a 95% confidence interval for the proportion of people who favor the tax plan, rounding values to the nearest thousandth.
Answers
The standard error of the sample proportion is sqrt[(0.42)(0.58)/400] = 0.032.
Using a 95% confidence level, the critical value is 1.96.
The margin of error is 1.96 * 0.032 = 0.063.
The 95% confidence interval is 0.42 ± 0.063, which is (0.357, 0.483).
Therefore, we can be 95% confident that the true proportion of people who favor the tax plan is between 0.357 and 0.483.
a strand of christmas lights measures 5/8 yards long. jake needs to outline a square with sides 1/5 yards long. does he have enough to outline the square?
Answers
Answer:
No, it's not long enough
Step-by-step explanation:
The perimeter of the square is 4/5. P=4s where s is the side length. 4/5 is larger than 5/8, so there will not be enough lights to cover the whole perimeter.
The price of a new car is £12500
It is reduced by £11625
Work out the percentage reduction
Answers
To work out the percentage reduction, we need to find the difference between the original price and the reduced price, and express that difference as a percentage of the original price:
Original price = £12500
Reduced price = £11625
Difference = £12500 - £11625 = £875
Percentage reduction = (Difference / Original price) x 100%
= (£875 / £12500) x 100%
= 7%
Therefore, the percentage reduction in the price of the car is 7%.
Answer:
Step-by-step explanation:
Discount \(= \pounds12,500 -\pounds11625= \pounds875\)
Percentage reduction \(=\frac{875}{12500} \times 100 = 7\)
The price of a new car was reduced by 7%.
a flipped coin can land in one of two states: heads or tails. write a vi that simulates simultaneously flipping four coins and determines how many of these coins land in the heads state.
Answers
The probability flipping of four coin that will give head is 0.93
Probability:
In statistics, Probability defines the possible way of occurring a particular event.
Given,
A flipped coin can land in one of two states: heads or tails.
Here we need to find the probability while flipping four coins then how many of these coins land in the heads state.
In order to calculate the probability of these event, we have to know the values of the sample space and the possible number of events,
Here the value of sample space is written as,
S = {HHHH, TTTT, HHHT, HHTH, HTHH, THHH, HHTT, HTTH, HTHT, THHT, THTH, TTHH, HTTT, THTT, TTHT, TTTH}
So, the value of n(S) = 16
The possible number of way to obtain the head state is,
E = {HHHH, HHHT, HHTH, HTHH, THHH, HHTT, HTTH, HTHT, THHT, THTH, TTHH, HTTT, THTT, TTHT, TTTH}
the value of n(E) = 15.
Therefore, the probability of getting head state is
=> P(E) = 15/16
=> P(E) = 0.93
To know more about Probability here.
https://brainly.com/question/11234923
#SPJ4
is this a polygon or not a polygon

Answers
This is not a polygon as it has a section (at the bottom) which is composed of non-straight segments.
A survey was given to a random sample of students attending college in which they were asked, "As a college student, do you feel like your high school prepared you for your college experience?" The data showed that 54% of the 600 students surveyed replied yes. What is the population of the statistical question? A. All students attending college B. All students in high school and college C. The 600 students who were surveyed D. The 54% of students who replied yes
Answers
Answer:
D
Step-by-step explanation:
Option A doesn't give any statistical data, B is the same as A, and C doesn't show accurately how many students replied yes or no.
POINTS AND BRAINLIEST! IF U ANSWER THIS! :)

Answers
Answer:
68%
Step-by-step explanation:
So we had 34 filets and 16 steaks.
We can add these two together to find the total amount of items sold.
\(34 + 16 = 50\)
So 50 total, but we're dealing with filets which is at 34
A percentage can be found by dividing 50 and 34
\(34\div 50\)
Giving us 0.68, also known as 68%
Helen can type at a rate of 70 words per minute.How many words can she type in 45 minutes?
Answers
Answer:
3150 wpm
Step-by-step explanation:
70/1=x/45
x=70*45 x=3150
Hope this helps plz hit the crown :D
Answer:
Helen can type 3150 words in 45 minutes.
Step-by-step explanation:
70 words Multiplied by 45 minutes
70×45=3150
Hope it helps!
4.
Find the perimeter of the triangle.
A. 294 m
B. 119 m
C. 84 m
D. 168 m

Answers
Answer:
84m
Step-by-step explanation:
The perimeter of a triangle is the sum of the length of the external sides.
Therefore, the perimeter of the triangle = 21m + 28m + 35m = 84m.
Hey there!
What is the formula for finding the perimeter of a triangle?
a + b + c = perimeter
What does the labels mean?
a = side 1
b = base
c = side 2
What should the equation look like?
21m + 28m + 35m = perimeter
How do should it be solve?
21m + 28m + 35m = perimeter
49m + 35m = perimeter
84m = perimeter
What is the answer to the question?
84m
Good luck on your assignment and enjoy your day!
~Amphitrite1040
3/x^2-1 - 2(x+3) = 5/x+1

Answers
Answer:
X=1.132589
Step-by-step explanation:
If im correct the answer would be x=1.132589
3
x2−1
−2(x+3)=
5
x+1
3
(x+1)(x−1)
+−2x−6=
5
x+1
Multiply all terms by (x+1)(x-1) and cancel:
3+(−2x−6)(x+1)(x−1)=5(x−1)
−2x3−6x2+2x+9=5x−5(Simplify both sides of the equation)
−2x3−6x2+2x+9−(5x−5)=5x−5−(5x−5)(Subtract 5x-5 from both sides)
−2x3−6x2−3x+14=0
(Use cubic formula)
x=1.132589
And dont forget to check your answer
x/6 = 3/8
(7th grade math)
Answers
Answer:
cross multiply so it becomes 8x=18 then divide both sides by 8 and x= 2 2/8
Step-by-step explanation:
HELP ME PLEASE!!! THIS IS DUE TONIGHT
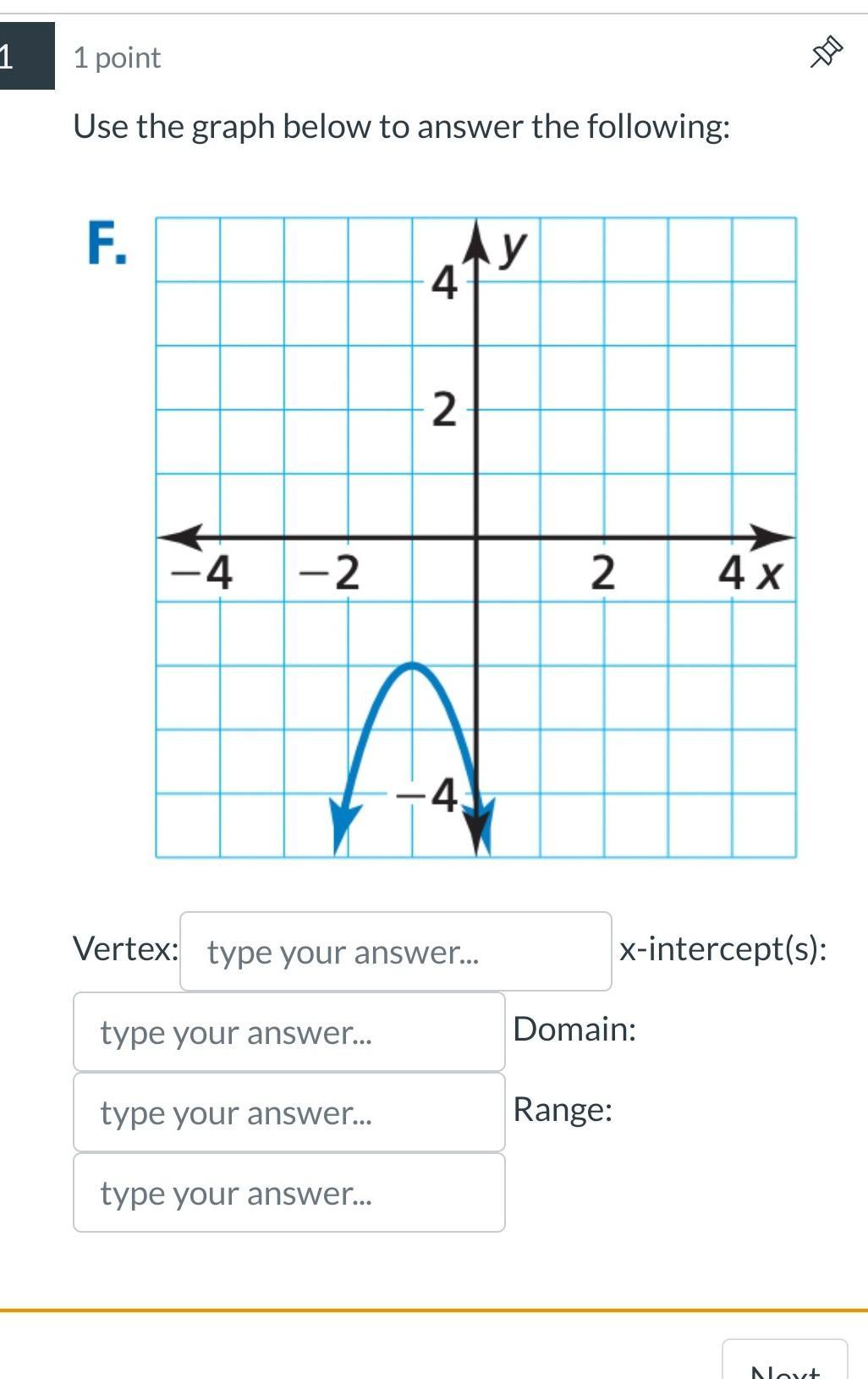
Answers
vertex: (-1,-2)
no x intercepts
domain: negative infinity to positive infinity
range: negative infinity to -2
Plz someone answer this I need the answer quick

Answers
Answer:
290 m^3Solution,
\(volume = (8 \times 5 \times 5) + (5 \times 6 \times 3) \\ \: \: \: \: \: \: \: \: \: \: \: \: = 200 + 90 \\ \: \: \: \: \: \: \: \: \: = 290 \: {m}^{3} \)
Hope this helps..
Good luck on your assignment..
-7/10+7/15+1/-20+-9/10+11/15+11/-20
what is the answer please help me .....!!!!
Answers
Answer:
-1
Step-by-step explanation:
-7/10+7/15+1/-20+-9/10+11/15+11/-20
= -7/10 + -9/10 + 7/15 + 11/15 + 1/-20 + 11/-20
= -16/10 + 18/15 + 12/-20
= -8/5 + 6/5 + -3/5
= (-8+6-3)/5
= -5/5
= -1
A curve passes through the point (0,5) and has the property that the slope of the curve at every point P is twice the y-coordinate of P. What is the equation of the curve?
Answers
If a curve passes through the point (0,5) and has the property that the slope of the curve at every point P is twice the y-coordinate of P. The equation of the curve is y = 5e^(²x).
How to find the equation?Let's assume that the equation of the curve is y = f(x), where f(x) is some unknown function.
We are given that the slope of the curve at every point P is twice the y-coordinate of P. This means that:
f'(x) = 2f(x)
where:
f'(x) is the derivative of f(x) with respect to x.
We can solve this differential equation by separating the variables and integrating:
1/f(x) df/dx = 2
Integrating both sides with respect to x, we get:
ln|f(x)| = 2x + C
where C is a constant of integration.
To find the value of C, we can use the fact that the curve passes through the point (0,5). Substituting x=0 and y=5 into the equation, we get:
ln|f(0)| = C
Since ln|f(0)| is just a constant, we can write it as another constant, say A. Therefore:
ln|f(x)| = 2x + A
Taking the exponential of both sides, we get:
|f(x)| = e^(²x+A)
Since f(x) cannot be negative (otherwise, the slope of the curve would be negative at some points), we can drop the absolute value signs:
f(x) = e^(²x+A)
To find the value of A, we can use the fact that the curve passes through the point (0,5). Substituting x=0 and y=5 into the equation, we get:
f(0) = e^A = 5
Therefore, A = ln(5). Substituting this value into the equation, we get:
f(x) = e^(²x+ln(5)) = 5e^(²x)
So the equation of the curve is y = 5e^(²x).
Learn more about equation here:https://brainly.com/question/2972832
#SPJ1
Kevin cuts four pieces of wood into fourths. How many fourths does he have?
Answers
Answer:
16/4
Step-by-step explanation:
BRAINLIEST?!
Answer:
16 fourths
Step-by-step explanation:
If one piece of wood is cut into fourths then you have 4 fourths. If you multiply that by 4 you have 16 fourths
Let A and B be two events in a sample space for which Pr(A) - 0.9923, Pr(B) = 0.0355700000000001 and Pr(AB) 0.02787. a. What is Pr(AUB)? b. What is Pr(AIB)? c. What is Pr(BIA)? d. What is Prt 2 )? e. What is Pr(A)? f. What is Pr(BP)? g. What is Pr((AUB)')?
Answers
For two events A and B in a sample space (a) Pr(AUB) = 1.0000000000001. (b) Pr(AIB) = 0.7840058543652 (c) Pr(BIA) =0.0280920459043 (d) Pr(A') = 1 - Pr(A) = 0.00769999999999996. (e) Pr(A) = 0.9923. (f) Pr(B') = 0.96443. (g) Pr((AUB)') = -1.11022302462516 x 10^-16.
Let A and B be two events in a sample space for which Pr(A) - 0.9923, Pr(B) = 0.0355700000000001 and Pr(AB) 0.02787 then :
a. To find Pr(AUB), we use the formula: Pr(AUB) = Pr(A) + Pr(B) - Pr(AB)
Plugging in the given values, we get: Pr(AUB) = 0.9923 + 0.0355700000000001 - 0.02787 = 1.0000000000001
b. To find Pr(AIB), we use the formula: Pr(AIB) = Pr(AB)/Pr(B)
Plugging in the given values, we get: Pr(AIB) = 0.02787/0.0355700000000001 = 0.7840058543652
c. To find Pr(BIA), we use the formula: Pr(BIA) = Pr(AB)/Pr(A)
Plugging in the given values, we get: Pr(BIA) = 0.02787/0.9923 = 0.0280920459043
d. Pr(A') = 1 - Pr(A) = 0.00769999999999996.
e. Pr(A) is given as 0.9923.
f. Pr(B') = 1 - Pr(B) = 0.96443.
g. To find Pr((AUB)'), we use the formula: Pr((AUB)') = 1 - Pr(AUB)
Plugging in the value we found in part (a), we get: g. Pr((AUB)') = 1 - Pr(AUB) = -1.11022302462516 x 10^-16.
Note that this result is not a valid probability since probabilities must be between 0 and 1.
To practice more questions for probability:
https://brainly.com/question/24756209
#SPJ11
Is the vertical component of velocity ever zero? If so, where?
Answers
The vertical component of velocity can be zero at specific points in the motion of an object.
What is Velocity?
Velocity is a vector quantity that describes the rate of change of an object's position in space over time. It is defined as the displacement of an object divided by the time interval during which the displacement occurred.
The vertical component of velocity can be zero at specific points in the motion of an object. This occurs when the object reaches the highest point in its vertical motion and begins to fall back down. At this point, the vertical component of velocity changes direction from upward to downward, and its magnitude becomes zero. This moment is known as the "instant of maximum height" or "instant of maximum altitude." Beyond this point, the vertical component of velocity becomes negative, indicating that the object is moving downward.
To learn more about Velocity, Visit
https://brainly.com/question/80295
#SPJ4