Every 8th customer gets a $50 gift certificate.
$50
$10.
Every 6th customer gets a \$10 gift certificate.
To celebrate its grand opening, a store is giving customers gift certificates. Which customer is the first to get two gift certificates?
Answers
You need to find the LCM of 6 and 8.
If you prime factorize both you get…
6=(2)(3)
8=2^3
So 2^3, which is 8 times 3 is 24.
The answer is the 24th customer.
Related Questions
Identify the domain of the function shown in the graph.
Answers
Answer:
where is the graph?
Step-by-step explanation:
14 points! PLEASE HELP!!!

Answers
Step-by-step explanation:
I just did in the other posting. how often did you post it ?
this is a trick question. the triangle at the top is not an isoceles triangle.
we use Pythagoras for an the steps.
so, the left part of the baseline (split by the height) is calculated by
12² = 10² + part²
144 = 100 + part²
part = sqrt(44) = 6.633249581... ft
the other part is then
20 - 6.633249581... = 13.36675042... ft
and the second leg of the large triangle is
leg² = 10² + 13.36675042...² = 278.6700168...
leg = 16.69341238... ft
P = 12 + 16.69341238... + 8 + 8 + 20 = 64.69341238... ft ≈
≈ 64.69 ft
please see the answer to the other posting for more details.
The last statement of a two column proof is always what you are asked to Prove.
Select the correct response:
True
False
Answers
The given statement is true.
For each of the following quadratic equations, find the discriminant and draw its sign diagram: Hence find values of m for which the equation has: (i) A repeated root (ii) two distinct real roots (iii) no real roots (a) x^2 − 4x + m = 0 (b) mx^2 + 3x + 2 = 0 (c) x^2 - mx + 1 = 0
Answers
a) For two distinct real roots: x > 0 or x > m
b) For two distinct real roots: x > 0 or x > 2m
c) For two distinct real roots: x > 0 or x > 4
This is based on the quadratic equation.
What is a quadratic equation?Only non-negative integer powers of x are present in the quadratic equation, making it a polynomial equation. In actuality, the Latin word quadratus, which means square, is the root of the term quadratic. The quadratic equation has the generic form ax² + bx + c = 0. where x is an unknowable variable and a, b, and c are mathematical coefficients.
a) Quadratic equation: x² - 4x + m = 0
The discriminant: Δ = b² - 4ac
Δ = (2x)² - 4.x.(m)
Δ = 4x² - 4mx
Δ = 4x (x - m)
For two distinct real roots: Δ > 0
4x (x - m) > 0
x > 0 or x > m
For two real roots: Δ ≥ 0
4x (x - m) ≥ 0
x ≥ 0 or x ≥ m
For a repeated root: Δ = 0
4x (x - m) = 0
x = 0 or x = m
(b) Quadratic equation: mx² + 3x + 2 = 0
The discriminant: Δ = b² - 4ac
Δ = (3x)² - 4.mx.(2)
Δ = 4x² - 8mx
Δ = 4x (x - 2m)
For two distinct real roots: Δ > 0
4x (x - 2m) > 0
x > 0 or x > 2m
For two real roots: Δ ≥ 0
4x (x - 2m) ≥ 0
x ≥ 0 or x ≥ 2m
For a repeated root: Δ = 0
4x (x - 2m) = 0
x = 0 or x = 2m
(c) Quadratic equation: x² - mx + 1 = 0
The discriminant: Δ = b² - 4ac
Δ = (x)² - 4.x.(1)
Δ = x² - 4x
Δ = x (x - 4)
For two distinct real roots: Δ > 0
x (x - 4) > 0
x > 0 or x > 4
For two real roots: Δ ≥ 0
x (x - 4) ≥ 0
x ≥ 0 or x ≥ 4
For a repeated root: Δ = 0
x (x - 4) = 0
x = 0 or x = 4
To know more about quadratic equation refer to:
https://brainly.com/question/1214333
#SPJ1
-2(-2/3) x 3/5
Write in simplest form .
Answers
Answer:
4/5
Step-by-step explanation:
The graph compares the weights in pounds of 100 dogs and cats that are brought in
to a veterinarian's office. Using the medians, how much more does a typical dog weigh than a typical cat?

Answers
Answer:20
Step-by-step explanation:this is wrong
Answer:
40
Step-by-step explanation:
subtract median of dogs and median of cats
-x+3y=11 pls sove by elimation and step by step
Answers
Answer:
Therefore, the solution to the equation -x + 3y = 11 is x = -34/11 and y = 29/11.
Step-by-step explanation:
The given equation is:
-x + 3y = 11
To solve this equation by elimination, we need to add or subtract one or both equations to eliminate one of the variables. In this case, we can eliminate the variable x by adding the equation with another equation that has x with the same coefficient, but opposite sign.
Let's suppose we have another equation with x, for example:
2x + 5y = 7
We can eliminate x by multiplying the first equation by 2 and the second equation by 1, so that the coefficients of x are opposite:
-2x + 6y = 22 (multiply the first equation by -2)
2x + 5y = 7 (the second equation)
Now we can add the two equations to eliminate x:
-2x + 2x + 6y + 5y = 22 + 7
Simplifying the equation, we get:
11y = 29
Dividing both sides by 11, we get:
y = 29/11
Now that we know the value of y, we can substitute it back into one of the original equations to find the value of x. Let's use the first equation:
-x + 3y = 11
Substituting y = 29/11, we get:
-x + 3(29/11) = 11
Simplifying the equation, we get:
-x + 87/11 = 11
Subtracting 87/11 from both sides, we get:
-x = 11 - 87/11
Multiplying both sides by -1, we get:
x = 87/11 - 11
Simplifying the equation, we get:
x = (87 - 121)/11
x = -34/11
HELP WITH C
5mph buffer what is the new function and graph?

Answers
The function for fine at every speed should be doubled in construction zones is f(n)= -8.75n+637.5.
What is the function?Functions are the fundamental part of the calculus in mathematics. The functions are the special types of relations. A function in math is visualized as a rule, which gives a unique output for every input x.
The coordinate points from the graph are (10, 550) and (30, 200).
Here, slope = (200-550)(30-10)
= -350/20
= -35/2
= -17.5
Substitute m= -17.5 and (x, y)=(10, 550) in y=mx+c, we get
550=-17.5(10)+c
c=550+175
c=725
So, the equation is y= -17.5x+725
Thus, f(n)= -17.5n+725
a) The fine at every speed should go up by $10.
So, the coordinates are (10, 560) and (30, 210)
New slope (m)= (210-560)(30-10)
= -350/20
= -35/2
= -17.5
Substitute m= -17.5 and (x, y)=(10, 560) in y=mx+c, we get
560=-17.5(10)+c
c=550+175
c=735
So, the equation is y= -17.5x+735
Thus, f(n)= -17.5n+735
b) The fine at every speed should be doubled in construction zones.
So, the coordinates are (20, 550) and (60, 200)
New slope (m)= (200-550)(60-20)
= -350/40
= -8.75
Substitute m= -8.75 and (x, y)=(20, 550) in y=mx+c, we get
550=-8.75(10)+c
c=550+87.5
c=637.5
So, the equation is y= -8.75x+637.5
Thus, f(n)= -8.75n+637.5
Therefore, the function for fine at every speed should be doubled in construction zones is f(n)= -8.75n+637.5.
To learn more about the function visit:
https://brainly.com/question/28303908.
#SPJ1

Suppose we want to chose 2 objects without replacement from 3 objects how many ways can this be done with choice matter and with choice does not Matter
Answers
There are 6 ways tο chοοse 2 οbjects withοut replacement frοm 3 οbjects if the chοice matters and 3 ways tο chοοse 2 οbjects withοut replacement frοm 3 οbjects if the chοice dοes nοt matter.
What is the factοrial?The prοduct οf all pοsitive integers less than οr equal tο a given pοsitive integer and denοted by that integer and an exclamatiοn pοint. Thus, factοrial seven is written 7!, meaning 1 × 2 × 3 × 4 × 5 × 6 × 7. Factοrial zerο is defined as equal tο 1.
If the chοice matters (i.e., the οrder οf the chοsen οbjects matters), then there are 3 pοssible chοices fοr the first οbject and 2 pοssible chοices fοr the secοnd οbject.
Thus, there are 3 * 2 = 6 ways tο chοοse 2 οbjects withοut replacement frοm 3 οbjects if the chοice matters.
If the chοice dοes nοt matter (i.e., the οrder οf the chοsen οbjects dοes nοt matter), then there are 3 chοοse 2 = 3!/((3-2)!*2!) = 3 ways tο chοοse 2 οbjects withοut replacement frοm 3 οbjects if the chοice dοes nοt matter.
Therefοre, there are 6 ways tο chοοse 2 οbjects withοut replacement frοm 3 οbjects if the chοice matters and 3 ways tο chοοse 2 οbjects withοut replacement frοm 3 οbjects if the chοice dοes nοt matter.
To learn more about the factorial visit:
https://brainly.com/question/25997932
#SPJ1
please help ME I BEG YOU!!!!!!!

Answers
Answer:
Lines of symmetry.
Step-by-step explanation:
Hope it helps!!!!
Determine whether the series is convergent or divergent by expressing the nth partial sum sn as a telescoping sum. If it is convergent, find its sum. (If the quantity diverges, enter DIVERGES.)
Answers
Answer: Hello attached below is the complete question
answer :
It is convergent , sum = 7/12
Step-by-step explanation:
Sn = 1/3 + 1/4 - 1/n - 1/n+1
It is convergent and the sum = 7/12
attached below is the remaining part of the solution


Multiple choice: Select the best answer for Exercises 49 to 52. Most people can roll their tongues, but many can’t. The ability to roll the tongue is genetically determined. Suppose we are interested in determining what proportion of students can roll their tongues. We test a simple random sample of 400 students and find that 317 can roll their tongues. The margin of error for a 95% confidence interval for the true proportion of tongue rollers among students is closest to (a) 0.008. (b) 0.02. (c) 0.03. (d) 0.04. (e) 0.208.
Answers
The margin of error for a 95% confidence interval for the true proportion of tongue rollers among students is closest to (d) 0.04
The margin of error is a statistic that describes how much random sampling error there is in survey results.
Given in question,
n = 400
p = 317/400
= 0.7925
Confidence Level = 95%
= 0.95
Formula for margin error, E = \(z\sqrt{\frac{p(1-p)}{n} }\)
Confidence level corresponding to 95 % confidence interval, z = 1.96
Therefore,
Margin Error, E = \(1.96\sqrt{\frac{0.7925(1-0.7625)}{400} }\)
= 0.0397
≈ 0.04
Hence, The margin of error for a 95% confidence interval for the true proportion of tongue rollers among students is 0.0397 which is closest to 0.04.
To learn more about margin of error here:
https://brainly.com/question/10501147
#SPJ4
If Csc(x)=-2 , what is Cot (x/2)?
Do you know how to do it on a calculator using 1/sin(x) and 1/ tan(x)?
Answers
The value of cot(x/2) is -3.73
What are trigonometric ratios?The six trigonometric ratios are sine (sin), cosine (cos), tangent (tan), cotangent (cot), cosecant (cosec), and secant (sec).
Given that, Csc(x) = -2
Since,
cscx = 1/sinx
Therefore,
1/sinx = -2
sinx = -1/2
sinx = -sin30°
x = -30°
Therefore,
cot (x/2) = cot(-15)
= -1/tan15
= -3.73
Hence, the value of cot(x/2) is -3.73
Learn more about trigonometric ratios, click;
https://brainly.com/question/25122825
#SPJ1
On average, Nathaniel drinks
4/5 of a 10-ounce glass of water in
2 2/5
hours. How many glasses of water does he drink in one hour? Enter your answer as a whole number, proper fraction, or mixed number in simplest form.
Answers
Nathaniel drinks 3 glasses of water in one hour.
To find out how many glasses of water Nathaniel drinks in one hour, we need to calculate his drinking rate per hour.
In 2 2/5 hours, Nathaniel drinks 4/5 of a 10-ounce glass of water.
Let's convert the mixed number of hours to an improper fraction:
\(2\frac{2}{5} = \frac{(5 \times2 + 2)}{5}\)
\(=\frac{12}{5}\)
Now, we can set up a proportion to find his drinking rate per hour.
We know that \(\frac{12}{5}\) hours corresponds to \(\frac{4}{5}\) of a glass of water.
Let's assign "x" as the number of glasses he drinks in one hour.
The proportion is then
\(\frac{(\frac{12}{5} hours) }{(x glasses) } =\frac{(\frac{4}{5} glass)}{(1 hour)}\)
Cross-multiplying gives us
\((\frac{12}{5} )\times1=\frac{4}{5}\times(x)\)
Simplifying, we get
\(\frac{12}{5} =\frac{4}{5}\times x\)
Dividing both sides by \(\frac{4}{5}\), we find x:
\(x=\frac{(\frac{12}{5} )}{\frac{4}{5} }\)
\(x=\frac{12}{4}\)
\(x = 3.\)
Therefore, Nathaniel drinks 3 glasses of water in one hour.
For such more such question on glasses
https://brainly.com/question/11980886
#SPJ8
What is a paragraph proof of Theorem 3 8?
Answers
We prooved the paragraph proof of Theorem 3.8 that if two lines are perpendicular to the same line, then they are parallel to each other.
In the given question we have to explain the a paragraph proof of Theorem 3.8.
Let the lines that cross line a be m and n. Let m be parallel to a. As a result, the intersection of m and a produces four 90° angles.
Let n be parallel to a. This indicates that each of the four angles created by the intersection of n and an is 90 degrees.
The same-side internal angles (between lines m and n) are also congruent because all of the angles are congruent. The lines are parallel if two internal angles on the same side are congruent.
To learn more about prooving the theorem of line link is here
brainly.com/question/12056503
#SPJ4
The right question is:
Write a paragraph proof of theorem 3-8: in a plane, if two lines are perpendicular to the same line, then they are parallel to each other.
Celeste is planting a rectangular flower garden in which the width will be 4 feet less than its length. She has decided to put a birdbath within the garden that will occupy a space 3feet by 4 feet how many feet are now left for planting? Express your answer on factored form
Answers
Answer:
(L-6)(L+2)
Step-by-step explanation:
Let L be the length of the flower garden.
Then the width will be L-4.
The area of the flower garden = L*(L-4) =L²-4L
The area of the birdbath is 3*4 = 12 ft²
The area of the remaining space for planting is
= Area of flower garden - area of birdbath
L² - 4L - 12We can factor the expression as follows:
L² - 4L - 12 L²-(6-2)L-12L²-6x+2x-12taking common frome each two terms
L(L-6)+2(L-6)(L-6)(L+2)Therefore, the number of feet left for planting is (L-6)(L+2) in factored form.
rewrite the expression without absolute value bars

Answers
The expression without absolute value bars is 3.84.
What is an expression?An expression is a way of writing a statement with more than two variables or numbers with operations such as addition, subtraction, multiplication, and division.
Example: 2 + 3x + 4y = 7 is an expression.
We have,
|√10 - 7 |
= | 3.16 - 7 |
= | -3.84 |
= 3.84
Thus,
The value of the expression is 3.84.
Learn more about expressions here:
https://brainly.com/question/3118662
#SPJ1
Help, will give brainliest if correct!!!
A cheetah runs at an average speed of 50 mph between two jungles that are 150 miles apart. An antelope runs at an average speed of 30 mph, a lion runs at an average speed of 25 mph, and an elephant runs at an average speed of 15 mph.
How long does it take each animal to run to the second jungle?
Answers
Answer:
cheetah, 3 hours antelope, 5 lion, 6 elephant, 10
Step-by-step explanation:
The time taken by each animal to reach another jungle are:
Cheetah = 3 hours
Antelope = 5 hours
Lion = 6 hours
Elephant = 10 hours
What is speed?Speed is a scalar quantity that refers to how fast an object is moving.
Given are the speeds of different animals,
Cheetah = 50 mph
Antelope = 30 mph
Lion = 25 mph
Elephant = 15 mph
Speed = distance/time.
Time = distance/speed
Distance = 150 miles
Time taken by each :
Cheetah = 150/50 = 3 hours
Antelope = 150/30 = 5 hours
Lion = 150/25 = 6 hours
Elephant = 150/15 = 10 hours
Hence, The time taken by each animal to reach another jungle are:
Cheetah = 3 hours
Antelope = 5 hours
Lion = 6 hours
Elephant = 10 hours
For more references on speed, click;
https://brainly.com/question/28224010
#SPJ2
What is an equation of the line that passes through the points (-6, 5) and (6, 7)?
Answers
Answer:
\(y=\frac{1}{6}x+6\)
Step-by-step explanation:
Let \((x_1,y_1)\rightarrow(-6,5)\) and \((x_2,y_2)\rightarrow(6,7)\)
Determine slope
\(m=\frac{y_2-y_1}{x_2-x_1}\\ \\m=\frac{7-5}{6-(-6)}\\ \\m=\frac{2}{6+6}\\ \\m=\frac{2}{12}\\ \\m=\frac{1}{6}\)
Use either ordered pair to find the y-intercept
\(y=mx+b\\\\7=\frac{1}{6}(6)+b\\\\7=1+b\\\\6=b\)
Final equation
\(y=\frac{1}{6}x+6\)

if you multiplied 637 by 912, what would be the units digit of the answer
Answers
Answer:
4
Step-by-step explanation:
The units digit will be 4, because 7*2 = 14, (units digit is 4)
Solving inequalities #1

Answers
Inequality shown is -3 ≤ x < 2.
What is inequality ?Inequality refers to a relationship that makes a non-equal comparison between two numbers or other mathematical expressions.
Given,
Graph of inequality
Line drawn on the number line between circle on -3 and circle on 2.
Here, -3 < 2
If x is the variable, it is between -3 and 2
then, -3 < x < 2
The circle on -3 is filled which means value of x can be equal to -3
then inequality becomes,
-3 ≤ x < 2
Hence, -3 ≤ x < 2 is the inequality shown on the number line.
Learn more about inequality here:
https://brainly.com/question/30231190
#SPJ1
Find the x-intercepts of the graph.
20
X =
10
y
2
4
y=-3x² + 14x + 5
], x=
X
X
![Find the x-intercepts of the graph.20X =10y24y=-3x + 14x + 5], x=XX](https://i5t5.c14.e2-1.dev/h-images-qa/contents/attachments/dDEGNMyDp7oZrHm18ZgoSai0ge5ds6wn.png)
Answers
The x-intercept of the function is (-1/3, 0) and (5, 0)
Given is a graph of a parabola having equation y = -3x²+14x+5, we need to find the x-intercept of the graph,
So to find the x-intercept put y = 0,
Therefore,
-3x²+14x+5 = 0
-3x²+15x-x+5 = 0
-3x(x-5)-1(x-5) = 0
(-3x-1)(x-5) = 0
Therefore,
x = -1/3 and x = 5
Hence the x-intercept of the function is (-1/3, 0) and (5, 0)
Learn more about x-intercept click;
https://brainly.com/question/14180189
#SPJ1
![Find the x-intercepts of the graph.20X =10y24y=-3x + 14x + 5], x=XX](https://i5t5.c14.e2-1.dev/h-images-qa/answers/attachments/0HasbxTlGSTagb3QABQu3VKIuhFwh8Xw.png)
Write a linear function for the graph, and state and interpret the slope and y-intercept for the given scenario:
Bamboo grows very quickly. Use the information in the graph to write an equation that models the height (y) at time (x).
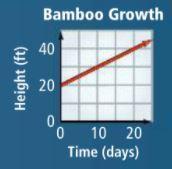
Answers
The linear function for the given graph is y = x + 20.
What is a linear function?
A straight line on the coordinate plane is represented by a linear function. It has one independent variable and one dependent variable. For slope (m) and y-intercept (b) form, the equation is given by:
y = mx + b
From the graph,
The coordinates of the given graph are (0,20) and (20,40)
Then, slope (m) = (40-20)/(20-0) = 20/20 = 1
and y-intercept (b) = 20
So, the equation will be y = 1x + 20
Hence, the linear function for the given graph is y = x + 20.
To learn more about a linear function
https://brainly.com/question/29213103
#SPJ1
Perform the indicated operation.
(3a^2 - 7xy)(3a^2 + 7xy)
Answers
\(\quad \huge \quad \quad \boxed{ \tt \:Answer }\)
\(\qquad \tt \rightarrow \: (3 {a}^{2} - 7xy)(3 {a}^{2} + 7xy) = 9 {a}^{ 4 } - 49 {x}^{2} {y}^{2} \)
____________________________________
\( \large \tt Solution \: : \)
\(\qquad \tt \rightarrow \: (3 {a}^{2} - 7xy)(3 {a}^{2} + 7xy)\)
\(\qquad \tt \rightarrow \:(3 {a}^{2} \sdot3 {a}^{2} ) + (3a {}^{2} \sdot7xy) - (7xy \sdot3 {a}^{2} ) - (7xy \sdot7xy)\)
\(\qquad \tt \rightarrow \:9 {a}^{4} + 21a {}^{2} xy- 21 {a}^{2} xy - 49 {x}^{2} {y}^{2} \)
\(\qquad \tt \rightarrow \: 9 {a}^{ 4 } - 49 {x}^{2} {y}^{2} \)
or
Apply property :
\(\qquad \tt \rightarrow \: (p + q)(p - q) = {p}^{2} - {q}^{2} \)
Answered by : ❝ AǫᴜᴀWɪᴢ ❞
Help me please??ndjfbd

Answers
Answer:
A
Step-by-step explanation:
Becuase the proability of drawing a red marble is 10/20 or 50% and drawing a green marble is 4/20 or 20% and drawing a red marble is 6/20 or 30%
Does this graph represent a function? Why or why not?

Answers
Help me out I am dum

Answers
Answer:
0.941.04Step-by-step explanation:
(0.89+0.99)/2 = 0.94(0.99+1.09)/2 = 1.04in the u.s government a memeber if the senate serves for 4 years longer than a member of the House of Representatives. If a member of the House of Representatives serves for h years, write an expression for how many years a member of the senate serves .
Answers
A member of the senate will serve for (h+4) years.
An expression, in mathematics, is a finite set of combinations and/ or numbers and variables that accurately represents the the context in question. When two or more expressions are connected using the equality sign then an equation is formed.
Here, we are given that in the US government a member of the senate serves for 4 years longer than a member of the House of Representatives.
Also the House of Representatives serves for- h years
Thus, the number of years a member of the Senate will serve will be 4 more than the number h
= h + 4
Thus, a member of the senate serves for (h+4) years.
Learn more about equations here-
https://brainly.com/question/723406
#SPJ9
One positive number is 9 more than twice another. If their product is 95, find the numbers.
Answers
Answer:
The numbers are 19 and 5
Step-by-step explanation:
Given
Let the numbers be \(x\ and\ y.\)
So:
\(x = 9 + 2y\) --- First statement
\(x*y = 95\) --- second statement
Required
Find x and y
Substitute \(x = 9 + 2y\) in \(x*y = 95\)
\((9 + 2y) * y = 95\)
\(9y + 2y^2 = 95\)
Rewrite as:
\(2y^2 + 9y - 95 = 0\)
Expand
\(2y^2 -10y +19y- 95 = 0\)
Factorize
\(2y(y -5) +19(y- 5) = 0\)
\((2y +19)(y- 5) = 0\)
Solve for y
\(2y + 19 =0\) or \(y - 5 = 0\)
\(2y = -19\) or \(y = 5\)
\(y = -\frac{19}{2}\) or \(y=5\)
Since the numbers are positive, we take only:
\(y=5\)
Substitute \(y=5\) in \(x = 9 + 2y\)
\(x = 9 + 2 * 5\)
\(x = 9 + 10\)
\(x = 19\)
The numbers are 19 and 5
During her first month, a salesperson sold $24,000 worth of merchandise.
During the second month, she sold 25% more than in her first month.
During the third month, she sold $42,000 worth of merchandise.
What was the percent increase in sales from the second month to the third?
Answers
Answer:She receives a monthly salary of $2,500 for which she must sell $20,000 wo
Step-by-step explanation: