Evaluate log6 base 10+ log45 base 10 - log27 base 10
Answers
Answer:
The correct answer is B.
Explanation:
log106 + log1045 - log1027
log10
6
×
45
27
= log102 x 5
log1010 = 1
Step-by-step explanation:
To solve the problem we must know about the properties of logarithms.
What are the Properties of logarithms?There are four basic properties of logarithms:
\(\rm log_aU+ log_aV = log_a(UV)\)\(\rm log_aU - log_aV = log_a(\dfrac{U}{V})\)\(\rm log_aU^n = n\ log_aU\)\(\rm log_ab = \dfrac{log_xb}{log_xa}\)The solution to the problem is 1.
Given to us
\(\rm log_{10}6+log_{10}45 - log_{10}27\)
Evaluation of the Logarithmic expressionTo solve the problem we will use the \(\rm log_aU - log_aV = log_a(\dfrac{U}{V})\) and \(\rm log_aU+ log_aV = log_a(UV)\) properties of logarithms, thus the solution to the problem can be calculated as shown below,
\(\rm log_{10}6+log_{10}45 - log_{10}27\)
\(=\rm {log_{10}(6\times 45)}-{log_{10}27}\)
\(=\rm {log_{10}(\dfrac{6\times 45}{27})\)
\(=\rm {log_{10}(\dfrac{6\times 45}{27})\)
\(=\rm log_{10}10\)
\(=1\)
Hence, the solution to the problem is 1.
Learn more about Logarithmic:
https://brainly.com/question/7302008
Related Questions
f(x)=|3x+5|+6
g(x)=7
find (f+g) (x).
Answers
Answer:
\((f+g) (x)=\mid 3x+5\mid+13\)
Step-by-step explanation:
Operation of Functions
Given:
\(f(x)=\mid 3x+5\mid+6\)
\(g(x)=7\)
The sum of (f+g) (x) is:
\((f+g) (x)=\mid 3x+5\mid+6+7\)
We cannot operate with the expression inside the absolute bars, thus:
\(\boxed{(f+g) (x)=\mid 3x+5\mid+13}\)
Let a1, a2, a3 be linearly independent vectors in R3, and let A = [a1 a2 a3]. Which of the following statements are true?(a) The reduced row echelon form of A is I3.(b) The rank of A is 3.(c) The system [A | b] has a unique solution for any vector b in
Answers
(a) The reduced row echelon form of A is not necessarily I3.
(b) The rank of A is 3.
(c) The system [A | b] has a unique solution for any vector b in R3.
(a) The reduced row echelon form of A is not necessarily I3. In fact, the reduced row echelon form of A can be any invertible 3x3 matrix, as long as it is not equal to I3. This is because any invertible matrix can be obtained by row operations from the identity matrix, and these row operations do not change the linear independence of the columns of A.
(b) The rank of A is 3. This is because the three vectors a1, a2, and a3 are linearly independent, so none of them can be written as a linear combination of the others. Therefore, the span of the three vectors is the entire space R3, and the rank of A is the dimension of this span, which is 3.
(c) The system [A | b] has a unique solution for any vector b in R3. This is true, because the rank of A is 3, which means that the columns of A span the entire space R3. Therefore, for any vector b in R3, there exists a unique linear combination of the columns of A that equals b, and this linear combination gives the solution to the system [A | b].
Learn more about row echelon form here
brainly.com/question/30356422
#SPJ4
A rectangle of Length (4x-1) cm and Breadth (2x), has an Area 10 cm² find x
Answers
Answer:
x = 1.25
Step-by-step explanation:
Givens
Area = L * w
L = 4x-1
w = 2x
Area = 10 cm^2
Formula
A = L * w
Area = 2x(4x - 1)
Solution
Area = 2x (4x - 1) = 10 Remove the brackets
2x * 4x - 2x = 10 Subtract 10 from both sides
8x^2 - 2x - 10 = 0 Factor
This factors into
(4x - 5)(2x + 2) = 0
4x - 5 = 0
4x = 5
x = 5/4
x = 1.25
2x + 2 = 0
2x = -2
x = - 1 When talking about area, this is an impossible answer.
cises 12.4 Slete the following: Find the intercepts and domain and perform the symmetry test on each parabola with equation: Graph the vertex, focus, and endpoints of the latus in problem 1. (a) y2 = 8x (c) y2 = - 4x (b) x² = 8y (d) x² = -4y

Answers
The y-intercept is given by the value of y when x = 0, and the x-intercept is given by the value of x when y = 0.
The domain is all values of x the function can assume in order to y exist.
The axis of symmetry is the axis where the function is mirrowed.
a) y² = 8x -> y = √(8x)
\(\begin{gathered} \text{y-intercept:} \\ x=0\to y^2=0\to y=0 \\ \text{x-intercept:} \\ y=0\to x=0 \\ \text{Domain: all real numbers} \\ \text{Axis of symmetry: }x=0 \end{gathered}\)b) x² = 8y
\(\begin{gathered} \text{ y-intercept:} \\ x=0\to y=\frac{0}{8}=0 \\ \text{ x-intercept:} \\ y=0\to x^2=0\to x=0 \\ \text{Domain: all real numbers} \\ \text{Axis of symmetry: }x=0 \end{gathered}\)c) y² = -4x -> y = √(-4x)
\(\begin{gathered} \text{ y-intercept:} \\ x=0\to y^2=0\to y=0 \\ \text{ x-intercept:} \\ y=0\to x=0 \\ \text{Domain: }x\le0 \\ \text{Axis of symmetry: }y=0 \end{gathered}\)d) x² = -4y
\(\begin{gathered} \text{ y-intercept:} \\ x=0\to y=\frac{0}{-4}=0 \\ \text{ x-intercept:} \\ y=0\to x^2=0\to x=0 \\ \text{Domain: all real numbers} \\ \text{Axis of symmetry: }x=0 \end{gathered}\)HELP PLEASE
NO RANDOM ANSWERS OR PHOTO ANSWER ANY OF THAT WILL BR REPORTED





Answers
Answer: The answer for the second one is solid figure
Step-by-step explanation: In geometry, three-dimensional shapes are solid figures.
Tanner bought 6 chocolates. Maggie bought c times as many chocolates as Tanner. Write an expression that shows how many chocolates Maggie bought.
Answers
Answer:
The equation that we most use to find how many chocolates Maggie bought would be:
6 x c = how many chocolates Maggie bought
Maggie bought c times as many chocolates as Tanner. This means to find how many chocolates Maggie bought we would multiply the c (Being how many times more chocolates Maggie bought) by 6 to find the number of chocolates Maggie bought`.
For example:
If the question stated Maggie bought 4 times as many chocolates as Tanner, the equation would be; 6 x 4 = how many chocolates Maggie bought. Which would be 24 chocolates.
Step-by-step explanation:
Have a great rest of your day
#TheWizzer

can you solve this question?

Answers
By the Mean Value Theorem, there exists a number c in (1, 7) such that ƒ'(c) = 2/c and 2/c = ln7 / 6, and c ≈ 0.909.
How to calculate the valueThe Mean Value Theorem (MVT) states that if a function ƒ(x) is continuous on the closed interval [a, b] and differentiable on the open interval (a, b), then there exists a number c in (a, b) such that:
ƒ'(c) = [ ƒ(b) - ƒ(a) ] / (b - a)
ƒ(x) = 2lnx - 8 is continuous on the closed interval [1, 7], since it is the sum and composition of continuous functions.
ƒ(x) = 2lnx - 8 is differentiable on the open interval (1, 7), since its derivative ƒ'(x) = 2/x is defined and continuous on (1, 7).
Therefore, the Mean Value Theorem can be applied to ƒ(x) on [1, 7]. To find the value of c, we need to solve the equation:
ƒ'(c) = [ ƒ(b) - ƒ(a) ] / (b - a)
Substituting the given values, we get:
2/c = [ 2ln7 - 2ln1 ] / (7 - 1)
2/c = ln7
c = 2 / ln7
c ≈ 0.909
Therefore, by the Mean Value Theorem, there exists a number c in (1, 7) such that ƒ'(c) = 2/c and 2/c = ln7 / 6, and c ≈ 0.909.
Learn more about mean on:
https://brainly.com/question/1136789
#SPJ1
Jerry has two same size circles divided into same number of equal parts. One circle has has 3/4 of the parts shaded and the other has 2/3 of the parts shaded. His sister says the least number of pieces could be divided into 7,
Answers
Answer:
Step-by-step explanation:
we know that triangle dfe is isosceles with base fe and that segment fb is congruent to segment ec because . segment df is congruent to segment by the definition of isosceles triangle. since these segments are congruent, the base angles, angles , are congruent by the isosceles triangle theorem. therefore, triangles are congruent by sas.
Answers
In a Isosceles triangle, DEF , ∆DFB is congruent to ∆DEC by SAS Congruence criteria, i.e., ∆DFB ≅ ∆DEC.
SAS congruance Criteria : If two sides and the angle between these two sides of one triangle are congruent to the corresponding sides and angle of another triangle, then the two triangles are congruent. We have a triangle DFE with base FE, see in above figure. The EC congruent to FB, i.e., FB ≅ EC. We have to prove ∆DFB ≅ ∆DEC.
Proof : It is known that ∆DEF is isocles triangle with base FE. So, two sides of triangle are equal.
In ∆DFB and ∆DEC,
=> DF = D E ( by definition of Isoceles triangle)
Also, base FB≅ EC ( since, we have)
Now, ∠DFE = ∠DEF => ∠DFB = ∠DEC ( because DE = DF in ∆DEF , corresponding angles of equal sides are equal) .
So, Two sides and the angle between the sides of one triangle, ∆DFB is congruent to the corresponding sides and angle of another triangle, ∆DEC. Therefore, by SAS Congruence criteria, Triangle DFB is congruent to triangle DEC, i.e., ∆DFB≅ ∆DEC. Hence proved..
To learn more about SAS Congruence rule, refer:
https://brainly.com/question/18922904
#SPJ4
Complete question:
Given: ΔDFE is isosceles with base FE; FB ≅ EC. Prove: ∆DFB ≅ ∆DEC.Complete the missing parts of the paragraph proof. We know that triangle DFE is isosceles with base FE and that segment FB is congruent to segment EC because . Segment DF is congruent segment by the definition of isosceles triangle. Since these segments are congruent, the base angles, angles , are congruent by the isosceles triangle theorem. Therefore, triangles are congruent by SAS

Consider the graph of f(x). What is the domain of f(x)?

Answers
Answer:
(-infinity,+infinity)
Step-by-step explanation:
Any real value of x can be defined with a unique output in this function.
Find the value of x, 6,4, 3x, 4x+1

Answers
Answer:
If two chords intersect in a circle, then the product of the segments of one chord equals the product of the segments of the other chord.
6(3x) = 4(4x + 1)
18x = 16x + 4
2x = 4
x = 2
A boat heads in the direction N 78° E. The speed of the boat relative to the water is 21 mi/h. The water is flowing directly south. It
is observed that the true direction of the boat is directly east. (Assume that the i vector points east, and the j vector points north.)
(a) Express the velocity of the boat relative to the water as a vector in component form. (Round your answer to one decimal
place.)
(20.5, 4.4)
Answers
Answer:
it is the vector
Step-by-step explanation:
para hacer 30 pasteles se necesitan 12kg de harina, cuantos kg de harina se requieren para hacer un pastel?
por favor ayuden me lo mas rapido posible
Answers
To make one cake, you would need 0.4 kg (or 400 grams) of flour.
To make 30 cakes, 12 kg of flour are needed.
We can find out how many kilograms of flour are needed for one cake by dividing the total amount of flour by the number of cakes.
Dividing 12 kg of flour by 30 cakes gives us:
12 kg / 30 cakes = 0.4 kg of flour per cake.
Therefore, to make one cake, you would need 0.4 kg (or 400 grams) of flour.
for such more question on grams
https://brainly.com/question/25545050
#SPJ8
i need help with this question please
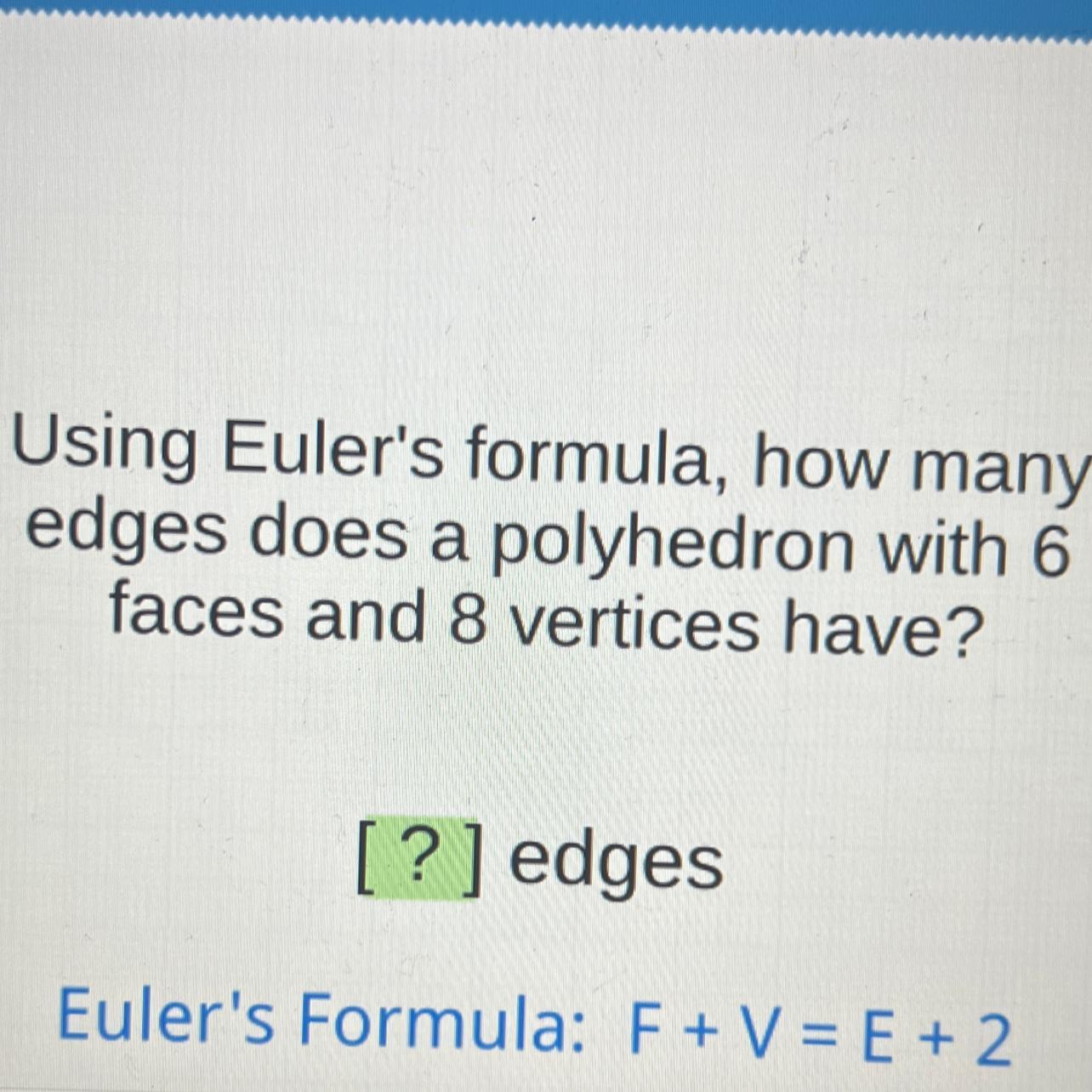
Answers
Answer:
A polyhedron with 6 faces and 8 vertices has 12 edges.
Step-by-step explanation:
Euler Formula is given in the problem:
F + V = E + 2We are told that the polyhedron has 6 faces (F = 6) and 8 vertices (V = 8). The only unknown variable in Euler's Formula now is E = number of edges.
Substitute the known variables into the formula and solve for E.
6 + 8 = E + 2 14 = E + 2 12 = E E = 12A polyhedron with 6 faces and 8 vertices has 12 edges.
Number of faces = 6
Number of vertices = 8
Formula used:
Euler's formula:
F + V - E = 2
F = Number of Faces of Polyhedron
V = Number of Vertices of Polyhedron
E = Number of Edges of Polyhedron
Calculations:
Let the polyhedron have E edges,
6 + 8 - E = 2
14 - E = 2
E = 14 - 2
E = 12
Therefore:
The Polyhedron has 12 edges
How many solutions does this system have? no solutions one unique solution O O two solutions O or an infinite number of solutions

Answers
Answer:
no solutions
Step-by-step explanation:
the equation of a line in slope- intercept form is
y = mx + c ( m is the slope and c the y- intercept )
equation of blue line is y = x + 2 , in slope- intercept form
with slope m = 1
equation of red line is y = x - 3 , in slope- intercept form
with slope m = 1
• Parallel lines have equal slopes
then the blue and red lines are parallel.
the solution to the system is at the point of intersection of the 2 lines
since the lines are parallel then they do not intersect each other.
thus the system shown has no solution.
A force of 30 Newtons will compress a spring of 10 meters. What is the spring constant of the spring?
Answers
Answer:
40
Step-by-step explanation:
IM IN A HURRY PLEASE HELP ME QUESTION IS DOWN BELOW WORTH 15 POINTS each

Answers
In the given figure, the measure of the central angle CAD is 80°, the major arc is arc CBD, and minor arc is arc CD. The measure of arc BEC is 2.27r and that of arc BC is 0.87r.
About the Central Angle:
An angle formed by two radii of a circle is known as a central angle. Thus, arc BC and arc CD both subtends central angles at the center.
Since BD is the diameter of the circle,
∠BAC + CAD = 180°
It is given that ∠BAC = 100°
⇒ ∠CAD = 180° - 100°
⇒ ∠CAD = 80°
About Major Arc:
The arc which subtends an angle greater than 180° at the center, is called a major arc.
Angle subtended by arc BEC = 360° - m(arc CD)
= 360° - 80°
= 280° > 180°
∴ Arc BEC is the major arc
About Minor Arc:
The arc which subtends an angle less than 180° at the center, is called a minor arc.
⇒ Arc CD is the minor arc.
Calculating arc BEC and arc BC:
Let us assume the radius of the circle is r.
Then, the formula of the measure of an arc is given by,
θ × (π/180) × r
Here, θ is the angle ( in degrees) subtended by the arc at the center.
Arc BEC = 260 × (π/180)r ......... [Put π = 3.14]
= 2.27r
Similarly, arc BC = 100 ×(π/180) × r .......... [Put π = 3.14]
= 0.87r
Learn more about an arc here:
https://brainly.com/question/16930503
#SPJ1
pls help asap giving brainliest !!!

Answers
Answer:
-2, 5/3
Step-by-step explanation:
y=2/3(-2)+3
2/3(-2)=-4/3
y=-4/3+3
The mark up on an item is $3.75. If the mark up is 35%, what was the original price? Round
your answer to the nearest hundredth. (Show all work)
Answers
Answer:
$10.71
Step-by-step explanation:
\(\frac{3.75}{y} =\frac{35}{100}\)
y × 35 = 3.75 × 100
35y = 375
35y ÷ 35 = 375 ÷ 35
\(y=10\frac{5}{7}\)
y = 10.7142857143
10.7142857143 round to 10.71
For the function f(x)=−x^2+x−7, find f(1)
Answers
Answer:
f(1) = -7
Step-by-step explanation:
Step 1: Define
f(x) = -x² + x - 7
f(1) = x = 1
Step 2: Substitute and Evaluate
f(1) = -(1)² + 1 - 7
f(1) = -1 + 1 - 7
f(1) = -7
answer these questions


Answers
A. True
B.true
C. False
D.true
Question 3: 40, 24
COMMON CORE REVIEW
1. Find the percent of change from 32 feet to 79 feet. Round to the nearest tenth, if necessary. Then state whether the percent of change is percent of increase or percent of decrease.
2. Factor this expression: 27+108n
3. Factor this expression: 56x + 16
THANKS AGAIN!!
Answers
Answer:
146.9
Step-by-step explanation:
I'm not sure of the answer but if I'm wrong tell me I'll continue trying it is a pleasure to help you.

If 2log3 Y + log3 x² = 4. find y
Answers
Answer:
Step-by-step explanation:
9/x
1).find the next number in the sequencea). 3,6,9,12_b). 4,25,46,57_c). 4,25,46,67_d). 3,6,12,24,48_2). solve the following a). 36×(18-3 + 5) exponent2 ÷5
Answers
1).find the next number in the sequence (Answers in bold)
a). 3,6,9,12_15
b). 4,25,46,57_68
c). 4,25,46,67_88
d). 3,6,12,24,48_96
2). solve the following
a). 36×(18-3 + 5) exponent2 ÷5
\(36\cdot\frac{(18-3+5)^2}{5}\text{ = 36 }\cdot\frac{20^2}{5}=36\cdot\frac{400}{5}=36\cdot\text{ 80 = }2880\)Answer:
2880
Explain how each location given in the table is related to the origin.

Answers
Refer to the attachment

Homework part2 need help asap

Answers
The key features of the given quadratic functions are listed below.
What is the graph of a quadratic function?In Mathematics and Geometry, the graph of any quadratic function would always form a parabolic curve because it is a u-shaped. Based on the first quadratic function, we can logically deduce that the graph is an upward parabola because the coefficient of x² is positive and the value of "a" is greater than zero (0) i.e 3 > 0.
For the quadratic function y = 3x² - 5, the key features are as follows;
Axis of symmetry: x = 0.
Vertex: (0, -5).
Domain: [-∞, ∞]
Range: [-5, ∞]
For the quadratic function y = -2x² + 12x - 15, the key features are as follows;
Axis of symmetry: x = 3.
Vertex: (3, 3).
Domain: [-∞, ∞]
Range: [-∞, 3]
For the quadratic function y = -x² + 1, the key features are as follows;
Axis of symmetry: x = 0.
Vertex: (0, 1).
Domain: [-∞, ∞]
Range: [-∞, 1]
For the quadratic function y = 2x² - 16x + 30, the key features are as follows;
Axis of symmetry: x = 4.
Vertex: (4, -2).
Domain: [-∞, ∞]
Range: [-2, ∞]
Read more on quadratic functions here: brainly.com/question/24020644
#SPJ1

Select the two sequences of transformations that would show that triangles ABC and AED are similar.

Answers
The two sequences of transformations that would show that triangles ABC and AED are similar are:
Dilating ΔABC by scale factor 1/2 and reflect A'B'C' over AD.
Reflect AED over line AB and Dilate A'E'D' by scale factor 2.
What is Similarity?Two triangles are said to be comparable if their two sides are in the same ratio as the two sides of another triangle and their two sides' angles inscribed in both triangles are equal.
Given:
as, From the Figure we have to dilate the sides of ΔABC by scale factor 1/2.
Then, sides of Δ will be Similar with equal side ratio.
Then we can have,
Dilating ΔABC by scale factor 1/2 and reflect A'B'C' over AD.
or, Reflect AED over line AB and Dilate A'E'D' by scale factor 2.
Learn more about Similarity here:
https://brainly.com/question/26451866
#SPJ1
If h represents hours and w represents wage , then how much more does steph earn per hour than marco
Answers
Stephanie earns $1.5 per hour more than what Marco earns.
Linear equationLinear equation is in the form:
y = mx + b
where y, x are variables, m is the rate of change and b is the y intercept.
Let w represent the wages after h hours.
From the table using the points:
(15, 157.5) and (24, 252)The rate of change (m) for Stephanie is:
\(m=\frac{y_2-y_1}{x_2-x_1} =\frac{252-157.5}{24-15} =10.5\)This means that Stephanie earn $10.5 per hour.
From the equation: w = 9h, Marco earns $9 per hour
Hence, Stephanie earns $1.5 per hour more than what Marco earns.
Find out more on linear equation at: https://brainly.com/question/14323743

Simplify the variable expression by evaluating its numerical part, and enter
your answer below.
g- 16/4 +2
Answers
simplification of expression will be g - 2
Our expression is g - 16/4 + 2
16/4 = 4
so we c will write it as
g - 4 + 2
which can be further simplified to
g - 2
Therefore, our simplified expression comes out to be g - 2.
For more questions related to simplification
https://brainly.com/question/2804192
#SPJ1
The darkness of the print is measured quantitatively using an index. If the index is greater than or
equal to 2.0 then the darkness is acceptable. Anything less than 2.0 means the print is too light and
not acceptable. Assume that the machines print at an average darkness of 2.2 with a standard
deviation of 0.20.
(a) What percentage of printing jobs will be acceptable? (4)
(b) If the mean cannot be adjusted, but the standard deviation can, what must be the new standard
deviation such that a minimum of 95% of jobs will be acceptable?
Answers
84.13% of the printing jobs will be acceptable.
The new standard deviation required to achieve a minimum of 95% of jobs acceptable is 0.121.
The darkness of the print is measured quantitatively using an index. If the index is greater than or equal to 2.0 then the darkness is acceptable. Anything less than 2.0 means the print is too light and not acceptable. The machines print at an average darkness of 2.2 with a standard deviation of 0.20.
The mean of the darkness of the print is µ = 2.2 and the standard deviation is σ = 0.20.Therefore, the z-score can be calculated as; `z = (x - µ) / σ`.The index required for acceptable prints is 2.0. Thus, the percentage of prints that are acceptable can be calculated as follows;P(X ≥ 2.0) = P((X - µ)/σ ≥ (2.0 - 2.2) / 0.20)P(Z ≥ -1) = 1 - P(Z < -1)Using the standard normal table, P(Z < -1) = 0.1587P(Z ≥ -1) = 1 - 0.1587= 0.8413.
To find the new standard deviation, we can use the z-score formula.z = (x - µ) / σz = (2.0 - 2.2) / σz = -1Therefore, P(X ≥ 2.0) = 0.95P(Z ≥ -1) = 0.95P(Z < -1) = 0.05Using the standard normal table, the z-score value of -1.645 corresponds to a cumulative probability of 0.05. Hence,z = (2.0 - 2.2) / σ = -1.645σ = (2.0 - 2.2) / -1.645= 0.121.
for such more question on deviation
https://brainly.com/question/475676
#SPJ8