Describe how to find the square of -12
Answers
Related Questions
7 cm7(Score for Question 3: __ of 4 points)3. A) Find the probability that a point chosen randomly inside the rectangle willbe in the triangle. Show all calculations. Round answer to the nearesthundredth.3 cmAnswer:4 cm5 cm2 cm2 cmB) Find the probability that a point chosen randomly inside the rectangle willbe in the shaded region. Show all calculations. Round answer to the nearest hundredth.Answer:

Answers
In this case, we'll have to carry out several steps to find the solution.
Step 01:
Data:
Diagram
Step 02:
a.
Big Rectangle Area = s²
= 7 cm * 5 cm
= 35 cm²
Triangle Area = (b * h) / 2
= (3 cm * 4 cm) / 2
= 12 cm² / 2 = 6 cm²
probability = Triangle Area / Big Rectangle Area
= 6 cm² / 35 cm² = 6 / 35 = 0.171
b.
Small Rectangle Area = s²
= 2 cm * 2 cm
= 4 cm²
Shaded region Area = Big Rectangle Area - Triangle Area - Small Rectangle Area
Shaded region Area = 35 cm² - 6 cm² - 4 cm² = 25 cm²
probability = Shaded region Area / Big Rectangle Area
= 25 cm² / 35 cm² = 25 / 35 = 5 / 7 = 0.714
The answer is:
a. probability = 6 / 35 = 0.171
b. probability = 5 / 7 = 0.714
A giraffe runs at a rate of 32 miles per hour. Which equation models the situation?
h = time spent running, in hours
d = distance run in miles
32h = d
32d = h
h = 32 + d
h = 32 – d
Answers
Think also about the speed = distance / time equation
In this case rate basically means speed
Answer: 32h = d because when you rearrange you will get d/h = 32 where distance over hours = speed which in this case is 32 mph
Answer:
32h=d
Step-by-step explanation:
yea, this is big brain time lol
also edge lol
please help with geometry!!!! ‼️‼️‼️‼️ i will mark brainliest

Answers
Answer:
P = 156 units
Step-by-step explanation:
the perimeter (P) is the sum of the 3 sides of the triangle.
tangents from an external point to the circle are congruent , that is
HK = HJ ( substitute values )
13x - 8 = 7x + 4 ( subtract 7x from both sides )
6x - 8 = 4 ( add 8 to both sides )
6x = 12 ( divide both sides by 2 )
x = 2
Then
HK = HJ = 7x + 4 = 7(2) + 4 = 14 + 4 = 18
and
KI = IL = 21
then
GL = GJ = 60 - 21 = 39
Thus
P = HK + KI + IG + GJ + JH = 18 + 21 + 60 + 39 + 18 = 156 units
take another guess: a student takes a multiple-choice test that has 10 questions. each question has four choices. the student guesses randomly at each answer. round the answers to three decimal places.
Answers
Value of P(5) is 0.058 by probability.
What is a math illustration of probability?
The likelihood or chance of an event happening is known as probability. For instance, there is only one way to receive a head and there are a total of two possible outcomes, hence the probability of flipping a coin and getting heads is 1 in 2. (a head or tail). P(heads) = 12 is what we write.Given that ,
p = 1 / 4 = 0.25
1 - p = 1 - 0.25 = 0.75
n = 10
Using binomial probability formula ,
P(X = x) = ((n! / x! (n - x)!) * px * (1 - p)n - x
(a) x = 5
P(X = 5) = ((10! / 5! (10 - 5)!) * 0.255 * (0.75)10 - 5
= ((10! / 5! (5)!) * 0.255 * (0.75)5
= 0.058
P(5) = 0.058
(b)
P(X > 3) = 1 - P(X \leq 3)
= 1 - P(X = 0) - P(X = 1) - P(X = 2) - P(X = 3)
= 1 - 0.776
= 0.224
P(ore than 3) = 0.224
Learn more about probability
brainly.com/question/11234923
#SPJ9
The complete question is-
Take another guess: A student takes a multiple-choice test that has 10 questions. Each question has four choices. The student guesses randomly at each answer. Round the answers to three decimal places Part 1 of2 (a) Find P(5) P(5)- Part 2 of2 (b) Find P(More than 3) P(More than 3)
What is the quotient of 56÷34?
Answers
Answer:
56/34 = \(\frac{28}{17}\) or \(1\frac{11}{17}\) or 1.65
Answer:
56/34=1.65 or 1 \(\frac{13}{20}\)
Step-by-step explanation:
Learning Task 3: Solve the following problems. Write your SOLUTION and
your answers in you notebook.
1. What is the quotient if 24 is divided by 48?
2. Jean has 35 m of wire for hanging pictures. She wants to divide it into
50 pieces for her frames. How long will she use for each frame?
3. Father left Php5.00 for his 7 children. How much did each child
receive?
4. Mang Ricky is a hardworking man who owns 6 hectares of land. In his
will, he divided his lot equally among his 7 sons. How much land will
each of his son receive?
5. Troy and Raffy went to the market to buy 15 kilos of meat. When they
came home, they divided the meat into 16 parts and put each part in
separate plastic bags for future use. How many kilos of pork does each
bag contain?
Answers
1. The quotient is 0.5.
2. She will use 0.7m long for each frame.
3. Each child received 0.71 Php.
4. Each son will receive 0.85 hectares.
5. Each bag contains 0,93 kilos.
1. What is the quotient if 24 is divided by 48?24 / 48 = X0.5 = X2. Jean has 35 m of wire for hanging pictures. She wants to divide it into 50 pieces for her frames. How long will she use for each frame?35 / 50 = X0.7 = X
3. Father left Php5.00 for his 7 children. How much did each child receive?5/7 = X0.71 = X
4. Mang Ricky is a hardworking man who owns 6 hectares of land. In his will, he divided his lot equally among his 7 sons. How much land will each of his son receive?6/7 = X0.85 = X
5. Troy and Raffy went to the market to buy 15 kilos of meat. When they came home, they divided the meat into 16 parts and put each part in separate plastic bags for future use. How many kilos of pork does each bag contain?15/16 = X0,93 = X
Learn more about maths in https://brainly.com/question/25927059
Determine the distance between (2,-3) and (-3,9)
Answers
Answer:
I hope this is right, 13 units
Step-by-step explanation:
Sorry if this is wrong
How many units are in the sum of the lengths of the three altitudes in a triangle with sides $7,$ $24,$ and $25$
Answers
56 units are in the sum of the lengths of the three altitudes in a triangle with sides $7,$ $24,$ and $25$
Properties of triangles are:
A triangle has three sides and three angles.
The sum of the angles of a triangle is always 180 degrees.
The exterior angles of a triangle always add up to 360 degrees.
The sum of consecutive interior and exterior angle is supplementary.
How to solve?Given:
Height: ha = 7
Height: hb = 24
Height: hc = 25
Sum to sides is:
25 +7 + 24 =56 units.
Learn more about Triangles:
https://brainly.com/question/4313883
#SPJ4
Match the following rational expressions to their rewritten forms.
Rational Expressions:
x^2+x+4/x-2
x^2-x+4/x-2
x^2-4x+10/x-2
x^2-5x+16/x-2
Rewritten Forms:
(x-2)+6/x-2
(x+3)+10/x-2
(x+1)+6/x-2
(x-3)+10/x-2

Answers
Answer:
Answer image is attached.
Step-by-step explanation:
Given rational expressions:
\(1.\ \dfrac{x^2+x+4}{x-2}\\2.\ \dfrac{x^2-x+4}{x-2}\\3.\ \dfrac{x^2-4x+10}{x-2}\\4.\ \dfrac{x^2-5x+16}{x-2}\)
And the rewritten forms:
\((x-2)+\dfrac{6}{x-2}\\(x+3)+\dfrac{10}{x-2}\\(x+1)+\dfrac{6}{x-2}\\(x-3)+\dfrac{10}{x-2}\)
We have to match the rewritten terms with the given expressions.
Let us consider the rewritten terms and let us solve them one by one by taking LCM.
\((x-2)+\dfrac{6}{x-2}\\\Rightarrow \dfrac{(x-2)^{2}+6 }{x-2}\\\Rightarrow \dfrac{x^2-4x+4+6 }{x-2}\\\Rightarrow \dfrac{x^2-4x+10}{x-2}\)
So, correct option is 3.
\((x+3)+\dfrac{10}{x-2}\\\Rightarrow \dfrac{(x+3)(x-2)+10}{x-2}\\\Rightarrow \dfrac{(x^2+3x-2x-6)+10}{x-2}\\\Rightarrow \dfrac{x^2+x+4}{x-2}\)
So, correct option is 1.
\((x+1)+\dfrac{6}{x-2}\\\Rightarrow \dfrac{(x+1)(x-2)+6}{x-2}\\\Rightarrow \dfrac{x^{2} +x-2x-2+6}{x-2}\\\Rightarrow \dfrac{x^{2} -x+4}{x-2}\)
So, correct option is 2.
\((x-3)+\dfrac{10}{x-2}\\\Rightarrow \dfrac{(x-3)(x-2)+10}{x-2}\\\Rightarrow \dfrac{x^2-3x-2x+6+10}{x-2}\\\Rightarrow \dfrac{x^2-5x+16}{x-2}\)
So, correct option is 4.
The answer is also attached in the answer area.

Evaluate the expression 30-14divided by 2
Answers
Answer:
23
Step-by-step explanation:
You have to do the division first unless it is a fraction.
14/2 = 7
30 - 7 = 23
If it was a fraction (with 30 - 14 on top and 2 on the bottom) Then the answer would be 8 > 30 - 14 = 16/2 = 8
Hope this helps.
You might expect the correlation between the cost of an Internet supplier service and the download speed provided by the service to be:
A substantial negative correlation
A substantial positive correlation
A small correlation
Answers
There is likely to be a substantial positive correlation between the cost of an internet service provider and the download speed offered by the service.
The correlation between the cost of an Internet supplier service and the download speed provided by the service is likely to have a substantial positive correlation.
The correlation between variables measures the strength of their association with one another, and it ranges from -1 to +1. When two variables have a positive correlation, they are expected to move in the same direction, meaning that as one variable rises, the other variable also rises.
Conversely, when two variables have a negative correlation, they are expected to move in opposite directions, meaning that as one variable rises, the other variable falls.
The cost of an internet service provider and the download speed offered by the service are expected to have a positive correlation because, in general, the faster the download speed of an internet service, the more expensive the service is likely to be.
As a result, as the cost of an internet service provider rises, the download speed offered by the service is likely to increase as well. Therefore, there is likely to be a substantial positive correlation between the cost of an internet service provider and the download speed offered by the service.
To know more about positive correlation visit:
https://brainly.in/question/2717104
#SPJ11
Ben has $267.19 in his checking account and needs to pay $453.04 for his car payment before the end of the month. Approximately how much more money does Ben need to be able to make his car payment?
A.
$185.00
B.
$720.00
C.
$450.00
D.
$275.00
Answers
Calculate the perimeter and the area of the figure
use 3.14 for pi
Answers
Answer:
You have not provided enough information to solve this problem.
Step-by-step explanation:
is there a picture you can post?
Or is there a shape you can define?
I really wish I could help you, but I need exact numbers and an exact shape so I can form an equation to solve your problem.
Sheila picks 4 pounds of blueberries for 10 dollars. How many pounds can she pick for 1 dollar?.
Answers
The amount of blueberries he can pick for 1 dollar is 2.5 pounds.
What is unitary in maths?A unitary state. This has an identity element in math, in an algebra. Whose inverse is equivalent to its adjoint (in mathematics, linear algebra, mathematical study of a matrix or operator).
How do you explain the unitary method?The unitary technique, in its most basic form, is used to calculate the value of a single unit from a specified multiple. How to determine the value of one pen, for instance, if the cost of 40 pens is Rs. 400. Utilizing the unitary technique is possible.
We know that
Sheila has 4 pounds of blueberries for 10 dollars.
Then for 1 dollar she will have
By unitary method:-
10/4=2.5
Hence sheila has 2.5 pound of blueberry for 1 dollar
To learn more about unitary click on the following link
https://brainly.com/question/23423168
#SPJ4
Does this relationship represents a function? Explain why or why not. (F.IF.A.1)
y
-5
4
-2
-5.4
-2.1
2 B4
5
X
Edit
View
Insert
Format
Tools Table HELP PLEASE
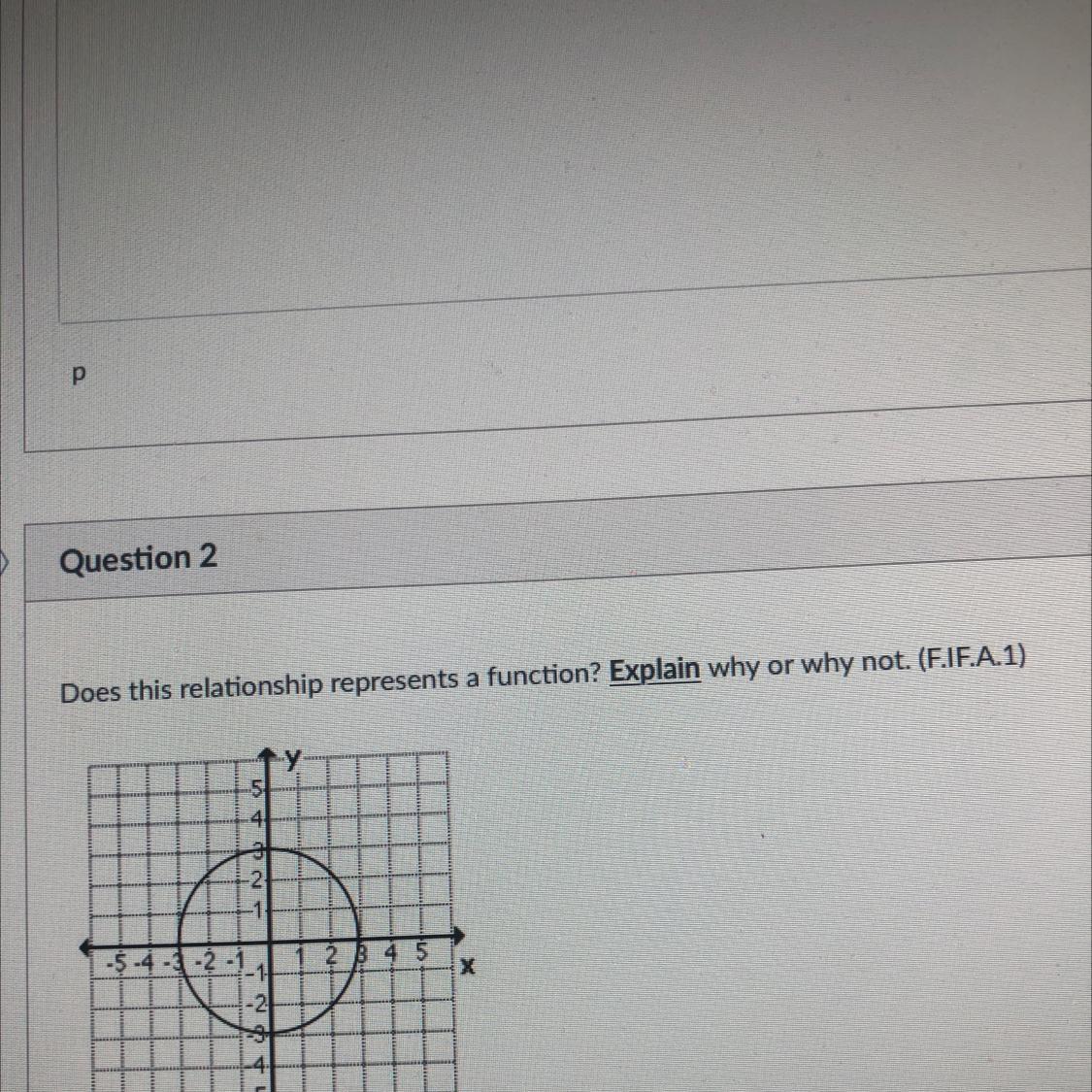
Answers
Step-by-step explanation:
no, it does not.
because almost every valid value of x (from -3 to +3) has 2 associated result values of y.
like x = 0 has y = 3 and y = -3.
but for a function there can be only ONE associated y value for every valid x value.
What is the population standard deviation of this data set?*
2 4 4 5 7 8
A. 0.5
B. 4.8
C. 2
D. 02.19
Answers
Answer:
C) 2
Step-by-step explanation:
step 1: Find mean of data set
2+4+4+5+7+8 = 30
30/6 = 5
Mean = 5
step 2: subtract each data value from the mean and square it
5-2 = 3; 3² = 9
5-4 = 1; 1² = 1
5-4 = 1; 1² = 1
5-5 = 0; 0² = 0
5-7 = -2; (-2²) = 4
5-8 = -3; (-3²) = 9
Add the squared results:
9+1+1+0+4+9 = 24
Divide 24 by 6 to get the Variance of 4
Take the square root of the Variance to get the Standard Deviation
\(\sqrt{4}\) = 2
find the value of x in the triangle shown below 1 4 x

Answers
Answer:
x = √17
Step-by-step explanation:
x² = 4² + 1²
x² = 17
x = √17
x² = y² + z²
x² = 4² + 1²
x² = 16 + 1
x² = 17
x = √17✅
0.8 divided by 0.6 then round to nearst hundredth
Answers
Answer: 1.33
Step-by-step explanation:
0.8 divided by 0.6= 1.33333333333
3 is in the hundredths place and 3 is in the thousandths place.
Since the digit in the thousandths place (3) is less than 5, the digit in the hundredths place (3) does not change.
The digits to the right of the hundredths place are dropped.
1.33333333 rounded to the nearest hundredth (two decimal places) = 1.33.
Solve for x: one fifth times the quantity 5 times x plus 10 end quantity plus 5 times x is greater than 32
a
x is less than eleven thirds
b
x is greater than eleven thirds
c
x < 5
d
x > 5
Answers
x is greater than eleven thirds.
How to solve inequality?Inequality are expression that have <, > , ≤ and ≥ .
Therefore,
1 / 5 (5x) + 10 + 5x > 32
Hence,
1 / 5 × 5x + 10 + 5x > 32
x + 10 + 5x >32
combine like terms
x + 10 + 5x >32
x + 5x + 10 > 32
Therefore,
x + 5x + 10 > 32
6x + 10 > 32
subtract 10 from both sides
6x + 10 - 10 > 32 - 10
6x > 22
divide both sides by 6
x > 22 / 6
x > 11 / 3
Therefore, x is greater than eleven thirds.
learn more on inequality here: https://brainly.com/question/2193595
#SPJ1
Dan uses the equation g left parenthesis x right parenthesis equals 5 x plus 50 to model the balance of his savings account after saving for x weeks. What is the value and meaning of g left parenthesis 60 right parenthesisblank in the context of the problem?
Answers
The value is 350.
From the question, we have
Dan uses the equation g(x)=5x+50 to model the balance of his savings account after saving for x weeks.
g(x)=5x+50
substituting the value x = 60, we get
g(60)=5*60+50
=300+50
=350
Multiplication:
Finding the product of two or more numbers in mathematics is done by multiplying the numbers. It is one of the fundamental operations in mathematics that we perform on a daily basis. Multiplication tables are the main use that is obvious. In mathematics, the repeated addition of one number in relation to another is represented by the multiplication of two numbers. These figures can be fractions, integers, whole numbers, natural numbers, etc. When m is multiplied by n, either m is added to itself 'n' times or the other way around.
To learn more about multiplication visit: https://brainly.com/question/5992872
#SPJ9
Consider the following proability density function: f(x) = { kx. 0<= x< 2, k(4-x). 2<= x <=4, 0. otherwise. find the value of k for which f(x) is a valid probability density funcion
Answers
The value of K for which f(x) is a valid probability density function is 1/4.
How to solve for the value of K\(\int\limits^4_0 {fx(x)} \, dx =1\)
\(\int\limits^2_0 {Kx} \, dx +\int\limits^4_2 {K(4-x)} \, dx =1\)
\(K[\frac{2^2}{2} -0]+[K[4(4-2)-(\frac{4^2}{2} -\frac{2^2}{2} )]=1\)
open the equation
\(K\frac{4}{2}+K[8 - (\frac{16}{2} -\frac{4}{2} )] = 1\\\)
\(2K+K[\frac{4}{2} ]=1\)
2K + 2K = 1
4K = 1
divide through by 4
K = 1/4
Read more on probability density function here
https://brainly.com/question/15714810
#SPJ4
Base: h=12yd, b=51yd
face 1: l=5yd, w=37yd
face 2: l=5yd, w=20yd
face 3: l=5yd, w=51yd
enter numerical value only.
sa = _____ yd2
Answers
The surface area of the rectangular prism with the given dimensions is 540yd².
To find the surface area (sa) of the rectangular prism with the given dimensions, we need to calculate the area of each face and add them together.
The formula for the area of a rectangle is length multiplied by width (A = l x w).
Face 1 has a length of 5yd and a width of 37yd, so its area is 5 x 37 = 185yd².
Face 2 has a length of 5yd and a width of 20yd, so its area is 5 x 20 = 100yd².
Face 3 has a length of 5yd and a width of 51yd, so its area is 5 x 51 = 255yd².
To find the total surface area, we add the areas of all three faces:
sa = area of face 1 + area of face 2 + area of face 3
sa = 185yd² + 100yd² + 255yd²
sa = 540yd²
Therefore, the numerical value of the surface area of the rectangular prism with the given dimensions is 540yd².
To know more about surface area, refer to the link below:
https://brainly.com/question/14987814#
#SPJ11
what is the rule of this translation?

Answers
Answer:
(7,11) 20 characters long
A sphere has a volume that is 36 cubic meters. Find the radius of the sphere.

Answers
Answer:
2.05m
Step-by-step explanation:
The Olympiad task For the 10th grade if you do not know how to solve it immediately sayCorrect answer 2

Answers
Given:-
\(f(\frac{1}{x+1})=\frac{1}{2}f(x),f(1-x)=1-f(x)\)To find:-
\(s_n=f(1)+f(\frac{1}{2})+f(\frac{1}{3})+\cdots+f(\frac{1}{n})\)Now we simplify above equation to solve it,
\(\begin{gathered} f(1)+f(\frac{1}{2})+f(\frac{1}{3})+\cdots+f(\frac{1}{n})=f(1)+f(\frac{2-1}{2})+f(\frac{3-2}{3})+\cdots+f(\frac{n+1-n}{n}) \\ f(1)+f(\frac{1}{2})+f(\frac{1}{3})+\cdots+f(\frac{1}{n})=f(1)+f(1-\frac{1}{2})+f(1-\frac{2}{3})+\ldots+f(1-\frac{(n-1)}{n}) \\ f(1)+f(\frac{1}{2})+f(\frac{1}{3})+\cdots+f(\frac{1}{n})=f(1)+1-f(\frac{1}{2})+1-f(\frac{2}{3})+\cdots+1-f(\frac{n-1}{n}) \\ f(1)+f(\frac{1}{2})+f(\frac{1}{3})+\cdots+f(\frac{1}{n})=f(1)-f(\frac{1}{2})-f(\frac{2}{3})-\cdots-f(\frac{n-1}{n})+1(n-1) \\ f(1)+f(\frac{1}{2})+f(\frac{1}{3})+\cdots+f(\frac{1}{n})=f(1)-f(\frac{1}{1+1})-f(\frac{2}{2+1})-\cdots-f(\frac{n-1}{(n-1)+1})+1(n-1) \end{gathered}\)By furthur simplification we get,
\(\begin{gathered} f(1)-f(\frac{1}{1+1})-f(\frac{2}{2+1})-\cdots-f(\frac{n-1}{(n-1)+1})+1(n-1)=f(1)-\frac{1}{2}f(1)-\frac{1}{2}f(2)-\cdots-\frac{1}{2}f(n-1)+1(n-1)_{} \\ \text{ =}-\frac{1}{2}f(1)-\frac{1}{2}f(2)-\cdots-\frac{1}{2}f(n-1)+1(n-1) \\ \text{ =-}\frac{1}{2}\lbrack f(1)+f(2)+...+f(n-1)\rbrack+1(n-1) \end{gathered}\)So the requiired answer is,
\(\text{-}\frac{1}{2}\lbrack f(1)+f(2)+...+f(n-1)\rbrack+1(n-1)\)A plumber has a very long piece of pipe that is used to run city water parallel to a major roadway. The pipe is cut into two sections. One section of pipe is 12 ft. shorter than the other. If 3 of the length of the shorter pipe is 120 ft., how long is the longer piece of the pipe?
Answers
If a plumber has a very long piece of pipe that is used to run city water parallel to a major roadway. The length of the longer piece of the pipe is: 172 ft.
How to find the length?Let x represent the longer piece
Let x - 12 represent the shorter piece
So,
(3)(x - 12) = 120
Multiply both sides by 4/3
x - 12 = 120 (4/3)
x - 12 = 160
Add 12 to both sides
x = 172 ft (longer piece)
Therefore the length of the longer piece is 172 ft.
Learn more about length here:https://brainly.com/question/2217700
#SPJ1
The correct question is:
A plumber has a very long piece of pipe that is used to run city water parallel to a major roadway. The pipe is cut into two sections. One section of the pipe is 12 ft. shorter than the other. If 3/4 of the length of the shorter pipe is 120 ft., how long is the longer piece of the pipe?
plz someone help me with this

Answers
Hey, There i hope you are haveing a great day :) So I look at the example so it should be the same I think like how you work out the mean by adding all the numbers and then diving them so, (8 + 4 + 8 + 8 + 10 + 2 + 4 + 4) = 48 and now you know you have got 8 numbers there a divide them so 48/8 = 6. and then I think you take away it so, 8 - 6 = 2 4 - 6 =-2 8 - 6 = 2 8 - 6 = 2 10 - 6 = 4 2 - 6 = -4 4 - 6 = -2 4 - 6 = -2 and then 2 + -2 + 2 + 2 + 4 + -4 + -2 + -2 divide 8 = 0 and you can't divide anything by 0 so that would be the answer.
What type of function is represented by the table
X= -4 -3 -2 -1
Y= 16 8 4 2
Answers
The function represented by the table is: \(Y = (1/2)^X\).This is an example of an exponential function.
What is Algebraic function ?
An algebraic function is a function created by applying the operation of addition, subtraction, multiplication, division, and extracting the nth root
To determine the type of function represented by the table, we can look at the pattern of the values of X and Y.
As we can see from the table, as X increases by 1, Y is divided by 2. This means that the relationship between X and Y is not linear but rather exponential. Specifically, the function is of the form Y = \(a(1/2)^X,\) where a is a constant.
To find the value of a, we can use one of the points in the table. Let's use the first point, (-4, 16):
16 = \(a(1/2)^(-4)\)
Multiplying both sides by\((1/2)^(-4)\):
\(16 * (1/2)^4\) = a
a = 16 * (1/16) = 1
Therefore, the function represented by the table is:
\(Y = (1/2)^X\)
This is an example of an exponential function.
Learn more about Root here
https://brainly.com/question/16932620
#SPJ1
While playing a real-time strategy game, Dan created military units for battle : long swordsmen, spearmen, and crossbowmen. Long swordsman require 20 units of food and 10 units of gold. Spearmen require 35 units of food and 40 units of wood. Crossbowmen require 40 units of wood and 30 units of gold. If Dan used 1300 units of gold, 2400 units of wood and 1375 units of food to create the units, how many of each type of military unit did he create?
Answers
We can set up a system of equations to represent the number of each type of unit Dan created. Let x be the number of long swordsmen, y be the number of spearmen, and z be the number of crossbowmen. We know that the total number of units of each resource used to create the units must equal the amount of that resource that Dan has. We can write the following system of equations to represent this:
20x + 35y + 40z = 1375 (1)
10x + 40y + 30z = 2400 (2)
30z + 40z = 1300 (3)
Equation (1) represents the total number of units of food used to create the units, equation (2) represents the total number of units of wood used, and equation (3) represents the total number of units of gold used.
To solve this system of equations, we can use substitution. First, we'll solve for z in equation (3) by dividing both sides by 70:
z = 1300 / 70
z = 18.57
Since z represents the number of crossbowmen, it must be an integer. Since 18 is the largest integer less than 18.57, we know that Dan created 18 crossbowmen.
Now we can substitute 18 for z in equation (1) to find the number of long swordsmen Dan created:
20x + 35y + 40(18) = 1375
20x + 35y + 720 = 1375
20x + 35y = 655
We can divide both sides of this equation by 55 to solve for y:
(20x + 35y) / 55 = 655 / 55
y = 19
Since y represents the number of spearmen, it must be an integer. Since 19 is the largest integer less than 19.57, we know that Dan created 19 spearmen.
Now we can substitute 18 for z and 19 for y in equation (2) to find the number of long swordsmen Dan created:
10x + 40(19) + 30(18) = 2400
10x + 760 + 540 = 2400
10x = 1200
x = 120
Since x represents the number of long swordsmen, it must be an integer. Therefore, we know that Dan created 120 long swordsmen.
In total, Dan created 120 long swordsmen, 19 spearmen, and 18 crossbowmen.
HELP ASAP IM BEING TIMEDD

Answers
Answer:
53/100
Step-by-step explanation:
First, we convert the fraction to a decimal number by dividing the numerator by the denominator:
8 / 15 = 0.533
There are two parts to the decimal number above:
Integer Part: 0
Fractional Part: 533
Now, we will make the Fractional Part just two digits (nearest hundredth) by using our rounding rules.*
In this case, Rule I applies, so 8/15 (or 0.533) rounded to the nearest hundredth in decimal format is:
0.53
Next, we will make 8/15 rounded to the nearest hundredth in fraction format. Since you can divide our decimal format answer above by 1 and keep the same value, you can make it like this:
0.53 = 0.53/1
Then, we multiply the numerator and denominator by 100 to get rid of the decimal point:
(0.53 x 100) / (1 x 100) = 53/100
That's it. 8/15 rounded to the nearest hundredth is displayed below (simplified if necessary):
53/100
Answer:
53.33% is the correct answer