Circles and polygons, look at picture (questions 8&9)

To maximize the seating area while using 45π + 60 meters of light strips, the radius of the semicircular stage should be approximately 29π/3 - 5 meters.

To maximize the seating area, we need to determine the dimensions of the rectangular auditorium that will give us the largest possible area while using the given length of light strips.

Let the length of the rectangular auditorium be L, and its width be W.

The seating area consists of the rectangular portion minus the semicircular stage. So, the seating area's length is L - 2r (subtracting the semicircle's diameter) and the seating area's width is W - 2r.

The perimeter of the seating area is the sum of the lengths of its four sides, excluding the semicircular stage. The perimeter is given as 45π + 60 meters.

Perimeter = 2(L - 2r) + 2(W - 2r) + πr = 45π + 60

Simplifying: 2L + 2W - 8r + πr = 45π + 60

Rearranging: 2L + 2W = 8r + 44π + 60

The area of the seating area is given by A = (L - 2r)(W - 2r).

We want to maximize A, so we need to express it in terms of a single variable. Since we have an equation with two variables (L and W), we can rewrite one of the variables in terms of the other.

Rearranging the perimeter equation: 2L + 2W = 8r + 44π + 60

Solving for L: L = (8r + 44π + 60 - 2W) / 2

Substituting L in terms of W into the area equation: A = [(8r + 44π + 60 - 2W) / 2 - 2r] (W - 2r)

Simplifying: A = (4r + 22π + 30 - W) (W - 2r)

Now we have the area equation in terms of a single variable, W. To maximize A, we can take the derivative of A with respect to W, set it equal to zero, and solve for W.

dA/dW = 2(4r + 22π + 30 - W) - (W - 2r) = 0

Solving for W: 8r + 44π + 60 - W = W - 2r

Simplifying: 10r + 44π + 60 = 2W

W = 5r + 22π + 30

Now that we have W in terms of r, we can substitute this expression back into the area equation to get the area in terms of r only.

A = (4r + 22π + 30 - (5r + 22π + 30)) ((5r + 22π + 30) - 2r)

Simplifying: A = (r - 22π) (3r + 22π + 30)

Expanding: A = 3r² + 8rπ + 30r - 66πr - 660π

Now, to find the maximum area, we can take the derivative of A with respect to r, set it equal to zero, and solve for r.

dA/dr = 6r + 8π + 30 - 66π = 0

Simplifying: 6r - 58π + 30 = 0

6r = 58π - 30

r = (58π - 30) / 6

r ≈ 29π/3 - 5

Therefore, to maximize the seating area while using 45π + 60 meters of light strips, the radius of the semicircular stage should be approximately 29π/3 - 5 meters.

Learn more about maximum area click;

https://brainly.com/question/29635164

#SPJ12

Answer:

0.083

Step-by-step explanation:

I think

Answer:

use formula

slope m =(y2-y1)/(x2-x1)

Step-by-step explanation:

for first one

(x1,y1)=(2,1)

(x2,y2)=(0,-3)

now

slope m =(y2-y1)/(x2-x1)=(-3-1)/(0-2)=-4/-2=2

try other thank you hope it helps

Answer:

x-8/x-6

Step-by-step explanation:

Step by Step Solution

More Icon

STEP

1

:

              8x - 32  

Simplify   —————————————

           x2 - 10x + 24

STEP

2

:

Pulling out like terms

2.1     Pull out like factors :

  8x - 32  =   8 • (x - 4)

Trying to factor by splitting the middle term

2.2     Factoring  x2 - 10x + 24

The first term is,  x2  its coefficient is  1 .

The middle term is,  -10x  its coefficient is  -10 .

The last term, "the constant", is  +24

Step-1 : Multiply the coefficient of the first term by the constant   1 • 24 = 24

Step-2 : Find two factors of  24  whose sum equals the coefficient of the middle term, which is   -10 .

     -24    +    -1    =    -25

     -12    +    -2    =    -14

     -8    +    -3    =    -11

     -6    +    -4    =    -10    That's it

Step-3 : Rewrite the polynomial splitting the middle term using the two factors found in step 2 above,  -6  and  -4

                    x2 - 6x - 4x - 24

Step-4 : Add up the first 2 terms, pulling out like factors :

                   x • (x-6)

             Add up the last 2 terms, pulling out common factors :

                   4 • (x-6)

Step-5 : Add up the four terms of step 4 :

                   (x-4)  •  (x-6)

            Which is the desired factorization

Canceling Out :

2.3    Cancel out  (x-4)  which appears on both sides of the fraction line.

Equation at the end of step

2

:

    ((x2)-4x)     8

 ———————————————-———

 (((x2)-10x)+24) x-6

STEP

3

:

              x2 - 4x  

Simplify   —————————————

           x2 - 10x + 24

STEP

4

:

Pulling out like terms

4.1     Pull out like factors :

  x2 - 4x  =   x • (x - 4)

Trying to factor by splitting the middle term

4.2     Factoring  x2 - 10x + 24

The first term is,  x2  its coefficient is  1 .

The middle term is,  -10x  its coefficient is  -10 .

The last term, "the constant", is  +24

Step-1 : Multiply the coefficient of the first term by the constant   1 • 24 = 24

Step-2 : Find two factors of  24  whose sum equals the coefficient of the middle term, which is   -10 .

     -24    +    -1    =    -25

     -12    +    -2    =    -14

     -8    +    -3    =    -11

     -6    +    -4    =    -10    That's it

Step-3 : Rewrite the polynomial splitting the middle term using the two factors found in step 2 above,  -6  and  -4

                    x2 - 6x - 4x - 24

Step-4 : Add up the first 2 terms, pulling out like factors :

                   x • (x-6)

             Add up the last 2 terms, pulling out common factors :

                   4 • (x-6)

Step-5 : Add up the four terms of step 4 :

                   (x-4)  •  (x-6)

            Which is the desired factorization

Canceling Out :

4.3    Cancel out  (x-4)  which appears on both sides of the fraction line.

Equation at the end of step

4

:

   x        8  

 ————— -  —————

 x - 6    x - 6

STEP

5

:

Adding fractions which have a common denominator :

5.1       Adding fractions which have a common denominator

Combine the numerators together, put the sum or difference over the common denominator then reduce to lowest terms if possible:

x - (8)     x - 8

———————  =  —————

  x-6       x - 6

Final result :

 x - 8

 —————

 x - 6

Answer:

ya

Step-by-step explanation:

25 percent is equally to 25 over 100 which is 1over4

Answer:

I dont know if this is supposed to be solved a certain way for a class, but as a bargain shopper, this is what I would do to figure out the best value.

Step-by-step explanation:

First divide $10 by 4:   10.00/4=2.5 or $2.50 per card

Then, divide $14 by 6:  14.00/6=2.3333333 so about $2.33 per card

Now, just look at the two answers and see which one is less.

If you buy 4 cards for $10, you will pay $2.50 for each card and if you buy 6 cards for $14, you will pay about $2.33 for each card. Therefore, the better value would be the 6 cards for $14.

The domain of the function h(x)=9x/x(x²-49) is (-∞, -7) ∪ (-7, 0) ∪ (0, 7) ∪ (7, ∞).

In math the term called the domain of a function is the set of its possible inputs which is the set of input values where for which the function is defined.

Here we have know the function is h(x)=9x/x(x²-49)

And we need to find the domain of the function.

Here we know that this function is defined for values where the denominator is not equal to zero.

Which is written as,

=> x(x²-49) ≠ 0

When we elaborate the equation based on the difference between the two squares property, it can be written as,

=> x(x + 7)(x - 7)  ≠ 0

Based on this we have identified that the value of x must not be

=> x  ≠ -7, 7, 0

Then the domain of the function is written as,

=> (-∞, -7) ∪ (-7, 0) ∪ (0, 7) ∪ (7, ∞).

To know more about domain here.

https://brainly.com/question/13113489

#SPJ4

Answer:

15/100×900

=135..........

It safe to assume that n ≤ 0.05 of all subjects in the population. We know that n is the sample size. However, the entire population size is not given in the question. Hence, we cannot assume that n ≤ 0.05 of all subjects in the population.

The answer is "Yes".

Therefore, the answer is "No". Verify np(1−p) ≥ 10, where

n = 100 and

p = 0.66

np(1−p) = 100 × 0.66(1 - 0.66)

≈ 100 × 0.2244

≈ 22.44 Since np(1−p) ≥ 10, the sample is considered large enough to use the normal distribution to model the sample proportion. Thus, the answer is "Yes".c. Null hypothesis H0: p = 0.66 Alternative hypothesis Ha: p < 0.66d. The sample proportion is:

p = 10/100

= 0.1. The test statistic is calculated using the formula:

z = (p - P)/√[P(1 - P)/n] where P is the population proportion assumed under the null hypothesis

P = 0.66z

= (0.1 - 0.66)/√[0.66 × (1 - 0.66)/100]

≈ -4.85 Therefore, the test statistic is -4.85 (rounded to two decimal places).e. To determine the p-value, we look at the area under the standard normal curve to the left of the test statistic. Using a table or calculator, we find that the area is approximately 0. Thus, the p-value is less than 0.0001 (rounded to 4 decimal places). Since the p-value is less than

α = 0.05, we reject the null hypothesis. Thus, there is sufficient evidence to support the claim that 66% of people mind if others smoke near a building entrance has decreased. Therefore, the answer is "There is sufficient evidence to support the claim that 66% of people mind if others smoke near a building entrance has decreased".

To know more about assume visit:

https://brainly.com/question/14442050

#SPJ11

The attached figure represents the box and whisker plots

To do this, we start by converting the tallies in the frequency table to numerical values.

So, we have:

                Monday      Tuesday      Wednesday     Thursday   Friday

On Time     6                   3                     2                     10             10

Late             1                   0                     3                     4               10

Next, we enter these data on a graphing/statistical calculator to create the box and whisker plots

See attachment

Read more about box and whisker plot at:

https://brainly.com/question/12343132

#SPJ1

(a) the approximate solution for the given equation within the interval 0.2 ≤ x ≤ 0.3 is approximately x ≈ 0.2875.

(b) the number of iterations cannot be negative, we can conclude that at least 0 iterations are necessary to obtain approximations accurate to within 10^-5.

(a) Using three iterations of the Bisection method, the approximate solution for the equation X cos x - 2x² + 3x - 1 = 0, within the interval 0.2 ≤ x ≤ 0.3, can be obtained as follows:

Iteration 1:

- Interval [a₁, b₁] = [0.2, 0.3]

- Midpoint c₁ = (a₁ + b₁) / 2 = (0.2 + 0.3) / 2 = 0.25

- Evaluate f(c₁) = c₁ cos c₁ - 2c₁² + 3c₁ - 1 = 0.25 cos 0.25 - 2(0.25)² + 3(0.25) - 1 ≈ -0.050

Since f(c₁) < 0, the root lies in the right half of the interval.

Iteration 2:

- Interval [a₂, b₂] = [0.25, 0.3]

- Midpoint c₂ = (a₂ + b₂) / 2 = (0.25 + 0.3) / 2 ≈ 0.275

- Evaluate f(c₂) ≈ -0.021

Since f(c₂) < 0, the root still lies in the right half of the interval.

Iteration 3:

- Interval [a₃, b₃] = [0.275, 0.3]

- Midpoint c₃ = (a₃ + b₃) / 2 ≈ 0.2875

- Evaluate f(c₃) ≈ 0.002

Since f(c₃) > 0, the root lies in the left half of the interval.

Thus, the approximate solution for the given equation within the interval 0.2 ≤ x ≤ 0.3 is approximately x ≈ 0.2875.

(b) To estimate the number of iterations necessary to obtain approximations accurate to within 10^-5, we can use the formula n ≥ (log(b - a) - log(TOL)) / log(2), where n represents the number of iterations, TOL is the desired tolerance (10^-5), and [a, b] is the initial interval.

In this case, [a, b] = [0.2, 0.3] and TOL = 10^-5. Substituting these values into the formula, we have:

n ≥ (log(0.3 - 0.2) - log(10^-5)) / log(2)

n ≥ (log(0.1) + 5) / log(2)

Using logarithmic properties, we can simplify this to:

n ≥ (1 - log(10)) / log(2)

n ≥ (1 - 1) / log(2)

n ≥ 0

Since the number of iterations cannot be negative, we can conclude that at least 0 iterations are necessary to obtain approximations accurate to within 10^-5.

In summary, three iterations of the Bisection method are used to approximate the solution for the given equation within the specified interval. To estimate the number of iterations necessary for a desired accuracy, a formula involving the initial interval and the tolerance is used. In this case, the number of iterations required is estimated to be 0 or more.

To learn more about Bisection method, click here: brainly.com/question/28138570

#SPJ11

Answer:

y=-3x + 32

Hope this

Answer:

h=15 m

Step-by-step explanation:

the formula for figuring out triangle side lengths is called the pythagorean theorem. it says a^2+b^2=c^2. c is always the hypotenuese of the triangle. we are trying to find he height not the hypotenuese so we say c^2-b^2=a^2.

17^2-8^2=h

289-64=225

h= the square root of 225

h= 15 m

Using trigonometric ratio, the length of AB is 3.4ft

Trigonometric ratios are the ratios of the length of sides of a triangle. These ratios in trigonometry relate the ratio of sides of a right triangle to the respective angle. The basic trigonometric ratios are sin, cos, and tan, namely sine, cosine, and tangent ratios. The other important trig ratios, cosec, sec, and cot, can be derived using the sin, cos, and tan respectively.

In this problem, to find AB, we have to use the cosine of the angle.

Cos θ = adjacent / hypothenuse

cos 28 = 3 / AB

AB = 3 / cos 28

AB = 3.4ft

The hypothenuse is 3.4ft

Learn more on trigonometric ratio here;

https://brainly.com/question/24349828

#SPJ1

The proportional relationship between the cost and the weight is c = 2.2w.

A proportional relationship is used to show the constant od proportionality between the variables.

One bag weighs 2.4 pounds and costs $5.28. The constant will be:

= 5.28/2.4

= 2.2

This can be expressed as c = 2.2w

where c = cost

w = weight

This shows the relationship.

Learn more about proportional relationship on:

brainly.com/question/2143065

#SPJ1

Answer: did you make that up ??

Step-by-step explanation:

Answer:

A. Alternate interior

B. Transitive property

C. Converse alternate interior angles theorem.

Answer:

144 dollars

Step-by-step explanation:

We are told that:

Lewis and his sister both begin with a balance of zero dollars.

Now, for every 4 dollars that Lewis saves in his account, his sister saves 9 dollar.

Now, if Lewis has 64 dollars in his account, it means he saved 4 dollars for 64/4 times or 16 times.

Therefore his sister saved 9 dollars for 16 times too.

Thus, his sister saved: 16 × 9 = 144 dollars

(a) The integers which divide 8 are -8, -4, -2, -1, 1, 2, 4, and 8. This collection is finite, as there are only eight elements in it.

(b) The integers which 8 divides are 8, 16, -8, -16, 24, -24, and so on. This collection is countably infinite, as it can be put into a one-to-one correspondence with the set of integers.

(c) The functions from N to N are uncountably infinite, since there are infinitely many possible functions from one countably infinite set to another.

(d) The set of strings over the English alphabet is uncountably infinite, since each string can be thought of as a binary string of infinite length, with each character representing a 0 or 1.

(e) The set of finite-length strings drawn from a countably infinite alphabet, A, is countably infinite, since it can be put into a one-to-one correspondence with the set of natural numbers.

(f) The set of infinite-length strings over the English alphabet is uncountably infinite, since it can be thought of as a binary string of infinite length, with each character representing a 0 or 1, and there are uncountably many such strings.
(a) The integers which divide 8: This set is finite, as there are a limited number of integers that evenly divide 8 (i.e., -8, -4, -2, -1, 1, 2, 4, and 8).

(b) The integers which 8 divides: This set is countably infinite, as there are infinitely many multiples of 8 (i.e., 8, 16, 24, 32, ...), and they can be put into one-to-one correspondence with the natural numbers.

(c) The functions from N to N: This set is uncountably infinite, as there are infinitely many possible functions mapping natural numbers to natural numbers, and their cardinality is larger than that of the natural numbers (i.e., it has the same cardinality as the power set of natural numbers).

(d) The set of strings over the English alphabet: This set is countably infinite, as there are infinitely many possible finite-length strings, but they can be enumerated in a systematic way (e.g., listing them by length and lexicographic order).

(e) The set of finite-length strings drawn from a countably infinite alphabet, A: This set is countably infinite, as each string has a finite length and can be enumerated in a similar manner to the English alphabet case.

(f) The set of infinite-length strings over the English alphabet: This set is uncountably infinite, as there are infinitely many possible infinite-length strings, and their cardinality is larger than that of the natural numbers (i.e., it has the same cardinality as the real numbers).

Learn more about integers here: brainly.com/question/15276410b

#SPJ11

The statement is false. When an economy shrinks at a constant annual rate, the cumulative decline over multiple years is not simply the sum of the annual rates of decline.

To calculate the cumulative decline over the four-year period, we need to use the concept of compound growth/decline.

If the economy shrinks at a rate of 10% per year for four consecutive years, the actual cumulative decline can be calculated as follows:

Cumulative decline = (1 - Rate of decline) ^ Number of years

In this case, the rate of decline is 10% or 0.1, and the number of years is 4.

Cumulative decline = (1 - 0.1) ^ 4

Cumulative decline = 0.9 ^ 4

Cumulative decline = 0.6561

So, the economy would actually shrink by approximately 65.61% over the four-year period, not 40%.

Learn more about   statement   from

https://brainly.com/question/27839142

#SPJ11

Answer:

C- m=102

Step-by-step explanation:

Just took the test on edg. :)

The value of m of the given parallel lines is; m = 102°

From the transversal line theorem, it states that If two parallel lines are cut by a transversal, then the pairs of consecutive interior angles formed are supplementary .

Now, from the given question we see that Horizontal and parallel lines e and f are cut by transversal d. Thus, if the bottom right angle is 78 degrees, then the top right angle which is m degrees is supplementary to it and as such is;

m = 180 - 78

m = 102

Read more about Transversal angle at; https://brainly.com/question/2141319

#SPJ9

3 = x/2

x = 3 * 2

x = 6

.......

Answer:

x=6

Step-by-step explanation:

cross multiply

x=3*2

x=6

Answer:

Step-by-step explanation:

f(x) = 3x - 2

h(x) = - 2x² + 3x - 6

First to find f(7/3) substitute the value of x that's 7/3 into f (x)

That's

To find h(-2) substitute the value of x that's - 2 into h(x)

We have

So we have

We have the final answer as

Hope this helps you

Answer:

reflexive property

Step-by-step explanation:

reflexive property states that a number equals itself, a = a, so since 16 = 16, or 16 equals itself, it's reflexive.

Answer:

7

Step-by-step explanation:

First you do the distributive property

3x -15=6

Second add 15 from both sides

3x=21

Divided by 3

×=7

Answer:

The constant of proportionality is 2.5 dollar per cupcake

Step-by-step explanation:

the required equation would be \(y=2.5x\).

Answer:

$2.50

Step-by-step explanation:

trust me I know I'm right

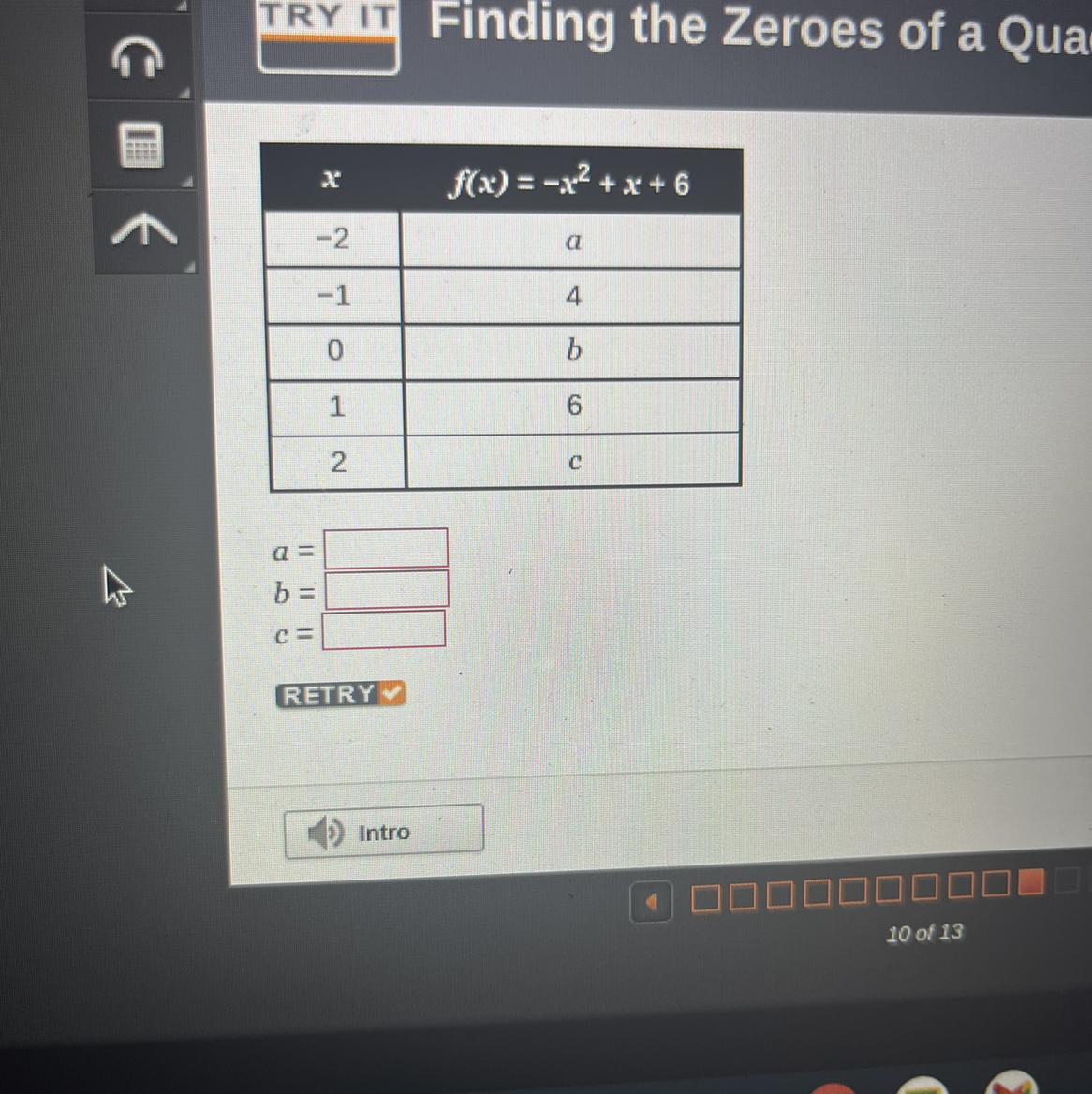
Answer:

Step-by-step explanation:

The probability of rolling a 1 is 0.85; then the probability of rolling not 1 is

1 - 0.85 = 0.15

Each box that states rolling a 1 gets 0.85. Each box that states not rolling 1 gets 0.15.

                                   second

                first              roll

                roll            

                             /     0.85   1

       1        0.85 /

                           \    0.15     not 1

                             /  0.85   1

not 1           0.15

                         \

                            \    0.15     not 1

Answer:

Option в. 42°

Step-by-step explanation:

According to the recipe a fraction of 7/3 cups of milk is required for 1 cup of flour.

What is fraction?

In mathematics, a fraction is used to denote a portion or component of the whole. It stands for the proportionate pieces of the whole. Numerator and denominator are the two components that make up a fraction. The numerator is the number at the top, and the denominator is the number at the bottom.

The amount of milk required for the recipe is = 1 and 3/4 cups

The amount of flour required for the recipe is = 3/4 cups

All the measurements are given in fractional form.

The amount of milk required for the recipe is -

= 1 + 3/4

= (4 + 3)/4

= 7/4 cups

So, a recipe requires 7/4 cups of milk for every 3/4 of flour.

Find the amount of milk required for 1 cup of flour = 4/4 cups of flour

If 7/4 cup of milk is required for 3/4 flour,

Then only 1/3  of 7/4 cup milk is required for 1/4 flour.

1/3 × 7/4 = 7/12 cups of milk

For 4/4 cups of flour 4 times more than 7/12 cups of milk is required -

4 × 7/12 = 7/3 cups of milk

Therefore, the amount of milk is 7/3 cups.

To learn more about fraction from the given link

https://brainly.com/question/20323470

#SPJ1

A recipe requires 1 and 3/4 cups of milk for every 3/4 of flour? How much milk is required for every cup of flour? Enter your number as a whole number, proper fractions or simplest form?

Answer:

Step-by-step explanation:

Answer is 5

2 + 5  = 7

3 + 5     8

The missing values are a = 0, b= 6 and c=4.

In mathematics, a function is an expression, rule, or law that establishes the relationship between an independent variable and a dependent variable. In mathematics, functions exist everywhere, and they are crucial for constructing physical links in the sciences.

We have function,

f(x) = -x² + x + 6

At x= -2

f(-2) = -(-2)² + (-2) + 6 = -4 -2  + 6= 0

At x = 0

f(0) = -0² + 0 + 6= 6

At x = 2

f(2) = -2² + 2 +6 = -4 + 8= 4

Learn more about Function here:

https://brainly.com/question/12431044

#SPJ5