Answers
Answer:
Look at the picture attached.
Step-by-step explanation:
Your x-intercept is 6 and your y-intercept is 4, that is where your 2 points will go. According to the slope, you should go up 2 and over 3, if you don't want to find the x-intercept. Then you would just plot the y-intercept which is 4, and then go up 2, over three and plot the next.
Hope this helps! :)

Answer:
Answer is in the picture, hope i got it right :)

Related Questions
HELP PLEASE ASAP!!!!!!HELP PLEASE ASAP!!!!!!HELP PLEASE ASAP!!!!!!HELP PLEASE ASAP!!!!!!HELP PLEASE ASAP!!!!!!HELP PLEASE ASAP!!!!!!HELP PLEASE ASAP!!!!!!HELP PLEASE ASAP!!!!!!HELP PLEASE ASAP!!!!!!HELP PLEASE ASAP!!!!!!HELP PLEASE ASAP!!!!!!HELP PLEASE ASAP!!!!!!HELP PLEASE ASAP!!!!!!HELP PLEASE ASAP!!!!!!HELP PLEASE ASAP!!!!!!HELP PLEASE ASAP!!!!!!HELP PLEASE ASAP!!!!!!HELP PLEASE ASAP!!!!!!HELP PLEASE ASAP!!!!!!HELP PLEASE ASAP!!!!!!

Answers
Answer:
Step-by-step explanation:

plssss answer due very soon

Answers
Answer:
Step-by-step explanation:

Answer:
2nd option
Step-by-step explanation:
\(4\frac{1}{3} *\frac{3}{5} =\frac{13}{3} *\frac{3}{5} =\frac{13}{5} =2\frac{3}{5}\)
When an alternating current of frequency f and peak current I_0 passes through a resistance R, then the power delivered to the resistance at time t seconds is P = I^2_0 R sin^2 2 pi ft. Write an expression for the power in terms of csc^2 2 pi ft. P = I^2_0 R/(csc^2 2 pi ft) P = I^2_0 R (csc^2 2 pi ft) P = I^2_0/(1 - csc^2 2 pi ft) P = I^2_0 R(1 - csc^2 2 pi ft)
Answers
The expression for the power delivered to a resistance in terms of csc^2 2 pi ft is P = I^2_0 R (csc^2 2 pi ft).
According to the given information, the power delivered to a resistance R when an alternating current of frequency f and peak current I_0 passes through it is represented by the equation P = I^2_0 R sin^2 2 pi ft.
To express this equation in terms of csc^2 2 pi ft, we can use the trigonometric identity csc^2 x = 1/sin^2 x. Substituting this identity into the equation, we get P = I^2_0 R (1/sin^2 2 pi ft).
Since csc^2 x is the reciprocal of sin^2 x, we can rewrite the equation as P = I^2_0 R (csc^2 2 pi ft). This expression represents the power delivered to the resistance in terms of csc^2 2 pi ft.
Therefore, the correct expression for the power delivered to the resistance in terms of csc^2 2 pi ft is P = I^2_0 R (csc^2 2 pi ft).
Learn more about resistance here:
https://brainly.com/question/33728800
#SPJ11
Zina solves a system of linear equations by elimination and finds that the solution to a system is (2, 2). One of the equations is a + b = 4. Which answer could be the other equation?
a + 3b = 4
2a + 2b = 2
4a + 4b = 8
a + 3b = 8
Answers
Answer: a + 3b = 8
Step-by-step explanation:
a + b = 4 is true, since (2,2) works: 2 + 2 = 4
Try (2,2) in the options to find which one is true for (2,2):
A) 2 + 3*2 = 4? No.
B) 4 + 4 = 2? No.
C) 8 + 8 = 8? No.
D) 2 + 6 = 8? YES.
Therefore, a + 3b = 8 can be another valid equation.
Answer:
D. a + 3b = 8
Kate is a buyer for a men’s fashion retail store. She will order a new cloth overcoat from Paris for the fall fashion season. Based on her experience, she expects to sell at least 100 coats, and at most 400, but she feels that any number of sales in between is equally likely. Therefore, she estimates that her sales are uniformly distributed between 100 and 400. The total cost to the store is $100 per coat, and the retail price is set at $180. Any coats left over at the end of season would be sold at $60 each.
part 1: a) How many coats should Kate buy if she wants to maximize profits?
part 2: b) Assume Kate buys the number of coats suggested in part a), what is the probability that the coats sell out? What is the probability that they do not sell out?
Answers
Part 1: Kate should buy 100 coats to maximize profits.Part 2: The probability that the coats sell out is 0.25 (25%), and the probability that they do not sell out is 0.75 (75%).
To maximize profits, Kate should consider the scenario where she sells all the coats without any left over at the end of the season.
Since the sales are uniformly distributed between 100 and 400, buying 100 coats ensures that she meets the minimum expected sales of 100. Purchasing more than 100 coats would increase costs without a guarantee of higher sales, potentially leading to excess inventory and lower profits.
Given that the sales are uniformly distributed between 100 and 400 coats, Kate's purchase of 100 coats covers the minimum expected sales.
The probability of selling out can be calculated by finding the proportion of the range covered by the desired sales (100 out of 300). Therefore, the probability of selling out is 100/300 = 0.25 or 25%. The probability of not selling out is the complement, which is 1 - 0.25 = 0.75 or 75%.
learn more about probability here:
https://brainly.com/question/31828911
#SPJ11
Solve the system by substitution.
3x-6=y
-6x+y=-9

Answers
Answer:
Step-by-step explanation:
y=3x-6
-6x+(3x-6)=-9
-3x-6=-9
-3x=-3
x=1,
Plug the x into other equations and it equals y
y=-3
(1,-3)
find three 2 by 2 matrices other than a = i that are their own inveses
Answers
These are three examples of 2x2 matrices (other than A = I and A = -I) that satisfy A² = I.
To find matrices that are their own inverses, we need to find matrices A such that A² = I, where I is the identity matrix.
Here are three examples of 2x2 matrices that satisfy A² = I:
A = [[1, 0], [0, -1]]
A² = [[1, 0], [0, -1]] * [[1, 0], [0, -1]] = [[11 + 00, 10 + 0(-1)], [01 + (-1)0, 00 + (-1)(-1)]]
= [[1, 0], [0, 1]]
Therefore, A is its own inverse.
A = [[0, 1], [1, 0]]
A² = [[0, 1], [1, 0]] * [[0, 1], [1, 0]] = [[00 + 11, 01 + 10], [10 + 01, 11 + 00]]
= [[1, 0], [0, 1]]
Therefore, A is its own inverse.
A = [[1, 1], [-1, 1]]
A² = [[1, 1], [-1, 1]] * [[1, 1], [-1, 1]] = [[11 + 1(-1), 11 + 11], [-11 + 1(-1), -11 + 11]]
= [[0, 2], [-2, 0]]
Therefore, A is its own inverse.
These are three examples of 2x2 matrices (other than A = I and A = -I) that satisfy A² = I.
The complete question is:
Find three 2 by 2 matrices, other than A = I and A = −I, that are their own inverses: A² = I.
To know more about matrices:
https://brainly.com/question/30646566
#SPJ4
Consider the function g(x)=−(x−1)^3−2. Which ordered pair lies on the inverse of the function?
(62,−3)
(−4, 123)
(3, 1)
(3,−6)
Answers
The ordered pair lie on the inverse of the function is (62,−3).
Option A is the correct answer.
What is a function?A function is a relationship between inputs where each input is related to exactly one output.
Example:
f(x) = 2x + 1
f(1) = 2 + 1 = 3
f(2) = 2 x 2 + 1 = 4 + 1 = 5
The outputs of the functions are 3 and 5
The inputs of the function are 1 and 2.
We have,
f(x) = -(x - 1)³ - 2
The inverse of f(x).
y = -(x - 1)³ - 2
interchange x and y and solve for y.
x = -(y - 1)3 - 2
(y - 1)³ = -2 - x
(y - 1)³ = -(2 + x)
Cuberoot on both sides.
y - 1 = ∛-(2 + x)
y = ∛-(2 + x) + 1
Now,
Substitute in the inverse of g(x).
(62, -3) = (x, y)
(−4, 123) = (x, y)
(3, 1) = (x, y)
(3,−6) = (x, y)
So,
y = ∛-(2 + x) + 1
y = ∛-(2 + 62) + 1
∛-1 = -1
y = -1∛64 + 1
y = -1 x 4 + 1
y = -4 + 1
y = -3
So,
(62, -3) ______(1)
And,
y = ∛-(2 + x) + 1
y = ∛-(2 - 4) + 1
∛-1 = -1
y = ∛(-2 + 4) + 1
y = ∛2 + 1
y = 1.26 + 1
y = 2.26
So,
(-4, 2.26) _______(2)
And,
y = ∛-(2 + x) + 1
y = ∛-(2 + 3) + 1
∛-1 = -1
y = -1∛5 + 1
y = -1 x 1.71 + 1
y = -1.71 + 1
y = -0.71
So,
(3, -0.71) _______(3)
And,
y = ∛-(2 + x) + 1
y = ∛-(2 + 3) + 1
∛-1 = -1
y = -1∛5 + 1
y = -1 x 1.71 + 1
y = -1.71 + 1
y = -0.71
So,
(3, -0.71) ______(4)
Thus,
From (1), (2), (3), (4) we see that,
(62, -3) is the solution to the inverse of g(x).
Learn more about functions here:
https://brainly.com/question/28533782
#SPJ1
Determine the circumference of each circle. Use 3.14 or 22 for .
Round to the nearest tenth if necessary.
Answers
Answer:
the circumference of the circle is about 23.55.
Step-by-step explanation:
hope this helps :D
Need answer now. Find m

Answers
180-119 = 61
Which line segment could be the diameter of this circle?

Answers
Answer:
K-A would make a circle because it goes across
HURRY!!!!
A line with a slope of 5 passes through the points (4,5) and (5,u) What is the value of u?
Answers
Answer:
See Below
Step-by-step explanation:
Slope is rise over run.
Rise is the difference in the y values
Run is the difference in the x values.
Rise/Run = 5 (slope)
You have both x values 4 from one point and 5 from the other point so the difference is 1. The Run is equal to 1
So you now have Rise / 1 = 5
The first y value you have is 5 and based on (rise/1 = 5), you know the difference in the y values must equal 5. That means the other y value has to be either 10 or 0.
0 will not work because that would make the slope negative since the line would slope downward from left to right.
help mee plss 5 mins

Answers
Answer:
20 ft
Step-by-step explanation:
It is 20 ft because you use pathagoren therom.
a^2 + b^2 = c^2
Answer:
The question cut off but I'm assuming this is basically just a find the area of the shaded region type of question. First thing you need to do is calculate the areas of the right triangle and the rectangle. Subtract the area of the triangle from the area of the rectangle.
Area of rectangle: 8 x 7 = 56ft^2
Area of the triangle: (6 x 2)/2 = 6ft^2
Subtract 6ft^2 from 56ft^2 and you get:
56 - 6 = 50ft^2
Area of shaded region is 50ft^2
Which of the following Boolean expressions is not equivalent to the expression num * -1 ≥ 10
A. (num < 0) AND (num * -1 = 10)
B. (num < -10) OR (num = -10)
C. (num * -1 > 10) OR (num = -10)
D. NOT num * -1 < 10
Answers
The Boolean expression that is not equivalent to the given expression "num * -1 ≥ 10" is option D: "NOT num * -1 < 10."
What is Boolean expression?
A Boolean expression is an expression or equation that evaluates to true or false. It includes the use of logical operators such as AND, OR, and NOT, as well as comparison operators such as equal to (=), greater than (>), less than (<), and more.
The Boolean expression that is not equivalent to the expression "num * -1 ≥ 10" is option D: "NOT num * -1 < 10."
Let's break down each option and evaluate its equivalence to the given expression:
A. (num < 0) AND (num * -1 = 10)
This expression checks if "num" is negative and if the absolute value of "num" is equal to 10. It does not directly represent the condition "num * -1 ≥ 10," so it is not equivalent.
B. (num < -10) OR (num = -10)
This expression checks if "num" is less than -10 or if "num" is exactly equal to -10. It also does not directly represent the condition "num * -1 ≥ 10," so it is not equivalent.
C. (num * -1 > 10) OR (num = -10)
This expression checks if the negative value of "num" is greater than 10 or if "num" is exactly equal to -10. Although it involves the negative value of "num," it represents the condition "num * -1 > 10" when "num" is positive. Therefore, it is equivalent to the given expression.
D. NOT num * -1 < 10
This expression checks if the negative value of "num" is not less than 10. While it involves the negative value of "num," it does not directly represent the condition "num * -1 ≥ 10." Additionally, the use of "NOT" operator flips the condition, which makes it different from the original expression. Hence, it is not equivalent.
Therefore, the Boolean expression that is not equivalent to the given expression "num * -1 ≥ 10" is option D: "NOT num * -1 < 10."
To learn more about Boolean Expressions from the given link
https://brainly.com/question/28330285
#SPJ4
What is the opposite of
V3?
HURRY!!!!!
Answers
Answer: V^3
V times V times V
Step-by-step explanation:
I think?
Find the horizontal shift 3 units to the right for the following equation y=3x+4
Answers
Therefore, the horizontal shift of 3 units to the right is achieved by subtracting 3 from the x-values in the equation.
To find the horizontal shift for the equation y = 3x + 4, we need to determine the change in the x-values or the shift in the x-direction.
In this case, the original equation is y = 3x + 4. To shift the graph 3 units to the right, we need to subtract 3 from the x-values.
The shifted equation will be y = 3(x - 3) + 4.
Therefore, the horizontal shift of 3 units to the right is achieved by subtracting 3 from the x-values in the equation.:
Learn more about horizontal shift here
https://brainly.com/question/30910246
#SPJ11
the time spent waiting in the line is approximately normally distributed. the mean waiting time is 7 minutes and the variance of the waiting time is 4 . find the probability that a person will wait for less than 11 minutes. round your answer to four decimal places.
Answers
To find the probability that a person will wait for less than 11 minutes, we need to standardize the value using the standard deviation and mean.
z = (x - μ) / σ
where x is the waiting time we want to find the probability for, μ is the mean waiting time, and σ is the standard deviation.
Plugging in the values, we get:
z = (11 - 7) / 2
z = 2
Using a standard normal distribution table or calculator, we can find the probability that a z-score is less than 2. This probability is approximately 0.9772.
Therefore, the probability that a person will wait for less than 11 minutes is 0.9772 (rounded to four decimal places).
math question on photo please

Answers
\(2ay + 3az = 2dx + 3dy\)
\(2ay - 3dy = 2dx - 3az\)
\(y(2a - 3d) = 2dx - 3az\)
\(y = \frac{2dx - 3az}{2a - 3d} \\ \)
Need help asap. Thanks!

Answers
Answer:
A- SAS
Step-by-step explanation:
The statements tell you the outer two lines are congruent and the top two angles as well, then through the properties of equality you know the inner line is congruent to itself. That means you have two sides and an included angle making it SAS.
if I hit myself and it hurts am I weak or am I strong
A weak
B strong
C dumb
D mentally weak
Answers
Answer:
b
Step-by-step explanation:
Answer:
C and A
Step-by-step explanation:
Janet attends state university and lives in an on campus dorm suite with 5 friends. They share cost of the monthly upgraded cable bill for their suite. Below is a listing of the bills for their freshman year. 9. Round the following value
Σ
619
X₁
to the nearest dollar.
Interpret the answer in the context of the problem.
Answers
Answer:
Step-by-step explanation: Step 1
1 of 2
step 1.2659 ;[26 Discovery Group
October 20
Last
Trade Time
Chg
Open
52-week High
52-week Low
Sales in 100s
High
Low
$38.50
4:00 P.M. ET
$1.56
$37.22
$76.19
$22.78
19,700
$40.10
$36.77
\begin{matrix} \text{Discovery Group} & \text{}\\ \text{October 20} & \text{}\\ \text{Last} & \text{\$42.00}\\ \text{Trade Time} & \text{4:00 P.M. ET}\\ \text{Chg} & \text{\$1.50}\\ \text{Open} & \text{\$42.50}\\ \text{52-week High} & \text{\$76.19}\\ \text{52-week Low} & \text{\$22.78}\\ \text{Sales in 100s} & \text{23,600}\\ \text{High} & \text{\$42.50}\\ \text{Low} & \text{\$42.00}\\ \end{matrix}
Discovery Group
October 20
Last
Trade Time
Chg
Open
52-week High
52-week Low
Sales in 100s
High
Low
$42.00
4:00 P.M. ET
$1.50
$42.50
$76.19
$22.78
23,600
$42.50
$42.00
Given the equation y= -3x+2.5, when y is 8.5, what value of x makes the equation true?
PLEASE EXPLAIN HOW YOU GOT YOUR ANSWER AS WELL !! ( im not very smart :c )
Answers
Answer:
x= -2
Step-by-step explanation:
First, write your equation with 8.5 substituted for y: 8.5= -3x +2.5
Next, subtract 2.5 from both sides to simplify the equation: 6= -3x
After that, divide both sides by -3 to get x by itself: -2 = x
So, when y=8.5, x=-2.
Abraham has visited 10 states already. He has a goal of visiting all 50 states. He plans to achieve this goal by visiting 5 new states per year (y) for the next several years. Which equation and solution shows the number of years that it will take for Abraham to meet his goal?
Answers
Answer:
10 + 5y = 50, y = 8 (years)
Step-by-step explanation:
5y - states. that Abraham still plans to visit.
10 + 5y = 50
5y = 50 - 10
5y = 40
y = 8
Answer: 10+5y=50; y=8 years
Step-by-step explanation:
Since Abraham has already visited 10 states, and he wants to visit 5 states per year, we get the equation 10+5y=50. The 10 is the number is states he has already visited. 5y represents the rate at which he visits the remaining states. 50 is the total amount of states.
To find the number of years, you get y alone.
10+5y=50
5y=40
y=8 years
under the surface z = 1+ x2y2 and above the region enclosed by x = y^2 and x = 4.
Answers
The volume under the surface z = 1 + x² y² and above the region enclosed by x = y² and x = 4 is (19π - 12)/6. This can be calculated by setting up and evaluating a triple integral using cylindrical coordinates.
The question asks for the region above x = y² and below x = 4, which can be visualized as a parabolic cylinder. The surface z = 1 + x²y² can be plotted on top of this region to give a solid shape. To find the volume of this shape, we need to integrate the function over the region. We can set up the integral using cylindrical coordinates as follows:
V = ∫∫∫ z r dz dr dθ
where the limits of integration are:
0 ≤ r ≤ 2
0 ≤ θ ≤ π/2
y^2 ≤ x ≤ 4
Plugging in the equation for z and simplifying, we get:
V = ∫∫∫ (1 + r² cos² θsin² θ) r dz dr dθ
Evaluating the integral gives:
V = (19π - 12)/6
The volume under the surface z = 1 + x² y² and above the region enclosed by x = y² and x = 4 can be found by integrating the function over the given region using cylindrical coordinates. The limits of integration are 0 ≤ r ≤ 2, 0 ≤ θ ≤ π/2, and y² ≤ x ≤ 4. Plugging in the equation for z and evaluating the integral gives (19π - 12)/6 as the final answer.
The volume under the surface z = 1 + x² y² and above the region enclosed by x = y² and x = 4 is (19π - 12)/6. This can be calculated by setting up and evaluating a triple integral using cylindrical coordinates.
To know more about integration visit:
brainly.com/question/31744185
#SPJ11
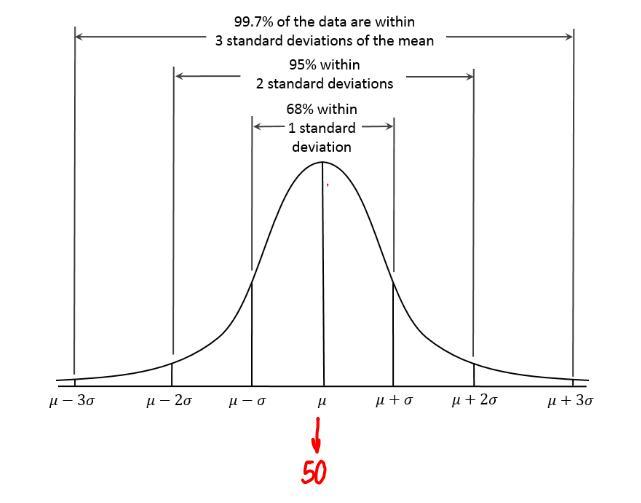
A standardized test is designed so that scores have a mean of 50 and a standard deviation of 4. What percent of scores are between 42 and 58?A.100%B. about 95.4%C. about 23.85%D. about 47.7%
Answers
Step 1. The information that we have is:
The mean:
\(\mu=50\)The standard deviation:
\(\sigma=4\)Step 2. To solve this problem and find what percent of scores are between 42 and 58, we use the empirical rule:
• The empirical rule for normally distributed data tells us that about 68% of the data falls under 1 standard deviation from the mean, about 95% falls under 2 standard deviations from the mean, and 99.7% of the data falls under 3 standard deviations from the mean.
Step 3. The following diagram represents the situation:
The marks on the graph are calculated as follows:
\(\begin{gathered} \mu-\sigma=50-4=46 \\ \mu+\sigma=50+4=54 \\ \mu+2\sigma=50-2\cdot4=50-8=58 \\ \mu-2\sigma=50-2\times4=50-8=42 \end{gathered}\)This is represented in the image:
Step 4. As you can see in the previous graph, 42 and 58 are 2 standard deviations away from the mean, this means that about 95% of the data will be between those values.
The option closest to 95% is B. about 95.4%
Answer: B. about 95.4%

a quadratic equation in standard form is written ax2 = bx c, where a, b, and c are real numbers and a is not zero. True or False
Answers
The given statement is correct.
Hence it is true.
We have a statement regarding the quadratic equations.
We have to verify whether it is true or not.
Since we know that,
A quadratic equation is an equation with a single variable of degree 2. Its general form is ax² + bx + c = 0, where x is variable and a, b, and c are constants, and a ≠ 0.
According to the question, we are provided with the standard form of the quadratic equation as - ax² + bx + c = 0.
If we compare the statement given in the question with the definition discussed above, then it can be concluded that the given statement is true. Equation ax² + bx + c = 0 is the standard form of a quadratic equation with a, b, and c as constant real numbers.
The constant 'a' cannot be 0, as this would reduce the degree of the equation to 1.
Hence, the given statement is correct.
To learn more about quadratic equations, visit:
brainly.com/question/26090334
#SPJ12
2. Determine the points of intersection of each pair of functions. a) y = 4x^– 15x + 20 and y = 5x – 4 = - - b) y = - 2x^ + 9x +9 and y = - 3x – 5

Answers
To determine the points of intersection we first equate the expressions, then we solve for x. Once we have the values of x for which the functions are equal we plu them on one of the function to find its corresponding value of y.
a)
Let's equate the functions and solve for x:
\(\begin{gathered} 4x^2-15x+20=5x-4 \\ 4x^2-15x-5x+20+4=0 \\ 4x^2-20x+24=0 \\ 4(x^2-5x+6)=0 \\ x^2-5x+6=0 \\ (x-3)(x-2)=0 \\ \text{ then} \\ x=3 \\ or \\ x=2 \end{gathered}\)Now we find the corresponding values of y for each value of x; to do this we use the second equation.
When x=3:
\(\begin{gathered} y=5(3)-4 \\ y=15-4 \\ y=11 \end{gathered}\)Hence the functions intersect at (3,11)
When x=2:
\(\begin{gathered} y=5(2)-4 \\ y=10-4 \\ y=6 \end{gathered}\)Hence the functions intersect at (2,6)
Therefore the function intersect at the points (3,11) and (2,6).
b)
Let's equate the functions and solve for x:
\(\begin{gathered} -2x^2+9x+9=-3x-5 \\ 2x^2-9x-9-3x-5=0 \\ 2x^2-12x-14=0 \\ 2(x^2-6x-7)=0 \\ x^2-6x-7=0 \\ (x-7)(x+1)=0 \\ \text{ then} \\ x=7 \\ or \\ x=-1 \end{gathered}\)Now we find the corresponding values of y for each value of x; to do this we use the second equation.
When x=7:
\(\begin{gathered} y=-3(7)-5 \\ y=-21-5 \\ y=-26 \end{gathered}\)Hence the functions intersect at (7,-26)
When x=-1:
\(\begin{gathered} y=-3(-1)-5 \\ y=3-5 \\ y=-2 \end{gathered}\)Hence the functions intersect at (-1,-2)
Therefore the function intersect at the points (7,-26) and (-1,-2).
Consider the following scenario to understand the relationship between marginal and average values. Suppose Lorenzo is a professional b. player, and his game log for free throws can be summarized in the following table.
Answers
The missing points from the Column is:
Game Free-Throw Percentage: 60 20 60 80
Average Free-Throw Percentage: 70 60 55 56.67
Game Game Total Game Average
Result Free-Throw Free-Throw
Percentage Percentage
1 8/10 8/10 80 80
2 6/10 14/20 60 70
3 1/5 15/25 20 60
4 3/5 18/30 60 55
5 8/10 26/40 80 56.67
In the "Total" column, we keep track of the cumulative number of successful free throws out of the total attempts.In the "Game Free-Throw Percentage" column, we calculate the percentage of successful free throws made in each game.In the "Average Free-Throw Percentage" column, we calculate the average free-throw percentage up to that game by dividing the cumulative successful free throws by the cumulative total attempts and multiplying by 100.Learn more about Cumulative Frequency here:
https://brainly.com/question/28491523
#SPJ4
The question attached here seems to be incomplete, the complete question is
Fill in the columns with Dmitri's free-throw percentage for each game and his overall free-throw average after each game.
Game Game Result Total Game Free-Throw Percentage Average Free-Throw Percentage
1 8/10 8/10 80 80
2 6/10 14/20
3 1/5 15/25
4 3/5 18/30
5 8/10 26/40
the volume of a tree stump can be modeled by considering it as a right cylinder. evelyn measures its circumference as 197 in and its volume as 92650 cubic inches. find the height of the stump in feet. round your answer to the nearest tenth if necessary.
Answers
The height of the tree stump is 2.48 feet.
To find the height of the tree stump, we can use the formula for the volume of a right cylinder: \(V = \pi r^2h\), where V represents the volume, r represents the radius, and h represents the height.
Given that the circumference of the tree stump is measured as 197 inches, we can use the formula for the circumference of a circle: C = 2πr, where C represents the circumference.
From the given circumference, we can solve for the radius, r, by dividing the circumference by 2π: r = C / (2π).
Plugging in the given circumference of 197 inches, we have: r = 197 / (2π) ≈ 31.416 inches.
Now, we can substitute the known values of the volume and radius into the volume formula: 92650 = π(31.416)^2h.
To solve for the height, h, we divide both sides of the equation by π(31.416)^2: h = 92650 / (π(31.416)^2) ≈ 29.74 inches.
Since we want to convert the height from inches to feet, we divide the height by 12: h = 29.74 / 12 ≈ 2.48 feet.
Therefore, the height of the tree stump is approximately 2.48 feet.
For more question on height visit:
https://brainly.com/question/28990670
#SPJ8
Multiply. (5√3 -2)²
Answers
Using multiplication to solve the square roots, (5√3 -2)², we get the answer as 79 - 20√3
How do we multiply square roots?To multiply a square root, multiple its radicands first—that is, the values that appear after the radical sign. If the radical sign is preceded by any coefficients, multiply those coefficients as well.
Using (a-b)² = a² + b² - 2ab
We have,
(5√3 -2)²
On expanding,
we have , (5√3)² + 2² - 2(5√3)(2)
⇒ 75 + 4 - 20√3
⇒ 79 - 20√3
To learn more about square root multiplication from given link
https://brainly.com/question/14245229
#SPJ4
