"
Suppose X is normally distributed with a mean of u = 12 and a standard deviation of g = 1.4. Find the z-score corresponding to x = 15.5. Show your work.
"
Answers
The z-score corresponding to x = 15.5 is 2.5.
To find the z-score corresponding to x = 15.5, we can use the formula:
Z = (X - \(\mu\)) / g
where Z is the z-score, X is the given value, \(\mu\) is the mean, and g is the standard deviation.
In this case:
Z = (15.5 - 12) / 1.4
= 3.5 / 1.4
= 2.5
Therefore, the z-score corresponding to x = 15.5 is 2.5.
Work:
Z = (15.5 - 12) / 1.4 = 3.5 / 1.4 = 2.5
To know more about z-score, refer here:
https://brainly.com/question/31871890
#SPJ4
Related Questions
Evaluate 10 + 2(3 + 2) + 5 + (36÷ 6). 71 31 41 21
Answers
Answer:
31
Step-by-step explanation:
What is the mean of the data set

Answers
Answer:
12.5 if all the numbers are one set. 15.3 for first row and 10 for last row if they are separate
Step-by-step explanation:
mean is found by adding all the data from the set and then dividing by how many data's there are. Mean can also be called the average of a set of data.
Find DE.
3x - 28
3x - 30
X
D
6
F
33

Answers
Solve the inequality 12(1/2 x - 1/3) > 8 - 2x
O A. x>-1
O B. x > 3
O C. X>1/2
O D. x>3/2
Answers
Answer:
\(D. \\x>\frac{3}{2}\)
Step-by-step explanation:
12(1/2x - 1/3) > 8 - 2x
6x - 4 > 8 - 2x
6x + 2x - 4 > 8 - 2x + 2x
8x - 4 > 8
8x - 4 + 4 > 8 + 4
8x > 12
8x ÷ 8 > 12 ÷ 8
x > 1.5
If the mean of x and y is 32 and the mean of y and z is 50, then what is the value of x - z?
Answers
Answer:
x - z = -36
Step-by-step explanation:
Given:
x + y y + z
---------- = 32 and ---------- = 50
2 2
These lead to x + y = 64 and y + z = 100
Subtracting the second equation from the first yields:
x + y = 64
-( y + z = 100)
------------------------
x - z = -36
Heston is thinking of a fraction equivalent to 6/7.
The numerator is greater than 20 and the denominator is less than 30.
What fraction is Heston thinking of?
Answers
Explanation:
Multiply the numerator and denominator by 4 to give you 24/28, which is equivalent to 6/7.
Given the function h of x equals negative 2 times the square root of x, which statement is true about h(x)?
Answers
The correct statement about the function h(x) = -2 square root of x is given as follows:
The function is decreasing on the interval (0, ∞).
How to define the function's behavior?The parent function in this problem is given as follows:
f(x) = square root of x.
The domain of this function is for non-negative values, that is, x ≥ 0, and the function is increasing over it's entire interval.
The transformed function is given as follows:
h(x) = -2 square root of x
The product by the negative number means that the function was reflected over the x-axis, meaning that when it was increasing, now it is decreasing.
The domain of the function remains the same, hence the first statement is the correct statement.
Missing InformationThe statements are given as follows:
The function is decreasing on the interval (0, ∞).The function is decreasing on the interval (–∞, 0).The function is increasing on the interval (0, ∞).The function is increasing on the interval (–∞, 0).More can be learned about functions at https://brainly.com/question/24808124
#SPJ1
ANSWER QUICK I WILL MAKE U BRAINLIEST

Answers
The answers are 1.67 glucose molecules, 36 glucose molecules, 15 glucose molecules and 8400 times.
What is proportionality?When two ratios are equivalent, they are in proportion. It is an equation or statement used to depict that two ratios or fractions are equal.
Given are some questions regarding production of glucose molecules during aerobic metabolism.
1) 1 glucose molecules produces 30 ATP molecules, how many for 50 ATP molecules :-
Using proportionality, let there be x glucose molecules required to produce 50 ATP molecules,
1 / 30 = x / 50
x = 5/3
x = 1.67
Therefore, there are 1.67 glucose molecules required to produce 50 ATP molecules.
2) 1 glucose molecules produces 6 ATP molecules, how many for 216 ATP molecules :-
Using proportionality, let there be x glucose molecules required to produce 216 ATP molecules,
1 / 6 = x / 216
x = 36
Therefore, there are 36 glucose molecules required to produce 216 ATP molecules.
3) 1 glucose molecules produces 30 ATP molecules, how many for 450 ATP molecules :-
Using proportionality, let there be x glucose molecules required to produce 450 ATP molecules,
1 / 30 = x / 450
x = 15
Therefore, there are 15 glucose molecules required to produce 450 ATP molecules.
4) Since, in one day it's 1200 times an ATP molecule is reused in a human body,
We need to find for a week, there are 7 days in a week,
Therefore, an ATP molecule is reused in a human body for a week = 1200 x 7 = 8400 times.
Hence, the answers are 1.67 glucose molecules, 36 glucose molecules, 15 glucose molecules and 8400 times.
Learn more about proportionality, click;
https://brainly.com/question/29126727
#SPJ1
g(x)=3x+2 and h(x)=ax+B
where a and b are constants
h(4)=22
g^-1(14)=h(1)
find the value of a and the value of b

Answers
Answer:
a=6
b=-2
Step-by-step explanation:
You can use the hint provided on the mathswatch question page, I've attached it below
step 1
h(4) = ax+b = (ax4)+b = 4a+b
it is given in the question that h(4)=22, therefore 4a+b=22
step 2
work out the equation for inverse g
g^-1(x) = \(\frac{x-2}{3}\)
step 3
input 14 into the equation in place of x to find g^-1(x)
\(\frac{x-2}{3} =\frac{14-2}{3} =\frac{12}{3} =4\)
so, g(x)^-1=4
step 4
find h(1):
h(1) = (ax1)+b = a+b
so, h(1)=a+b
step 5
a+b=4 (as it is given in the question that g^-1(14) is equal to h(1))
step 6
Now you have two simultaneous equations:
4a+b=22
a+b=4
then, solve for a:
4a+b=22
- a+b=4
3a=18
a=6
then, input answer for a into either equation (I've gone with the a+b=4 equation):
6+b=4
-6 - 6
b=-2
(p.s i know this is very late but hope it helps someone)

Question 3
10 points
Save Answer
If the monthly marginal cost function for a product is MC = C'(x) = 4x + 20 and the cost of producing 2 units is $78, find the Total Cost Function [C(x)] for the product.
a. ·C(x) = 2x2 + 20x + 30
b. C(x) = 2x² + 20x + 78
c. C(x) = 2x2 + 20
d. C(x) = x² 2 + 20x + 50
Answers
To find the Total Cost Function C(x) for a product, we need to integrate the given marginal cost function MC(x). Given that MC(x) = 4x + 20 and the cost of producing 2 units is $78, we can determine the Total Cost Function.
The Total Cost Function C(x) represents the cumulative cost of producing x units. To find C(x), we need to integrate the marginal cost function MC(x) with respect to x.
Integrating MC(x), we get:
C(x) = ∫(MC(x))dx = ∫(4x + 20)dx.
Integrating each term separately, we obtain:
C(x) = 2x² + 20x + C,
where C is the constant of integration.
To find the value of C, we use the given information that the cost of producing 2 units is $78. Substituting x = 2 and C(x) = 78 into the equation, we have:
78 = 2(2)² + 20(2) + C,
78 = 8 + 40 + C,
78 = 48 + C,
C = 78 - 48,
C = 30.
Therefore, the Total Cost Function for the product is:
C(x) = 2x² + 20x + 30.
Hence, the correct answer is option a. C(x) = 2x² + 20x + 30.
Learn more about Total Cost Function here:
https://brainly.com/question/29262808
#SPJ11
ASAP!! Please help me. I will not accept nonsense answers, but will mark as BRAINLIEST if you answer is correctly with solutions.

Answers
Answer: B. H(t) = -6.9(t - 2.3)² + 112
Step-by-step explanation:
The vertex form of a quadratic equation is: y = a(x - h)² + k
where (h, k) is the vertex ⇒ k is the maximum height
the distance traveled is when y = 0 ⇒ \(x=\sqrt{\dfrac{-k}{a}}+h\)
Given: H(t) = -7.1(t - 2.3)² + 98
maximum height (k) = 98 feet
distance traveled (x) = \(\sqrt{\dfrac{-98}{-7.1}}+2.3\) = 6.02 seconds
A) H(t) = -7.5(t - 2.2)² + 112
maximum height (k) = 112 feet
distance traveled (x) = \(\sqrt{\dfrac{-112}{-7.5}}+2.2\) = 6.06 seconds
B) H(t) = -6.9(t - 2.3)² + 112
maximum height (k) = 112 feet
distance traveled (x) = \(\sqrt{\dfrac{-112}{-6.9}}+2.3\) = 6.33 seconds
C) H(t) = -6.9(t - 2.4)² + 95
maximum height (k) = 95 feet
This has a lower height than the given equation.
D) H(t) = -7.5(t - 2.3)² + 95
maximum height (k) = 95 feet
This has a lower height than the given equation.
Both options A and B travel higher and stay in the air longer than last year's winner, however option B stays in the air longer than option A.
1 × 2 = 2
2 × 2 = 4
The ratios show the same relationship between chili peppers and tomatoes.
Which of these ratios is equivalent to 2:3?
Answers
Answer:
what are the ratios hope this helps
1. (1 point) Let x be a real number. Show that a (1 + x)2n > 1+ 2nx for every positive integer n.
Answers
For a real number x, by using mathematical induction it is shown that a\((1 + x)^{2n}\) > 1 + 2nx for every positive integer n.
To prove the inequality a\((1 + x)^{2n}\) > 1 + 2nx for every positive integer n, we will use mathematical induction.
The inequality holds true for n = 1, and we will assume it is true for some positive integer k.
We will then show that it holds for k + 1, which will complete the proof.
For n = 1, the inequality becomes a\((1 + x)^2\) > 1 + 2x.
This can be expanded as a(1 + 2x + \(x^2\)) > 1 + 2x, which simplifies to a + 2ax + a\(x^2\) > 1 + 2x.
Now, let's assume the inequality holds true for some positive integer k, i.e., a\((1 + x)^{2k}\) > 1 + 2kx.
We need to prove that it holds for k + 1, i.e., a\((1 + x)^{2(k+1)}\) > 1 + 2(k+1)x.
Using the assumption, we have a\((1 + x)^{2k}\) > 1 + 2kx.
Multiplying both sides by \((1 + x)^2\), we get a\((1 + x)^{2k+2}\) > (1 + 2kx)\((1 + x)^2\).
Expanding the right side, we have a\((1 + x)^{2k+2}\) > 1 + 2kx + 2x + 2k\(x^2\) + 2\(x^2\).
Simplifying further, we get a\((1 + x)^{2k+2}\) > 1 + 2(k+1)x + 2k\(x^2\) + 2\(x^2\).
Since k and x are positive, 2k\(x^2\) and 2\(x^2\) are positive as well.
Therefore, we can write a\((1 + x)^{2k+2}\) > 1 + 2(k+1)x + 2k\(x^2\) + 2\(x^2\) > 1 + 2(k+1)x.
This proves that if the inequality holds for some positive integer k, it also holds for k + 1.
Since it holds for n = 1, it holds for all positive integers n by mathematical induction.
Therefore, we have shown that a\((1 + x)^{2n}\) > 1 + 2nx for every positive integer n.
Learn more about mathematical induction here:
https://brainly.com/question/29503103
#SPJ11
The measure of angle 1 is (10x+8)° and the measure of angle 3 is (12x- 10)°.
What is the measure of angle 2 in degrees?
9
98
82
16

Answers
Step-by-step explanation:
option b ie. 98 degree
if you do angle 2 +10x+8=180
from 10x +8 and 12x -10 we can get the value of X
1.
(04.02 LC)
Which of the following is a common characteristic of a binomial distribution? (4 points)
There are more than two possible outcomes.
The probability of success is the same in all trials.
There are infinitely many observations.
You should perform x trials until you observe a success.
Each trial is dependent on the previous trial.
Answer: The probability of success is the same in all trials.
Answers
The probability of success, p, is the same for each trial. Each outcome is either a success (P) or a failure (Q). The correct option is A.
What is the independent probability?Independence is a fundamental notion in probability theory, as in statistics and the theory of stochastic processes.
You should perform x trials until you observe a success.
We have given that,
There are exactly two possible outcomes success and failure.
We have to determine the following is NOT a common characteristic of a binomial distribution.
By process of elimination:-
A binomial experiment is a statistical experiment that must meet certain requirements
All trials are independent.
There are a fixed number of n trials.
The probability of success, p, is the same for each trial.
To learn more about the probability visit:
brainly.com/question/24756209
#SPJ1
The toll to a bridge is 2.50. A three-month pass costs $12.00 and reduces the toll to $0.50. A six month pass $37 and permits crossing the bridge for no additional fee. How many crossing per three month period does it take for the three month pass to be the best deal.
Answers
c=number of crossings; Q=quarterly pass; S=semi-annual pass; P=pay as you go
P=$2.50c
Q=$12+$0.50c
S=$40
Compare single pay and quarterly ticket:
$2.50c=$12+$0.50c Subtract $0.50c from each side
$2.00c=$12 Divide each side by $2.
c=6 6 crossings is the break even for single pay and quarterly ticket: more crossings favor the ticket.
Compare quarterly and semi-annual tickets:
3 month pro-rated cost of semi annual ticket=$20
$20=$12+$0.50c Subtract 12 from each side
$8=$0.50c Divide each side by $0.50
16=c The break=even point between quarterly and semi annual tickets is 16 crossings per 3 months. More than this favors the semi annual ticket.
ANSWER: The three month pass is the best deal if you cross between 6 and 16 times during the three month period.
I hope this help you
If the measure of arc MOP = 11x-38 and the measure of angle
LMP = 3x+41, find the measure of angle NMP.
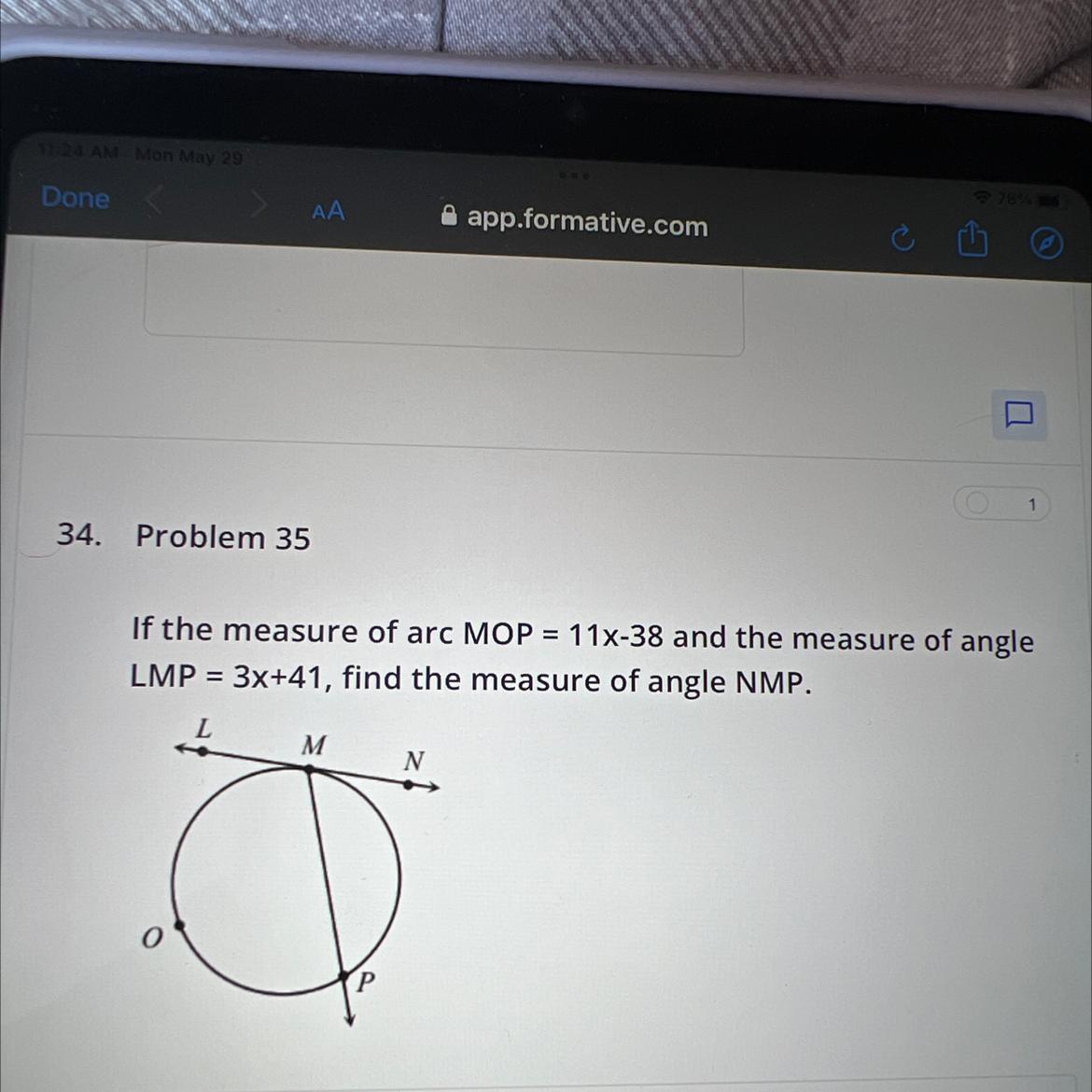
Answers
Answer:
mop=11x-38
Lmp=3x+41
we kow that,
area of circle=2pier²If a+b=2 find the value of a3+b3+6ab
Answers
Answer:
Step-by-step explanation:
Factor the sum of cubes
a³ + b³ = (a+b)(a² - ab +b²)
= 2a² - 2ab + 2b²
a³ + b³ + 6ab = 2a² + 4ab + 2b²
= 2(a² + 2ab +b²)
= 2(a+b)²
= 8
At the neighborhood grocery, 5 pounds of salmon cost $49. How much would it cost
to buy 4.7 pounds of salmon?
Answers
Answer:
$46.06
Step-by-step explanation:
Divide $49 by 5
9.8
So it costs $9.80 for 1 pound of salmon
Multiply $9.80 by 4.7
$46.06
Hope this helped :)
The annual property taxes on Jean's new home are 4. 23% of the value of the home. Her home cost \$238,500. What are the property taxes on her new home?
Answers
Jean would need to pay $10088.55 as property tax on her new home which cost $238500
What is an equation?An equation is an expression that shows the relationship between two or more numbers and variables.
Let x represent the value of Jeans property tax.
Jean's new home are 4. 23% of the value of the home. For a home of $238500:
x = 4.23% of $238500 = 0.0423 * $238500 = $10088.55
Jean would need to pay $10088.55 as property tax on her new home which cost $238500
Find out more on equation at: https://brainly.com/question/2972832
this graph shows a proportional relationship. what’s the constant of proportionality ?

Answers
Answer: 3
Step-by-step explanation:
7 x 3 = 21
Answer:
40
Step-by-step explanation:
i guessed for you, and i got it right.
Hahshxnxjennshsne did. D d d s s d d d
Answers
Answer:
are you okay?
Step-by-step explanation:
Drone sales for a New York based firm over the last 4 weeks are depicted in the table below Week Unit Sales 700 724 720 3 728 4 What is the equation of the trend line and the predicted sales for week 6? 0 718 + 10t and 798 730 + 8t and 778 O 734+10t and 774 698+8t and 746
Answers
The predicted sales for week 6 is 740
In the case of linear regression using excel formula, we can find the intercept and the slope value for the regression model.
The equation of linear regression is similar to the slope formula what we have learned before in earlier classes such as linear equations in two variables.
It is given by; Y= a + bXForecast\($=\mathrm{A}+\mathrm{B}(\mathrm{x})$\)
Where A is the intercept of the data, B is the slope, and X is the period.
Intercept \(=\) INTERCEPT (Range Y, Range X)
Slope \(=\) Slope (Range Y, Range X)The above data generates the equation.
Now the data corresponds to an intercept value of 698 and a slope of 8.
Therefore, for period \($6, x=6$\)
The equation \($=698+(8 * 6)=746$\)
The intermediate calculations are in the table below:
PERIODUNITSFORECAST
____________________
1 700 706
2 724 714
3 720 722
4 728 730
5 738
6 740
Therefore, the predicted sales for week 6 is 740.
For more such questions on Linear regression:
https://brainly.com/question/29665935
#SPJ4
Find the area of the kite with measurements of 6cm 1cm 11cm
Answers
The area of the kite is \(66 \ cm^2\).
To find the area of a kite, you can use the formula: Area = \(\frac{(diagonal \ 1 \times diagonal \ 2)}{2}\)
In this case, the measurements given are \(6\) cm, \(1\) cm, and \(11\) cm. However, it is unclear which measurements correspond to the diagonals of the kite.
If we assume that the 6 cm and 11 cm measurements are the diagonals, we can calculate the area as follows:
Area = \(\frac{6 \times 11 }{2}\)
= \(66\) cm²
If the \(1\) cm measurement is one of the diagonals, and the other diagonal is unknown, it is not possible to calculate the area accurately without the measurement of the other diagonal. Without knowledge of the lengths of both diagonals of the kite, it is not possible to determine the exact area as it depends on the specific dimensions.
Therefore, the area of the kite is \(66 \ cm^2\).
For more such questions on area:
https://brainly.com/question/26403859
#SPJ8
Write and graph the inverse variation in which y = 4 when x = 2.
Answers
An inverse variation is the one in which the product of x and y is a constant:
\(xy=k\)We can find the value of this constant by replace x and y for the given values:
\(\begin{gathered} (2)(4)=k \\ k=8 \end{gathered}\)Now, replace k in the initial equation and then solve for y:
\(\begin{gathered} xy=8 \\ y=\frac{8}{x} \end{gathered}\)It means that the relationship is:
\(y=\frac{8}{x}\)The graph of this relationship is:

Can someone help me with this? Please and thank you

Answers
Given
The slope of the line is 3.
And, the coodinates of x and y on the graph is (-1,1).
To find the equation of the line using slope point form.
Explanation:
The equation of the line using slope point form is given as,
\(y-y_1=m(x-x_1)\)Then, for m=3 and point (-1,1).
We get,
\(\begin{gathered} y-1=3(x-(-1)) \\ y-1=3(x+1) \\ y-1=3x+3 \\ y=3x+3+1 \\ y=3x+4 \end{gathered}\)Hence, the equation of the line is y=3x+4.
Charlie subtracts two polynomials, 5x^2 – 9x^2, and says that the difference proves that polynomials are not closed under subtraction.Kate argues that the difference does in fact show that polynomials are closed under subtraction. Who is correct? Type only 'Charlie' orKate' as your answer in the box.
Answers
Charlie
Here, we want to check if polynomials is closed under subtraction or not
To check this, we proceed either ways, if the answer is same, then it is closed. If otherwise, then it is not
We have;
\(\begin{gathered} 5x^2-9x^2=-4x^2 \\ \\ \text{And;} \\ \\ 9x^2-5x^2=4x^2 \end{gathered}\)As we can see, both results are not equal
If subtraction was closed under polynomials, then the results of both should have been equal. This means Charlie is correct.
x^2 +4x+4=0 resolver en forma cuadratica
Answers
Answer:
Solve the equation for x by finding a , b , and c
of the quadratic then applying the quadratic formula.
x = − 2 Double roots
Solve the inequality. 5+5(-7x+ 1) ≥ -130 write answer in the format variable inequality number
Answers
Answer:
x<_ 4
Step-by-step explanation:
first you distribute 5 to equal 5-35x+5 then you would carry the non variables to one side to be -35x>_-140 and the you would flip the sides because te variable is negative so it would be -35x<_ -140 the divid to get x<_4
Distribute -5
5+-35x+5>-130
Combine like terms
10+-35x>-130
Subtract 10 from both sides
-35x>-140
Divide by -35 on each side
(Flip the sign since you divide by a negative)
-35x/35 > -140/35
ANSWER x is less than or equal to 4
How do you regroup in 2nd grade math?
Answers
Answer:
To regroup means to rearrange groups in place value to carry out an operation.