An airplane ascends from the ground at an angle
of 17°. When the airplane has traveled a
horizontal distance of 650 ft, what is its height
above the ground to the nearest foot?
Answers
Related Questions
Solve for the unknown number in the equation ________ − 17 = 38 − 25.
Answers
Answer:
I believe the answer is 30.
Step-by-step explanation:
Answer:
___30_____ − 17 = 38 − 25
Step-by-step explanation:
________ − 17 = 38 − 25
Do the subtraction on the right side.
________ − 17 = 13
Add 17 to both sides.
________ = 30
Answer:
___30_____ − 17 = 38 − 25
Brigid is picking strawberries at the Pick-Your-Own Farm. Her goal is to pick 5 bushels of strawberries. She has already picked 1
1
2
bushels, and she picks at a rate of
5
8
bushel per hour. The scenario is represented as
5
8
h + 1
1
2
= 5, where h is the number of hours she picks. How many more hours will it take Brigid to fill 5 bushels of strawberries?
2 and StartFraction 3 Over 16 EndFraction hours
2 and StartFraction 3 Over 16 EndFraction hours
5 and three-fifths hours
10 and two-fifths hours
Answers
Answer:
We can start by isolating the variable "h".
5 8 h + 1 1 2 = 5
Subtracting 11/2 from both sides:
5 8 h = 5 - 1 1 2
Simplifying:
5 8 h = 8 1 2
Dividing both sides by 5/8:
h = 8 1 2 ÷ 5 8
Converting the mixed number to an improper fraction:
h = (8 x 8 + 1) ÷ 5 8
h = 65/8
Now, we can convert this fraction to a mixed number:
h = 8 1/8
Brigid has already picked for 8 1/8 hours, so the amount of time needed to pick the remaining strawberries is:
5 - (1 1/2 + 5/8 x 8) = 5 - (3 5/8) = 1 3/8
Therefore, Brigid still needs to pick for 1 3/8 hours to fill 5 bushels of strawberries. The answer is 1 and 3/8 hours or 2 and 3/16 hours (if simplified).
Step-by-step explanation:
2 years ago, father's age was nine times the son's age but 3 years letter it will be 5 times only. Find the present ages of the father and the son
Answers
The required present ages of the father and the son are 47 years and 7 years respectively.
Let the present age of the father and the son be x years and y years, respectively.
According to the question,
Condition I,
2 years ago, the father's age was nine times the son's age.
or,(x−2)=9(y−2)
or,x−2=9y−18
or,x=9y−18+2
or,x=9y−16 - (i)
Condition II,
3 years later, the father's age will be five times the son's age.
or,(x+3)=5(y+3)
or,x+3=5y+15 - (ii)
Put the value of x from equation (i) in equation (ii), and we get,
or,(9y−16)+3=5y+15
or,9y−16=5y+15−3
or,9y−5y=12+16
or,4y=28
or,y=284
∴y=7
Put the value of y in equation (i), and we get,
or,x=9×7−16
or,x=63−16
∴x=47
So, (x,y) = (47,7)
Therefore, the required present ages of the father and the son are 47 years and 7 years respectively.
Learn more about age calculation here: https://brainly.com/question/26423521
onsider the series 310+32+152+752+3252+.... does the series converge or diverge? select answers from the drop-down menus to correctly complete the sta
Answers
The geometric series that has been provided is of divergence, since r>5.
The series is given as /10 + 3/2 + 15/2 + 75/2 + 325/2 +.....
The common ratio is
3/2 ÷ 3/10
= 3/2 ×10/3
= 5
Then,
15/2 ÷ 3/2
= 15/2 × 2/3
= 5
Thus, the common ratio is greater than 5. Therefore, the series is divergence.
Hence, the given geometric series is divergence, since r>5.
A series is considered to be convergent if the partial sums tend to a particular value, also known as a limit. In contrast, a divergent series is one whose partial sums do not approach a limit. Typically, the Divergent series either reach, reach, or don't reach a particular number.
To learn more about convergence and divergence: https://brainly.com/question/25881495
#SPJ4
On Tuesday, the Beef Market sold 400 pounds of prime rib steak at $9.98 per pound and 120 pounds of rib-eye steak at $6.49 per pound. What was the average cost in dollars per pound of the steaks sold on Tuesday
Answers
The average cost in dollars per pound of the steaks sold on Tuesday is 9.17 per pound.
Average cost:First step is to calculate the total cost for steaks
Total cost=400×$9.98 + 120×$6.49
Total cost=$4770.8
Second step is to calculate the total pound
Total pound=400 + 120
Total pound= 520
Third step is to calculate the average cost in dollars
Average cost=$4770.8 / 520
Average cost= 9.174615385
Average cost=9.17 per pound (Approximately)
Inconclusion the average cost in dollars per pound of the steaks sold on Tuesday is 9.17 per pound.
Learn more about average cost here:https://brainly.com/question/25799822
A teacher runs each morning before school. Last week, he ran a total of 5 3/4 miles. This week, he ran 4/5 of the total number of miles he ran last week. What is the total number of miles that the teacher ran last week and this week?
Answers
"What is the total number of miles that the teacher ran last week and this week, if he ran a total of 5 3/4 miles last week and 4/5 of that distance this week?" is 10 13/20 miles.
To find the total number of miles that the teacher ran last week and this week, we need to:
Determine how many miles the teacher ran this week.
Add that number to the total number of miles the teacher ran last week.
Let's start by finding out how many miles the teacher ran this week:
The teacher ran 4/5 of the total number of miles he ran last week, which means he ran:
(4/5) x 5 3/4 miles = 4 3/5 miles
Next, we can add the number of miles the teacher ran last week to the number of miles he ran this week to find the total number of miles he ran:
5 3/4 miles + 4 3/5 miles = 10 13/20 miles
Therefore, the teacher ran a total of 10 13/20 miles last week and this week combined.
plzzz help me plz Show how to find the x-intercepts of this parabola using factoring and the Zero Product Property. Select the answer which is the sum of all solutions to this equation, rounded to the nearest tenth.
y = -10x2 + 8x + 24
Answers
Answer:
x = -6/5 and x = 2
Step-by-step explanation:
y = -10x² + 8x + 24
0 = -2(5x+6)(x-2)
5x+6 = 0 x-2 = 0
5x = -6 x = 2
x = -6/5
2 regular pizzas and 3 small pizzas cost £22 6 regular pizzas and 12 small pizzas cost £78
find the cost of one each
Answers
Answer:
Regular pizza: £5
Small pizza: £4
Step-by-step explanation:
Let x = regular pizza
Let y = small pizza
6x + 12y = 78
2x + 3y = 22
I'm going to use elimination method to solve for y
6x + 12 y = 78
-3(2x + 3y = 22) = -6x -9y = -66
Eliminate the x
3y = 12
Divide both sides by 3
3y/3 = 12/3
y = 4
Substitute the y in one of the original equations
2x + 3(4) = 22
2x + 12 = 22
Subtract both side by 12
2x + 12 - 12 = 22 - 12
2x = 10
Divide both sides by 2 to solve for x
2x/2 = 10/2
x = 5
Answer:
small $3.4 regular $6.2
Step-by-step explanation:
2r +3s = 22.6
6r *12s = 78
Multiply the first by -3
-6r -9s = -67.8
6r +12 s = 78
Add both (6r will be cancelled)
3s = 10.2
s = 3.4 small = 3.4 (3 small = 10.2)
2r +10.2 = 22.6
2r = 12.4
r = 6.2
What are the solutions of the compound inequality 2d + 3 ≤ –11 or 3d – 9 > 15?
Answers
Step-by-step explanation:
1.
\(2d + 3 \leqslant - 11 \\ 2d \leqslant - 11 - 3 \\ 2d \leqslant - 14 \\ \frac{2d}{2} \leqslant \frac{ - 14}{2} \\ d \leqslant - 7\)
2.
\(3d - 9 > 15 \\ 3d > 15 + 9 \\ 3d > 24 \\ \frac{3d}{3} > \frac{24}{3} \\ d = 8\)
Welcome
Solve the system of equations:
y= 2x - 2
y= x2 - x-6
O A. (-1,-5) and (4,2)
O B. (0, -2) and (2, 2)
O C. (-1,-4) and (4, 6)
D. (-2,0) and (3,0)
Answers
Answer:
C) (-1, -4) and (4, 6)
Step-by-step explanation:
\(\textsf{Equation 1}:y=2x-2\)
\(\textsf{Equation 2}:y=x^2-x-6\)
Substitute Equation 1 into Equation 2 and solve for x:
\(\implies 2x-2=x^2-x-6\)
\(\implies x^2-3x-4=0\)
Find two numbers that multiply to -4 and sum to -3: -4 and 1
Rewrite the middle term as the sum of these two numbers:
\(\implies x^2-4x+x-4=0\)
Factorize the first two terms and the last two terms separately:
\(\implies x(x-4)+1(x-4)=0\)
Factor out the common term \((x-4)\):
\(\implies (x+1)(x-4)=0\)
\(\implies (x+1)=0 \implies x=-1\)
\(\implies (x-4)=0 \implies x=4\)
Substitute the found values of x into Equation 1 and solve for y:
\(x=-1 \implies y=2(-1)-2=-4\)
\(x=4 \implies y=2(4)-2=6\)
Therefore, the solution to the system of equations is:
(-1, -4) and (4, 6)
according to the graph, the system of linear equations has how many solutions?
a) 1
b) 2
c) no solutions
d) infinitely many

Answers
Answer: 1 solution
Step-by-step explanation:
The system of linear equations has a unique solution. Then the correct option is A.
What is a linear equation?A connection between a number of variables results in a linear model when a graph is displayed. The variable will have a degree of one.
The linear equation is given as,
y = mx + c
Where m is the slope of the line and c is the y-intercept of the line.
If the slopes are different then the system has one solution.
From the graph, the slope of the lines is different. Then the system of linear equations has a unique solution.
Thus, the correct option is A.
More about the linear equation link is given below.
https://brainly.com/question/11897796
#SPJ6
60 PTS WILL MARK BRAINLIEST IF CORRECT {see image} Write a rule for the function whose graph can be obtained from the given parent function by performing the given transformations. shift the graph 3 units to the left, reflect it across the x-axis and shrink it vertically by a factor of one half (1/2)

Answers
Answer:
y = -1/2 | x+3|
Step-by-step explanation:
y = f(x + C) C > 0 moves it left
C < 0 moves it right
y = Cf(x) C > 1 stretches it in the y-direction
0 < C < 1 compresses it
y = −f(x) Reflects it about x-axis
Our parent function is
f(x) = |x|
We want it 3 units left
y = f(x + 3)
y = |x+3|
Then reflected across the x axis
y = −f(x)
y = -|x+3|
Then shrink by 1/2 vertically
y = Cf(x)
y = -1/2 | x+3|
Answer:
y = -1/2 | x+3|
Step-by-step explanation:
simplify
\( \sqrt[6]{5} \times \sqrt[2]{5} = \)
sumplify
Answers
Step-by-step explanation:
\( \sqrt[6]{5} \times \sqrt[2]{5} \)
\( = {5}^{ \frac{1}{6} } \times {5}^{ \frac{1}{2} } \)
\( = {5}^{ \frac{1}{6} + \frac{1}{2} } \)
\( = {5}^{ \frac{1 + 3}{6} } \)
\( = {5}^{ \frac{4}{6} } \)
\( = {5}^{ \frac{2}{3} } \)
\( = \sqrt[3]{ {5}^{2} } (ans)\)
Give two solutions of the inequality: p ≤ 3
Answers
Answer:
3,2
Step-by-step explanation:
3 is equal to 3 which can be it and 2 is less than 3
Find AC
I'd appreciate any help!
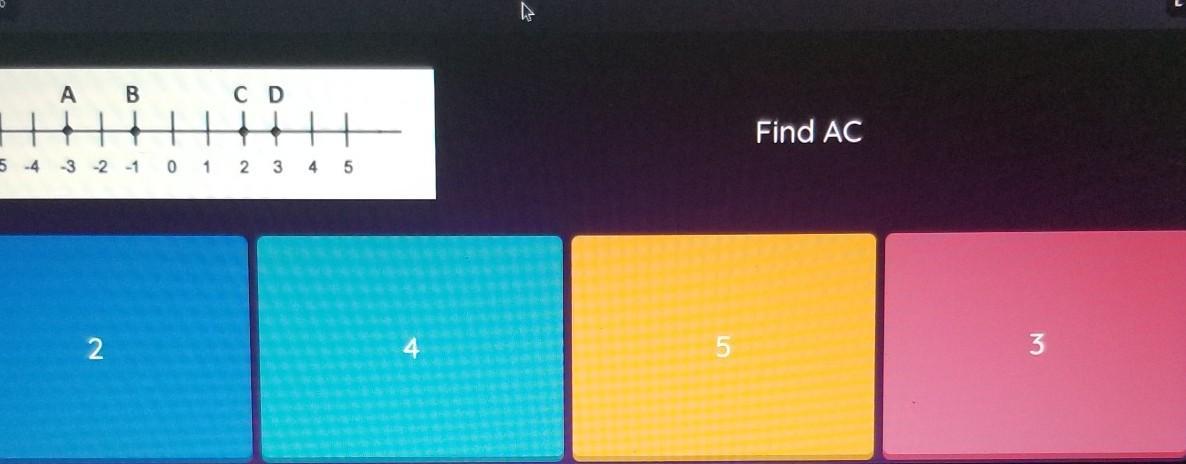
Answers
Explanation:
2 - (-3) = 5
3. What is the value of this expression (3.5 x 10 to the third power)?
(10 Points)
O 3500
O 3.50
000.35
O 0.350
Answers
Answer:
3500
Step-by-step explanation:
3.5 * 10^3 means you move the decimal 3 places to the right.
When calculating npv, the present value of the nth cash flow is found by dividing the nth cash flow by 1 plus blank______ rate raised to the nth power.
Answers
The discount rate raised to nth power.
What is NPV?NPV is defined as Net Present Value. It is mainly used in capital budgeting and investment planning for the purpose of analyzing the project profit. NPV is the difference between the present value of cash inflows and the present value of cash outflows in a period of time. The occurrence of cashflows in a series at different times is termed as NPV. NPV is a measure widely used in finance and commercial real estate. It is used to help taking decisions in accounting calculation. The cashflow that is discounted can be termed as NPV.
A simple example for NPV is, If a security offers a series of cashflows with an NPV of $30,000 and an investor pays exactly $30,000 for it, then the investor's, NPV is $0.
To know more about NPV, visit:
https://brainly.com/question/13084964
#SPJ4
What is 5log3+log4 as a single logarithm?
Answers
\(~~~5 \log 3 + \log4 \\\\=\log 3^5 + \log 4~~~~~~~~~~~~~~~~~~;[\log_b m^n = n \log_b m]\\\\=\log(3^5 \cdot 4)~~~~~~~~~~~~~~~~~~~~~~;[\log_b(mn) = \log_b m + \log_b n]\\\\=\log(972)\)
The smaller triangle is dilated to create the larger triangle. The center
of dilation is plotted, but not labeled.

Answers
Answer:
Point D is the point of dilation.
Step-by-step explanation:
If the smaller triangle A'B'C' is dilated to form a larger triangle ABC by the dilation about point D,
Ratio of the DA and DA' will be equal to the ratio of DB and DB'.
Length of DA = 3 units
Length of DA' = 1 unit
Coordinates of D → (3, 0)
Coordinates of B → (9, 3)
Coordinates of B' → (5, 1)
Use the formula of distance between the two points to find find the length of DB and DB'
Length of DB = \(\sqrt{(x_2-x_1)^2+(y_2-y_1)^2}=\sqrt{(3-0)^2+(9-3)^2}\)
\(=\sqrt{45}\)
\(=3\sqrt{5}\)
Length of DB' = \(\sqrt{(5-3)^2+(1-0)^2}\)
\(=\sqrt{5}\)
Ratio of DB and DB' = \(\frac{3\sqrt{5} }{\sqrt{5} } =3:1\)
Ratio of DA and DA' = 3 : 1
Since, \(\frac{DA}{DA'}=\frac{DB}{DB'}\)
Therefore, smaller triangle is dilated by a scale factor of 3 to form the bigger triangle about the point D.

Two solutions of different concentrations of acid are mixed creating 40 mL of a solution that is 32% acid. One-quarter o...
Answers
Answer:
See explanation below
Step-by-step explanation:
Question is incomplete. Here's the whole question:
"Two solutions of different concentrations of acid are mixed creating 40 ml of a solution that is 32% acid. one-quarter of the solution is made up of a 20% acid solution. the remaining three-quarters is made up of a solution of unknown concentration, c. equation can be used to determine c, the unknown concentration?
a) 30c + 10(0.2) = 40(0.32)
b) (c) + (0.2) = 40(0.32)
c) (c)( (0.2)) = 40(0.32)
d) 30(c)(10(0.2)) = 40(0.32)"
With this, we can now solve the question.
We have a solution that was made mixing2 different solutions of acid. In this case, we can assume the following:
Solution 1 = 40 mL 32% acid
Solution 2 = 1/4 solution 1, 20% acid
Solution 3 = 3/4 solution 1, c% acid.
Thus we can say:
Solution 1 = Solution 2 + Solution 3 (1)
Now, 1/4 of the volume of solution 1, means that we use 10 mL of a 20%acid, and the remaining 30 mL is the unknown acid.
With this, we can discart option b) and c) because these option are not considering the volume of solution 3 used. Option d is not doing any sum, only multiplications, so, this is not the option to use. We can easily say it's option a, but let's prove it.
In general terms, concentrations and volume are related, and if we have two solutions, the information of these two solutions must be conserved in the resulting solution. In other words:
M₁V₁ = M₂V₂
According to this, the concentration of the unknown acid would be:
30c + 0.2*10 = 40(0.32)
30c + 2 = 12.8
30c = 10.8
c = 0.36
Which means that the concentration of the acid is 36%
Hope this helps
Answer:
A. a) 30c + 10(0.2) = 40(0.32) !
Step-by-step explanation:
EDG
If -8-8y=6-2y, what is the value of y?
Answers
Answer:
-7/3
Step-by-step explanation:
The first step is to combine like numbers. Although there are several different ways to go about doing this, I started by adding 2y to both sides which left me with -8-6y=6. I then added 8 to both sides and got 6y=14. Now divide 6 on both sides to get "y" by itself which left me with y = -14/6. When you simplify the answer you get -7/3.
I’m confused on this

Answers
Answer:
x = 25
Step-by-step explanation:
The mistake here is the squaring. When you square one side, you have to do the same on the other side:
\(\sqrt{3x+6}\) = 9
\((\sqrt{3x+6}) ^{2}\) = \(9^{2}\)
3x + 6 = 81
3x = 75
x = 25
\(\huge\boxed{x=25}\)
The mistake is in Step 1. The solver should have also squared \(9\) when squaring the other side of the equation.
Correctly solving\(\begin{aligned}\sqrt{3x+6}&=9\\(\sqrt{3x+6})^2&=9^2\\3x+6&=81\\3x+6-6&=81-6\\3x&=75\\\frac{3x}{3}&=\frac{75}{3}\\x&=25\end{aligned}\)
you hire movers to deliver your new couch. it weighs 60 kilograms. how much does it weigh in pounds
Answers
Answer:
132.277 if this isnt the right answer i just googled how many pounds is 60 kg
Step-by-step explanation:
(b) Problem 15: Find the rate of change for this two-variable equation. y-x = 10
Answers
The rate of change for the equation y - x = 10 is 1.
To find the rate of change for the equation y - x = 10, we need to determine how y changes with respect to x.
We can rewrite the equation as y = x + 10 by adding x to both sides.
Now, we can observe that the coefficient of x is 1. This means that for every unit increase in x, y will increase by 1. Therefore, the rate of change for this equation is 1.
In other words, as x increases by 1 unit, y will increase by 1 unit as well.
As a result, 1 represents the rate of change for the equation y - x = 10.
for such more question on rate of change
https://brainly.com/question/22455842
#SPJ8
Three fourths times four sixths?
Answers
Answer:
0.5
Step-by-step explanation:
An iceberg and a ship separated by 98 miles are moving directly toward each other. The
iceberg is floating at 1 mile per hour, whereas the ship is traveling at 12 miles per hour.
Assuming that neither changes course, how long will it be before the two collide?
If necessary, round your answer to the nearest minute.

Answers
It will take approximately 3.77 hours for the iceberg and the ship to collide, rounded to the nearest minute, this is 3 hours and 46 minutes.
Velocity is a term used in physics to describe the rate at which an object changes its position. It is a vector quantity, meaning that it has both a magnitude (numerical value) and a direction.
Let's call the distance between the iceberg and the ship "d" and let's use the formula:
distance = rate × time
We know that the iceberg is moving at a rate of 1 mile per hour and the ship is moving at a rate of 12 miles per hour. Since they are moving towards each other, their effective rate of approach is the sum of their individual rates, which is 1 + 12 = 13 miles per hour.
We want to find out how long it will take for the two to collide, so let's use "t" to represent the time (in hours) it will take for the collision to occur.
Using the formula distance = rate × time, we can write:
d = 13t
We also know that the initial distance between the iceberg and the ship is 98 miles. Therefore, we can write:
d = 98 - 1t - 12t
The first term, 98, represents the initial distance between the iceberg and the ship. The second term, 1t, represents the distance that the iceberg moves in "t" hours, and the third term, 12t, represents the distance that the ship moves in "t" hours.
Now we can equate the two expressions for "d" and solve for "t":
13t = 98 - 1t - 12t
13t + 12t + 1t = 98
26t = 98
t = 3.77 hours
Therefore, it will take approximately 3.77 hours for the iceberg and the ship to collide, rounded to the nearest minute, this is 3 hours and 46 minutes.
To know more about velocity follow
https://brainly.com/question/28725327
#SPJ1
A similarity transformation consisting of a reflection
and a dilation is performed on a figure, and one point maps to itself. Explain how this can happen.
Answers
If the point in question is on the line of reflection, then the point won't move. Any point on a line of reflection stays where it is.
If that same point is the center of dilation, then it also doesn't move. The center of dilation is the only point that doesn't move in a dilation.
So the two conditions are:
The point must be on the line of reflectionThe point must be the center of dilationThese two facts mean that the line of reflection must go through the center of dilation.
Consider the following cumulative distribution function for the discrete random variable X.
x 1 2 3 4
P(X ≤ x) 0. 30 0. 44 0. 72 1. 00
What is the probability that X equals 2?
Question 3 options:
a) 0. 14
b) 0. 44
c) 0. 30
d) 0. 56
Answers
The probability that X equals 2 is 0.44.
We have given cumulative distribution function for the discrete random variable X. The probability that X equals 2 can be found by taking the difference between the probability that X is less than or equal to 2 and the probability that X is less than or equal to 1.
P(X = 2) = P(X ≤ 2) - P(X ≤ 1)
Using the cumulative distribution function given in the problem, we find:
P(X ≤ 2) = 0.30 + 0.44 = 0.74
P(X ≤ 1) = 0.30
Therefore,
P(X = 2) = 0.74 - 0.30 = 0.44
So the probability that X equals 2 is 0.44.
Learn more about Probability here
https://brainly.com/question/31604065
#SPJ4
Cara earn a bae pay of $1,800 per month at a car dealerhip plu a commiion of 6% of her ale. What are Cara' total earning in a month in which he ale $40,000 worth of merchandie?
Answers
Using the concept of percentages the total earning of Cara can be found to be $4200.
What are percentages?Percentage is a number expressed as a fraction of 100. The % sign means to divide the number by 100.
How to solve percentages?Cara's earning = commission + basic salary
basic salary = $1800 (this is constant)
commission = 6% of $40000
= (6/100)*40000
= $2400
Cara's earning = 1800 + 2400
Hence, her earning is $4200
To learn more about percentages visit the link below:
brainly.com/question/29306119
#SBJ4
−8tan 1+tan2x Use appropriate identities to rewrite the following expression in terms containing only first powers of sine
Answers
By using Pythagorean identities the expression can be written as
-8 (sin ( x ) + 1 -sin 2x)
The Pythagorean identity is an important identity in trigonometry derived from the Pythagorean theorem. These identities are used to solve many trigonometric problems where, given a trigonometric ratio, other ratios can be found. The basic Pythagorean identity, which gives the relationship between sin and cos, is the most commonly used Pythagorean identity:
sin2θ + cos2θ = 1 (gives the relationship between sin and cos)
There are two other Pythagorean identities as follows :
sec2θ - tan2θ = 1 (gives the relationship between sec and tan)
csc2θ - cot2θ = 1 (gives the relationship between csc and cot)
Given expression is:
-8tanx/ 1 +tan2x
we know that:
By the Pythagorean Theorem:
1 + tan²x = sec²x
and tan x = sin x/cos x
and, sec x = 1/cos x
Now, we can write as:
-8tanx / 1 +tan²x
= -8 tan x / sec²x
= -8 sin x /cos x ÷ 1/cos²x
= -8 sin x/cos x × cos²x/1
= -8 (sin ( x ) + 1 -sin 2x)
Complete Question:
Use appropriate identities to rewrite the following expression in terms containing only first powers of sine:
−8tan 1 + tan2x.
Learn more about Pythagorean identities
https://brainly.com/question/10285501
#SPJ4