American Airlines flights from Dallas to Chicago are on time 80% of the time, suppose 15 flights are randomly selected and the number of on-time flights is recorded. a.) Explain why this ?s a binomial experiment. b.) Find and interpret the mean. c.) Find and interpret the probability that exactly 10 flights are on time. d.) Find and interpret the probability that at least 10 flights are on time. e.) Find and interpret the probability that fewer than 10 flights are on time. f.) Find and interpret the probability between 8 and 10, inclusive, flights are on time.
Answers
a) It satisfies the four requirements of a binomial experiment.
b) On average, we can expect 12 out of the 15 flights to be on time.
c) Here is a 19.6% chance that exactly 10 out of the 15 flights are on time.
d) There is a 99.96% chance that at least 10 out of the 15 flights are on time.
e) There is a 0.04% chance that fewer than 10 out of the 15 flights are on time.
f) There is a 54.7% chance that between 8 and 10, inclusive, out of the 15 flights are on time.
a) How to find that this is a binomial experiment?This is a binomial experiment because it satisfies the four requirements of a binomial experiment:
There are a fixed number of trials,Each trial has two possible outcomes (on time or not on time), The probability of success (on time) is constant for each trial,The trials are independent.b) How to find mean?The mean of a binomial distribution is μ = np, where n is the number of trials and p is the probability of success.
In this case, n = 15 and p = 0.8, so the mean is μ = np = 15 x 0.8 = 12. Interpretation: on average, we can expect 12 out of the 15 flights to be on time.
c) How to find probability that exactly 10 flights are on time?The probability of exactly 10 flights being on time can be calculated using the binomial probability formula:
P(X = k) = (n choose k) x p^k x (1-p)^(n-k), where X is the number of on-time flights, k = 10, n = 15, and p = 0.8.
Plugging in these values, we get:
P(X = 10) = (15 choose 10) x 0.8^10 x 0.2^5 ≈ 0.196
Interpretation: There is a 19.6% chance that exactly 10 out of the 15 flights are on time.
d) How to find probability hat at least 10 flights are on time?The probability of at least 10 flights being on time can be calculated using the binomial cumulative distribution function or by finding the complement of the probability that fewer than 10 flights are on time. Using the complement method, we can write:
P(X ≥ 10) = 1 - P(X < 10) = 1 - P(X ≤ 9)
To find P(X ≤ 9), we can use the binomial cumulative distribution function with n = 15, p = 0.8, and k = 9:
P(X ≤ 9) = Σ(k=0 to 9) (15 choose k) x 0.8^k x 0.2^(15-k) ≈ 0.0004
So, P(X ≥ 10) = 1 - P(X ≤ 9) ≈ 0.9996. Interpretation: There is a 99.96% chance that at least 10 out of the 15 flights are on time.
e) How to find probability that fewer than 10 flights are on time?The probability that fewer than 10 flights are on time can be calculated using the binomial cumulative distribution function:
P(X < 10) = Σ(k=0 to 9) (15 choose k) x 0.8^k x 0.2^(15-k) ≈ 0.0004
Interpretation: There is a 0.04% chance that fewer than 10 out of the 15 flights are on time.
f) How to find probability between 8 and 10?The probability between 8 and 10, inclusive, flights being on time can be calculated by adding the probabilities of each possible outcome between 8 and 10:
P(8 ≤ X ≤ 10) = P(X = 8) + P(X = 9) + P(X = 10)
Using the binomial probability formula with n = 15, p = 0.8, and k = 8, 9, and 10, we get:
P(8 ≤ X ≤ 10) ≈ 0.547
Interpretation: There is a 54.7% chance that between 8 and 10, inclusive, out of the 15 flights are on time.
Learn more about binomial distribution
brainly.com/question/31197941
#SPJ11
Related Questions
How do you use the zero factor property to solve a quadratic equation?.
Answers
By applying the Zero Product Property, which asserts that if the product of two numbers equals zero, then at least one of the quantities must also equal zero, quadratic equations in factored form can be solved.
Any factored quadratic equation, such as (a + b)(a b) = 0, can be solved using the Zero Product Property. If a+b=0, then either an or b must also equal zero, according to the zero product property. We can solve problems like (x+2)(x-5)=0 thanks to this fundamental characteristic.
According to the zero product property, if the sum of two quantities is zero, then either one or both of the quantities must also be zero. This equation can be rewritten as (x + 3) (2 x 1) = 0 to help you solve it.
Learn more about quadratic equation Visit: brainly.com/question/1214333
#SPJ4
Martha use 1 piece of paper and 1 piece of ribbon to make kites. The paper comes in packs of 3 pieces and the ribbon comes in packs of 4 pieces. What is the least number of kites Martha can make without having supplies left over?
ANSWER QUICKKKKKK
Answers
Give the sin, cos, and tan for the point on the Unit Circle at 5pi/3°

Answers
Since you want to solve this using a calculator you have to options, if you have a advance calculator you can just directly input the nnumber and then it will give you the answer.
While using a standard calculator, we must first convert the radian measurement into degree measurement to find the sin, cos and tan of an angle. Let's start;
First, we must conver 5π/3 into degree measurement to do that we just have to multiply our converting factor 180/π (since 180° is equal to π)
\(\frac{5\pi}{3}(\frac{180}{\pi})=\frac{900\pi}{3\pi}=300\)Therefore 5π/3 is just equal to 300°.
Then using a CALCULATOR you can directly input the following;
\(\begin{gathered} \sin 300^{\circ}=-\frac{\sqrt[]{3}}{2}=-0.866 \\ \cos 300^{\circ}=\frac{1}{2}=0.5 \\ \tan 300^{\circ}=-\sqrt[]{3}=-1.732 \end{gathered}\)Please help! Will mark brainliest if correct

Answers
Answer:
the answer is rectangle. I hope it helps!
Answer:
Triangle
Step-by-step explanation:
Because it looks like a Triangle 3 sides
how to solve equations with fractions and variables in the denominator
Answers
To solve equations with fractions and variables in the denominator, we need to eliminate it by moving it to the other side. To do this, we multiply both sides of the equation by the term in the denominator. This will cancel out the fraction and leave us with a simpler equation to solve.
For example, suppose we have the equation (3 + x) / x = 2. To get rid of the fraction, we multiply both sides by x. This gives us: x * (3 + x) / x = x * 2. The x in the numerator and denominator cancel out, leaving us with:
3 + x = 2x. Now we can solve for x by subtracting x from both sides: 3 = x
This is the solution of the equation.
To know more about variables here: brainly.com/question/15078630
#SPJ11
Bags of dog food are labeled 1 through 50. What is the probability that the dog will choose a numbered dog bag that is not a multiple of 11
Answers
Probability helps us to know the chances of an event occurring. The probability that the dog will choose a numbered dog bag that is not a multiple of 11 is 8%.
Given that Bags of dog food are labelled 1 through 50.
Now, the bags that are multiples of 11 are 11, 22, 33, and 44, therefore, a total of 4 bags are there.
Now, the probability that the dog will choose a numbered dog bag that is not a multiple of 11 is,
Probability = Number of bags that are not a multiple of 11/Total number of bags
= (50 - 4)/50
= 23/25
= 0.92
Hence, the probability that the dog will choose a numbered dog bag that is not a multiple of 11 is 8%.
Learn more about Probability:
brainly.com/question/795909
#SPJ4
What is the domain of ggg? Choose 1 answer: Choose 1 answer: (Choice A) A The xxx-values -7−7minus, 7, -4−4minus, 4, 000, 333, and 444 (Choice B) B -4 \leq x \leq 8−4≤x≤8minus, 4, is less than or equal to, x, is less than or equal to, 8 (Choice C) C The xxx-values -4−4minus, 4, -3−3minus, 3, 000, 222, and 888 (Choice D) D -7 \leq x \leq 4−7≤x≤4
Answers
The domain of ggg is option D: -7 ≤ x ≤ 4.
To determine the domain of a function, we need to identify the set of all possible values for the independent variable, in this case, x, for which the function is defined.
In option D, the domain is specified as -7 ≤ x ≤ 4. This means that x can take any value within the closed interval from -7 to 4, inclusive.
In other words, the domain of ggg includes all real numbers between -7 and 4, including -7 and 4 themselves. This interval represents the range of values for x that satisfy the given conditions for the function ggg.
Learn more about function from
https://brainly.com/question/11624077
#SPJ11
Describe the meaning of the translation (x + 6, y + 10).
All points are moved 6 units right and 10 units up
All points are moved 6 units left and 10 units up
All points are moved 6 units left and 10 units down
All points are moved 6 units right and 10 units down
Answers
Answer:
Step-by-step explanation:
The meaning of the translation (x + 6, y + 10) is that all points in a coordinate plane are moved 6 units to the right and 10 units up.
In other words, for any point (x, y) in the original position, after the translation, the new coordinates would be (x + 6, y + 10). This means that the x-coordinate of each point is increased by 6 units, resulting in a shift to the right, and the y-coordinate is increased by 10 units, resulting in a shift upwards.
A car covered a certain distance at a speed of 24 mph. While returning, the car covered the same distance at a speed of 16 mph. What was the average speed of the car for the entire journey?
please healp
Answers
Answer:
(96/5) mph, or 19.2 mph
Step-by-step explanation:
Average speed = (total distance traveled) / (total time elapsed)
Here we need to calculate the two elapsed times (going and returning)
Going: t1 = d/(24 mph)
Returning: t2 = d/(16 mph)
Less time is required for the outward bound trip.
16d + 24d
Total time elapsed: t = t1 + t2 = d/24 + d/16 = ------------------- = (5/48)*d hr
16*24
Thus, the average speed is (total distance traveled) / (total elapsed time)
2d
which here is --------------------- = (96/5) mph, or 19.2 mph
(5/48)d
If we take average speed into account in any direction, it equals (24 + 16) / 2 = 40 / 2 = 20mph.
What is average speed?Average speed is calculated by dividing a number by the time it takes to obtain that number. The SI unit of speed is meters per second.The average speed is represented by a scalar value. The magnitude indicates its absence of direction.Since this distances were the same, the average speed can be calculated directly by adding the speeds and dividing by 2. There is no need to weigh anything.If we consider speed in any direction, the average speed is:
(24 + 16) / 2 = 40 / 2 = 20mph
However, if direction is important, one number must be negative because the directions are opposite:
(24 - 16) / 2 = 8 / 2 = 4mph (in the positive direction)
Depending on the circumstances, either of these could be your response.
To learn more about average speed, refer to:
brainly.com/question/24739297
#SPJ2
What is the slope in the equation y = 2/5x - 6?

Answers
Answer:
2/5
Step-by-step explanation:
y=2/5x-6
m=2/5
y=mx+b
Slope: 2/5
y-intercept: 6
Step-by-step explanation:
Just Did Now, Am not Really good at asking questions
gabriella went skiing. she paid $35 to rent skis and $15 an hour to ski. if she paid a total of $95, how many hours did she ski?
Answers
Gabriella skied for 6 hours, Let x be the number of hours that Gabriella skied. We know that she paid $35 for ski rental and $15 per hour for skiing,
for a total of $95. We can set up the following equation to represent this information:
35 + 15x = 95
Solving for x, we get:
15x = 60
x = 4
Therefore, Gabriella skied for 6 hours.
Here is a more detailed explanation of how to solve the equation:
Subtract $35 from both sides of the equation.
15x = 60
15x - 35 = 60 - 35
15x = 25
Divide both sides of the equation by 15.
15x = 25
x = 25 / 15
x = 4
Therefore, x is equal to 4, which is the number of hours that Gabriella skied.
To know more about equation click here
brainly.com/question/649785
#SPJ11
How to plot the function 2x+1 and 3x ∧
2+2 for x=−10:1:10 on the same plot. x=−10:1:10;y1=2 ∗
x+1;y2=3 ∗
x. ∧
2+2;plot(x,y1,x,y2) x=−10:1:10;y1=2 ∗
x+1;y2=3 ∗
x,a ∧
2+2; plot( x,y1); hold on: plot( x,y2) x=−10:1:10;y1=2 ∗
x+1;y2=3 ∗
x. ∧
2+2;plot(x,y1); plot (x,y2) Both a and b What is the syntax for giving the tag to the x-axis of the plot xlabel('string') xlabel(string) titlex('string') labelx('string') What is the syntax for giving the heading to the plot title('string') titleplot(string) header('string') headerplot('string') For x=[ 1
2
3
] and y=[ 4
5
6], Divide the current figure in 2 rows and 3 columns and plot vector x versus vector y on the 2 row and 2 column position. Which of the below command will perform it. x=[123];y=[45 6]; subplot(2,3,1), plot(x,y) x=[123]:y=[45 6): subplot(2,3,4), plot (x,y) x=[123]:y=[456]; subplot(2,3,5), plot(x,y) x=[123];y=[456]; subplot(3,2,4), plot( (x,y) What is the syntax for giving the tag to the y-axis of the plot ylabel('string') ylabel(string) titley('string') labely('string')
Answers
To plot the function 2x+1 and 3x^2+2 for x = -10:1:10 on the same plot, we will use the following command:
x = -10:1:10;
y1 = 2*x + 1;
y2 = 3*x.^2 + 2;
plot(x, y1);
plot(x, y2)
This will plot both functions on the same graph.
To tag the x-axis of the plot, we can use the command `xlabel('string')`, and to tag the y-axis, we can use `ylabel('string')`.
Therefore, the syntax for giving the tag to the x-axis is `xlabel('string')`, and the syntax for giving the tag to the y-axis is `ylabel('string')`.
We can provide a heading to the plot using the command `title('string')`. Hence, the syntax for giving the heading to the plot is `title('string')`.
To plot vector x versus vector y in the 2nd row and 2nd column position, we use the command `subplot(2, 3, 4), plot(x, y)`. Therefore, the correct option is:
x = [123];
y = [456];
subplot(3, 2, 4);
plot(x, y).
To know more about command visit:
https://brainly.com/question/32329589
#SPJ11
Twenty less than thrice a number is 223
Answers
\(~~~~~~3x-20=223\\\\\implies 3x = 223+20\\\\\implies 3x = 243\\\\\implies x = \dfrac{243}{3}\\\\\implies x =81\\\\\text{The number is 81.}\)
What is the rate of change

Answers
Answer:
Step-by-step explanation:
y2-y1 9-5 4
--------- =. -------- =. ---- = 2
x2-x1 3-1 2
you are watching basketball games at a sports bar. at one point, 3 games simultaneously have a player shooting a free throw. the graphics on screen tell you that player a has a 0.84 chance of making their shot, player b has a 0.70 chance of making their shot, and player c has a 0.79 chance of making their shot. assuming independence of the players, what is the probability that exactly 1 player makes their shot?
Answers
If we are watching basketball games at sports-bar, then the probability that exactly 1 player makes their shot is 0.11436.
In order to calculate the probability that exactly 1 player makes their shot, we consider the individual probabilities of each player making their shot and the probabilities of the other players missing.
Let us denote the event that player A makes their shot as A, player B makes their shot as B, and player C makes their shot as C.
We want to calculate P(exactly 1 player makes their shot), which can be written as P((A and not B and not C) or (not A and B and not C) or (not A and not B and C)).
Since the events are assumed to be independent, we multiply the probabilities of each individual-event. So, required probability can be written as follows :
P(exactly 1 player makes their shot) = P(A and not B and not C) + P(not A and B and not C) + P(not A and not B and C)
= P(A) × P(not B) × P(not C) + P(not A) × P(B) × P(not C) + P(not A) × P(not B) × P(C),
= (0.84) × (1 - 0.70) × (1 - 0.79) + (1 - 0.84) × (0.70) × (1 - 0.79) + (1 - 0.84) × (1 - 0.70) × (0.79),
= 0.05292 + 0.02352 + 0.03792,
= 0.11436,
Therefore, the required probability is 0.11436.
Learn more about Probability here
https://brainly.com/question/24322238
#SPJ4
Find: (6m5 + 3 – m3 – 4m) – (–m5 + 2m3 – 4m + 6)
Answers
Answer: 7m⁵ -3m³ - 3
Working:
= (6m⁵ + 3 - m³ - 4m) -(-m⁵ + 2m³- 4m +6)
= 6m⁵ + 3 - m³ - 4m +m⁵-2m³+4m - 6
= 6m⁵ + m⁵-m³ -2m³ -4m + 4m - 6 +3
= 7m⁵ -3m³ - 3
Answered by Gauthmath must click thanks and mark brainliest
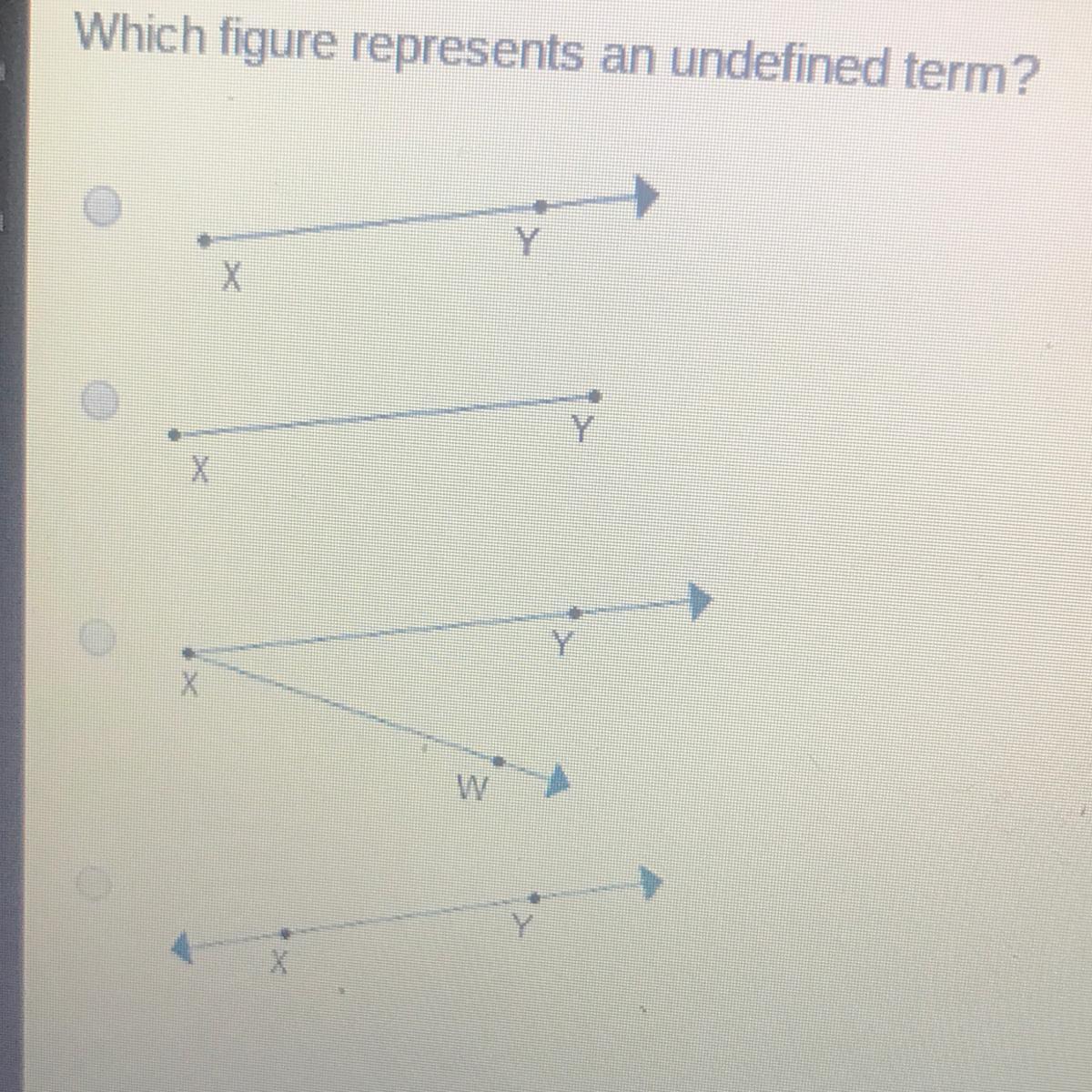
Please help which figure represents an under defined term?
Answers
Answer:
D
Step-by-step explanation:
Because its a line and the only three undefined terms are line,point,plane
Which angle has the greatest measure?

Answers
Answer:
It's G
Step-by-step explanation:
It has an acute angle while two of the other answers are vertical angles & the other answer choice is obtuse
The angle 12 has the greatest measure than the angle 5,7 and angle 8 option (F) ∠12 is correct.
What is an angle?When two lines or rays converge at the same point, the measurement between them is called a "Angle."
From the figure
The angle 5 and angle 7 are vertically opposite angles.
The angle 8 is an acute angle which is less than 90 degree.
The angle 12 is an obtuse angle which is greater than 90 degree.
Thus, the angle 12 has the greatest measure than the angle 5,7 and angle 8 option (F) ∠12 is correct.
Learn more about the angle here:
brainly.com/question/7116550
#SPJ2
Find the value of 6^5 ?
Answers
hope this helps :))
how to find volume of a prism
Answers
Answer:
- HOW TO FIND VOLUME OF PRISM -
1st step - Write the formula for finding the volume of a regular pentagonal prism. The formula is V = [1/2 x 5 x side x apothem] x height of the prism.
2nd step - Find the area of the pentagonal base face. Let's say the length of a side is 6 cm and the length of the apothem is 7 cm.
3rd step - Find the height. Let's say the height of the shape is 10 cm.
4th step - Multiply the area of the pentagonal base face times the height. ...
and last step - State your answer in cubic units.
Step-by-step explanation:
:):):)
A circular pie with a 14-inch diameter is cut into 10 equal-sized slices. If you ate 4 slices of pie, how much area of pie did you eat? Round to the nearest tenth.
Answers
Answer:
40%
Step-by-step explanation:
Since the pie is divided into 10 equal sections, we can assume they are all equivalent areas. So 4 slices out of the total of 10 would mean that 4/10 or 40% of the pie has disappeared, with you as the only witness. I suggest removing the evidence by consuming the remaining 6 slices.
what would be the sum and carry for this function on a truth table
Q = (X*2) + (Y/2) + Z
Answers
To find the sum and carry for this function on a truth table, we must first complete the truth table by computing the function's values for all possible inputs.
The given function is\(Q = (X*2) + (Y/2) + Z\)
Here's the truth table:X Y Z Q0 0 0 00 0 1 10 1 0 20 1 1 31 0 0.5 1.51 0 1 21 1 0.5 3.5 1 1 3
From the truth table above, we can see that the sum of Q for all eight input combinations is 12.
To find the carry, we will need to determine the maximum output value for the given function.
We can observe that the highest output value is 3.5.
Therefore, there is no carry for this function since the highest output value is less than four.
To know more about the word maximum visits :
https://brainly.com/question/30693656
#SPJ11
If you have 2 1/2 yards of fabric and want to make 3 pillows how much fabric will you need to use for each pillow
Answers
We know that
• You have 2 1/2 yards of fabric.
,• You want to make 3 pillows.
To find the number of yards of fabric you can use for each pillow, we just have to divide. We know that 2 1/2 is equivalent to 5/2 yards.
\(\frac{\frac{5}{2}}{3}=\frac{5}{2\cdot3}=\frac{5}{6}\)Therefore, you can use 5/6 yards of fabric for each pillow.y = 2x -1, y = 3x + 5 solve
Answers
Answer:
the answer is x= -6 and y= -13
Adapt the proof in the text that there are infinitely many primes to prove that there are infinitely many primes of the form 3k + 2, where k is a nonnegative inte- ger. (Hint: Suppose that there are only finitely many such primes 91,92, ..., In, and consider the number 39192 ... 9n – 1.]
Answers
We must conclude that there are infinitely many primes of the form 3k + 2, where k is a non-negative integer.
To adapt the proof:
We can use a similar contradiction argument.
Suppose that there are only finitely many primes of the form 3k + 2, say p1, p2, ..., pn.
Let N = 3p1p2...pn + 2. Note that N is of the form 3k + 2 for some non-negative integer k.
Now, let's consider the prime factorization of N. Either N is prime and of the form 3k + 2, in which case we have found a new prime of the desired form, contradicting our assumption that there are only finitely many such primes. Or, N is composite and has a prime factorization consisting only of primes of the form 3k + 1 (since any prime of the form 3k + 2 would divide N). But this implies that N itself is of the form 3k + 1.
Now, let's consider the number M = 3N + 2. M is also of the form 3k + 2, and so must have a prime factorization consisting only of primes of the form 3k + 1. But since N is of the form 3k + 1, we have
M = 3(3p1p2...pn + 1) + 2 = 9p1p2...pn + 5. This means that M has a prime factorization consisting of primes of the form 3k + 2, which contradicts our assumption that there are only finitely many such primes.
Therefore, we must conclude that there are infinitely many primes of the form 3k + 2, where k is a non-negative integer.
To know more about Integer:
https://brainly.com/question/27908445
#SPJ11
A point charge q with mass m is released from rest at the origin in an external magnetic field and an external electric field. Both of the external fields are uniform but the electric field points in the z-direction and the magnetic field points in the r-direction: Ē= Ek B = Boî where E, and Bo are constants. The electric field accelerates the point charge along the z-axis, but once the point charge has acquired a non-zero velocity along the z-direction it will also experience a magnetic force. a) Using Newton's second law and the Lorentz force, show that the acceleration of the point charge in each direction must satisfy the following: dr dy dz d2 EO dy dt2 dt2 dt dt2 Во dt where w is the cyclotron frequency qВо =0 mn and r(t), y(t), and z(t) give the position of the point charge along each axis as functions of time. b) The above equations are called coupled differential equations because they mix derivatives of y with derivatives of z (and vice versa). Show that these equations can be de-coupled by taking another time derivative. By de-coupled, I mean you should have an equation containing only derivatives of y with respect to t and an equation containing only derivatives of z with respect to t. c) The de-coupled equations are easier to solve, but let's not go through the actual steps. Instead I will just give you the general solution to the equations given in part a): r=Cit+C2 Eot y=C3 cos wt + C4 sin wt + + C5 Во z = C4 cos wt - C3 sin wt + C6 where C1, C2, C3, C4, C5, and Co are arbitrary constants. Show that these functions solve the equations from part a). You do NOT need to derive them, just plug them directly into the equations from part a). d) Recall that at t = 0 the point charge is at rest and located at (, y, z) = (0,0,0). Use these initial conditions to determine the values of the arbitrary constants. e) (Optional) Now try to sketch the motion of the charged particle. This can be tricky. It is helpful to take your expressions for y(t) and z(t) and notice that you can manipulate them into the equation for a circle, but one in which the center of the circle moves along the y-axis with constant speed. This is called cycloid motion and is identical to a wheel rolling at constant velocity.
Answers
a) The equation of motion in the z-direction is given by d²z/dt² = E₀ * q / m
b) We already have an expression for d²z/dt²
c) The given functions y(t) and z(t) satisfy the equations of motion from part a).
d) The conditions yield are
C₃ = 0
C₄ = 0
C₆ = 0
e) The exact shape of the cycloid depends on the specific values of the constants and the parameters of the system.
a) To derive the equations of motion for the charge, we start with Newton's second law:
F = m * a
Considering only the forces due to the external electric and magnetic fields, the net force can be written as:
F = q * E + q * (v x B)
where q is the charge of the particle, E is the electric field, v is the velocity vector, and B is the magnetic field. Since the charge is initially at rest, the velocity vector v is zero at t = 0.
Since the electric field is constant, the acceleration a of the charge must also be constant in the z-direction. Therefore, we can write:
a = d²z/dt² = E₀ * q / m
Now, let's consider the magnetic force. The magnetic force is given by:
F = q * (v x B)
Since the charge is initially at rest, the magnetic force is initially zero. However, once the charge acquires a non-zero velocity along the z-direction, it experiences a magnetic force perpendicular to both the velocity and the magnetic field. This magnetic force causes the charge to move in a circular motion in the yz-plane.
Therefore, we can write the equation of motion in the y-direction as:
F,y = m * d²y/dt² = q * vₓ * Bo
Dividing both sides by m:
d²y/dt² = q * vₓ * Bo / m
Using the relationship between the cyclotron frequency w and the product of charge, magnetic field, and mass:
q * Bo = m * w
we can rewrite the equation of motion in the y-direction as:
d²y/dt² = w * vₓ
Similarly, the equation of motion in the z-direction is given by:
d²z/dt² = E₀ * q / m
b) To decouple the coupled differential equations, we can take another time derivative of the equations of motion in the y and z directions.
Taking the time derivative of d²y/dt² = w * vₓ, we get:
d³y/dt³ = w * d(vₓ)/dt
Since we already have an expression for d²z/dt², we can leave it as it is.
c) Now, let's verify that the given general solution satisfies the equations of motion derived in part a).
For the y-direction equation of motion:
d²y/dt² = w * vₓ
Using the given general solution for y(t):
y = C₃ * cos(wt) + C₄ * sin(wt) + C₅ * Bo
Taking the first and second time derivatives:
dy/dt = -C₃ * w * sin(wt) + C₄ * w * cos(wt)
d²y/dt² = -C₃ * w² * cos(wt) - C₄ * w² * sin(wt)
Comparing this with the equation of motion, we can see that:
w * vₓ = -C₃ * w² * cos(wt) - C₄ * w² * sin(wt)
The terms on the right-hand side of the equation match the left-hand side when we choose:
C₃ = 0
C₄ = -vₓ / w
Now, let's move on to the z-direction equation of motion:
d²z/dt² = E₀ * q / m
Using the given general solution for z(t):
z = C₄ * cos(wt) - C₃ * sin(wt) + C₆
Taking the first and second time derivatives:
dz/dt = -C₄ * w * sin(wt) - C₃ * w * cos(wt)
d²z/dt² = -C₄ * w² * cos(wt) + C₃ * w² * sin(wt)
Comparing this with the equation of motion, we can see that:
E₀ * q / m = -C₄ * w² * cos(wt) + C₃ * w² * sin(wt)
The terms on the right-hand side of the equation match the left-hand side when we choose:
C₄ = E₀ * q / (m * w)
C₃ = 0
d) To determine the values of the arbitrary constants, we use the initial conditions provided: at t = 0, the point charge is at rest and located at (x, y, z) = (0, 0, 0).
From the given general solution, we have:
y(0) = C₃ * cos(0) + C₄ * sin(0) + C₅ * Bo = 0
z(0) = C₄ * cos(0) - C₃ * sin(0) + C₆ = 0
e) Finally, let's briefly discuss the motion of the charged particle. The solutions for y(t) and z(t) indicate that the particle moves in cycloid motion. The function y(t) describes the vertical displacement, and it oscillates sinusoid ally as the charge moves along the z-axis. The function z(t) describes the horizontal displacement, and it varies in a periodic manner as the charge moves in a circular path in the yz-plane.
If we visualize the motion in three dimensions, we can imagine a wheel rolling along the y-axis with constant speed, while simultaneously oscillating up and down. This combination of translational and oscillatory motion is known as cycloid motion.
To know more about Newton's second law here
https://brainly.com/question/15280051
#SPJ4
please take a pic of all the quiz answers of this please I NEED IT QUICK!!!

Answers
The sum of the given fraction is -1/3
Sum of fractionsFractions are expressions written as a ratio of two integers
Given the expression below;
5/6+(-1 1/6)
Convert the mixed fraction into improper fraction
5/6+(- 7/6)
Expand
5/6 - 7/6
Subtract
-2/6
-1/3
Hence the sum of the given fraction is -1/3
Learn more on sum of fraction here: https://brainly.com/question/17220365
#SPJ1
Fred's net worth is shown in the table below.
Assets are positive numbers and liabilities are negative
numbers If Fred's net worth is $74,000 how much
does he owe in credit card debt?
Item
House (current value)
Checking Account
Credit Card Debt
Vehicle (current value)
Student Loans
Personal Loans
Savings Account
Value
$105,900
$375
$13,500
-$32,000
-$800
$1,275
A) $6,725
B) $7,560
C) $9,750
D) $12,500
E) $14,250
F) $17,325
Answers
Fred owes $14,250 in credit card debt.
We must total up all of Fred's assets and liabilities, then subtract them to arrive at the amount he owes on his credit cards.
Let's figure it out:
Total Assets:
House (current value) = $105,900
Checking Account = $375
Vehicle (current value) = $13,500
Savings Account Value = $1,275
Total Liabilities:
Credit Card Debt = ?
Student Loans = $32,000
Personal Loans = $800
Total Assets - Total Liabilities = Net Worth
105,900 + 375 + 13,500 + 1,275 - (Credit Card Debt + 32,000 + 800) = 74,000
Solving for Credit card debt =
Credit Card Debt = 74,000 - 88,250
Credit Card Debt = -14,250 [The negative sign identifies it as a liability, as you can see.]
Therefore, Fred owes $14,250 in credit card debt.
Learn more about credit card debt click;
https://brainly.com/question/30626568
#SPJ1
You buy the following from Best Buy:
1 new Chromebook for $550, 1 wireless mouse for $20, 1 wireless keyboard for $20
What is the Subtotal: $
You use your Elite Members Best Buy credit card to save 6%, how much do you save: $
What is the new Subtotal: $
Sales tax is 5%: $
What is the total: $
t
Answers
Answer:
You buy the following from Best Buy:
1 new Chromebook for $550, 1 wireless mouse for $20, 1 wireless keyboard for $20
What is the Subtotal:
$550 + $20 + $20 = $590$590You use your Elite Members Best Buy credit card to save 6%, how much do you save:
$590 * 0.06 = $35.4$35.4What is the new Subtotal: $
$590 - $35.4 = $554.6$554.6Sales tax is 5%: $
$554.6 * 0.05 = $27.73$27.73What is the total: $
$554.6 + $27.73 = $582.33$582.33Step-by-step explanation:
heart and star pls <3 brainliest will be appreciated <3(っ◔◡◔)っ -{ elyna s }-Write a word description of the set. {−5, −4, −3, −2, −1}