Alexis invests 200 in a bank account that earns 1.45% interest compound annually. Write the models the growth of his own investment over X years?
Answers
Answer:
\(f(x) = 200( {1.0145}^{x} )\)
Related Questions
The mass of the Sun is 2 x 1030 kg.
The mass of the largest known star is 315 times the mass of the Sun.
(c) Work out the mass of this star.
Give your answer in kg in standard form.
Answers
The mass of the star in exponential form is M = 6.3 x 10³² kg
What is Exponential Form?The exponential form is an easier way of writing repeated multiplication involving base and exponents. It has a base and a power
The exponential form of a number is a way of representing a number using exponents, where the base is typically a number greater than 1.
It is often used in mathematics and science to represent large or small numbers more efficiently. It can also be used to simplify certain mathematical operations, such as multiplication and division, by using the properties of exponents.
Given data ,
Let the mass of the star be represented as M
Now , mass of the Sun is 2 x 10³⁰ kg
And , mass of star M = 315 x mass of sun
So , M = 2 x 10³⁰ x 315
From the laws of exponents , we get
M = 630 x 10³⁰
M = 6.30 x 10² x 10³⁰
On simplifying , we get
M = 6.3 x 10³² kg
Hence , the mass of the star is M = 6.3 x 10³² kg
To learn more about exponential form click :
https://brainly.com/question/29337692
#SPJ2
Solve 2x³+4x² - 16x=0.
The roots are x =
X =
and x =
Answers
Answer:
x ∈ {-4, 0, 2}
Step-by-step explanation:
You want the solutions to the cubic 2x³ +4x² -16x = 0.
FactorsWe observe that x and 2 are factors of all terms, so this can be written ...
2x(x² +2x -8) = 0
The quadratic will have binomial factors with constants that are factors of -8 that have a sum of 2.
2x(x +4)(x -2) = 0
SolutionsSolutions are the values of x that make the factors zero:
x = 0
x +4 = 0 ⇒ x = -4
x -2 = 0 ⇒ x = 2
The solutions are x = -4, 0, 2.

What is the multiplicative inverse of (- 3 4?
Answers
Multiplicative inverse of -3/4's is -4/3
A number that, when multiplied by the inputted number, produces the result 1 is said to be the multiplicative inverse of that number. It is a number's reciprocal according to the multiplicative inverse definition. A^-1 or denotes the multiplicative inverse of the number "a".
The multiplicative inverse of a number x is represented by x^-1, which has the property that when multiplied by the original number, it has a value of 1. For instance, 2^-1 is the multiplicative inverse of 2, as it meets the formula: 2 x 2^-1 = 2 x 12 = 1. The reciprocal of a number is another name for it.
Learn more about inverse Visit: brainly.com/question/3831584
#SPJ4
What number on the number line represents ground level?
0
-1
-2
-3
-4
-5
-6
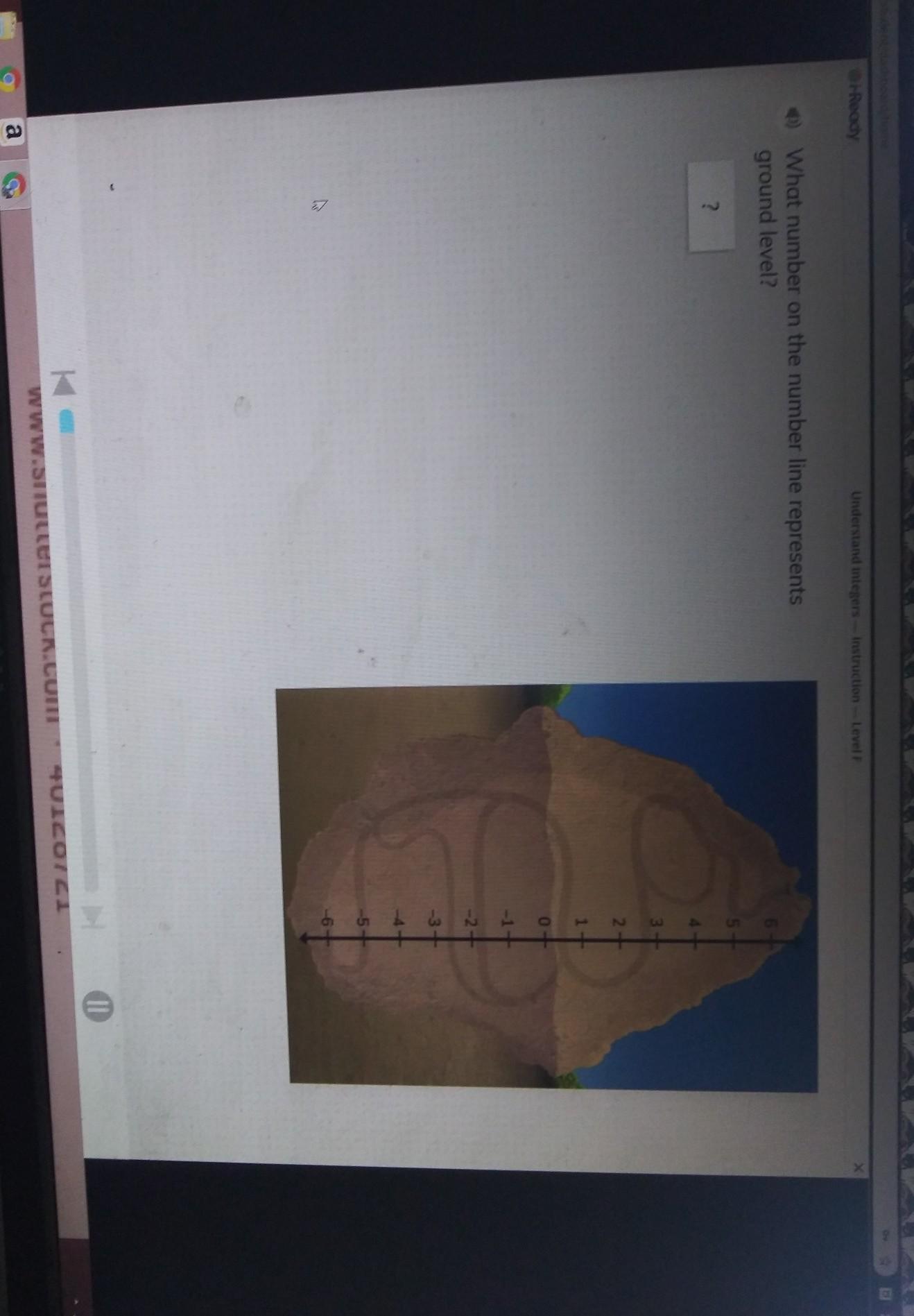
Answers
Answer:
0 represents ground level.
Step-by-step explanation:
0 on the number line represents ground level. Based on the photo, the 0 is in between the underground and the space above. The answer cannot be one of the negative numbers because those are all shown to be underground in the photo.
There are 6 bananas 4 apples and 3 oranges for every 1 orange there are how many bananas
Answers
Answer:
There are 18 bananas
Step-by-step explanation:
6 x 3 = 18
18 bananas.
What is the equation of the line in slope-intercept form 

Answers
The slope-intercept formula is one of the formulas used to determine a line's equation. Y = mx + b is the slope-intercept formula for a line with slope m and y-intercept b. Any point on the line is (x, y) in this case.
What is slope-intercept form?Using a straight line's slope and the location of its y-axis intersection, the slope intercept equation can be used to determine the general equation of the line. Y = mx + b is the equation in slope intercept form.
One of the formulas used to get a line's equation is the slope-intercept formula. Y = mx + b is the slope-intercept formula for a line with slope m and y-intercept b. Any point on the line is (x, y) in this case.
The variables we'll use in our function will be as follows:
m = slope, and b = y-intercept.
It is written as y = mx + b. The m and b variables are changed to numbers, while the x and y variables stay as letters (for example, y = 2x + 4, slope = 2, and y-intercept = 4).
The slope and intercept from an equation are used in the following video through a few examples.
y = mx + b
The slope and intercept are the two integers that make up this equation, which is why it is known as the slope-intercept form.
Remember, the slope (m) is the amount being multiplied to x and the intercept (b) is the number being added or subtracted
To learn more about slope-intercept refer to:
brainly.com/question/1884491
#SPJ1
Q. What is the area? A) 3 sq units B) 9 sq units C) 6 sq units D) 12 sq units

Answers
Considering the measure of each "small square" 1 x 1:
Each side of the complete square (a) measure 3 units.
Thus, since the area (A) is A = a², the area is:
\(\begin{gathered} A=3^2 \\ A=9 \end{gathered}\)Answer: 9 sq units.

a boat is 324 feet from the base of cliff. if the distance from the top of the cliff to the boat is 81 more than twice the height of the cliff. find the height of the cliff. round to the nearest tenth of a foot if necessary.
Answers
Given: A boat is located 324 feet from the base of the cliff.
The distance from the top of the cliff to the boat is 81 more than twice the height of the cliff, or 2h + 81.
We want to find: The height of the cliff, h.
Equations:
h + (2h + 81) = 324 (The height of the cliff plus the distance from the top of the cliff to the boat is equal to 324 feet)
3h + 81 = 324 (Expanding the left side of equation 1)
3h = 243 (Subtracting 81 from both sides of equation 2)
h = 81 (Dividing both sides of equation 3 by 3)
Therefore, the height of the cliff, h, is 81 feet.
To know more about Algebra
https://brainly.com/question/4344214
#SPJ4
Find the slope and y-intercept for the table.
slope:
y-intercept:

Answers
Answer:y is 60 slope is -10
Step-by-step explanation:
Please help my mathematic

Answers
a bag contains 14 blue, 6 red, 12 green, and 8 purple marbles. 25 marbles are removed from the bag randomly. how many of the removed marbles were green if the chance of drawing a green marble from the bag is now 3/5?
Answers
The number of green marbles removed from the bag is 3.
We will use the Formula of Probability:
In mathematics, the probability is defined as the ratio between the number of favorable outcomes and the total number of outcomes.
p(A) = n(A) / n(S)
p(A) is the probability of event A.
n(A) is the number of outcomes with respect to A.
n(S) is the total number of possible outcomes.
Given in the Question:
A bag contains 14 blue, 6 red, 12 green, and 8 purple marbles.
So the total number of marbles in the bag = 40
It is given that 25 marble are removed from the bag randomly
thus, the remaining marbles in the bag = 40 - 25 = 15
It is given that the probability of drawing a green marble from the bag after removing 25 marbles is 3/5.
So, for finding the number of green marbles in the bag after removing we can write,
n(A) = p(A) × n(S)
here, A is the event of drawing the number of green marbles and p(A) = 3/5 and n(s) = 15 (after removing)
Thus, the number of green marbles in the bag after removing = 3/5 × 15
So, the number of green marbles left in the bag = 9
then, the green marbles removed from the bag = 12 - 9 = 3.
Therefore, only 3 green marbles are removed from the bag.
To Learn more about similar Probability Questions:
https://brainly.com/question/21898039
find the midpoint of the line segment with these endpoints (1,9),(3-8)
Answers
The midpoint of the line segment with these endpoints (1,9),(3-8) is (2,0.5).
When the endpoints of a line segment are known, the midpoint of the line segment is given by:
X1+X2 Y1 + Y2
( ______ + ______ )
2 2
This forms a new coordinate you can call (x₃,y₃).
Here: (x₁,y₁) = (1,9), (x₂,y₂) = (3-8).
substituting the values in the formula we get the answer,
X3 = 2,
Y3 = 0.5.
so finally, the midpoint of the line segment with these endpoints (1,9),(3-8) is (2,0.5).
To know more about midpoints refer to:
https://brainly.com/question/14687140
Please help I needed it badly

Answers
Answer:
The answer is C trust me I did this
I need help again, but I did it step by step and I want to make sure it is the correct answer. Please help, thxs!!

Answers
Answer:
13!
Step-by-step explanation:
I hope I'm right and I hope it helps! *If I'm wrong again that would be really embarrassing.*
Resuelve: 6 (3n + 6) - 6 = -3 (n + 4)
Answers
Answer:
n = -2
Step-by-step explanation:
Dame lo más inteligente
( give me brainllest)

Mark has $18.00. What is the greatest number of days he can rent a bicycle? 2d=18 where d represents the number of days of the rental
Answers
Answer:
9
Step-by-step explanation:
9x2 is 18, so he can rent a bike for nine days if the rent is $2
Find the value of x.
A. 5.58
B. 9.14
C. 15.2
D. 10
PLEASE HELP!!! :(

Answers
Answer:
D. 10
Step-by-step explanation:
\({ \sf{(12x + 12) \degree = 79\degree + (5x + 3)\degree}} \\ { \sf{ \{outer \: angle \: is \: equal \: to \: sum \: of \: inner \: angles \}}} \\ { \sf{12x - 5x = 79 + 3 - 12}} \\ { \sf{7x = 70}} \\ { \sf{x = 10}}\)
Dan runs for 15 minutes at an average speed of 8 miles per hour.
He then runs for 50 minutes at an average speed of 9 miles per hour.
It takes Carol 75 minutes to run the same total distance that Dan runs.
Work out Carol's average speed.
Give your answer in miles per hour.
Answers
Carol's average speed is approximately 4.06 miles per hour.
We have,
We can use the formula:
distance = speed × time
First, let's find out how far Dan runs. We can start by converting his times to hours:
15 minutes = 0.25 hours
50 minutes = 0.83 hours
Now we can use the formula above to find the distances he runs:
distance1 = speed1 × time1 = 8 mph × 0.25 hours = 2 miles
distance2 = speed2 × time2 = 9 mph × 0.83 hours ≈ 7.47 miles
Total distance
= distance1 + distance2
= 9.47 miles
Since Carol runs the same total distance, we can use the formula to find her average speed:
average speed = total distance ÷ total time
We know the total distance is approximately 9.47 miles.
To find the total time, we need to add Dan's two times:
Total time
= 15 minutes + 50 minutes + 75 minutes
= 140 minutes
= 2.33 hours
Now we can substitute into the formula:
Average speed
= 9.47 miles ÷ 2.33 hours
= 4.06 mph
Therefore,
Carol's average speed is approximately 4.06 miles per hour.
Learn more about speed here:
https://brainly.com/question/7359669
#SPJ1
His school fundraiser raised 120% of last year‘s gold and 25% of this year’s school did the fundraiser raise more money this year. Explain your reasoning.
Answers
The fundraiser did not raise more money this year compared to last year.
What is a percentage?The percentage means the required value out of 100.
It is calculated by dividing the required value by the total value and multiplying by 100.
The percentage change is also calculated using the same method.
In percentage change we find the difference between the values given.
Example:
Required percentage value = a
total value = b
Percentage = a/b x 100
We have,
To determine if the fundraiser raised more money this year, we need to compare the amount raised this year to the amount raised last year.
Let's assume that the amount raised last year was $100.
If the fundraiser raised 120% of last year's goal.
Then the amount raised this year.
= 120% x $100
= 120/100 x 100
= $120
Now,
Let's assume that the goal for this year was $200.
If the fundraiser raised 25% of this year's goal.
Then the amount raised this year.
= 25% x $200
= 25/100 x 200
= $50
We can see that,
The fundraiser did not raise more money this year.
Thus,
The fundraiser did not raise more money this year compared to last year.
Learn more about percentages here:
https://brainly.com/question/11403063
#SPJ1
x+6/4 + x-3/5 = 5x-4/8
Answers
Answer:
x = 7/15
Step-by-step explanation:
x + 6/4 + x - 3/5 = 5x - 4/8
x + x - 5x = -4/8 - 6/4 + 3/5
-3x = -2/4 - 6/4 + 3/5
-3x = -8/4 + 3/5
-3x = -2 + 3/5
-3x = -10/5 + 3/5
-3x = -7/5
x = (-7/5)/-3
x = -7/(5*-3)
x = -7/-15
x = 7/15
Check:
7/15 + 6/4 + 7/15 - 3/5 = 5(7/15) - 4/8
14/15 + 6/4 - 3/5 = 35/15 - 2/4
56/60 + 90/60 - 36/60 = 140/60 - 30/60
146/60 - 36/60 = 110/60
A pool table is shown. On the table, a triangle is drawn to connect the cue ball, the eight ball, and the top right pocket. The distance between the cue ball and eight ball is 8 inches, the distance between the eight ball and the pocket is 6 inches, and the distance between the cue ball and the pocket is unknown.
Answers
Using Pythagoras' theorem we found that when the angle is greater than 90°, the distance between the cue ball and the pocket is greater than 10 inches.
What is Pythagoras' Theorem?
A right triangle's three sides are related in Euclidean geometry by the Pythagorean Theorem. According to this statement, the square of the side of a triangle known as hypotenuse is equal to the sum of squares of the other two sides of the triangle.
The complete question is given below in the image.
It is given that a triangle is formed by the cue ball, eight ball and pocket as shown below.
The ∠y is greater than 90°.
The two sides of the triangle are 8 in. and 6in.
Two find the minimum distance between the cue ball and the pocket, we assume that the minimum angle formed by the paths of the cue ball and eight ball is 90°.
That is ∠y = 90°.
Then the triangle becomes a right-angle triangle.
So the distance x becomes the hypotenuse.
Using the Pythagoras theorem, we can find the hypotenuse of the triangle.
x² = 8² + 6²
x² = 100
x = 10 inches.
This is the minimum distance between the cue ball and pocket when y = 90°.
Therefore using Pythagoras' theorem we found that when the angle is greater than 90°, the distance between the cue ball and the pocket is greater than 10 inches.
To learn more about Pythagoras' theorem, follow the link.
https://brainly.com/question/343682
#SPJ1


help
thanks!!!!!!!!!!!!!!!!!!!!!!!!!

Answers
the picture below shows the shape of a design painted on the side of a building. The design was formed by combining triangles and rectangles.
What is the area of the wall covered by the design?

Answers
Therefore , the solution of the given problem of surface area comes out to be 212 square feet of the wall are therefore covered by the design.
What exactly does an area mean?The total size of the object can be determined by calculating how much room would be required to completely cover its exterior. When choosing a similar product with a cylindrical form, the environment is taken into account. Anything's total dimensions are determined by its surface area. The amount of water that a cuboid can hold depends on the number of sides that link its four trapezoidal shapes.
Here,
We must first determine the area of each individual form before adding them together to determine the portion of the wall that the design covers.
Taking a look at the rectangle first, we can observe that it has the following area:
=> 120 square feet= 10 feet x 12 feet.
=> 40 square feet = (1/2)(10 ft)(8 ft).
Consequently, the two triangles' combined area is:
=> 80 square feet = 2 x 40 square feet.
=> (12 square feet) = (1/2)(6 ft)(4 ft).
The total area of all the shapes is as follows:
=> 212 square feet= 120 square feet, 80 square feet, and 12 square feet.
=> 212 square feet of the wall are therefore covered by the design.
To know more about surface area visit:
https://brainly.com/question/2835293
#SPJ1
Answer: the answer is 261 ^2 ft!
Step-by-step explanation:
You need to determine the area under the curve on the y-axis with the following conditions: The curve 4-6x-7+y=0 Limited by the y y' axes Lines y=-5 y=5 Make the graph and fill in the calculated area
Answers
To determine the area under the curve with the given conditions, we first need to find the points where the curve intersects the y-axis and the lines y = -5 and y = 5.
The equation of the curve is given by:
4 - 6x - 7 + y = 0
To find the y-intercept, we set x = 0 and solve for y:
4 - 7 + y = 0
y = 3
So, the curve intersects the y-axis at (0, 3).
Next, we find the points where the curve intersects the lines y = -5 and y = 5.
For y = -5:
4 - 6x - 7 - 5 = 0
-6x - 8 = 0
x = -4/6 = -2/3
So, the curve intersects y = -5 at (-2/3, -5).
For y = 5:
4 - 6x - 7 + 5 = 0
-6x + 2 = 0
x = 2/6 = 1/3
So, the curve intersects y = 5 at (1/3, 5).
Now, we can plot the graph and calculate the area under the curve between y = -5 and y = 5.
The graph should include the points (0, 3), (-2/3, -5), and (1/3, 5). The curve will pass through these points.
To calculate the area, we integrate the equation of the curve with respect to y between y = -5 and y = 5.
The equation of the curve in terms of y can be rearranged as:
y = 6x + 3
To integrate with respect to y, we need to express x in terms of y:
x = (y - 3)/6
Now we can calculate the area using the integral:
A = ∫[from y = -5 to y = 5] (x(y)) dy
A = ∫[from y = -5 to y = 5] ((y - 3)/6) dy
Evaluating the integral, we get:
A = [((y - 3)/6)y] [from y = -5 to y = 5]
A = ((5 - 3)/6)(5) - ((-5 - 3)/6)(-5)
A = (2/6)(5) - (-8/6)(5)
A = (10/6) + (40/6)
A = 50/6 = 25/3
Therefore, the calculated area under the curve between y = -5 and y = 5 is 25/3 square units.
To know more about equations visit-
brainly.com/question/32073464
#SPJ11
PLEASE HElp asap
Solve for x.
x = [?]
X + 19
7x + 9
![PLEASE HElp asap Solve for x.x = [?]X + 197x + 9](https://i5t5.c14.e2-1.dev/h-images-qa/contents/attachments/AjHw5XttQIYeYAvbID5YsoaNe7e2hLDH.png)
Answers
8x + 28 = 180
8x = 180 - 28
8x = 152
X = 152 : 8
X = 19
Can someone help me with this question?
This is question number 2

Answers
Answer:
y= -10x + 50
Step-by-step explanation:

Mrs Mabaspacked , prudence's mom packed a cooler box bag for the day of the painting . Two six pack cans fit exactly on top of each other in the cooler bag. A can has a diameter of 6 cm and a height of 8,84 cm 0:41 EZ07/67/90 dy the information given in the information above and answer the questions that follow. 2.1 2.2 2.3 2.4 Calculate the volume in ml of one can of cold drink, rounded to the nearest whole number. Determine the height of the cooler bag, rounded to the nearest whole number. Determine the volume in ml of the cooler bag if the breadth of the bag is 12 cm and the length 18 cm. Each can have a label on them as shown by the image below Piesse Circumference of the can NEW Diet, Soda 0 Calories! Calculate the length of the lable. CALORIES PER SERVING Nutrition Fac Hight of the can (3) (2) (3) (2) 27 [10]
Answers
2.1 The volume in ml of one can of cold drink is 83 ml.
2.2 The height of the cooler bag is 18 cm.
2.3 The volume in ml of the cooler bag if the breadth of the bag is 12 cm and the length 18 cm is 3,888 ml.
2.4 The circumference of the can is 18.84 cm.
How to calculate the volume of a cylindrical can?In Mathematics and Geometry, the volume of a cylinder can be calculated by using this formula:
Volume of a cylinder, V = πr²h
Where:
V represents the volume of a cylinder.h represents the height or length of a cylinder.r represents the radius of a cylinder.By substituting the given side lengths into the volume of a cylinder formula, we have the following;
Volume of can = 3.14 × (6/2)² × 8.84
Volume of can = 83.27 cm³.
Note: 1 cm³ = 1 ml
Volume of can in ml = 83.27 ≈ 83 ml.
Part 2.2.
For the height of the cooler bag, we have:
Height of cooler bag = 2 × height of can
Height of cooler bag = 2 × 8.84
Height of cooler bag = 17.68 ≈ 18 cm.
Part 2.3
Volume of cooler bag = length × breadth × height
Volume of cooler bag = 18 × 12 × 18
Volume of cooler bag = 3,888 ml.
Part 2.4
The circumference of the can is given by:
Circumference of circle = 2πr
Circumference of can = 2 × 3.14 × 3
Circumference of can = 18.84 cm.
Read more on cylinder here: brainly.com/question/14060443
#SPJ1
Missing information:
The question is incomplete and the complete question is shown in the attached picture.

Select the ones that correctly demonstrate the operations of scientific notation
Note that more than one option may be correct. Select all the correct answers
A. (4.0 x 103)(5.0 x 105) = 2 x 10^9
B. 4.5x105/9.0x10° = 2 x 10^4
C. (2.1 x 105) + (2.7 x 105) = 4.8 x 10^5
D. (3.1 x 105) - (2.7 x 102) = 0.4 x 10^3
Answers
Answer:
A and C
Step-by-step explanation:
A. \
(4.0 x 5.0)(10^3 x 10^5)
20 x 10^8
2.0 x 10^9
B. doesn't work, do not understand last number
C.
(2.1 + 2.7) (10^5 + 10^5) since powers are the same, do not need to change anything
4.8 x 10^5
D.
(3.1 - 2.7) (10^5 - 10^2) since powers are not the same, you need to make them equal
Do not need to subtract powers, hence D is untrue
Liz works as a lifeguard at her community pool and at the beach. On Saturday, she worked at the pool for 6 hours and earned $46.50. On Sunday, she worked at the beach for 5 hours and earned $46.25. How much more per hour did Liz earn at the beach than at the pool?
Answers
Answer:
$1.50 per hour more then at the pool
Step-by-step explanation:
7.75 per hour at the pool
9.25 per hour at the beach
9.25 - 7.75 = $1.5
A triangle has a perimeter of 8x 7. the first side of the triangle has a length of 4x, and the second side has a length of 2x − 3. what is the length of the third side of the triangle?
Answers
If triangle has a perimeter of 8x + 7. the first side of the triangle has a length of 4x, and the second side has a length of 2x − 3, the length of the third side of the triangle is 2x + 10.
To find the length of the third side of the triangle, we need to use the fact that the perimeter of a triangle is the sum of its three sides.
We are given that the perimeter of the triangle is 8x + 7, and we know the lengths of two sides: the first side has a length of 4x, and the second side has a length of 2x - 3. Let's use x as a common factor to simplify the expression for the perimeter:
Perimeter = 4x + (2x - 3) + (length of third side)
Perimeter = 6x - 3 + (length of third side)
8x + 7 = 6x - 3 + (length of third side)
Now we can solve for the length of the third side:
length of third side = 8x + 7 - 6x + 3
length of third side = 2x + 10
In general, when solving problems like this, it's important to remember that the perimeter of a triangle is the sum of its three sides, and to use algebraic equations to solve for unknown variables.
To learn more about triangle click on,
https://brainly.com/question/30293328
#SPJ4