A savings account of $5, compounded at an annual interest rate of 7% for 5 years will have how much total in the account?
Answers
Answer:
$6.75
Step-by-step explanation:
S.I=PRT/100
S.I=5×7×5/100
S.I=175/100
S.I=1.75
Principal + interest= total amount in account
$5+$1.75=$6.75
Related Questions
Please solve the attached question please!!!

Answers
Answer:
13
Step-by-step explanation
I noticed that 9 and 12 were skipped to 15 so I subtracted 15 to 6 and got 9 so I did 4 plus 9 and got 13 so yup hope this helped!!!
Answer:
10
Step-by-step explanation:
You will do cross multiplication 4×15 over 6= 10
g a sample of size 86 will be drawn from a population with mean 90 and standard deviation 24 . use the ti-83 plus/ti-84 plus calculator.
Answers
If the sample size 86 is drawn from population having mean 90 and S.D. as 24 , then the probability that mean will be more than 89 is 0.6517 .
In the question ,
it is given that ,
the sample size of the population (n) is = 86 ;
the mean (μ) = 90 , the standard deviation(σ) is = 24 ;
The probability that the mean will be more than 89 is represented as :
P(x > 89) = P((x-μ)/σ√n > (89 - 90)/24√86 )
= P(z > -0.39) ⇒ 1 - P(z ≤ -0.39)
from z table , we write the simplified equation as ;
= 1 - 0.3483
= 0.6517 .
Therefore , the required probability is 0.6517 .
The given question is incomplete , the complete question is
A sample of size 86 will be drawn from a population with mean 90 and standard deviation 24 . Find the probability that mean will be more than 89. Round the answer to at least four decimal places.
Learn more about Probability here
https://brainly.com/question/17285542
#SPJ4
If x = 2, y = 3 and z = -5, find the value of square root of x + y squared + z squared
Answers
The value of square root of x + y squared + z squared is 30
How to solve algebra?x = 2, y = 3 and z = -5
\(( \sqrt{x + y} )^{2} + z ^{2} \)
substitute the value of x, y and z
\( = ( \sqrt{2 + 3} )^{2} + - 5 ^{2} \)
simplify the square root and square
\( = (2 + 3) + 25\)
\( = 5 + 25\)
\( = 30\)
Ultimately, x + y squared + z squared is 30
Read more on algebra:
https://brainly.com/question/4344214
#SPJ1
when a square number is written as a product of prime numbers what can you say about the factors?
Answers
Answer:
The square of a prime number is prime
help meeeeee pwease
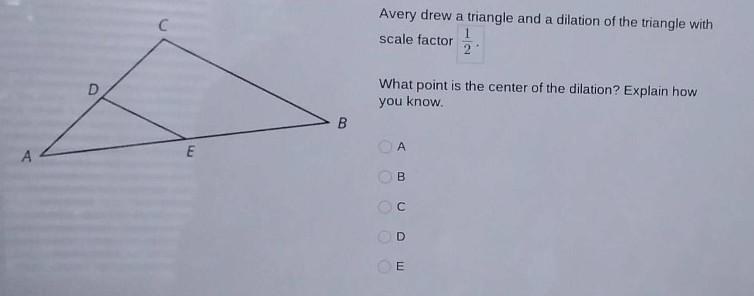
Answers
This month my metro water services bill was $36.34 and my Madison Suburban Utilty District bill was $26.03. My total water bill was $
Answers
Total water bill for the month is $62.37.
It seems that you may have accidentally left out the total amount of your water bill.
The total amount by simply adding the amounts of the individual bills together:
Total water bill =\($36.34 + $26.03\)
= \($62.37\)
You have not provided enough information to determine your total water bill.
You have only given the amounts of your individual bills from Metro Water Services and Madison Suburban Utility District.
To find your total water bill, you simply need to add the two bills together.
So, the total amount you owe for water this month would be:
Total water bill = \($36.34 + $26.03\)
= \($62.37\)
It appears that you may have forgotten to include the full amount of your water bill by accident.
Simple addition of the separate bill amounts yields the following sum:
Water bill total = \($36.34 + $26.03\)
= \($62.37\)
Your total water bill cannot be calculated because not enough information has been given.
Only the amounts of your individual Metro Water Services and Madison Suburban Utility District bills have been provided.
You just need to combine the two invoices together to get your total water bill.
As a result, this month's total water bill for you would be:
Water bill total = \($36.34 + $26.03\)
= \($62.37\)
For similar questions on water bill
https://brainly.com/question/31119915
#SPJ11
Complete each ratio table.
28
42
56
3.5
14
112.8
56.4
14.1
2,820
1,410
Answers
Answer: i don’t know
Step-by-step explanation:
Answer:34
Step-by-step explanation:
What is the circumference of a circle whose diameter is 5.7 ft? Use 3.14 for pi . Round your answer to the nearest tenth and enter it in
the box
Answers
Answer:
C≈17.91
Step-by-step explanation:
Answer:
17.91
Step-by-step explanation:
Find the hypotenuse of a right triangle with side lengths of 7 and 3. Round your
answer to one decimal place.
Answers
Answer:
7.616
Step-by-step explanation:
=7.6
The monthly utility bills in a city are normally distributed, with a mean of $ and a standard deviation of $. Find the probability that a randomly selected utility bill is (a) less than $, (b) between $ and $, and (c) more than $. (a) The probability that a randomly selected utility bill is less than $ is 0.0076. (Round to four decimal places as needed.) (b) The probability that a randomly selected utility bill is between $ and $ is 0.6859. (Round to four decimal places as needed.) (c) The probability that a randomly selected utility bill is more than $ is 0.0764. (Round to four decimal places as needed.)
Answers
Complete Question
The complete question is shown on the first uploaded image
Answer:
a
\(P(X < 65) = 0.003467\)
b
\(P(86 < X < 110 )= 0.63928 \)
c
\(P(X > 120 ) =0.062024\)
Step-by-step explanation:
From the question we are told that
The mean is \(\mu = \$ 100\)
The standard deviation is \(\sigma = \$ 13\)
Generally the probability that the monthly utility bills is less than $65 is mathematically represented as
\(P(X < 65) = P(\frac{X - \mu }{\sigma } < \frac{65 - 100 }{13 } )\)
Generally
\(\frac{X - \mu }{\sigma } = Z (The\ standardized \ value \ of\ X )\)
So
\(P(X < 65) = P(Z < -2.70 )\)
From the z-table
\(P(Z < -2.70 ) = 0.003467\)
So
\(P(X < 65) = 0.003467\)
Generally the probability that a randomly selected utility bill is between $86 and $110 is mathematically represented as
\(P(86 < X< 110 )= P( \frac{86 -100}{ 13} < \frac{X - \mu}{\sigma } < \frac{86 -100}{ 13} )\)
=> \(P(86 < X < 110 )= P( \frac{86 -100}{ 13} < Z < \frac{110 -100}{ 13} )\)
=> \(P(86 < X < 110 )= P(-1.08 < Z < 0.77 )\)
=> \(P(86 < X < 110 )= P(Z < 0.77 ) - P(Z < -1.08 ) \)
From the z-table
\(P(Z < 0.77 ) = 0.77935\)
and
\(P(Z < -1.08 ) = 0.14007\)
So
\(P(86 < X < 110 )= 0.77935 - 0.14007 \)
=> \(P(86 < X < 110 )= 0.63928 \)
Generally the probability that the monthly utility bills is more than $120 is mathematically represented as
\(P(X >120 ) = P(\frac{X - \mu }{\sigma } > \frac{120 - 100 }{13 })\)
=> \(P(X > 120 ) = P(Z > 1.538 )\)
From the z-table
\(P(Z > 1.54 ) = 0.062024\)
So
\(P(X > 120 ) =0.062024\)

Please help........ thank you

Answers
Hi!
So, first, let's just form the equation:
We know that all the side lengths equal 12 cm.
So the equation will be:
5 + 3 + x = 12
The answer you put in the box is:
5 + 3 + x
To find the value of x, we just solve this equation:
5 + 3 + x = 12
Combine like terms:
8 + x = 12
Same to both sides:
x = 12 - 8
x = 4
I hope this helped, let me know if you have any questions.
I just need the true or false questions done. Thank you

Answers
4. True
5. False
6. False
7. True
8. True
9. True
10. False
Plz help I’ll give 20 points

Answers
Answer: D
Step-by-step explanation: y=2 so 2+2=4+1=5
What is the equation of the vertical line through (−5,−2)
Answers
Answer: x = -5
Step-by-step explanation:
x = -5
For a vertical line in a coordinate plane, all points on the line have the same x-coordinate, so we can define the line just by giving the x-coordinate of any point on that line.
I hope this helps.
Michelle is hosting a party that starts at 6:00 PM. She realizes she did not freeze the ice cubes! She puts ice cubes in the freezer at 1:00 PM hoping they freeze by the time the party starts. The water is 82 degrees Fahrenheit when put into the freezer and will freeze when it reaches 32 degrees Fahrenheit. In Michelle's freezer the water cools to 80% of the temperature from the previous hour. Will the ice cubes freeze before the party starts?
Answers
Answer:
2 hours?
Step-by-step explanation:
I put it in a calc
Find the missing side in the similar figures below:

Answers
The missing side in the similar figure below is 42.
We are given a diagrammatic expression of two triangles and we are asked to find the missing side in a similar triangle. To be able to solve this question, we need to understand what are triangles and the possible types of triangles.
What is a Triangle?A triangle is a plane figure that has three sides and three angles. The major types of triangles include:
Right angle triangle (an angle that is 90°)Isosceles triangle (two sides of the triangle are equal)Scalene triangle ( no sides are equal)The example of the triangle given is a right-angle triangle and can be solved by using the Pythagoras theorem.
The Pythagoras theorem states that the hypotenuse squared is equal to the sum of the opposite squared and the adjacent squared.
hyp² = opp² + adj²
48² = x² + 24²
2304 = x² + 576
x² = 2304 - 576
x² = 1728
x = √1728
x = 41.57
x ≅ 42
Learn more about triangles here:
https://brainly.com/question/12852445
En una plaza Lucio camina en tramos rectos, a partir del asta bandera, en un punto cambia la dirección girando 150º a su izquierda, avanza 64 metros y se detiene. Para regresar al asta tiene que girar 75º a la izquierda, ¿A qué distancia se encuentra del punto inicial?
Answers
Lucio is 64 meters from the starting point.
How to solveThe square has four sides of equal length, so Lucio has walked half the length of one side.
To return to the starting point, he needs to walk the other half of the side, which is 64 meters.
The angle Lucio turns is irrelevant, as long as he turns 180 degrees in total.
With this in mind, it can be seen that based on the parameters and the conditions, Lucio is 64 meters from the starting point because the angle to which he turns is irrelevant.
Read more about angles here:
https://brainly.com/question/25716982
#SPJ1
The question in English:
In a square Lucio walks in straight sections, starting from the flagpole, at one point he changes direction turning 150º to his left, advances 64 meters and stops. To return to the pole you have to turn 75º to the left. How far are you from the starting point?
Li Wei and Colleen have the same reading assignment. After one week Li Wei has read 90 pages and Colleen has read 126 pages. If Li Wei can you read 30 pages in an hour and Colleen henry 24 pages an hour, when will they be on the same page 
Answers
Equating the expressions, if Li Wei can read 30 pages in an hour and Colleen Henry 24 pages an hour, they will be on the same page in 6 hours.
What are equivalent expressions?Equivalent expressions are two or more algebraic expressions that have the same value when the variables are substituted with real numbers.
In this situation, we can determine the time that Li Wei and Colleen Henry can be on the same page by equating the expressions representing their reading rates.
The number of pages Li Wei can read in a week = 90
The number of pages Collen can read in a week = 126
Li Wei's reading rate per hour = 30 pages
Collen's reading rate per hour = 24 pages
Let the hours that Li Wei and Colleen can be on the same page= x
Expressions:The total number of pages Li Wei can read = 90 + 30x
The total number of pages Colleen can read = 126 + 24x
For Li Wei and Colleen to be on the same, the two expressions are equated.
90 + 30x = 126 + 24x
6x = 36
x = 6
Learn more about equivalent expressions at https://brainly.com/question/2972832.
#SPJ1
35 POINTS!!!
What is the measurement angle of angle C and D in the image

Answers
Answer:
c = 45 and e = 30
Step-by-step explanation:
Since we know that A = 45, B = 90, we can calculate C knowing that the total angle of a triangle is 180, so you do 180-45-90 = 45
Finding the angle C helps us find F by subtracting 45 from 180, since a straight line is 180 degrees, which is 135.
We know that E = 15, and what was said earlier about how the toal angle is 180 in a triangle, we can subtract 15 and 135 from 180, which is 30.
Hope that answers your question
Consider the function
graphed at right.
Join multiple intervals
with a union.
A) Give the interval(s)
where the function is
increasing
B) Give the interval(s)
where the function is
decreasing
C) Give the interval(s)
where the function is
constant.
D) Give the domain of
the function using
interval notation
E) Give the range of the
function using interval
notation
Answers
Answer:
A) The interval(s) where the function is increasing are: $[-3,-1]$ and $[1,3]$.
B) The interval(s) where the function is decreasing is: $[-1,1]$.
C) The interval(s) where the function is constant is: $[-4,-3]$ and $[3,4]$.
D) The domain of the function is $[-4,4]$.
E) The range of the function is $[-1,3]$.
Step-by-step explanation:
Sure, here is a step-by-step explanation:
The given graph is a piecewise function consisting of three line segments, so we need to analyze the behavior of the function on each segment.
A) To find the interval(s) where the function is increasing, we look for the parts of the graph where the slope of the line is positive. We see that the function is increasing on the intervals $[-3,-1]$ and $[1,3]$. To write this in interval notation with a union, we can write: $[-3,-1] \cup [1,3]$.
B) To find the interval(s) where the function is decreasing, we look for the parts of the graph where the slope of the line is negative. We see that the function is decreasing on the interval $[-1,1]$. So, the interval where the function is decreasing is $[-1,1]$.
C) To find the interval(s) where the function is constant, we look for the parts of the graph where the slope of the line is zero. We see that the function is constant on the intervals $[-4,-3]$ and $[3,4]$. So, the interval where the function is constant is $[-4,-3] \cup [3,4]$.
D) To find the domain of the function, we need to consider all the values of $x$ for which the function is defined. From the graph, we see that the function is defined for all $x$ in the interval $[-4,4]$. So, the domain of the function is $[-4,4]$.
E) To find the range of the function, we need to consider all the values of $y$ that the function can take. From the graph, we see that the smallest value of $y$ is $-1$ and the largest value is $3$. So, the range of the function is $[-1,3]$.
Simplify the expression 1/6(12x-30)+18x

Answers
Which of the following statements is true regarding that equation |x+3|-2=k?

Answers
The true statement is if k = - 1 there are solutions, but if k = - 3 there are no solutions
How to determine which statement is true regarding the equation |x+3|-2=k?
Given: |x+3|-2=k
Below are rules for solving absolute value equations:
1. If p is positive and |y| = p, then
x = p OR y = -p
(two equations are set up)
2. If p is negative and |y| = p, then
No solution
3. If p is zero and |y| = p, then
One solution
Using the rules:
|x+3|-2=k
when k = -1
|x+3|-2= -1
|x+3| = 1
Rule 1 is applicable here. Thus, there are solutions
when k = -3
|x+3|-2 = -3
|x+3| = -1
Rule 3 is applicable here. Thus, no solution
Therefore, if k = - 1 there are solutions, but if k = - 3 there are no solutions. The 2nd option is the true statement
Learn more about absolute value equation on:
brainly.com/question/28728226
#SPJ1
Please help!! markup and markdown word problems.
1. A boat is marked up 20% on the original price. The original price was $50. What is the sale price of the boat before sales tax?
2. Fred buys a video game disk for $4. There was a discount of 20%. What is the sales price?
3. A painting is on sale at 50% off. The sale price is $320. What was the original price?
Answers
Answer:
Step-by-step explanation:
1. A boat is marked up 20% on the original price. The original price was $50. What is the sale price of the boat before sales tax?
$50(1+0.20) = $60
2. Fred buys a video game disk for $4. There was a discount of 20%. What is the sales price?
$4(1-0.2) = $3.20
3. A painting is on sale at 50% off. The sale price is $320. What was the original price?
X*(1-0.5) = $320
X = $320/0.5
X = $640
Which sequence of transformations will map PQ onto RS?

Answers
A sequence of transformations that would map PQ onto RS include the following: A. a 90° clockwise rotation about the origin, then a reflection in the x-axis.
What is a reflection over the x-axis?In Mathematics and Geometry, a reflection over the x-axis is represented by this transformation rule (x, y) → (x, -y). This ultimately implies that, a reflection over or across the x-axis would maintain the same x-coordinate while the sign of the x-coordinate changes from positive to negative or negative to positive.
For a 90° clockwise rotation about the origin, the new coordinates of P is given by:
(x, y) → (y, -x)
P (-6, 10) → (10, -(6)) = P' (10, -6)
Next, we would apply a reflection over or across the x-axis;
(x, y) → (x, -y)
P' (10, -6) → (10, -(-6)) = S (10, 6)
Read more on rotation here: brainly.com/question/28854313
#SPJ1
In his collection, Marco has 7 large gold coins, 10 large silver coins, 12 small gold
coins, and 3 small silver coins. If he randomly picks a coin, what is the probability
that it is gold, given that the coin is small?
Answers
The Probability that the randomly chosen coin is gold, given that it is small, is 4/5 or 0.8 when expressed as a decimal.
To find the probability that the randomly chosen coin is gold, given that it is small, we need to determine the number of small gold coins and the total number of small coins.
From the given information, Marco has 12 small gold coins and 3 small silver coins, making a total of 12 + 3 = 15 small coins.
The probability that the coin is gold, given that it is small, can be calculated as the ratio of the number of small gold coins to the total number of small coins:
P(Gold|Small) = Number of Small Gold Coins / Total Number of Small Coins
P(Gold|Small) = 12 / 15
Simplifying the fraction, we have:
P(Gold|Small) = 4 / 5
Therefore, the probability that the randomly chosen coin is gold, given that it is small, is 4/5 or 0.8 when expressed as a decimal.
For more questions on Probability .
https://brainly.com/question/25839839
#SPJ8
A data set has a median of 12, an upper quartile of 15, a lower quartile of 10, a minimum of 4, and a maximum of 20. Which statement is true of the box plot of this data set? The box will go from 10 to 12. A line dividing the box will be at 12. The left whisker will go from 4 to 15. The right whisker will go from 12 to 20.
answer = Option (B) A line dividing the box will be at 12. <=======+
Answers
Answer:
B
Step-by-step explanation:
got it
Answer:
B is correct
Step-by-step explanation:
A data set has a median of 12, an upper quartile of 15, a lower quartile of 10, a minimum of 4, and a maximum of 20. Which statement is true of the box plot of this data set?
The box will go from 10 to 12.
A line dividing the box will be at 12.
The left whisker will go from 4 to 15.
The right whisker will go from 12 to 20.
N
50°
AOMN~ ARPQ
Find 0.
M
Ө
0 = [?]°
<
P
70°
R
![N50AOMN~ ARPQFind 0.M0 = [?]<P70R](https://i5t5.c14.e2-1.dev/h-images-qa/contents/attachments/veKTlRrJcwtcPEhjGPeUEj7WPgDpy10G.png)
Answers
Answer:
60°
Step-by-step explanation:
In similar triangles, the corresponding angles are congruent.
∠O = R
O = 70°
In ΔOMN,
∠O + ∠M + ∠N = 180 {Angle sum property of triangle}
70 + 50 + Ф = 180
120 + Ф = 180
Subtract 120 from both sides,
Ф = 180 - 120
Ф = 60°
how did you find the gcf of the numerical coefficients of each term
Answers
The required GCF for the expression is 5y
Since we are not given the expression to get the GCF. Using the function
5x²y + 25y²
From both terms, find the common factor
5x²y = 5 × x × x × y25y² = 5 × 5 × y × yFrom both factors, we can see that 5 and y are common to both
Multiply the factors to get the GCFGCF = 5 × y
GCF = 5y
Hence the required GCF for the expression is 5y
NB: Same process is application to any other expressions
Learn more here: https://brainly.com/question/219464
I need a help with this hw

Answers
The values of the trigonometric ratios are: a) - 7/5, b) -75 c ) -1/5 d ) -25/12
What is trigonometric ratio?
Trigonometric ratios are measurements of the lengths of two sides of a triangle. There are three basic trigonometric ratios: sine, cosine, and tangent. Sine ratios are the ratios of the length of the side opposite the angle they represent over the hypotenuse
the give parameters are:
tanα = -4/3 where π/2 <α<π and sinβ = √3/2, 0<β<π/2
a) sin(α+β)
Using the trig ratios tan = opp/adj
but from pyth. rule, 4² + 3² = h²
16+9 = h²
h = √25 = 5
Now from trig Sin(α+β)
4/5 + 3/5
=- 7/5
b) cos(α+β)
cos = ad/hypo
4/5 + 3/5
= -7/5
c) Sin(α-β)
4/5 - 3/5
-1/5
d) tan(α-β)
= -4/3 - 3/4
= (-16 - 9)/ 12
-25/12
Learn more about trigonometric ratios on https://brainly.com/question/23130410
#SPJ1
Find the difference quotient f(x+h) -f(x)/h , where h does not equal 0, for the function below.
f(x)=-3x^2+6x-6
Answers
The difference quotient for the function f(x)=-3x²+6x-6 is -3h+6.
Now, let's move on to finding the difference quotient for the function f(x)=-3x²+6x-6. The difference quotient is a formula used to find the average rate of change of a function between two points.
The formula for the difference quotient is:
f(x+h) - f(x)
So, to find the difference quotient for the function f(x)=-3x²+6x-6, we first need to find f(x+h) and f(x):
f(x+h) = -3(x+h)² + 6(x+h) - 6
= -3(x² + 2xh + h²) + 6x + 6h - 6
= -3x² - 6xh - 3h² + 6x + 6h - 6
f(x) = -3x² + 6x - 6
Now we can plug these values into the difference quotient formula:
f(x+h) - f(x)
= [-3x² - 6xh - 3h² + 6x + 6h - 6] - [-3x² + 6x - 6]
= [-3x² - 6xh - 3h² + 6x + 6h - 6 + 3x² - 6x + 6] / h
= [-3h² + 6h] / h
= -3h + 6
This tells us the average rate of change of the function between x and x+h is -3h+6.
To know more about function here
https://brainly.com/question/28193995
#SPJ1