A puppy weighs534pounds.
how many ounces does the puppy weigh?
a.23 oz
b.46 oz
c.92 oz
ds.184 oz
Answers
Answer:
none of those answers are correct unless u mean 5 and 3/4
Step-by-step explanation:
Related Questions
How would I solve this problem?

Answers
Answer:
(x+1)²=-1
Step-by-step explanation:
x²-7x=-9x-2
x²+2x+2=0
If you solved this using the quadratic formula, you’d end up with -1+i and -1-i.
but if you subtracted 1 from both sides, you’d have
x²+2x+1=-1
Which is:
(x+1)²=-1
I suck at math..
Katoni bought 2 1/2 dozen pencils. There are 12 pencilsin a dozen. How many pencils did Katoni buy?
Answers
Answer:
30 pencils.
Step-by-step explanation:
Okay, So The answer is 30 pencils. First we must know that 2 dozen is equivalent to 24. Our fraction part is 1/2, what is 1/2 of a dozen? 6, so we must add 6 to 24 to get our answer of 30! Hope that helped : D
I've been very confused for hours now on when to put an imfity sign when doing quadratic inequalities pls help
Answers
Answer:
The symbol (∞) is read as infinity. and indicates that the set is unbounded to the right on a number line. Interval notation requires a parenthesis to enclose infinity. The square bracket indicates the boundary is included in the solution.
Step-by-step explanation:
find x,y,z : 4x^2+2y^2+2z^2-4xy-4xz+2yz-2y+6z+10=0
Answers
I hope this helps.
Answer:-
∴x−3y−4z=0
Explanation:
First we rearrange the equation of the surface into the form f(x,y,z)=0
x2+2z2=y2
∴x2−y2+2z2=0
And so we have our function:
f(x,y,z)=x2−y2+2z2
In order to find the normal at any particular point in vector space we use the Del, or gradient operator:
∇f(x,y,z)=∂f∂xˆi+∂f∂yˆj+∂f∂zˆk
remember when partially differentiating that we differentiate wrt the variable in question whilst treating the other variables as constant. And so:
∇f=(∂∂x(x2−y2+2z2))ˆi+
(∂∂y(x2−y2+2z2))ˆj+
(∂∂z(x2−y2+2z2))ˆk
=2xˆi−2yˆj+4zˆk
So for the particular point (1,3,−2) the normal vector to the surface is given by:
∇f(1,3,−2)=2ˆi−6ˆj−8ˆk
So the tangent plane to the surface x2+2z2=y2 has this normal vector and it also passes though the point (1,3,−2). It will therefore have a vector equation of the form:
→r⋅→n=→a⋅→n
Where →r=⎛⎜⎝xyz⎞⎟⎠; →n=⎛⎜⎝2−6−8⎞⎟⎠, is the normal vector and a is any point in the plane
Hence, the tangent plane equation is:
⎛⎜⎝xyz⎞⎟⎠⋅⎛⎜⎝2−6−8⎞⎟⎠=⎛⎜⎝13−2⎞⎟⎠⋅⎛⎜⎝2−6−8⎞⎟⎠
∴(x)(2)+(y)(−6)+(z)(−2)=(1)(2)+(3)(−6)+(−2)(−8)
∴2x−6y−8z=2−18+16
∴2x−6y−8z=0
∴x−3y−4z=0
In which quadrants do solutions for the inequality y is less than or equal to two sevenths times x plus 1 exist? i, iii, and iv i, ii, and iii i and iv all four quadrants
Answers
The solutions for the inequality y ≤ 2/7x + 1 exist in quadrants I, III, and IV.
What is the quadrants?
The quadrants are the four regions that result from dividing a Cartesian coordinate plane into four parts using two perpendicular lines, the x-axis and y-axis.
The inequality y ≤ 2/7x + 1 represents a line on the x-y plane. The solutions for the inequality are the points on the plane that lie below or on the line. In a standard Cartesian coordinate system with x-axis and y-axis, the x-axis separates the plane into two parts: the upper half-plane (quadrant I) and the lower half-plane (quadrants III and IV). The y-axis separates the plane into two more parts: the right half-plane (quadrants I and IV) and the left half-plane (quadrants II and III).
So the solutions for the inequality y ≤ 2/7x + 1 lie in the region of the x-y plane that is in the lower half-plane (quadrants III and IV) and the right half-plane (quadrant I). Therefore, the solutions exist in quadrants I, III, and IV.
To learn more about quadrants, Visit
https://brainly.com/question/25038683
#SPJ4
Answer:
AS: I, III, and IV
Step-by-step explanation:
Q's; In which quadrants do solutions for the inequality y is less than or equal to two thirds times x minus 4 exist?
AS: I, III, and IV
Look at the graph for a better understanding :P

A simple random sample of 15 year old boys from one city is obtained in their weights are listed below use a 0.01 significance level to test the claim the these sample ways come from a population with a mean equal to 148 pounds. Assume that the standard deviation of the weight of a 15-year-old boys in the city is known to be 16.1 pounds. Use the traditional method of testing hypotheses.

Answers
Answer:
\/\/\/
Step-by-step explanation:
What you are testing is a distribution of means, because you have a sample of eleven individuals (N=11), a population mean, and the population variance. Basically, the problem is asking: what is the likelihood that the sample mean (153.45 lbs) could have been obtained from a population where (M=149) if the null hypothesis is true?
p1: 15 year old boys with a mean weight of 153.45 lbs from City "Unknown".
p2: 15 year old boys with a mean weight of 149.00 lbs from the population.
H1: The sample of boys from City "Unknown" was not drawn from a population where the average weight of 15 year old boys = 149lbs.
H0: The sample of boys from City "Unknown" was drawn from a population where the average weight of 15 year old boys = 149lbs.
Mean = 149 [μM = μ = 149]
Variance = 16.2squared/11 = 262.44/11 = 23.86 [σM2 = σ2/N]
Standard Deviation = 4.88 [σM = √σM2 = √(σ2/N)]
Shape = normal
Using .01 level of significance for a two-tailed test, the cutoff sample score is +/- 2.575
Z = (M-µ) / σM = (153.45 - 149) / 4.88 = .91
.91 < 2.575
σM or the Standard Error of the Mean is 4.88 so...
for 99% confidence interval,
lower limit = 153.45 + (-2.575)(4.88) = 140.88
upper limit = 153.45 + (2.575)(4.88) = 166.02
the 99% confidence interval = 140.88 — 166.02 (lbs)
the population mean (µ=149.00) is included in the interval, which confirms results of Z test.
Conclusion: retain null, there is <.01 probability that the sample from City "Unknown" was drawn from a population where the mean weight is NOT = 149.00lbs.
An environmental charity set a target to plant trees on 9000 m² of land in
September.
In September, it planted trees on 0.017 km² of land.
4
a) What area of land did the charity plant trees on? Give your answer in m²
b) Did it reach its target?
Answers
The area of land the charity planted was 17000 m². The charity met its target
What is an equation?An equation is an expression that is used to show how numbers and variables are related using mathematical operators
1 km = 1000 m
1 km² = 1000000 m²
An environmental charity set a target to plant trees on 9000 m² of land in September.
In September, it planted trees on 0.017 km² of land. Hence:
Area of land planted = 0.017 km² * 1000000 m² per km² = 17000 m²
17000 m² is greater than 9000m². The charity met its target
Find out more on equation at: https://brainly.com/question/29174899
#SPJ1
The long hand of the clock is about 5 inches long. How far does the end of the long hand of the clock travel in 2 1/2 hours?
Answers
The end of the long hand of the clock travels about 78.54 inches (25π) in 2 1/2 hours.
What is clock ?
Clock can be defined as a machine in which a device that performs regular movements in equal intervals of time is linked to a counting mechanism that records the number of movements
The long hand of the clock completes one full revolution in 12 hours. Therefore, in one hour, it travels a distance equal to the circumference of a circle with a radius of 5 inches, which is given by 2πr = 2π(5) = 10π inches.
In 2 1/2 hours, the long hand of the clock travels a distance equal to (2 1/2) x 10π = 25π inches.
So, the end of the long hand of the clock travels about 78.54 inches (25π) in 2 1/2 hours.
Learn more about clock here : brainly.com/question/82007
#SPJ1
what are the first six digits of the mathematical sign pi?
Answers
The first six digits of the mathematical sign PI are 3.14159.
Pi is a mathematical constant that is commonly used in geometry and trigonometry. The number is defined as the ratio of a circle's circumference to its diameter, and it is approximately 3.14159.
The value of PI has been computed to millions of decimal places, but the first six digits, which are 3.14159, are the most often used in computations.
The first six digits of the mathematical sign PI are 3.14159. These digits are frequently used to calculate the value of a circle's circumference or area. Pi is irrational, which means it can't be expressed as a fraction of two integers, and it goes on indefinitely without repeating.
Learn more about pi : https://brainly.com/question/31248489
#SPJ11
ASAP
Aqua town charges $4.50 admission plus $0.75 for each ride. The cost at Wet World is $2.50 admission plus $1.25 per ride.
a) Write an equation for the cost of going to Aqua Town.
b) Write an equation for the cost of going to wet world.
c) How many rides would you have to ride for both water parks to cost the same amount? What would the cost be? Write a system of equations( put your two equations equal to each other)to solve for x and y. Write your answer in the form (x,y).
Answers
Answer:a: y=4.5+0.75x
B: y=2.5+1.25x
C: 7.5
Step-by-step explanation:
A fish is swimming at a constant rate toward the ocean floor. The equation y=-7x-3 can be used to represent this situation, where y is the depth of the fish in meters below sea level and x is the number of seconds the fish has been swimming. Which statement best describes the depth of the fish, given this equation?
A. From a starting point of 7 meters below sea level, the fish is descending 3 meters per second.
B. From a starting point of 7 meters below sea level, the fish ascending 3 meters per second.
C. From a starting point of 3 meters below sea level, the fish is descending 7 meters per second.
D. From a starting point of 3 meters below sea level, the fish is ascending 7 meters per second.
Answers
Answer:
i think the answer is either c or d, but since the 7 is negative, i'd try c first.
Step-by-step explanation:
tell me if this is wrong.
ari thinks the perfect milkshake has 5 scoops of ice cream for every 3 spoonfuls of caramel. freeze zone makes batches of milkshakes with 10 scoops of ice cream and 7 spoonfuls of caramel. what will ari think about the amount of caramel in freeze zone's milkshakes?
Answers
Answer:
Step-by-step explanation:
Since we have been given that
Ari has 3 ounces of caramel for each 5 scoops of frozen yogurt .
Freeze Zone makes bunches of milkshakes with 6 ounces of caramel and 8 scoops of frozen yogurt.
As per Ari,
For each 5 scoops of frozen yogurt, he wants 3 ounces of caramel
For each 1 scoop of frozen yogurt, he wants
3 ounces of Caramel
For each 8 scoops of frozen yogurt, he wants
3/5 * 8 = 24/5= 4.8 ounces
In this way, Ari considers sum caramel is something else for ideal milkshake as there is just requirement for 4.8 ounces of caramel however Freeze Zone is utilizing 6 ounces of caramel.
Answer:
Step-by-step explanation:
5456
equations is the inverse of y = x^2-5?
Answers
Answer:
Step-by-step explanation:
1. Interchange x and y. We get: x = y^2 - 5
2. Solve for y: y^2 = x + 5, so that y = ±√(x + 5)
Help me with number 5 rn i need help its due soon.

Answers
Answer:
It's option B
= - 1/3 -(-5/12)
= - 1/3 +5/12
Because minus and minus is plus
- - = =
Hope it's help ^_^
if a rod is equal to 5 and a half yards, and a bridge is 770 yards, how many rods is that?
Answers
Answer:
The answer is 140
Step-by-step explanation:
770÷5.5=140
140 rods
770 yards ➗ 5.5 yards in every rod = 140 rods
In a study examining the effect of alcohol on reaction time, Liguori and Robinson (2001) found that even moderate alcohol consumption significantly slowed response time to an emergency situation in a driving simulation. In a similar study, researchers measured reaction time 30 minutes after participants consumed one 6-ounce glass of wine. Again, they used a standardized driving simulation task for which the regular population averages m = 400 msec. The distribution of reaction times is approximately normal with a = 40. Assume that the researcher obtained a sample mean of M = 422 for the n = 25 participants in the study.
1. Are the data sufficient to conclude that the alcohol has a significant effect on reaction time? Use a two-tailed test with a =. 1.
2. Do the data provide evidence that the alcohol significantly increased (slowed) reaction time? Use a one-tailed test with a =. 5.
3. Compute Cohen's d to estimate the size of the effect
Answers
The p-value for a one-tailed test with 24 degrees of freedom and a t-value of 3.5 is less than 0.01 and an effect size of 0.57 indicates that alcohol consumption has a significant impact on reaction time, and the magnitude of the effect is moderate to large.
To determine if the data is sufficient to conclude that alcohol has a significant effect on reaction time, we need to conduct a hypothesis test. We can use a two-tailed test because we are not sure if alcohol will increase or decrease the reaction time.
The null hypothesis (H0) is that the mean reaction time is equal to the regular population average of 400 msec. The alternative hypothesis (Ha) is that the mean reaction time is not equal to 400 msec due to alcohol consumption.
With a significance level of α = 0.1, we can use a t-test to test the hypotheses. The formula for the t-value is:
\(t = \frac{M - \mu}{s \sqrt{n}}\)
Where M is the sample mean, μ is the population mean, s is the sample standard deviation, and n is the sample size.
Plugging in the values from the problem, we get:
\(t = \frac{(422 - 400)}{(40 \sqrt{25})} = 3.5\)
The degrees of freedom for this test is n-1 = 24. Using a t-table or calculator, we find that the p-value for a two-tailed test with 24 degrees of freedom and a t-value of 3.5 is less than 0.01.
Therefore, we reject the null hypothesis and conclude that alcohol consumption has a significant effect on reaction time.
To determine if the data provides evidence that alcohol significantly increased (slowed) reaction time, we can use a one-tailed test because we are only interested in whether alcohol increases reaction time.
The null hypothesis (\(H_0\)) is that the mean reaction time is equal to the regular population average of 400 msec. The alternative hypothesis (Ha) is that the mean reaction time is greater than 400 msec due to alcohol consumption.
With a significance level of α = 0.05, we can use a t-test to test the hypotheses.
Using the same formula as before, we get:
\(t = \frac{(422 - 400)}{40 \sqrt{25} }=3.5\)
The p-value for a one-tailed test with 24 degrees of freedom and a t-value of 3.5 is less than 0.01.
Therefore, we reject the null hypothesis and conclude that the data provides evidence that alcohol significantly increased (slowed) reaction time.
Cohen's d is a measure of effect size that allows us to determine the magnitude of the difference between two means in terms of standard deviation units. Cohen's d is calculated by taking the difference between the two means and dividing it by the pooled standard deviation.
The formula for Cohen's d is:
\(d = \frac{M_1 - M_2}{s}\)
Where \(M_1\) and \(M_2\) are the means of the two groups being compared, and s is the pooled standard deviation.
In this case, we can use the regular population average of 400 msec as the comparison group, and the sample mean of 422 msec as the experimental group. The pooled standard deviation can be calculated using the formula:
\(s=\sqrt{\frac{((n_1-1)s_1^2 + (n_2-1)s_2^2)}{ (n_1 + n_2 - 2)} }\)
Where \(s_1\) and \(s_2\) are the standard deviations of the two groups, and \(n_1\)and \(n_2\) are the sample sizes.
Using the values from the problem, we get:
\(s=\sqrt{\frac{((25-1)40^2 + (1-1)0^2)}{ (25 + 1 - 2)} } = 38.91\)
Plugging in the values for\(M_1\), \(M_2\), and s, we get:
d = (422 - 400) / 38.91 = 0.57
The effect size (Cohen's d) of 0.57 suggests a moderate to large effect of alcohol on reaction time. According to Cohen's guidelines, an effect size of 0.2 is considered small, 0.5 is considered medium, and 0.8 or higher is considered large.
Therefore, an effect size of 0.57 indicates that alcohol consumption has a significant impact on reaction time, and the magnitude of the effect is moderate to large.
It is worth noting that Cohen's d only provides an estimate of effect size, and other factors such as the context of the study and the specific population being studied may influence the interpretation of the effect size. Nevertheless, Cohen's d is a widely used measure of effect size that allows for meaningful comparisons across studies and different variables.
To know more about null hypothesis click here:
brainly.com/question/28920252
#SPJ4
IF ANY OF YOU SOLVE I WILL GIVE BRAINLIEST AND EVERYTHING ELSEEEE PLS HELP
4x - 4; x=7
6 - x + y; x=5, y=3
Answers
Answer:
1. 4x-4=24
2. 6-x+y=4
Step-by-step explanation:
Answer:
4x(7) - 4= 24
6 - 5 + 3 = 4
Step-by-step explanation:
The junior and senior classes at Central High School were asked to choose a destination for a field trip. The results are shown in the given two-way frequency table.
Central High School Field Trip Destination
Amusement Park Museum Broadway Show Total
Juniors 57 21 42 120
Seniors 64 44 58 166
Total 121 65 100 286
What percentage of surveyed students chose the amusement park?
A.
38.55%
B.
47.50%
C.
19.93%
D.
42.31%
Answers
Answer:
D) 42.31%
Step-by-step explanation:
If 121 people went to the amusement park out of 286 total students, then the percentage of surveyed students that chose the amusement park would be 121/286=0.4230769231=42.31%, making option D correct.
Can anybody tell me if the answer I picked is right

Answers
Answer:
the answer is the second answer choice
Step-by-step explanation:
You have to pay close attention to the open and closed dots on beggining and end of your line
Been struggling with this question can someone help?

Answers
Answer:
a and b
Step-by-step explanation:
............. ...... ..
Answer:
The answer is B
Step-by-step explanation:
\(\frac{x}{4}=16\\\mathrm{Multiply\:both\:sides\:by\:}4\\\frac{4x}{4}=16\cdot \:4\\\mathrm{Simplify}\\x=64\)
The cost of 6 team sweatshirts is $84.
At this price, how much would it cost to
buy sweatshirts for a whole team of 24
players?
Answers
Answer:
336
Step-by-step explanation:
Use the function c=r+6 to find the value of c when r=10. c=
Answers
Answer:
c=16
Step-by-step explanation:
Answer:
16
Step-by-step explanation:
c = r+6
If r = 10 then,
c = 10+6
c = 16
Miguel compró 17 botellas de cerveza en el mercado y quiere trasladarlas a su vehiculo. Si solamente puede transportar 3 botellas a la vez, cuál es el mínimo número de viajes que debe hacer para llevar todas las botellas
Answers
Answer:
Minimum 6 tripsStep-by-step explanation:
Miguel bought 17 bottles of beer at the market and wants to transfer them to his vehicle. If he can only transport 3 bottles at a time, what is the minimum number of trips he must make to carry all the bottles?-----------------
The minimum number of trips is:
17/3= 5 2/3 ≈ 6 rounded upWe considered 2/3 as the whole 1 because you can't make partial trips
all you need is in the photo please answer fast

Answers
To solve for d:
1. Remove parenthesis:
\(0.2d-1.2=0.3d+5-3+0.1d\)2. Leave the terms with d in one side of the equation:
-Add 1.2 in both sides of the equation:
\(\begin{gathered} 0.2d-1.2+1.2=0.3d+5-3+0.1d+1.2 \\ 0.2d=\text{0}.3d+5-3+0.1d+1.2 \end{gathered}\)-Substract 0.3d and 0.1d in both sides of the equation:
\(\begin{gathered} 0.2d-0.3d-0.1d=0.3d-0.3d+5-3+0.1d-0.1d+1.2 \\ 0.2d-\text{0}.3d-0.1d=5-3+1.2 \end{gathered}\)3. Opperate similar terms:
\(-0.2d=3.2\)4. Divide into (-0.2) both sides of the equation:
\(\begin{gathered} \frac{-0.2}{-0.2}d=\frac{3.2}{-0.2} \\ \\ d=-16 \end{gathered}\)Then, d is -16five hamburgers cost 5.25 at this rate what is the cost of 8 hamburgers
Answers
Answer: 8.4
Step-by-step explanation: 5.25 divided by 5 is 1.05. So 1.05 x 8 is 8.4
2. Price of 1 is 1.05
3. 1.05 multiply by 8 is 8.40
ANSWER: $8.40 for 8
Is the following relation a function? No or yes
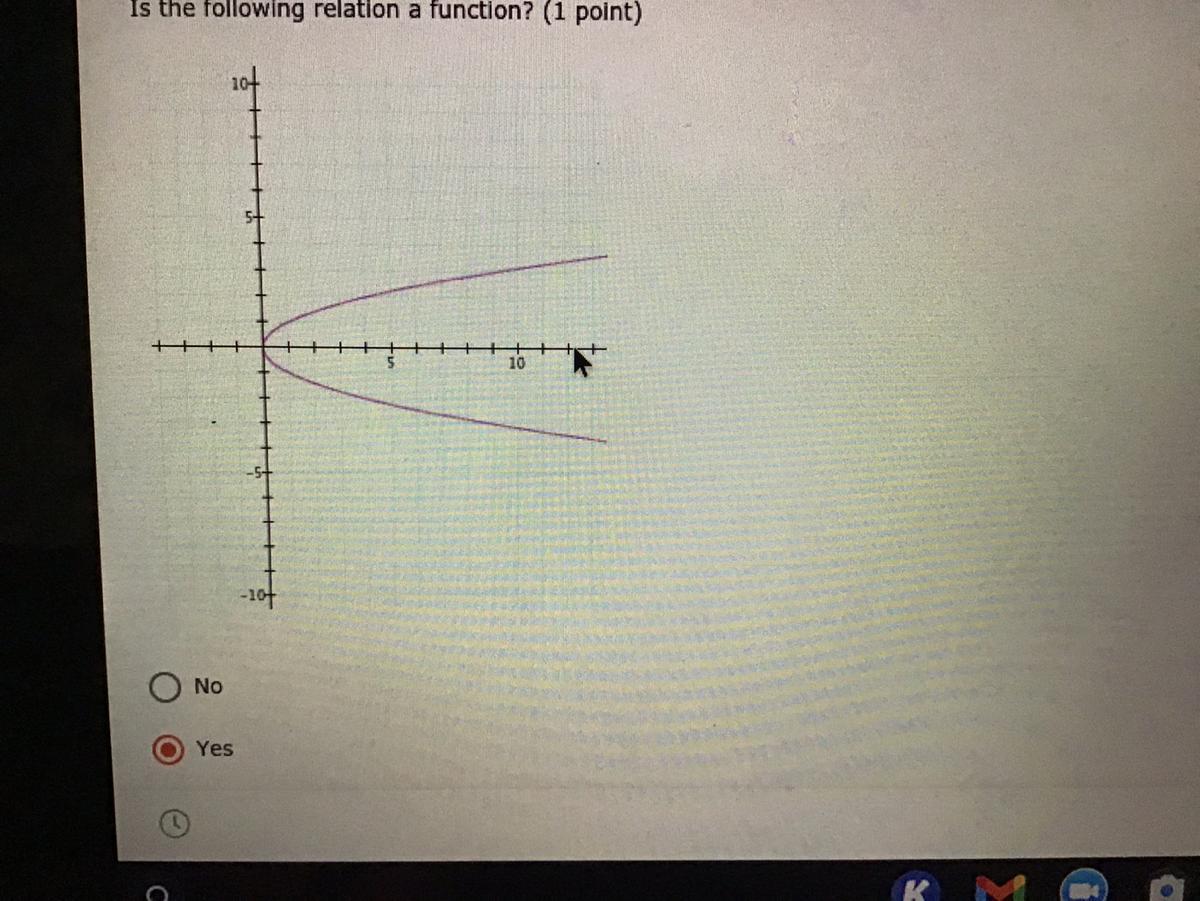
Answers
solve: -1/2 x + 3 = −x + 7
a) -8
b) 2
c) 1/2
d) 8
Answers
Match the graph with the correct equation.

Answers
Answer:
work shown and pictured

find the point on the curve r(t) = (-2sin(t),2cos(t),e^t) where the tangent line is parallel to the plane
Answers
The point on the curve where the tangent line is parallel to the plane is (-2sin(arccos(-a/2)), 2cos(arcsin(-b/2)), c).
To find the point on the curve r(t) = (-2sin(t),2cos(t),e^t) where the tangent line is parallel to the plane, we need to find the derivative of the curve and set it equal to the normal vector of the plane.
The derivative of the curve r(t) is:
r'(t) = (-2cos(t), -2sin(t), e^t)
The normal vector of the plane is (a,b,c).
To find the point where the tangent line is parallel to the plane, we need to set the derivative equal to the normal vector:
-2cos(t) = a
-2sin(t) = b
e^t = c
Solving for t, we get:
t = arccos(-a/2)
t = arcsin(-b/2)
t = ln(c)
Plugging these values of t back into the original equation for the curve, we can find the point on the curve where the tangent line is parallel to the plane:
x = -2sin(arccos(-a/2))
y = 2cos(arcsin(-b/2))
z = e^(ln(c))
So the point on the curve where the tangent line is parallel to the plane is (-2sin(arccos(-a/2)), 2cos(arcsin(-b/2)), c).
For more similar questions on curve:
brainly.com/question/26460726
#SPJ11
WILL MARK BRAINLIEST
On a coordinate plane, an absolute value graph has a vertex at (10, 6).
The graph of h (x) = StartAbsoluteValue x minus 10 EndAbsoluteValue + 6 is shown. On which interval is this graph increasing?
(–∞, 6)
(–∞, 10)
(6, ∞)
(10, ∞)
Answers
Answer:
(6, infinity) should be the answer.
Answer:
(10, ∞)
Step-by-step explanation:
Just took test. I got you bro