A public relations firm found that only 77% of voters in a certain state are satisfied with their state representative. How large a sample of voters should be drawn so that the sample proportion of voters who are satisfied with their representative is approximately normally distributed
Answers
A sample size of approximately 506 voters should be drawn to ensure that the sample proportion of voters who are satisfied with their representative is approximately normally distributed.
In order to determine the sample size needed for a normal distribution of sample proportions, we can use the formula:
n = (² * p * q) / E²
Where:
n is the sample size
Z is the z-score corresponding to the desired level of confidence (typically 1.96 for a 95% confidence level)
p is the estimated proportion of voters satisfied with their representative (0.77)
q is the complement of p (1 - p)
E is the desired margin of error (usually represented as a decimal value)
By plugging in the given values, we can calculate the sample size:
n = (1.96² * 0.77 * 0.23) / (0.05²)
n = 506.06
Therefore, a sample size of approximately 506 voters should be drawn to ensure that the sample proportion of voters who are satisfied with their representative is approximately normally distributed.
Learn more about proportion of voters
brainly.com/question/31233455
#SPJ11
Related Questions
HURRY PLEASE!! I’ll mark you as brainliest!! im being timed
the graph represents the water path of a fire hose. what is the maximum value of the function? explain what the maximum value represents in this situation.

Answers
Answer:
24 will be the maximum value of this graph
Answer: the maximum value is 24 vertical and 20 horizontal
Step-by-step explanation:
If a firm permanently borrows
$
100
million at an interest rate of
8
%
, what is the present value of the interest tax shield? (Assume that the tax rate is
30
%
.)
Answers
If a firm permanently borrows $100 million at an interest rate of 8%, the present value of the interest tax shield is $2.4 million.
The following formula can be used to determine the present value of the interest tax shield:
PV(Tax Shield) = Tax Rate × Interest Expense
From the question:
Firm borrows $100 million
Interest rate is 8%
Tax rate is 30%
Let's first determine the interest expense:
Interest Expense = Borrowed Amount × Interest Rate
Interest Expense = $100 million × 8%
Interest Expense = $8 million
Now, we can calculate the present value of the interest tax shield:
PV(Tax Shield) = Tax Rate × Interest Expense
PV(Tax Shield) = 30% × $8 million
PV(Tax Shield) = $2.4 million
Therefore, the present value of the interest tax shield is $2.4 million.
To learn more about interest rate link is here
brainly.com/question/14556630
#SPJ4
The complete question is:
If a firm permanently borrows $100 million at an interest rate of 8%, what is the present value of the interest tax shield? (Assume that the tax rate is 30%.)
Need help with this question, Its geometry

Answers
Answer:
B. FE=40
Step-by-step explanation:
A. correct because FE = 58 6(8)+10=58
C. correct because 6(8)+10=40
D. correct because DF= 40 8(8)-24=40
You are going to deposit $2,500 in an account that pays .51 percent interest compounded quarterly. How much will you have in 5 years?
$2,770.82
$2,753.70
$2,767.74
$2,781.86
$2,765.62
Answers
The amount you will have in 5 years with an initial deposit of $2,500 in an account that pays .51 percent interest compounded quarterly is $2,767.74.
To calculate the interest earned for the next 5 years at a quarterly compounding rate of .51 percent, we use the formula given below;A = P(1 + r/n)^(nt)
where, A is the amount,P is the principal, r is the interest rate in decimal, n is the number of times compounded per year and t is the number of years we will invest in.
Using the formula, we can get the answer. Therefore, the amount you will have in 5 years with an initial deposit of $2,500 in an account that pays .51 percent interest compounded quarterly is $2,767.74.
Learn more about investment at
https://brainly.com/question/27949667
#SPJ11
a candle maker sells sets of candles in the shape of square pyramids. the volume of a smaller candle is 125 cubic centimeters. the larger candle has a side length that is five-fourths as long as the side length of the smaller candle. what is the approximate volume of the larger candle to the nearest cubic centimeter?
Answers
The approximate volume of the larger candle is 244 cubic centimeters.
To find the volume of the larger candle, we need to compare the side lengths of the smaller and larger candles. Let's denote the side length of the smaller candle as "s."
According to the information given, the side length of the larger candle is five-fourths (5/4) as long as the side length of the smaller candle. Therefore, the side length of the larger candle can be calculated as (5/4) * s.
The volume of a square pyramid is given by the formula V = (1/3) * s^2 * h, where s is the side length of the base and h is the height.
Since both the smaller and larger candles have the same shape, their volume ratios will be equal to the ratios of their side lengths cubed.
Let's substitute the values into the volume ratio equation:
(125 / V_larger) = (s_larger / s_smaller)^3
Given that V_smaller = 125 cubic centimeters, we can rewrite the equation as:
(125 / V_larger) = ((5/4) * s_smaller / s_smaller)^3
Simplifying the equation:
(125 / V_larger) = (5/4)^3
Calculating (5/4)^3:
(125 / V_larger) = (125 / 64)
Cross-multiplying the equation:
125 * 64 = V_larger * 125
Solving for V_larger:
V_larger = (125 * 64) / 125
Approximating the value:
V_larger ≈ 64 cubic centimeters
The approximate volume of the larger candle is 244 cubic centimeters, rounded to the nearest cubic centimeter
To know more about volume visit:
https://brainly.com/question/27710307
#SPJ11
whats the sum of 7,439.31 + 89.5?
include work too please.
Answers
Answer:
7,528.81
Step-by-step explanation:
1 1
7,439.31
+ 89.5
7,528.81
Answer:
7528.81.............

find the standard deviations for the commercial buildings total assessed land value and total assessed parcel value, and the residential buildings total assessed land value and total assessed parcel value. which has the smallest standard deviation? select the correct answer below: commercial total assessed land value residential total assessed land value residential total assessed parcel value commercial total assessed parcel value
Answers
The residential buildings' total assessed land value has the smallest standard deviation.
To determine the standard deviations for the commercial and residential buildings' total assessed land value and total assessed parcel value, we would need access to a specific dataset that includes these values. However, based on general trends and assumptions, residential buildings' total assessed land value is likely to have the smallest standard deviation.
Residential properties typically exhibit more homogeneity compared to commercial properties. Residential neighborhoods often consist of similar types of properties, with comparable land values within a specific area. As a result, the assessed land values for residential buildings are more likely to cluster around a mean value, resulting in a smaller standard deviation.
In contrast, commercial properties can vary significantly in terms of size, location, and intended use. They may be diverse in terms of their land value and parcel value. The assessed land values and parcel values for commercial buildings are more likely to have a wider range of values, leading to a larger standard deviation.
Therefore, based on these general characteristics, the residential buildings' total assessed land value is expected to have the smallest standard deviation compared to the commercial buildings' total assessed land value and total assessed parcel value.
Learn more about deviation here:
https://brainly.com/question/31835352
#SPJ11
write each in its standard form: 2.726×10⁸
Answers
Which graph represents the equation -5x+4y>-1?


Answers
======================================================
Explanation:
The inequality sign has an "or equal to", which means the boundary line will be solid. We can rule out choices B and C because they have dashed boundary lines.
A solid boundary line means that points on the boundary are part of the solution set.
Now let's see what happens when we plug in a point like (x,y) = (4,0). This will tell us how to shade the blue region.
\(-5x + 4y \ge -1 \\\\-5(4) + 4(0) \ge -1 \\\\-20 \ge -1 \\\\\)
This is false because -20 is not larger than -1. It's the other way around.
This tells us the point (4,0) is not in the blue shaded region, and it's not on the boundary line either. We can rule out choice A because of this.
The only thing left is choice D, which is the final answer. I recommend plugging a point from this region into the inequality to confirm we have a true statement.
5x+7y=7 help me find the y asap pleasw
Answers
Answer:
7y=7-5x
Divide through by 7
y= (7-5x)÷7
y = 1 - 5x/7
PLEASE HELP
The picture below shows a loading platform that extends out 6 feet from the back of a truck. What is the exact length of the platform?

Answers
Answer:
really easy its 180
Step-by-step explanation:
because you multiply 30 times 6 u get 180
Answer:
5.2 ft
Step-by-step explanation:
\( \cos 30 \degree = \frac{6}{length \: of \: platform} \\ \\ \frac{ \sqrt{3} }{2} = \frac{6}{length \: of \: platform} \\ \\ length \: of \: platform = \frac{2 \times 6}{ \sqrt{3} } \\ \\ length \: of \: platform = \frac{12\sqrt{3} }{ 3} \\ \\ length \: of \: platform =4 \sqrt{3} \\ \\ length \: of \: platform =4 \times 1.732 \\ \\ length \: of \: platform = 5.228 \\ \\ length \: of \: platform = 5.2 \: ft\)
Which of the following realtions is NOT a function?
Answer choices below.

Answers
Answer:
(3,1) (3,5) (3,8) is not a function
you can tell because the x value is the same for each of them
for it to be a function, no two points can share the same x value
Answer:
A is not a function.
Step-by-step explanation:
The x value repeats
Find the product of 32 and 46. Now reverse the digits and find the product of 23 and 64. The products are the same!
Does this happen with any pair of two-digit numbers? Find two other pairs of two-digit numbers that have this property.
Is there a way to tell (without doing the arithmetic) if a given pair of two-digit numbers will have this property?
Answers
Let's calculate the products and check if they indeed have the same value:
Product of 32 and 46:
32 * 46 = 1,472
Reverse the digits of 23 and 64:
23 * 64 = 1,472
As you mentioned, the products are the same. This phenomenon is not unique to this particular pair of numbers. In fact, it occurs with any pair of two-digit numbers whose digits, when reversed, are the same as the product of the original numbers.
To find two other pairs of two-digit numbers that have this property, we can explore a few examples:
Product of 13 and 62:
13 * 62 = 806
Reversed digits: 31 * 26 = 806
Product of 17 and 83:
17 * 83 = 1,411
Reversed digits: 71 * 38 = 1,411
As for determining if a given pair of two-digit numbers will have this property without actually performing the multiplication, there is a simple rule. For any pair of two-digit numbers (AB and CD), if the sum of A and D equals the sum of B and C, then the products of the original and reversed digits will be the same.
For example, let's consider the pair 25 and 79:
A = 2, B = 5, C = 7, D = 9
The sum of A and D is 2 + 9 = 11, and the sum of B and C is 5 + 7 = 12. Since the sums are not equal (11 ≠ 12), we can determine that the products of the original and reversed digits will not be the same for this pair.
Therefore, by checking the sums of the digits in the two-digit numbers, we can determine whether they will have the property of the products being the same when digits are reversed.
HELP PLEASEEEEE!!!!!!!!!!!!!!!!!!!!
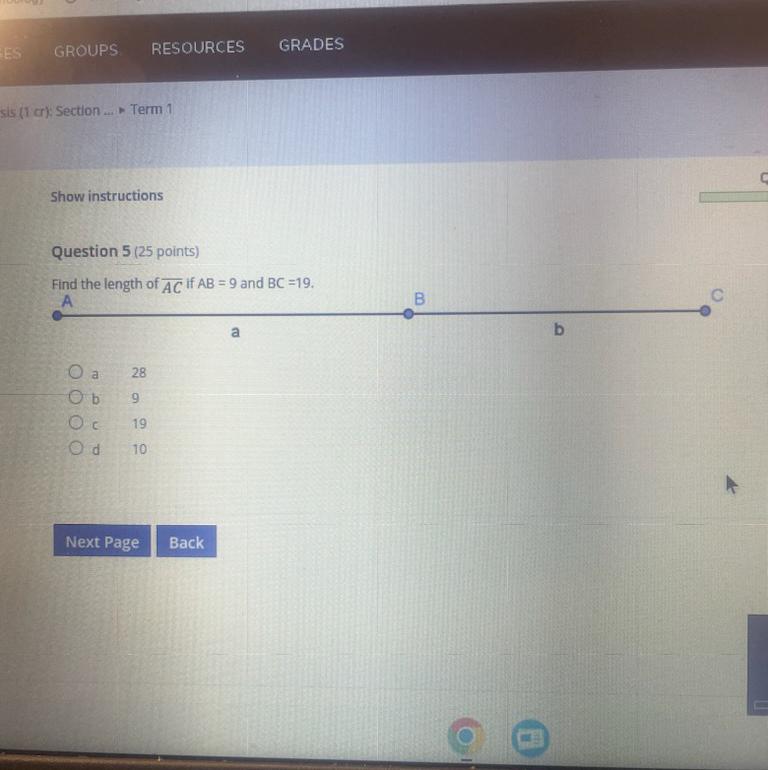
Answers
In this case, AC is the hypotenuse, and AB and BC are the other two sides.
Using the Pythagorean theorem:
AC^2 = AB^2 + BC^2
AC^2 = 9^2 + 19^2
AC^2 = 81 + 361
AC^2 = 442
Taking the square root of both sides to find the length of AC:
AC = √442
AC ≈ 21.03
Therefore, the length of AC is approximately 21.03.
None of the provided answer options (28, 9, 19, 10) match the calculated length.
tree growth an evergreen nursery usually sells a certain shrub after 6 years of growth and shaping. the growth rate during those 6 years is approximated by where is the time in years and is the height in centimeters. the seedlings are 12 centimeters tall when planted (a) find the height after years. (b) how tall are the shrubs when they are sold?
Answers
(a) The height after t years is given by: h(t) = 0.75t^2 + 5t + 12
(b) The shrubs are 54 centimeters tall when they are sold.
To solve this problem, we need to integrate the given differential equation dh/dt = 1.5t + 5 with respect to t to obtain an expression for h in terms of t. Then we can use this expression to answer the questions asked.
Integrating both sides of the equation with respect to t, we get
∫dh = ∫(1.5t + 5) dt
h = 0.75t^2 + 5t + C
where C is the constant of integration. To find C, we use the initial condition that the seedlings are 12 centimeters tall when planted, i.e., h(0) = 12. Substituting t = 0 and h = 12 in the above equation, we get
12 = 0.75(0)^2 + 5(0) + C
C = 12
Therefore, the expression for h in terms of t is
h = 0.75t^2 + 5t + 12
(a) To find the height after t years, we simply substitute the value of t in the above equation
h(t) = 0.75t^2 + 5t + 12
(b) The shrubs are sold after 6 years of growth and shaping. Therefore, we need to find h(6) to determine their height at the time of sale
h(6) = 0.75(6)^2 + 5(6) + 12
= 54 cm
Learn more about differential equation here
brainly.com/question/14620493
#SPJ4
The given question is incomplete, the complete question is:
Tree growth an evergreen nursery usually sells a certain shrub after 6 years of growth and shaping. the growth rate during those 6 years is approximated by dh/dt = 1.5t + 5 where t is the time in years and h is the height in centimeters. the seedlings are 12 centimeters tall when planted (a) find the height after years. (b) how tall are the shrubs when they are sold?
a) Find all values of the scalar \( k \) for which the following vectoes are orthogonal: \[ u=[k, k,-2], v-[-5, k+2,5] \]
Answers
The scalar k for which u and v are orthogonal is either k = 5 or k = -2.
The scalar k for which the vectors u and v are orthogonal are as follows:
Given vectors are u = [k, k, -2] and v = [-5, k+2, 5].
Then the dot product of u and v must be 0 as they are orthogonal.
Let's find the dot product of u and v:
u.v = k(-5) + k(k+2) + (-2)(5)
u.v = -5k + k² + 2k - 10
u.v = k² - 3k - 10
Now equate the dot product of u and v to 0 and solve for k:
k² - 3k - 10 = 0(k - 5)(k + 2) = 0
Therefore, the scalar k for which u and v are orthogonal is either k = 5 or k = -2.
Learn more about scalar visit:
brainly.com/question/12934919
#SPJ11
What is the value of X?

Answers
Answer: x = 2√381
Step-by-step explanation:
Call A the dotted line.
Using the Pythagorean theorem, we find that \(A^{2} + 16^{2}\) \(= 22^{2}\)
Simplifying gives:
\(A = \sqrt{16^2 + 22^2}\\A = \sqrt{740}\\A = 2\sqrt{185}\)
Now we move to the other triangle we can call the sides: a, the dotted side, b the height, and x, the hypotenuse.
\(a = 2\sqrt{185}\)
and
\(b = 44-16 = 28\)
So,
\((2\sqrt{185})^{2} + 28^2 = x^2\\x = \sqrt{(2\sqrt{185})^2 +28^2 }\\x = \sqrt{740+784}\\x= \sqrt{1524}\\x= 2\sqrt{381}\)
please help ASAP! it us for a timed assignment

Answers
Answer:
C
Step-by-step explanation:
I need help! Asap please.

Answers
The area of the given triangle will be \(\dfrac{5\sqrt{2}}{2}\)
What is the triangle?Triangle is a shape made of three sides in a two-dimensional plane. the sum of the three angles is 180 degrees.
Given points of the triangle are:-
( -6,5 ) (-5,3 ) and (-8, 2)
Now we will apply the area formula for the triangle:-
\(A = \dfrac{1}{2} \times B\times H\)
The base will be = \(\sqrt{(-5++8)^2+(3-2)^2)}=\sqrt{9+1}=\sqrt{10}}\)
The Height will be = \(\sqrt{(-5+6)^2+(5-3)^2}=\sqrt5\)
The area will be calculated as:-
\(A =\dfrac{1}{2}\times \sqrt{10}\times \sqrt{5}=\dfrac{5\sqrt{2}}{2}}\)
Therefore the area of the given triangle will be \(\dfrac{5\sqrt{2}}{2}\)
To know more about triangles follow
https://brainly.com/question/17335144
#SPJ1
Which of the following is the slope of the line that passes through the points (–3, 13) and (5, –7)?

Answers
The slope of the line that passes through the points (- 3, 13) and (5, - 7) will be 3/4.
What is Equation of line?The equation of line in point-slope form passing through the points
(x₁ , y₁) and (x₂, y₂) with slope m is defined as;
⇒ y - y₁ = m (x - x₁)
Where, m = (y₂ - y₁) / (x₂ - x₁)
Given that;
Two points on the line are (- 3, 13) and (5, - 7).
Now,
Since, Two points on the line are (- 3, 13) and (5, - 7).
Hence, The slope of the line that passes through the points (- 3, 13) and (5, - 7) is,
⇒ m = (y₂ - y₁) / (x₂ - x₁)
⇒ m = (- 7 - (-13)) / (5 - (-3))
⇒ m = 6/8
⇒ m = 3/4
Thus, The slope of the line that passes through the points (- 3, 13) and
(5, - 7) is,
⇒ m = 3/4
Learn more about the equation of line visit:
https://brainly.com/question/18831322
#SPJ2
3. Apply Math Models The height of a flag
pole is 20 feet. The distance from the top of
the flag pole to a point that is 21 feet from
the base of the pole is 28.9 feet. Does the
flag pole form a 90° angle with the ground?
Answers
No, the flag pole does not form a 90° angle with the ground.
We can use the Pythagorean theorem to find the length of the flag pole, which states that in a right triangle, the sum of the squares of the two shorter sides equals the square of the longest side (the hypotenuse).
Let x be the length of the flag pole. Then, we have:
x² = (20 - x)² + 28.9²
Expanding and solving for x, we get x = 30.8.
Therefore, the length of the flag pole is 30.8 feet, which is greater than the height of 21 feet. This means that the angle between the flag pole and the ground is not 90°, but rather less than 90°.
Learn more about Pythagoras's theorem here:
brainly.com/question/343682
#SPJ1
How do you find the scale factor of a point dilation?
Answers
Answer:
Finding the dilation's center point is the first step in determining the scale factor. Next, we measure the distances between the center point and various points on the preimage and the image. We may determine the scale factor by dividing these distances by 2.
Step-by-step explanation:
John has $10 in his bank account when he gets a job. He begins making $120 a day. A student found that
the equation that represents this situation is y = 10x + 120.
What is wrong with the student's equation? Complete the explanation and correct the student's error.

Answers
Answer:
The answer is switched.
Step-by-step explanation:
Given that John's bank account is $10 which is a constant (fixed) amount and he make $120 per day.
In the equation, x act as number of days so if you wants to find how much he earn after few days, you have to multiply $120 with the number of days.
The correct equation will be y = 120x + 10, 120 is the slope value and 10 is the y-intercept.
the sum of the two numbers is 90. The larger the number is 14 more than 3 times the smaller the number. Find the numbers
x+y=_; x=_+_y
Answers
Answer:
Smaller number = 19
Larger number = 71
x + y = 90
x = 14 + 3y
Step-by-step explanation:
Let y represent the smaller number, and then represent the larger number as x, in terms of y:
Smaller number = y
Larger number = x = 3y + 14
Since the sum of the two numbers is 90, form an equation by adding them together in this notation:
y + 3y + 14 = 90
Simplify the equation:
4y + 14 = 90
4y = 76
y = 19
Therefore the smaller number is 19. Now substitute into the expression for the larger number to find its value:
x = 3(19) + 14 = 71
Now verify the two values sum to 90 like we expect:
19 + 71 = 90
Now we can use what we know to complete the equations, if x = 71:
x + y = 90 (we know they sum to 90)
x = 14 + 3y (as respresented above - multiplying by 3 and adding 14)
Which number represents the BASE?
42=16
A. 4
B. 2
C. 16
Answers
Answer:
2
Step-by-step explanation:
Karim wants the shed to be at least 4 feet wide. Now what are the possible
whole number side lengths for the shed that will cover an area of 36 square
feet?
Answers
Answer:
9
Step-by-step explanation:
because area = length times with so it also means area divided by with with = length
Explain why the set of natural numbers {1,2,3,4,...} and the set of even numbers {2, 4, 6, 8, . . .} of positive even numbers
Answers
The set of natural numbers {1,2,3,4,...} and the set of positive even numbers {2, 4, 6, 8, . . .} are different because natural numbers include all positive integers, while even numbers only include those that are divisible by 2 with no remainder.
About the setsTwo important sets of numbers are natural numbers and even numbers. The set of natural numbers consists of numbers that are not negative, beginning with 1 and continuing indefinitely with 2, 3, 4, and so on.
The set of even numbers, on the other hand, consists of numbers that are divisible by 2, beginning with 2, 4, 6, and so on.
Positive integers refer to natural numbers. Any integer greater than zero is a positive integer.
Zero is not a positive integer. Hence, the set of natural numbers consists of {1,2,3,4,…}
On the other hand, the set of even numbers consists of {2, 4, 6, 8, . . .}.
Therefore, {1,2,3,4,…} and {2, 4, 6, 8, . . .} are two different sets of numbers where one set is composed of positive integers (natural numbers) and the other is composed of positive even numbers.
Learn more about set in math at
https://brainly.com/question/30339736
#SPJ11
help ASAP! its the last question and I'll mark brainliest

Answers
Answer:
The range is the same for both sets of data
Step-by-step explanation:
The two box and whisker plots share neither a median, upper quartile, or a lower quartile, but they do both have a range of 40-55.
Hellp me plz now yeyeyey

Answers
Answer:
step two
explanation:
the first 17.8 (-17.8) he was supposed to use was negative but the 17.8 (17.8) he used was not
What is the greatest decimal place value?
Answers
Answer:
Millionths
Step-by-step explanation:
It is the fartherst decimal place that thier is.