A motorcycle 500 feet from your current position is driving toward you at a constant rate of 50 feet per second. The distanced (in feet) of the motorcycle from you
you after t seconds is given by the equation |500 - 50t| - d = 0. At what times is the car 75 feet from you?

Answers
Answer:
Step-by-step explanation:
Step 1: Substitute d for 75
|500-50t| - 75 = 0
Step 2: Move the constant to one side
|500-50t| = 75
Step 3: Separate the equation into two possible cases
500−50t=75
500−50t=−75
Step 4: Solve the equation for t
t = 17/2 = 8.5
t = 23/2 = 11.5
Solution:
D: 8.5 seconds and 11.5 seconds
Related Questions
0.75x+4x-5 step by step
Answers
Answer:
4.75x-5
Step-by-step explanation:
Answer:
4.75x - 5
Step-by-step explanation:
Step 1: Combine Variables
0.75x + 4x = 4.75x
Simplified - 4.75x - 5
Which equation is the inverse of y = 16x² +1?
X
O Y=√16
O Y= 16
-1
0 У.
О У.
y=√x -1
±√√x-1
4
Answers
Answer:
\(x = + - \sqrt{ \frac{y - 1}{16} } \)
Step-by-step explanation:
\(y - 1 = 16 {x}^{2} \\ \frac{y - 1}{16} = {x}^{2} \\ + - \sqrt{ \frac{y - 1}{16} } = {x} \\ x = + - \sqrt{ \frac{y - 1}{16} } \)
In the figure below, m1 = (x+84)° and m2 = 2x°.
Find the angle measures.
Answers
Answer: The angle measures cannot be determined from the information given. The equation m1 = (x+84)° and m2 = 2x° only specify a relationship between two angles, but do not specify specific angle measures. To determine the angle measures, additional information or constraints are needed.
Step-by-step explanation:
In regression analysis, the variable that is being predicted is the a. response, or dependent, variable b. independent variable c. intervening variabled. is usually x
Answers
The correct answer is (a) response, or dependent, variable
In regression analysis, the variable that is being predicted is the response variable, which is also known as the dependent variable. The dependent variable is the variable that is being explained or predicted by the regression model.
The independent variable(s), on the other hand, are the variable(s) that are used to explain or predict the variation in the dependent variable. These variables are also called predictor variables or explanatory variables.
Intervening variables are variables that come between the independent variable(s) and the dependent variable and affect the relationship between them. These variables are also known as mediator variables or intermediate variables.
Therefore, the correct answer is (a) response, or dependent, variable.
To know more about "Regression analysis" refer here:
https://brainly.com/question/28178214#
#SPJ11
Find. What part of 40 is 16?
Answers
Answer:
40%
Step-by-step explanation:
because convert the fraction 16/40 and the answer is 40%
._.
Which of these best describes the location of (9,0) on a coordinate grid? Question 12 options: In Quadrant I In Quadrant II On the x-axis On the y-axis
Help quick!
Answers
Step-by-step explanation:
Quadrant I: Both values are positive.
Quadrant II: x-value is -ve, y-value is +ve.
On the x-axis: y-value is 0.
On the y-axis: x-value is 0.
The point (9, 0) has a y-value of 0.
=> It lies on the x-axis.
to calculate the variable cost per unit using the high-low method, the change in is divided by the change in:______
Answers
To calculate the variable cost per unit using the high-low method, the change in total cost is divided by the change in activity level.
The high-low method is a cost analysis technique used to estimate the variable and fixed components of a mixed cost. It involves using the highest and lowest levels of activity to calculate the variable cost rate and then using this rate to estimate the fixed cost component.
To calculate the variable cost per unit using the high-low method, first, the total cost and activity level are recorded for the highest and lowest levels of activity. Then, the change in total cost is calculated by subtracting the total cost at the lower level of activity from the total cost at the higher level of activity. Similarly, the change in activity level is calculated by subtracting the lower level of activity from the higher level of activity. Finally, the change in total cost is divided by the change in activity level to obtain the variable cost per unit.
It is important to note that the high-low method assumes a linear relationship between cost and activity, which may not always hold true. Therefore, this method should be used with caution and other cost analysis techniques should also be considered.
To learn more about variable visit;
https://brainly.com/question/17344045
#SPJ11
The western starts at 6:35 pm and ends at 9:10 how long is the movie
Answers
hope this helps
Answer: 2 hours and 35 minutes. Aka 2:35
Step-by-step explanation: to find when it starts and ends, subtract 6:35pm by 9:10pm
So 9-6 is 3 and there is a problem when you do 10-35. 35 is bigger which means you have to take away an hour so the 3 becomes a 2 and then you add 60 onto the 10 even though the limit is 60 minutes because it equals an hour. So you now have 2 hours and 70 minutes - 35. Well that is 35 because 35+35 = 70 meaning the movie is 2 hours, 35minutes
Mike had $1,800 in his bank account. Thirty days later, his account balance was $570. Write an integer an integer to represent the average change in his balance each day during his time
Answers
Answer:
The average change in balance each day during his time $41
Step-by-step explanation:
Here, we want to write an integer to represent the average balance change in the 30 days period.
First, let’s find difference between opening and closing balance= (1800-570) = 1230
We shall now divide this by 30 = 1230/30 = $41
Answer:
The average chang in his time is 41
Step-by-step explanation:
HELP!!! very urgent!!
a text book store sold a combined total of 297 physics and biology textbooks in a week. the number of physics textbooks sold was 71 more than the number of biology books sold. how many textbooks of each type were sold?
Answers
Answer:
h=84
Step-by-step explanation:
You need to set up two equations. The first will be math + history = 240, or m + h = 240. and than you have to h = m - 72 and after that you have to use the second equation to replace the "h" in the first equation: m + m - 72 = 240. and than do 2m - 72 = 240 and solve it. and add 72 to both sides 2m=312 than divide both sides by 2 which will be m = 156 which than you do 156+ h and that will give you 240. than subtract 156 from both sides and your Answer would be here=84.
please do number 7. Thank you

Answers
Answer:
search mo po sa goggleStep-by-step explanation:
I hope it's help{2, 3, 8, 19, 46, ...}
Answers
Answer:
111
Step-by-step explanation:
Multiply the number by two and then add the number right before it.
3*2+2=8
8*2+3=19
19*2+8=46
46*2+19=111
and so on.
Use the properties of the natural logarithm to expand each logarithmic expression. Round answers to 3
decimal places, if necessary. a. In(7x) = Preview 5x b. In Preview x + 3 c. In (x 8) = Preview d. 15,000 In(xy4) =
Answers
a. ln(7x) can be expanded as ln(7) + ln(x). b. ln(x + 3) remains as it is, since it cannot be simplified further. c. ln(x^8) can be expanded as 8ln(x). d. 15,000ln(xy^4) can be expanded as ln(x) + 4ln(y) + ln(15,000).
a. To expand the logarithmic expression ln(7x), we can use the property of the natural logarithm that states ln(ab) = ln(a) + ln(b).
Therefore, ln(7x) can be expanded as ln(7) + ln(x).
b. Similarly, the logarithmic expression ln(x + 3) can be expanded using the property ln(ab) = ln(a) + ln(b).
Hence, ln(x + 3) remains as it is since we cannot simplify it further.
c. Expanding the logarithmic expression ln(x^8) can be done using the property ln(a^b) = b * ln(a).
Thus, ln(x^8) becomes 8 * ln(x).
d. Expanding the logarithmic expression 15,000ln(xy^4) can be done by applying the property ln(ab) = ln(a) + ln(b).
Therefore, 15,000ln(xy^4) can be expanded as 15,000[ln(x) + ln(y^4)].
Learn more about expanded here
https://brainly.com/question/17921963
#SPJ11
1. The population average fuel efficiency of U.S. light vehicles for 2005 was 21 mpg. If the standard deviation of the population was 2.9 and the gas ratings were normally distributed, what is the probability that the mean for a random sample of 25 light vehicles is under 20 mpg
Answers
The probability that the mean mpg for a random sample of 25 light vehicles is 0.042341
What is probability?Probability theory, a subfield of mathematics, gauges the likelihood of an occurrence or a claim being true. An event's probability is a number between 0 and 1, where approximately 0 indicates how unlikely the event is to occur and 1 indicates certainty. A probability is a numerical representation of the likelihood or likelihood that a particular event will occur. Alternative ways to express probabilities are as percentages from 0% to 100% or from 0 to 1. the percentage of occurrences in a complete set of equally likely possibilities that result in a certain occurrence compared to the total number of outcomes.
z = (x-μ)/σ/n, where
x is the raw score = 20 mpg
μ is the population mean = 21 mpg
σ is the population standard deviation = 2.9
n = random number of samples \(x < 20\)
\(= z = 20 - 21/2.9/\sqrt{25} \\= z = -1/2.9/5\\= z = -1.72414\\\)
P-value from Z-Table:
\(P(x < 20) = 0.042341\)
To know more about probability visit:
https://brainly.com/question/11234923
#SPJ4
there are $n$ different points on a circle. the number of triangles whose vertices are among the $n$ points is positive, and equal to the number of hexagons whose vertices are among the $n$ points. what is $n?$
Answers
The number of points on circle or the value of 'n' is 9.
From the combination formula, C(n, r) = n!/(r!(n - r)!)
So here total number of points on circle is = n
The number of points needed to form a triangle is = 3.
So number of triangles whose vertices are among those n points is = C(n, 3)
The number of points needed to form a hexagon = 6
So number of hexagon whose vertices are among those n points is = C(n, 6)
According to given information this numbers are equal, so the equation best suited to this situation is,
C(n, 3) = C(n, 6)
n!/(3!(n - 3)!) = n!/(6!(n - 6)!)
[n(n - 1)(n - 2)]/3! = [n(n - 1)(n - 2)(n -3)(n - 4)(n - 5)]/6!
(n - 3)(n - 4)(n - 5) = 6!/3!
(n - 3)(n - 4)(n - 5) = 6*5*4
We can see that both sides form AP. So comparing the both sides,
n - 3 = 6
n = 6 + 3 = 9
Again from n - 4 = 5
n = 5 + 4 = 9
Again from n - 5 = 4
n = 4 + 5 = 9
Hence the number of points is 9.
To know more about combination formula here
https://brainly.com/question/28065038
#SPJ4
Nancy hits golf balls off the practice tee with an initial velocity of 180 ft/sec with four different clubs. How far down the fairway does the ball hit the ground if it comes off the club making the specified angle with the horizontal? (a) 15
(b) 20
(c) 25
(d) 30
Answers
Based on these observations, option (d) 30 seems to be the most reasonable choice for a longer distance down the fairway.
To determine how far down the fairway the ball hits the ground, we need to consider the horizontal range of the golf ball. The range is the horizontal distance traveled by the ball before it hits the ground.
The range can be calculated using the formula:
Range = (Initial Velocity)² * sin(2 * Launch Angle) / g
Where:
Initial Velocity is the initial velocity of the golf ball (180 ft/sec).
Launch Angle is the angle at which the ball is hit off the tee.
g is the acceleration due to gravity (approximately 32.2 ft/sec²).
Since you haven't provided the launch angle for each club, we cannot determine the exact range for each one. However, we can make some general observations based on the given answer choices:
(a) 15: This option implies a lower launch angle, which would likely result in a shorter range.
(b) 20: Similar to option (a), a lower launch angle would generally result in a shorter range.
(c) 25: This option suggests a moderate launch angle, which could correspond to a reasonable range.
(d) 30: A higher launch angle typically leads to a longer range, so this option may result in a greater distance.
Based on these observations, option (d) 30 seems to be the most reasonable choice for a longer distance down the fairway.
However, without specific launch angles for each club, we cannot determine the exact answer.
To learn more about Velocity visit:
brainly.com/question/18084516
#SPJ11
HELP PLEASE PLEASE PLEASE

Answers
Answer:
We'll need 2 points so we'll choose (-6, 0) and (0, -3).
Let's calculate the slope (or 'm') using
Slope or m = (Y2 -Y1) ÷ (X2 -X1)
Slope ('m') = (-3 -0) / (0, - -6) = -3 / 6 = -1/2
Then we calculate the equation using:
(y - y1) = m • (x -x1)
(y - 0) = -1/2 * (x - -6)
y = -1/2 x -3
Source: http://www.1728.org/distance.htm
Step-by-step explanation:
Answer:
x + 2y = - 6Step-by-step explanation:
X-intercept: (-6, 0)
Y-intercept: (0, -3) ⇒ b = -3
Slope: \(m=\dfrac{y_2-y_1}{x_2-x_1}\)
\(m=\dfrac{0+3}{-6+0}=-\dfrac12\)
Slope-intercept form y = mx + b
\(y=-\frac12x-3\) {multiply both sides by 2}
2y = - x - 6 {add x to both sides}
x + 2y = - 6
What is the equation of this line?


Answers
The equation of the line would be y = (-1/4)x.
Option (D) is correct.
What is the slope-intercept form of a line?
The slope-intercept form of a line is a way of representing the equation of a line in the form y = mx + b, where m is the slope of the line and b is the y-intercept. The y-intercept is the point at which the line crosses the y-axis.
From the graph, we can say that the line is passing through the points (-4, 1) and (4, -1)
So slope = -1/4.
And the y-intercept is 0. as the line is passing through the origin.
So by using a slope-intercept form of a line, we can write
y = mx + c
y = (-1/4)x+0
y = -1/4x
Hence, the equation of the line would be y = (-1/4)x.
To learn more about the slope-intercept form of a line, visit:
brainly.com/question/1884491
#SPJ1
if you choose two cards out of a deck of cards, what are the chances one is a red face card and the other is a black non-face card?
Answers
Using the probability, if you choose two cards out of a deck of cards, then the chances one is a red face card and the other is a black non-face card is 1/2.
In the given question,
If you choose two cards out of a deck of cards, then we have to find the chances one is a red face card and the other is a black non-face card.
As we know that in a deck having 52 cards. In which 26 are red and 26 are black.
We have to choose a red face card.
As we know that in a deck have 12 face cards. In which 6 red face cards and 6 black face cards.
So the chance of getting red face card is
P(R)=Total number of red face cards/Total number of cards
P(R)=6/52
We have to choose a black non-face card.
We know that in a deck have 6 black face cards and total black cards are 26. So the non face cards are 20.
So the chance of getting black non-face card is
P(B)=Total number of black non-face cards/Total number of cards
P(B)=20/52
Now the chances of getting one is a red face card and the other is a black non-face card is
P(R or B)=P(R)+P(B)
P(R or B)=6/52+20/52
P(R or B)=(6+20)/52
P(R or B)=26/52
P(R or B)=1/2
Hence, if you choose two cards out of a deck of cards, then the chances one is a red face card and the other is a black non-face card is 1/2.
To learn more about probability link is here
brainly.com/question/11034287
#SPJ4
An object of mass 480 kg is in free fall in a vacuum where there is no air resistance. Determine the acceleration of the object.
Answers
Since the object is in free fall the acceleration of the object is approximately
\(_{}9.81ms^{-2}\)Solve the differential equation. y ′ +2y=15y= 515 +ce 2x y= 21 +ce −2xy= 215 +e 2 +ce −2 y=15+ce 2x
Answers
It seems there are some errors in the provided equations. Let's go through them one by one and correct them:
Equation 1: y' + 2y = 15
The correct form of this equation is:
y' + 2y = 15
Equation 2: y = 515 + ce^(2x)
It seems there is an extra "=" sign. The correct form is:
y = 515e^(2x) + ce^(2x)
Equation 3: y = 21 + ce^(-2x)
Similarly, there is an extra "=" sign. The correct form is:
y = 21e^(-2x) + ce^(-2x)
Equation 4: y = 215 + e^(2) + ce^(-2)
It seems there is an incorrect placement of "+" sign. The correct form is:
y = 215 + e^(2x) + ce^(-2x) Equation 5: y = 15 + ce^(2x)
There is an extra "=" sign. The correct form is:
y = 15e^(2x) + ce^(2x)
If you would like to solve any particular equation, please let me know.
Learn more about equations here
https://brainly.com/question/14686792
#SPJ11
A principal wishes to implement a decision that has to be a number between 0 and 1; that is, a decision d needs to be implemented where 0 sdS1. The difficulty for the principal is that she does not know what decision is appropriate given the current state of the economy, but she would like to implement a decision that exactly equals what is required given the state of the economy. In other words, if the economy is in state s (where 0 sS 1) the principal would like to implement a decision d s as the principal's utility Up (or loss from the maximum possible profit) is given by Up--s-d With such a utility function, maximising utility really means making the loss as small as possible. For simplicity, the two possible levels of s are 0.4 and 0.7, and each occurs with probability 0.5 There are two division managers A and B who each have their own biases. Manager A always wants a decision of 0.4 to be implemented, and incurs a disutility Ua that is increasing the further from 0.4 the decision d that is actually implement, specifically U-0.4-d.Similarly, Manager B always wants a decision of 0.7 to be implement, and incurs a disutility UB that is (linearly) increasing in the distance between 0.7 and the actually decision that is implemented - that is Ug--10.7 Each manager is completely informed, so that each of them knows exactly what the state of the economy s is (a) The principal can opt to centralise the decision but before making her decision given she does not know what the state of the economy is - she asks for recomm endation s from her two division mana gers. Centralisation means that the principal commits to implement a decision that is the average of the two recommendations she received from her managers. The recommendations are sent simultaneously and cannot be less than 0 or greater than 1 Assume that the state of the economy s = 0.7. What is the report (or recommendation) that Manager A will send if Manager B always truthfully reports s? (b) Again the principal is going to centralise the decision and will ask for a recommendation from both managers, as in the previous question. Now, however assume that both managers strategically make their recommendations. What are the recommendations rA and rB made by the Managers A and B, respectively, in a Nash equilibriunm
Answers
A. Manager A wants the decision to be 0.4, so they would recommend a decision of 0.4 to the principal.
B. The recommendations in the Nash equilibrium would be rA = 0.4 and rB = 0.7.
(a) If Manager B always truthfully reports the state of the economy (s = 0.7), Manager A would send a recommendation that minimizes their disutility Ua. In this case, Manager A wants the decision to be 0.4, so they would recommend a decision of 0.4 to the principal.
(b) In a Nash equilibrium, both managers strategically make their recommendations based on their own utility. Manager A wants to minimize their disutility Ua, which increases as the decision deviates from 0.4. Manager B wants to minimize their disutility UB, which increases as the decision deviates from 0.7.
To find the Nash equilibrium, we need to consider the recommendations made by both managers simultaneously. Let's denote the recommendations as rA (from Manager A) and rB (from Manager B). The principal's decision, d, would be the average of the recommendations, so d = (rA + rB) / 2.
Given that both managers strategically choose their recommendations, they will aim to minimize their disutility. In this case, Manager A would recommend a decision of 0.4 (as it minimizes Ua), and Manager B would recommend a decision of 0.7 (as it minimizes UB). Therefore, the recommendations in the Nash equilibrium would be rA = 0.4 and rB = 0.7.
Learn more about Nash equilibrium from
https://brainly.com/question/34120544
#SPJ11
Function g is a transforamtion of the parent cosine function such that g (x) =3cos(x+2)+1 which paragrsugh representz g?
Answers
The correct option is (C) The graph of Function g is a transformation of the parent cosine function such that g(x) = 3 cos(x + 2) + 1 as it the graph of cosine function.
The ratio between the adjacent side and the hypotenuse is known as the cosine function (or cos function) in triangles. One of the three primary trigonometric functions, cosine is the complement of sine (co+sine) and one of the three main trigonometric functions.
A graph is a structure that resembles a set of objects in mathematics, more specifically in graph theory, in which some pairs of the objects are conceptually "related." The objects are represented by mathematical abstractions known as vertices, and each pair of connected vertices is referred to as an edge.
Option (C) gives a graph of the parent cosine function is transformed into function g such that g(x) = 3 cos(x + 2) + 1 on the cosine function graph.
To know more about graph:
brainly.com/question/17267403
#SPJ4


Answer:
see photo
be sure to look closely, the top curves go to 4 and the bottom goes to -2, there are 2 with this same shape but the other one does not go high enough.
Step-by-step explanation:
Plato/Edmentum

What is the solution for the system
of equations shown IN the graph?

Answers
Answer:
(2,4)
Step-by-step explanation:
The solution for the system is where the two lines intersect.
The two lines intersect at x = 2 and y =4
(2,4)
Answer:
(2, 4)
Step-by-step explanation:
Solutions on graphs are where 2 lines meet on a point.
For this graph, both lines (equations) have a solution of (x=2, y=4).
Picture is included for more detail.

A triangle has two sides of length 1 and 4. What is the largest possible whole-number length
for the third side?
Answers
Using the triangle inequality theorem, the largest possible whole-number length for the third side is 4.
How to Apply the Triangle Inequality Theorem to Find the Length of the Third Side of a Triangle?The third side of a triangle must be shorter than the sum of the other two sides and longer than the difference between the other two sides.
So, for a triangle with sides of length 1, 4, and x (where x is the length of the third side), we have:
1 + 4 > x
4 + x > 1
1 + x > 4
Simplifying these inequalities, we get:
5 > x
x > 3
x > -3 (this inequality is always true)
The largest possible whole-number length for the third side is 4, since it is the largest integer that satisfies the above inequalities.
Learn more about the triangle inequality theorem on:
https://brainly.com/question/309896
#SPJ1
A 12-foot ladder is placed against a wall with a 45-degree angle between the ladder and the ground. Using two different
methods, determine how far away the ladder is from the wall.
Answers
Answer:
24
Step-by-step explanation:
the wall meets the at a 45 degree angle and because of this we can draw a traingle allowing us to use the 45,45,90 method so then we do 12 times 2 and we get 24
PLSSS HELP!! WILL GIVE BRAINLIEST!

Answers
Answer:
Although it's blurry, I believe y is equal to 3.
Step-by-step explanation:
You can see where x (the horizontal line) = 7, then go up to where the diagonal line meets that point, which is at y (the vertical line) = 3.
Answer:
y = \(2 \frac{11}{12}\) gallonsStep-by-step explanation:
The water run at a constant speed with the slope of 5/12.
Slope-intercept form would be y = 5/12x.
So if we were to plug it in the equation,
y = 5/12 (7) = 35/12 or 2 11/12.
Hope this helps ;D
helpppp the whole question is in the picture

Answers
Answer:
y=-1/4(x+3)²+3
Step-by-step explanation:
Answer and explanation:
We khow that the standard form of a parabola is written this way:
ax^2 + bx +c
It can be factored if it has roots
◇◇◇◇◇◇◇◇◇◇◇◇◇◇◇◇◇
In the graph we notice that the parabola has two x-intercepts wich means two roots
Let p and q be the roots
The equation can be written as:
a (x-p) (x-q)
We can khow the value of p and q from the graph
The parabola crosses the x-axis in -6,25 and 0.5
So the equation is:
Y=a(x-0.5) (x+6.25)
a is missing but we can find it
□□□□□□□□□□□□□□□□□□
Replace x and y bu the coordinates of a point in the parabola
Let's take (-3;3)
3= a (3-0.5) (3+ 6.5)
3 =a* 2.5* 9.5
a= 3/(2.5*9.5) = 0.12
So the equation is :
y= 0.12(x-0.5)(x+6.5)
y= (0.12x-0.6)(x+6.5)
y= 0,12x^2 + 0.78x -0.6x- 3.9
y= 0.12x^2 +0.18x-3.9
Divide by 3 to simplify :
y= 0.4x^2+0.6x-1.3
Multiply by 10 to get rid of the decimal numbers
y= 4x^2 + 6x -13
Find the missing length
I WILL GIVE BRAINLIEST
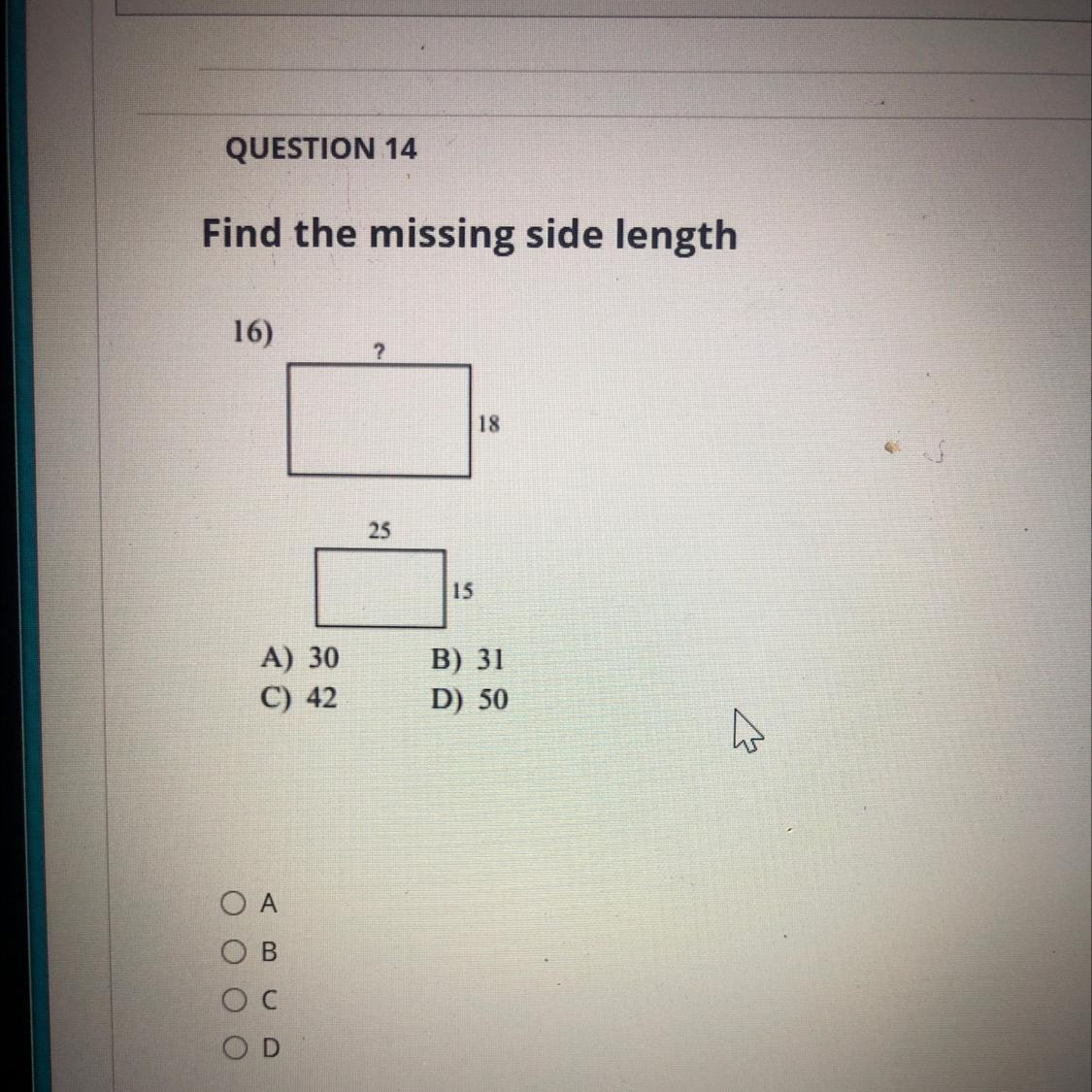
Answers
Answer: 30
Step-by-step explanation: Assuming that the side lengths correspond with one another you can set up a ratio to solve
It should look something like this: 15/25 = 18/x
With this equation set up you can solve by cross multiplying. 25(18)=15x
Then start sloving:
25(18)=15x
450=15x
450/15=15x/15 (divide both sides by 15)
30=x
a gardener uses a total of 61.5 gallons of gasoline in one month. of the total amount of gasoline, was used in his lawn mowers. how many gallons of gasoline did the gardener use in his lawn mowers in the one month? to get credit, you must show all of your work. answers only will be counted as incorrect (whether it is correct or not!) question 4 options:
Answers
The gardener used 40.5 gallons of gasoline in his lawn mowers in the one month.
Let's say the amount of gasoline used in the lawn mowers is x gallons.
Then, the rest of the gasoline (61.5 - x) would have been used for other purposes.
Since the total amount of gasoline used is 61.5 gallons, we can set up an equation:
x + (61.5 - x) = 61.5
Simplifying this equation, we get:
x + 61.5 - x = 61.5
Combining like terms, we get:
61.5 = 61.5
This equation is true, so we know that our assumption that x is the amount of gasoline used in the lawn mowers is correct.
Therefore, the gardener used x = 40.5 gallons of gasoline in his lawn mowers in the one month.
Know more about gasoline here:
https://brainly.com/question/639934
#SPJ11