A merchant sells goods which cost $240 for $299. What percentage is his profit of the cost price?
Answers
Answer:
24.58%
Step-by-step explanation:
Given that,
Initial cost = $240
Final cost = $299
We need to find the percentage in his profit of the cost price.
Profit = Final cost - Initial cost
= $299 - $240
= $59
Percentage,
\(\%=\dfrac{59}{240}\times100\\\\=24.58\%\)
So, the required percentage is equal to 24.58%.
Related Questions
what are the answers? thank you.

Answers
Answer:
176.78cm squared,23.57cm,Step-by-step explanation:
inorder to solve the area of the shaded area;°/360ñRsquared
arc length you use ;°/360ñD
Find the sum or difference. Write your answer in simplest form
3/8 + 1/4
Answers
Answer:
5/8
Step-by-step explanation:
5/8 which is in simplest form
Answer:
5/8
Step-by-step explanation:
3/8+1/4 = 3/8+2/8 = 5/8
Which of the following is/are true? (select all that apply) Invertible matrices are square. If B and C are inverses of a matrix A, then B=C. If A and B are invertible nxn matrices, that AB⊤ is invertible. Every square matrix is invertible. Elementary matrices are invertible.
Answers
The correct statement of the true statement is (A) an invertible matrix is a square matrix with an inverse.
What are Invertible matrices?A square matrix is said to be invertible if the identity matrix results from multiplying the matrix by its inverse.
An identity matrix is a matrix with a major diagonal made up entirely of 1s and all other values being 0s.
A square matrix with an inverse is known as an invertible matrix.
If and only if the determinant is not zero, we can say that a square matrix is invertible.
To put it another way, a 2 x 2 matrix can only be inverted if it has a determinant other than 0.
A non-square matrix (an m-by-n matrix where m n) lacks an inverse.
Therefore, the correct statement of the true statement is (A) an invertible matrix is a square matrix with an inverse.
Know more about Invertible matrices here:
https://brainly.com/question/30651670
#SPJ1
Complete question:
Which of the following is/are true? (select all that apply)
a. Invertible matrices are square.
b. If B and C are inverses of a matrix A, then B=C.
c. if A and B are invertible nxn matrices, that AB⊤ is invertible.
d. Every square matrix is invertible.
e. Elementary matrices are invertible.
What is the M.A.D. (mean absolute deviation) of the following data set?
8 9 9 7 8 6 9 8
Answers
The mean absolute deviation is 0.75
How to determine the mean absolute deviationTo calculate the mean absolute deviation (M.A.D.), you need to find the average of the absolute differences between each data point and the mean of the data set
From the information given, we have that the data set is;
8 9 9 7 8 6 9 8
Let's calculate the mean, we get;
Mean = (8 + 9 + 9 + 7 + 8 + 6 + 9 + 8) / 8
Mean = 64 / 8
Divide the values
Mean = 8
Let's determine the absolute difference, we get;
Absolute differences=
|8 - 8| = 0
|9 - 8| = 1
|9 - 8| = 1
|7 - 8| = 1
|8 - 8| = 0
|6 - 8| = 2
|9 - 8| = 1
|8 - 8| = 0
Find the mean of the absolute differences:
Average of absolute differences = (0 + 1 + 1 + 1 + 0 + 2 + 1 + 0) / 8
Absolute difference = 6 / 8 = 0.75
Learn more about mean absolute deviation at: https://brainly.com/question/447169
#SPJ1
Find the principal needed now to get the given amount; that is, find the present value.
To get $600 after 4 years at 7% compounded monthly
Answers
The required present value needed now to get $700 after 4 years at 11% compounded monthly is $451.73.
To find the present value of $600 after 4 years at 7% compounded monthly, we can use the formula for compound interest:
Compound Interest =P(1+r/n)^rt
Substituting the given values, we get:
Compound Interest =600 (1+7/n)^4
Simplifying this equation, we get
P = 600 / 1.487
P = 451.73
Therefore, the present value needed now to get $600 after 4 years at 7% compounded monthly is $451.73.
Learn more about present value here:
brainly.com/question/17322936s
#SPJ1
The quotient of a number and-3 is 13. What is the equation and number?
Answers
Answer:
-39
Step-by-step explanation:
you do-3 times 13 and you get -39. -39/-3 is 13
A division is a process of splitting a specific amount into equal parts. The quotient of a number and-3 is 13, then the number is -39.
What is Division?A division is a process of splitting a specific amount into equal parts.
Division has different parts like, divisor, dividend, remainder and Quotient.
The quotient is the answer of division.
The number we obtain when we divide one number by another is the quotient.
Let the number be x.
So x/-3=13
Use cross multiplication
x=-39
Hence x/-3=13 is the equation and number is -39.
To learn more on Division click:
https://brainly.com/question/21416852
#SPJ2
Select the correct answer.
Which expression in factored form is equivalent to this expression?
4(x2 – 2x) – 2(x2 – 3)
A. (2x − 3)(x + 1)
B. 2(x + 1)(x + 3)
C. (2x + 3)(x + 1)
D. 2(x - 1)(x – 3)
Took the test. This is the answer.
Answers
Answer:
D. 2(x - 1)(x – 3)
Step-by-step explanation:
4(x2 – 2x) – 2(x2 – 3)
We would first of all expand the expression given
This becomes
= 4x2 - 8x - 2x2 + 6
Rearrange to enable us simplify
4x2 - 2x2 - 8x + 6
= 2x2 - 8x + 6
Factorize
2 (x2 - 4x + 3)
factorizing further using the factors of 3 that add up to -4
2(x2 - x - 3x + 3)
pick out the common factors
2(x(x-1) -3(x-1)
2(x-1)(x-3)
Option D. 2(x - 1)(x – 3) is right
Using the Factor Theorem, the factored form equivalent to this expression is:
\(2(x - 1)(x - 3)\), given by option D.
The Factor Theorem states that a polynomial function with roots \(x_1, x_2, \codts, x_n\) is given by:
\(f(x) = a(x - x_1)(x - x_2) \cdots (x - x_n)\)
In which a is the leading coefficient.In this problem, the expression is:
\(4(x^2 - 2x) - 2(x^2 - 3) = 0\)
\(4x^2 - 8x - 2x^2 + 6 = 0\)
\(2x^2 - 8x + 6 = 0\)
Which is a quadratic equation with coefficients \(a = 2, b = -8, c = 6\).
Hence:
\(\Delta = b^2 - 4ac = (-8)^2 - 4(2)(6) = 16\)
\(x_1 = \frac{-b + \sqrt{\Delta}}{2a} = \frac{8 + 4}{4} = 3\)
\(x_2 = \frac{-b - \sqrt{\Delta}}{2a} = \frac{8 - 4}{4} = 1\)
Hence, the expression is:
\(2(x - 1)(x - 3)\), given by option D.
You can learn more about the Factor Theorem at https://brainly.com/question/24380382
product of x-1 divided by 2x^2+x-3
Answers
Answer:
Images has the answers
Step-by-step explanation:
https://www.wolframalpha.com/input/?i=%28X-1%29%2F%282x%5E2%2Bx-3%29



Determine the values of x in the equation x2 = 64.
Answers
Explain= When a variable(the letter next to the number in this case the “x”) is placed next two a coefficient(the number next to a variable, in this case “2”), that means the variable is being multiplied by that number. To solve for x, we must divide the sum of x2 (64) by the coefficient. The number will equal x.
x2=64? X = 32!
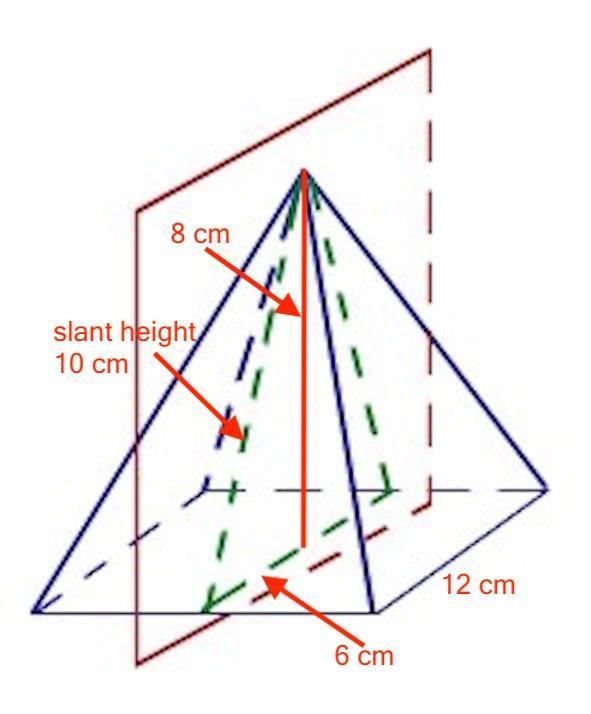
help I'm confused there are many formulas, and do not know which one is right

Answers
9514 1404 393
Answer:
384 cm²
Step-by-step explanation:
It is always a good idea to take a look at what you have and what you are being asked before you go launching into formulas. Then you can pick the formula(s) appropriate to your needs.
__
Your pyramid has a square base, so four (4) triangular faces. The area being asked for is the total of the areas of the square base and those triangular faces.
The formula for the area of a square is ...
A = s² . . . . . where s represents the side length
Here, the side of the square is 12 cm, so the area of the square base will be ...
A = (12 cm)² = 144 cm²
__
The formula for the area of a triangle is ...
A = 1/2bh . . . . . where b is the base length and h is the altitude
In this problem, you're not given the altitude of the triangular face (also called the "slant height" of the triangle). Instead, you're given the height of the pyramid. So, you have to determine the slant height.
Consider the cross section you get when you cut the pyramid with a vertical plane through the peak and the middle of opposite sides. It will be an isosceles triangle with a base equal to the side length of the pyramid, and side lengths equal to the slant height of the pyramid face. See the attachment for an idea of what this looks like.
In order to determine the length of the side of that cross-section triangle, draw its altitude. That is the 8 cm height of the pyramid. Then use the Pythagorean theorem to find the hypotenuse of the right triangle with legs 6 cm and 8 cm:
slant height = √(8² +6²) = √100 = 10 . . . . cm
Now, we can find the area of one of the four triangular faces:
A = (1/2)(12 cm)(10 cm) = 60 cm²
__
The surface area of the pyramid is then ...
surface area = base area + 4 × triangular face area
surface area = 144 cm² + 4 × 60 cm² = (144 +240) cm²
surface area = 384 cm²
These box plote show daily low temperatures for a sample of days in two
different towns
Town A
15 20
30
40 45
H
Town B
15 20 25 30
58
H
0
5 10 15 20 25 30 35 40 45 50 55 60
Degrees (F)
Which statement is the most appropriate comparison of the centers?
O A. The median temperature for both towns is 30%.
OB. The median for town A, 301, is greater than the median for town B.
25
C. The median temperature for both towns is 20"
D. The mean for town A, 30%, is greater than the mean for town B, 25.
Answers
Answer:
D. The mean for town A, 30%, is greater than the mean for town B, 25.
Step-by-step explanation:
From the boxplot Given ; the median value which is the value at the point in between the box ;
Th median value of Town A is 30 While the median for town B = 25
Evaluating the statements given, the most appropriate statement for the comparison made is :
The mean for town A, 30%, is greater than the mean for town B, 25 ; this is true because ; 30 > 25

Triangle xyz is reflected across the y axis and then dilated which describes the dilation used to create triangle x’y’z?

Answers
Answer:
D2/3
Step-by-step explanation:
Answer: D2
Step-by-step explanation:
Algebra 1, please help,

Answers
Answer:
I don’t even know
Step-by-step explanation:
Suppose that there are two types of tickets to a show: advance and same-day. Advance tickets cost $30 and same-day tickets cost $25. For
there were 60 tickets sold in all, and the total amount paid for them was $ 1625. How many tickets of each type were sold?
performance,
X
Number of advance tickets sold:
Number of same-day tickets sold: I
6
?
Answers
The number of advance tickets sold is 25 and same day tickets sold is 30.
Data Given;
Advance Ticket = $30Same-day Ticket = $25Total Ticket = $60Total Cost of Ticket = $1625System of EquationsLet the number of advance ticket be represented by x
Let the number of same-day ticket be represented by y
\(x + y = 60\\ 30x + 25y = 1625\)
From the system of equations above, we can easily pick any to start our calculation.
Using equation (i),
x + y = 60
x = 60 - y ...equation(iii)
Put equation (iii) into equation (ii)
\(30x + 25y = 1625\\ x = 60 - y\\ 30(60 - y) + 25y = 1625\\ 1800 - 30y + 25y = 1625\\ 1800 -5y = 1625\\ 5y = 1800 - 1625\\ 5y = 175\\ 5y/5 = 175/5\\ y = 35\)
Put the value of y into equation (i)
\(x+y = 60\\ x + 35 = 60\\ x = 60 - 35\\ x = 25\)
From the calculation above, the number of advance tickets sold is 25 and same-day tickets is 30.
Learn more on system of equations here;
https://brainly.com/question/13729904
Write an exponential function in the form y=ab^x that goes through the points (0,14) and (6,869)
Answers
Answer:
y= 1/2 (2)x
Step-by-step explanation:
:D I hope this is right! sorry if its not ; )
22. which month did the business make the most profit
23. which month did the business make the least profit

Answers
Answer:
JulyMarchis your answer. I HOPE YOUR DAY GOES GREAT.
HELP QUICK PLZ
The cost to purchase a song from iTunes is $0.99 per song. To purchase from Napster, you must be a member. The Napster membership fee is $10, in addition to $0.89 per song. How many songs would need to be downloaded for the monthly price of Napster to be the same as iTunes?
Answers
Answer:
It will take
100 songs for the monthly price of Napster to cost the same as iTunes.
HELP PLEASE URGENT!!!
A Ferris wheel is 50 meters in diameter and boarded from a platform that is 4 meters above the ground. The six o'clock position on the Ferris wheel is level with the loading platform. The wheel completes 1 full revolution in 2 minutes. How many minutes of the ride are spent higher than 38 meters above the ground?
answer in minutes.
Answers
The number of minutes spent higher than 38 meters above the ground on the Ferris wheel ride is approximately 1.0918 minutes.
To solve this problem, we need to determine the angular position of the Ferris wheel when it is 38 meters above the ground.
The Ferris wheel has a diameter of 50 meters, which means its radius is half of that, or 25 meters.
When the Ferris wheel is at its highest point, the radius and the height from the ground are aligned, forming a right triangle.
The height of this right triangle is the sum of the radius (25 meters) and the platform height (4 meters), which equals 29 meters.
To find the angle at which the Ferris wheel is 38 meters above the ground, we can use the inverse sine (arcsine) function.
The formula is:
θ = arcsin(h / r)
where θ is the angle in radians, h is the height above the ground (38 meters), and r is the radius of the Ferris wheel (25 meters).
θ = arcsin(38 / 29) ≈ 1.0918 radians
Now, we know the angle at which the Ferris wheel is 38 meters above the ground.
To calculate the time spent higher than 38 meters, we need to find the fraction of the total revolution that corresponds to this angle.
The Ferris wheel completes one full revolution in 2 minutes, which is equivalent to 2π radians.
Therefore, the fraction of the revolution corresponding to an angle of 1.0918 radians is:
Fraction = θ / (2π) ≈ 1.0918 / (2π)
Finally, we can calculate the time spent higher than 38 meters by multiplying the fraction of the revolution by the total time for one revolution:
Time = Fraction \(\times\) Total time per revolution = (1.0918 / (2π)) \(\times\) 2 minutes
Calculating this expression will give us the answer in minutes.
For similar question on diameter.
https://brainly.com/question/28162977
#SPJ8
Please hurry need help, Answer choices-
A.9
B.-2
C.11
D.3

Answers
The numerical value of x in angle ABD is 9 as angle ABC is divided into two equal halves.
What is the numerical value of x?An angle bisector divided an angle into two equal halves.
From the diagram:
Line BD divides angle ABC into two equal halves.
Angle ABD = ( 3x - 7 ) degrees
Angle DBC = 20 degrees
Since angle ABD and DBC are equal haves;
Angle ABD = Angle DBC
Plug in the values:
( 3x - 7 ) = 20
Solve for x:
3x - 7 = 20
Add 7 to both sides:
3x - 7 + 7 = 20 + 7
3x = 27
x = 27/3
x = 9
Therefore, the value of x is 9.
Option A)9 is the correct answer.
Learn more about angle bisector here: brainly.com/question/28565813
#SPJ1
evaluate f(x)=x/x-5 when x=3
Answers
Answer:
Step-by-step explanation:
3/3-5=3/-2
The mean height of three boys is 150 centimeters. The mean height of two girls is 145.Find the mean height of five students
Answers
A mean is an arithmetic average of a set of observations. The mean height of the five students is 148.
What is Mean?A mean is an arithmetic average of a set of observations. it is given by the formula,
Mean = (Sum of observations)/Number of observations
Given that the mean height of three boys is 150 centimeters. Therefore, the sum of height of the three boys is,
Mean Height of boys = Sum of height of boys / Number of boys
150 = Sum of height of boys/ 3
Sum of height of boys = 150 x 3 = 450
Also, given that the mean height of two girls is 145 centimeters. Therefore, the sum of height of the two girls is,
Mean Height of girl = Sum of height of girls/ Number of girls
145 = Sum of height of girls/ 2
Sum of height of girls = 145 x 2 = 290
Further, the mean height of five students is,
Mean height of five students = Sum of height of students / Number of students
Mean height of five students = (450 + 290)/ 5
Mean height of five students = 740 / 5
= 148
Hence, the mean height of the five students is 148.
Learn more about Mean here:
https://brainly.com/question/16967035
#SPJ1
Why did the troll give us the clues
We had no money in our pockets
He was nice
So we can get confused
Answers
Answer:
lol
Step-by-step explanation:
im laughing in class
Evaluate the given expression when x = 16 and y = 11.
x² - 3y + x
The answer is
Answers
Answer:
(16)2-3(11)+16
32-33+16
-1+16
=15
cos xº [Hint: Change degree into radian] find the derivative from definition
Answers
The derivative of the function cos(xº) in radians is y' = -sin(xπ/180)
Finding the derivative of the functionFrom the question, we have the following function definition that can be used in our computation:
cos(xº)
Changing the degree into radian, we have
cos(xπ/180)
Express as a function
So, we have
y = cos(xπ/180)
When the cosine function is differentiated, we have
y' = -sin(xπ/180)
Hence, the differentiated function is y' = -sin(xπ/180)
Read more about derivative at
https://brainly.com/question/5313449
#SPJ1
What is the reference angle for the angle with the measure 2x/3
Answers
The reference angle for the angle with the measure 2x/3 is 54⁰
How to determine the reference angle?You should understand that a reference angle is the acute angle to a given angle.
The given angle is 2x/3
We should understand that since the angle has the variable x
= x + 2x/3 = 90⁰
Simplifying the fraction to have
(3x+2x)/3 = 90
Cross and multiply we have 5x=270⁰
Making x the subject
x= 270/5
x=54⁰
Therefore, the reference angle with the measure 2x/3 is = 54⁰
Learn more about reference angles on https://brainly.com/question/1603873
#SPJ1
(3w^2 + 7W + 2) + (w^2 + 2W + 8)
Answers
Answer:
4w^2+9w+10
Step-by-step explanation:
(3w^2+7w+10+w^2+2W
Combine like terms
4w^2+7w+10+2w
Combine like terms
4w^2+9w+10
Answer:
4w^2+9w+10
Step-by-step explanation:
Which situation(s) has (have) a net result of zero?
Select ALL that apply.
The temperature was -5 °F and fell 5 degrees.
O Rich owed Peter $3 then spent $3.
A plane reaches an altitude of 15,000 feet then descends
15,000 feet.
D
Combine two atoms each with a charge of negative 2 and one atom
with a charge of positive 4.
D
Stephen deposited $100 in his bank account then bought a $100
coat.
Tameka drove from a city with an elevation of 39 feet to a city with
an elevation of 78 feet.
Answers
The situations that have a net result of zero are:
Rich owed Peter $3 then spent $3.Combine two atoms each with a charge of negative 2 and one atom with a charge of positive 4.Which situation(s) has (have) a net result of zero?The first situation results in a debt of $3 and then payment of $3, which cancels out the debt.
The second situation involves combining three atoms with a total charge of zero (2 atoms with a charge of negative 2, and 1 atom with a charge of positive 4).
Learn more about net result of zero here:https://brainly.com/question/30202590
#SPJ1
there are two hydrogen atoms and one oxygen atom in a water molecule. complete the table, and graph the ordered pairs on the coordinate graph.
Answers
Answer:
______ allows data to be transmitted by the computer in ahu-man-of riendly from
A camera has a list price of $579.99 before tax. If the sales tax rate is 7.25%, find the total cost of the camera with sales tax included. Round your answer to the nearest cent, as necessary
Answers
Answer:
519.99(.0825) = 42.899
519.99 + 42.899 = $562.89
Step-by-step explanation:
Azra has two red pens,one blue pen and one black pen. If she randomly selects two from her desk with replacement. What is the probability that she takes the blue and black pens?
Answers
Answer:
2/4
Step-by-step explanation:
2 red, 1 blue, 1 black = 4 pens
Probability is normally done is fractions so the answer will be something out if 4
Since there are 1 blue and 1 black the answer will be 2/4