A jeepney ride cost 11 pesos for the first 4 kilometers and each additional integer kilometers adds 3.50 pesos to the fare, use a piece-wise function to represent the jeepney fare in terms of the distance f(d) in kilometers.
Answers
Answer:
\(f(d)=\left\{ \begin{matrix} 11 & \text{for} & 0< d\leq 4 \\-3+3.5d & \text{for}& d > 4 \end\)
Step-by-step explanation:
Cost of first 4 kilometers ride = 11 pesos.
So, for the first 4 km, the fair is constant i.e.
for 1 km the fare is 11 pesos,
for 2 km the fair is also 11 pesos,
similarly, for 3 km of 4 km, the fare is 11 pesos.
Hence, for the distance \(0\geq d\geq 4\), the fair function,
\(f(d)=11\cdots(i)\)
After 4 km, there is an increment of $ 3.50 for each kilometer.
So, the fare function up to 5 kilometers,
\(f(d)=11+3.5=14.5\)
So, the fare function up to 6 kilometers, i.e for the distance \(5<d\leq6\),
\(f(d)=11+2\times3.5=18\)
This can be arranged as the fare function up to 6 km,
\(f(d)=11+(6-4)\times3.5=18\)
Similarly, the fare function up to \(d\) kilometer \((n>4)\),
\(f(d)=11+(d-4)\times3.5\)
\(\Rightarrrow f(d)=-3+3.5d\cdots(ii)\)
Hence, from equations (i) and (ii),
\(f(d)=\left\{ \begin{matrix} 11 & \text{for}\; 0< d \leq 4 \\-3+3.5d & \text{for}\; d > 4 \end\)
Related Questions
Use the distributive property to simplify this expression -2x(3x + x-5)
Answers
Answer:
− 8 x ^2 + 10 x
Step-by-step explanation:
8x+10x
18x.
it is due today, please help me. If you just want points get out of my question page...


Answers
To completely solve the problem, Karim should convert the product back into standard decimal notation.
We cannot directly apply the product of powers property because the binomial is not raised to a power itself.
Miscellaneous problem(1) To convert a number from scientific notation to standard decimal notation, we multiply the coefficient (0.0258) by 10 raised to the power of the exponent (-8).
0.0258 × \(10^{-8\) = 0.0258 × 0.00000001
= 0.000000000000258
(2) The product of powers property states that when you raise a term with an exponent to another exponent, you can simplify it by multiplying the exponents.
In the expression \((3z + y)^3\), we have a binomial term (3z + y) raised to the power of 3. This is not a situation where we can directly apply the product of powers property because the binomial is not raised to a power itself.
To simplify the expression, we need to expand it using the binomial theorem or by applying the concept of binomial expansion.
More on powers property can be found here: https://brainly.com/question/2499975
#SPJ1
Find the measure of the indicated angle.
99⁰
96⁰
98⁰
92°
L
120°
K
N
M
64

Answers
Answer:
? = 92°
Step-by-step explanation:
the chord- chord angle ? is half the sum of the measures of the arcs intercepted by the angle and its vertical angle, that is
? = \(\frac{1}{2}\) (LM + AK) = \(\frac{1}{2}\) (120 + 64)° = \(\frac{1}{2}\) × 184° = 92°
What are the zeros of f(x) = x^2 - x - 12?
A. x= -4 and x= 3
B. x=-3 and x = 4
C. x=-2 and x = 6
D. x=-6 and x = 2

Answers
Answer:
B. x = -3 and x = 4
General Formulas and Concepts:
Algebra I
Terms/CoefficientsFactoringSolving quadraticsStep-by-step explanation:
Step 1: Define
Identify
f(x) = x² - x - 12
Step 2: Solve for x
Factor: (x - 4)(x + 3) = 0Solve: x = 4, -3Is 3/4 a repeating decimal
Answers
Answer:
No.
Step-by-step explanation:
3/4 = 0.75
Answer: non-repeating
Step-by-step explanation:
1-7 blanks! Plz help!

Answers
Y-int: (0,-12)
Vertex: (5, 1/2)
AOS: x=5
Max/Min Value: (5, 1/2)
Evaluate |x - y| + 4 if x = -1, y = 3, and z = -4.
Answers
Answer:
8
Step-by-step explanation:
Substitute the values in the expression, we have:
\(\displaystyle{|-1-3|+4}\)
Evaluate:
\(\displaystyle{|-4|+4}\)
Any real numbers in the absolute sign will always be evaluated as positive values. Thus:
\(\displaystyle{|-4|+4 = 4+4}\\\\\displaystyle{=8}\)
Hence, the answer is 8. A quick note that z-value is not used due to lack of z-term in the expression.
what's the answer to
-12(d-5)-29=43
Answers
Answer:
d=-1
Step-by-step explanation:
The answer would be d = -1
please help me with this please i need the amswers for today,

Answers
The coordinates of each points are:
A = (-0.5, -5)
B = (0.8, -1.8)
C = (-2.6, -2.2)
D = (-1.5, -8.9)
E = (2.5, -6.5)
F = (-1.5, 3.1)
G = (-2.4, 4.1)
H = (1.5, 3)
I = (2.7, 1.5)
J = (-1.5, 0)
K = (-6.5, 0)
We have,
The coordinates of each point are in an ordered form.
i.e
(x, y)
x is the x-axis value.
y is the y-axis value.
Thus,
A = (-0.5, -5)
B = (0.8, -1.8)
C = (-2.6, -2.2)
D = (-1.5, -8.9)
E = (2.5, -6.5)
F = (-1.5, 3.1)
G = (-2.4, 4.1)
H = (1.5, 3)
I = (2.7, 1.5)
J = (-1.5, 0)
K = (-6.5, 0)
Learn more about coordinates here:
https://brainly.com/question/13118993
#SPJ1
Please help I’ll mark you as brainliest if correct!!

Answers
Answer:
230 in²
Step-by-step explanation:
14×5=70×3=210
(5×4)÷2=10×2=20
210+20=230
Answer:
It should be 161.5
Step-by-step explanation:
Sorry it’s gonna take long time to explain, but I guess that’s the right answer
Which of the following expressions has the greatest value?

Answers
Answer:
2^5
Step-by-step explanation:
1^10 = 1 (1*1*1*1*1....)
5^2 = 25 (5*5)
2^5 = 32 (2*2*2*2*2)
3^3 = 27 (3*3*3)
Need help with this asap please

Answers
The point - slope form of the equation of line is, \(y-1=\frac{2}{3}x-\frac{2}{3}\).
The slope - intercept form of the line is,\(y=\frac{2}{3} x+\frac{1}{3}\).
What is point - slope form and slope - intercept form of the line?
Point-slope form is represented as y - y 1 = m (x - x 1), where (x 1, y 1) is the supplied point and m is the given slope. The variables "x" and "y" remain.
Y = mx + b, where m is the slope and b is the y-intercept, is the equation's slope-intercept form.
The given points are (-2, -1) and (1, 1).
First to find the slope m of the line by using the given points.
\(m = \frac{y_2-y_1}{x_2 -x_1} = \frac{1-(-1)}{1-(-2)} = \frac{2}{3}\)
Now to find the point slope form of the equation of line.
Consider, m = 2/3 and the point(1, 1)
So,
\(y-y_1=m(x-x_1)\\y-1 = \frac{2}{3}(x-1)\\ y-1=\frac{2}{3} x -\frac{2}{3} \\\)
This is the point slope form of the equation of line satisfying the given condition.
Next, to find slope - intercept form of the line.
Consider,
\(y-1=\frac{2}{3}x-\frac{2}{3}\\ y=\frac{2}{3}x -\frac{2}{3} +1\\ y=\frac{2}{3}x+\frac{1}{3} \\\)
This is the slope - intercept form of the equation of line.
To know more about the point - slope form and slope - intercept form of the line, click on the link
https://brainly.com/question/18989413
#SPJ13
A small town experienced an explosive population increase Originally the town had population 170 within 3 years the town's population increased by 400% what is the town current population
Answers
Answer:
Step-by-step explanation:
We need to first find out how much 400% of 170 is and then add that increase to the original 170 people.
4(170) = 680 and
680 + 170 = 850 people after 3 years.
What is the area of the square that measures 3.1 m on each side
Answers
The area of the square with a side length of 3.1 meters is 9.61 square meters.
To find the area of a square, we need to multiply the length of one side by itself. In this case, the square has a side length of 3.1 m.
Area of a square = side length × side length
Substituting the given side length into the formula:
Area = 3.1 m × 3.1 m
To perform the calculation:
Area = 9.61 m²
It's worth noting that when calculating the area, we are working with squared units. In this case, the side length is in meters, so the area is expressed in square meters (m²). The area represents the amount of space enclosed within the square.
Remember, to find the area of any square, you simply need to multiply the length of one side by itself.
The area of the square with a side length of 3.1 meters is 9.61 square meters.
For more such questions on square
https://brainly.com/question/27307830
#SPJ8
\(2x+5y=19x-2y=-4
Answers
The result of the system of equation is given by x = -18/ 49 and
y = 17/49
The equals sign is a symbol used in fine formulas to denote the equivalency of two expressions.
The veritably primary step in resolving a variable equation is to precisely identify the values of the variables that lead to a equivalency. The equation's results are the values of the unknown variables that satisfy the equivalency, which are frequently appertained to as the variables for which the equation must be answered.
We'll break the equations by elimination system
2x 5y = 1
9x- 2y = - 4
Multiplying the first equation by 2 and the alternate equation by 5 and adding we get
4x 10y = 2
45x- 10y = -20
49x = -18
or, x = -18/ 49
Now substituting the value of x in the equation we get
2x 5y = 1
y = 17/49
Hence the results of the equations is given by
x = -18/ 49 and y = 17/49
To learn more about equations visit:
https://brainly.com/question/16763389
#SPJ1
indicate below whether the equation in the box is true or false.
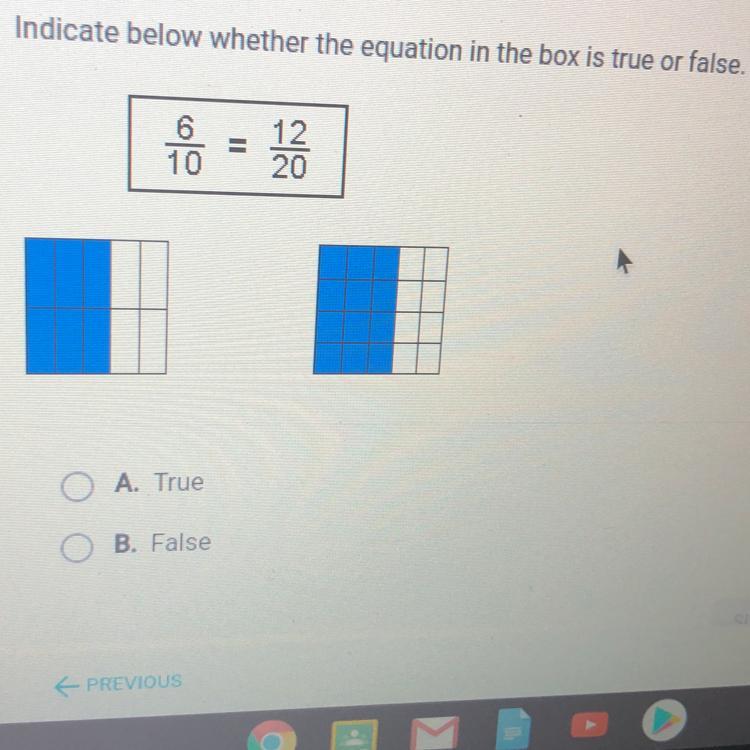
Answers
Answer:
True
Step-by-step explanation:
Multiply the top the same as the bottom
6*2=12
10*2=20
Answer:
The answer is true.
Step-by-step explanation:
6/10 = 12/20 because if you multiply 2 to the numerator and the denominator of 6/10, then the resulting fraction is 12/20. Because 12/20 = 12/20, the answer is true.
whats the equation of a line that passes through point (-1,3) with slope of 1
Answers
The equation of the line that passes through the point (-1, 3) with a slope of 1 is y = x + 4.
To find the equation of a line that passes through the point (-1, 3) with a slope of 1, we can use the point-slope form of a linear equation.
The point-slope form of a linear equation is given by:
y - y1 = m(x - x1)
where (x1, y1) represents the coordinates of a point on the line, and m represents the slope of the line.
Using the given point (-1, 3) and slope 1, we substitute these values into the point-slope form equation:
y - 3 = 1(x - (-1))
Simplifying:
y - 3 = x + 1
Now, we can rewrite the equation in the standard form:
y = x + 4
Therefore, the equation of the line that passes through the point (-1, 3) with a slope of 1 is y = x + 4.
for such more question on line
https://brainly.com/question/1821791
#SPJ8
Brainliest if you get it right :)
Q: Which graph represents a proportional relationship?
I need this by today!

Answers
Answer:
option C
Step-by-step explanation:
I hope now it's helped
Use a system of linear equations with two variables and two equations to solve.
175 students enrolled in a freshman-level chemistry class. By the end of the semester, 4 times the number of students passed as failed. Find the number of students who passed, and the number of students who failed.
Answers
Answer:
35 failed and 140 passed
Step-by-step explanation:
Let p = number who passed
f = number who failed
p+f = 175
4 times the number of students passed as failed, so we need to balance this to set it equal
4f = p
Substitute this into the first equation
4r+f = 175
5f = 175
5f/5 =175/5
f = 35
Now find the number who passed
p = 4f
p = 4*35
p =140
Pls help, ill give brainliest

Answers
Find the values of x and y. (90 - 4y)° (50 - 2x)° (х +5y)° (2x+3y)°

Answers
1) According to the Vertical angle Theorem
We can state that
50-2x =90-4y
-2x +4y=90
2x -4y = -90
In addition to that, since those three angles make up a straight angle:
2x +3y + x +5y +50 -2x = 180º Combine like terms
x+6y =180º
2) Now, we can set this linear system:
x +6y = 180 x-2 To eliminate x
2x -4y = -90
-2x -12y =-360
2x -4y = -90
----------------
-16y = -450
16y= 450
y = 28.12
y ≅ 28
x + 6(28)=180
x+168=180
x=12
Which expressions are equivalent to the one below? Check all that apply.
9x
A. 3. 3x
B. 32.34
O C. 3.32%
D. (3.3)*
E. 3*. 3x
F. 32x
Answers
Answer:
The answer would be A. 3.3x
Step-by-step explanation:
3.3x can be broken down as 3.3.x
3.3 equals 9 and the 'x' is multiplied to 9.
Hence, it equals to 9x.
The expressions are equivalent to the 9ˣ is
3²ˣ = 3ˣ . 3ˣ = (3 . 3)ˣ = 9ˣ
Option C, D and F are correct.
What is Expression?
In mathematics, an expression is a phrase that has at least two numbers or variables and at least one math operation. Addition, subtraction, multiplication, or division are all examples of math operations. An expression's structure is as follows:
(Number/variable, Math Operator, Number/variable) is an expression.
We have to find the Equivalent Expression to
So, we will check each option one by one as
1. 3² * 3ˣ
\(3^{2+x\) ≠ 9ˣ
2. 3 * 3²ˣ
\(3^{1+2x\) ≠ 9ˣ
3. 3²ˣ
= (3²)ˣ
= 9ˣ
4. 3ˣ x 3ˣ
= 3(ˣ⁺ˣ)
= 3²ˣ
= (3²)ˣ
= 9ˣ
5. 3 x 3ˣ ≠ 9ˣ
6. (3 . 3)ˣ
= 9ˣ
Learn more about Equivalent Expression here:
https://brainly.in/question/4185961
#SPJ7
Help me out please please

Answers
Answer:
490000
Step-by-step explanation:
Substituting \(x=40\),
\(I=-425(40)^2 + 45500(40) - 650000=490000\)
Calculate the trade discount rate.Trade discount =Trade discount list price The list price for a boat is $5500. The cost to the retailer is $3850. What is the trade discount rate?
Answers
Given:
The list price for a boat is $5500.
The cost to the retailer is $3850.
Required:
We need to find the trade discount rate.
Explanation:
Consider the trade discount rate formula.
\(Trade\text{ disounct rate=}\frac{Listprice-sellingprice}{List\text{ price}}\times100\)Substitute list price=5500 and selling price =3850 in the formula.
\(Trade\text{ disounct rate=}\frac{5500-3850}{5500}\times100\)\(Trade\text{ disounct rate=}\frac{1650}{5500}\times100\)\(Trade\text{ disounct rate=30 \%}\)Final answer:
\(Trade\text{ disounct rate=30 \%}\)
Out of 300 people how many say fall is favorite season
Answers
Answer:Compare this experimental probability to the population probability that fall is the favorite season. a. Out of 300 people, 4 people would say that fall is their favorite season.
Step-by-step explanation:
Pls send only answers - no work




Answers
Answer:
No
Step-by-step explanation:
If you are just seeking answers, you are basically cheating and trying to get someone to do your work...which will not help you learn the material ....sad.
The question and the triangle are in the image.For part B I just forgot the formula I use to find the length of the segments. If you give me the formula that would be awesome so I can do it by myself. But part C I'll need help with

Answers
Solution:
Given:
Two transversals with four line segments.
\(AC,CE,BD,DF\)Part A:
For the line segment AC, the length is the distance between points A and C.
\(\begin{gathered} A=(5,7) \\ C=(6,4) \\ \text{where;} \\ x_1=5,y_1=7 \\ x_2=6,y_2=4 \\ \\ \text{The distance betwe}en\text{ two points is given by;} \\ d=\sqrt[]{(x_2-x_1)^2+(y_2-y_1)^2} \\ \\ \text{Hence,} \\ d=\sqrt[]{(6-5)^2+(4-7)^2} \\ d=\sqrt[]{1^2+(-3)^2} \\ d=\sqrt[]{1+9} \\ d=\sqrt[]{10} \\ To\text{ the nearest hundredth,} \\ d_{AC}\approx3.16 \end{gathered}\)For the line segment CE, the length is the distance between points C and E.
\(\begin{gathered} C=(6,4) \\ E=(7,1) \\ \text{where;} \\ x_1=6,y_1=4 \\ x_2=7,y_2=1 \\ \\ \text{The distance betwe}en\text{ two points is given by;} \\ d=\sqrt[]{(x_2-x_1)^2+(y_2-y_1)^2} \\ \\ \text{Hence,} \\ d=\sqrt[]{(7-6)^2+(1-4)^2} \\ d=\sqrt[]{1^2+(-3)^2} \\ d=\sqrt[]{1+9} \\ d=\sqrt[]{10} \\ To\text{ the nearest hundredth,} \\ d_{CE}\approx3.16 \end{gathered}\)For the line segment BD, the length is the distance between points B and D.
\(\begin{gathered} B=(17,7) \\ D=(16,4) \\ \text{where;} \\ x_1=17,y_1=7 \\ x_2=16,y_2=4 \\ \\ \text{The distance betwe}en\text{ two points is given by;} \\ d=\sqrt[]{(x_2-x_1)^2+(y_2-y_1)^2} \\ \\ \text{Hence,} \\ d=\sqrt[]{(16-17)^2+(4-7)^2} \\ d=\sqrt[]{(-1)^2+(-3)^2} \\ d=\sqrt[]{1+9} \\ d=\sqrt[]{10} \\ To\text{ the nearest hundredth,} \\ d_{BD}\approx3.16 \end{gathered}\)For the line segment DF, the length is the distance between points D and F.
\(\begin{gathered} D=(16,4) \\ F=(15,1) \\ \text{where;} \\ x_1=16,y_1=4 \\ x_2=15,y_2=1 \\ \\ \text{The distance betwe}en\text{ two points is given by;} \\ d=\sqrt[]{(x_2-x_1)^2+(y_2-y_1)^2} \\ \\ \text{Hence,} \\ d=\sqrt[]{(15-16)^2+(1-4)^2} \\ d=\sqrt[]{(-1)^2+(-3)^2} \\ d=\sqrt[]{1+9} \\ d_{}=\sqrt[]{10} \\ To\text{ the nearest hundredth,} \\ d_{DF}\approx3.16 \end{gathered}\)Part B:
On the first transversal, the ratio of the lengths of the line segments formed on it is;
\(\begin{gathered} \frac{AC}{CE}=\frac{3.16}{3.16} \\ \\ \text{Hence, the ratio is;} \\ AC\colon CE=1\colon1 \end{gathered}\)On the second transversal, the ratio of the lengths of the line segments formed on it is;
\(\begin{gathered} \frac{BD}{DF}=\frac{3.16}{3.16} \\ \\ \text{Hence, the ratio is;} \\ BD\colon DF=1\colon1 \end{gathered}\)From the ratio of the lengths of each transversal, it is noticed that they are the same.
Factor the GCF from the expression 30x-6y.
Answers
Answer:
6(5x-y)
Step-by-step explanation:
i hope this helps :)
What is the simplified answer to (2/3)2?
Answers
The simplified form of the exponent simplified answer to (2/3)² is 4/9.
What is an exponent?An exponent is the number of times that a number is multiplied by itself. It should be noted that the power is an expression which shows the multiplication for the same number. For example, in 6⁴ , 4 is the exponent and 6⁴ is called 6 raise to the power of 4.
The expression can be illustrated thus:
= (2/3)²
= 2/3 × 2/3.
= 4/9
The value is 4/9.
Learn more about exponents on:
brainly.com/question/13669161
#SPJ1
Select a personal or professional example of a measurement you use routinely. Convert the measurement either from U.S customary units to metric units, or from metric units to U.S. customary units. You may choose more than one measurement and may choose among weight, length, temperature, etc. Show each step of your conversion and be sure to include all units from the original and converted measurements (for example, yards to meters, degrees Celsius to degrees Fahrenheit).
Answers
A personal example of a measurement I use routinely is converting weight from U.S. customary units to metric units. Let's convert pounds to kilograms.
To convert pounds to kilograms, we use the conversion factor of 1 pound = 0.453592 kilograms.
For example, if I have a weight of 150 pounds, I can calculate the equivalent weight in kilograms as follows:
150 pounds * 0.453592 kilograms/pound = 68.0388 kilograms
Therefore, 150 pounds is approximately equal to 68.0388 kilograms.
In this conversion, we multiply the weight in pounds by the conversion factor to obtain the weight in kilograms. By using the appropriate conversion factor, we can accurately convert weights from U.S. customary units to metric units.
It's important to note that conversion factors may vary slightly depending on the rounding used and the exact value of the conversion factor.
For such more question on metric units
https://brainly.com/question/30951620
#SPJ8