a car is traveling at 50 mph due south at a point 1/2 mile north of an intersection. another car is traveling at 40 mph due west at a point 1/4 mile east of the same intersection. at what rate is the distance between the two cars changing?
Answers
The distance between the two cars is changing at a rate of 48 mph.
Now, let's think about how the distance between the two cars is changing over time. We can use the Pythagorean theorem to find the distance between the two cars at any given time:
distance² = (change in x)² + (change in y)²
where "distance" is the distance between the two cars, and "change in x" and "change in y" are the distances that the two cars have traveled in the x and y directions, respectively.
Now, we just need to plug in the values we have for the positions and speeds of the two cars.
Plugging these values in, we get:
rate of change of distance = [(-0.25)(-40) + (-1)(-50)] / distance
We still need to find the value of "distance" to complete this calculation. Using the Pythagorean theorem again, we can find that:
distance² = (0.5 + 0.25)² + (-1 - 0)² = 1.5625
So, distance = 1.25 (since distance is positive, we can ignore the negative square root).
Finally, we can plug in the values we have for distance, change in x, and rates of change of x and y, to get:
rate of change of distance = [(-0.25)(-40) + (-1)(-50)] / 1.25 = (10 + 50) / 1.25 = 48 mph
To know more about distance here
https://brainly.com/question/4199102
#SPJ4
Related Questions
a catering company needs 8.75 lb of shrimp for a small party. they buy lb of jumbo shrimp,3 2 3 lb of medium-sized shrimp, and some mini-shrimp. how many pounds of mini-shrimp do they2 5 8 buy?
Answers
Finally, we have \(2\frac{11}{24}\) lbs of mini shrimps.
Given that;
A catering company needs 8.75 lbs of shrimp for a small party. they buy \(3\frac{2}{3}\) lbs of jumbo shrimps, \(2\frac{5}{8}\) lbs of medium-sized shrimps, and some mini-shrimps.
The total of 8.75th is changed as \(8\frac{3}{4}th\\\)
Sum of \(3\frac{2}{3}\) + \(2\frac{5}{8}\) this is bought by the company.
So, to purchase the remaining \(8\frac{3}{4}th\\\) - \(3\frac{2}{3}\) - \(2\frac{5}{8}\)
We use a common denominator of 24 for the fraction parts.
Change \(8\frac{3}{4}\) as \(8\frac{18}{24}\)
Similarly, convert;
\(3\frac{2}{3}\) as \(3\frac{16}{24}\)
\(2\frac{5}{8}\) as \(2\frac{15}{24}\)
By solving all the above conversions, we get;
\(\frac{42-16-15}{24}\) = 11/24
Considering all the factors together we have \(2\frac{11}{24}\) lbs of mini shrimps.
To learn more about denominator click here:
brainly.com/question/7067665
#SPJ4
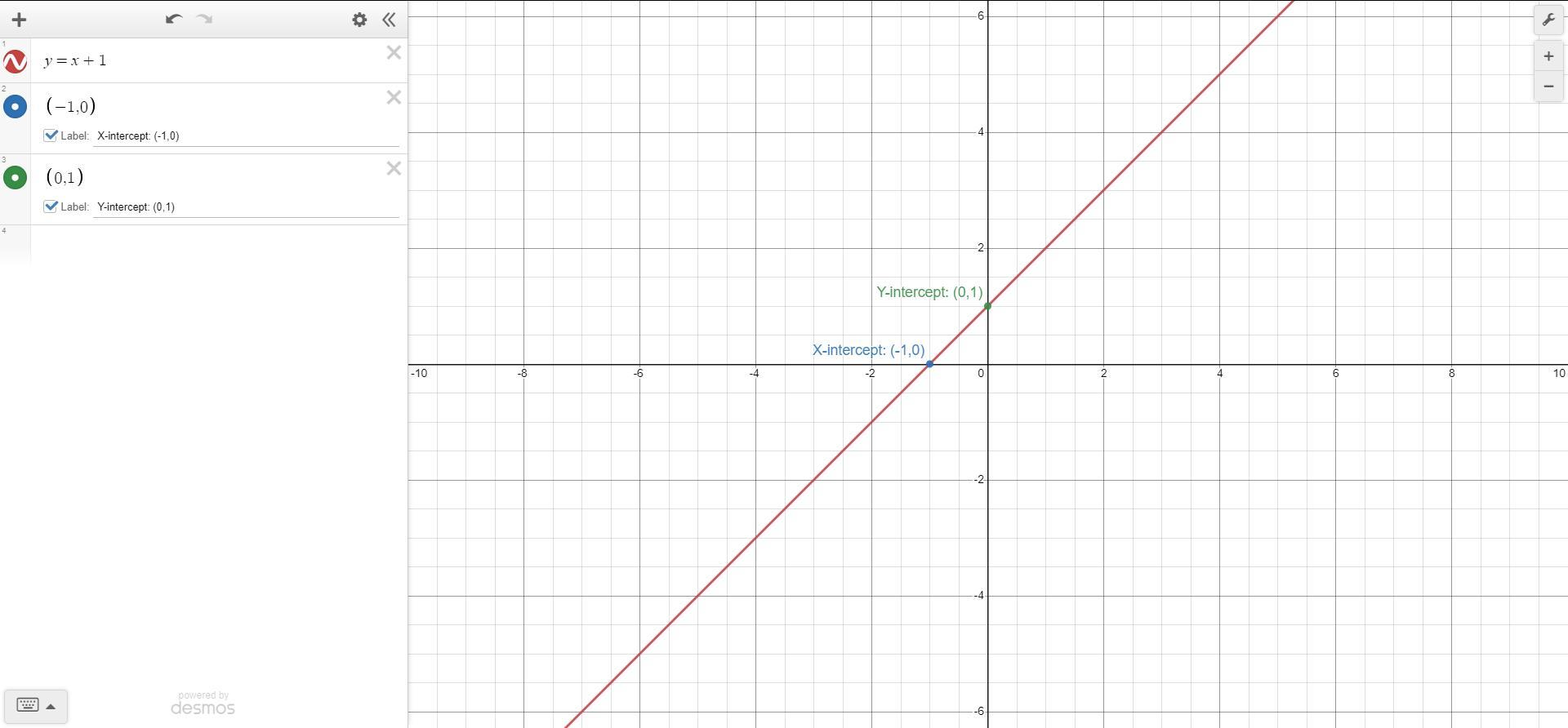
y = x + 1
in graph form
Answers
Answer:
See graph
Step-by-step explanation:
Camden is making accessories for the soccer team. He uses 632.25 inches of fabric on headbands for 41 players and 4 coaches. He also uses 361.21 inches of fabric on wristbands for just the players. How much fabric was used on a headband and wristband for each player?
Answers
Headbands for 41 players and 4 coaches (45)
632.25 inches / 45 = 14.055 inches
Wristbands for 41 players
361.21 / 41 = 8.81 inches
14.055 + 8.81 = 22.86 inches
Camden used 22.86 in of fabric for each player.
What is division?Division is breaking a number up into an equal number of parts.
Given that, Camden uses 632.25 inches of fabric on headbands for 41 players and 4 coaches. He also uses 361.21 inches of fabric on wristbands for just the players.
4 coaches + 41 players = 45 persons
Fabric used for headband for one person = 632.25 / 45 = 14.05 in
Fabric for 41 players = 14.05 × 41 = 576.05 in
Fabric for wristband = 361.25
Total fabric used on players = 361.25+576.05 in = 937.26 in
For each of them = 937.26 in / 41 = 22.86 in
Hence, Camden used 22.86 in of fabric for each player.
For more references on division, click;
https://brainly.com/question/21416852
#SPJ6
For all real numbers , a,b and c, if a>b and c>0, then a-c ___b-c

Answers
-------------------------------------------------------------------------------------------------------------
Answer: \(\textsf{a - c } > \textsf{ b - c}\)
-------------------------------------------------------------------------------------------------------------
Given: \(\textsf{a} > \textsf{b and c} > \textsf{0}\)
Find: \(\textsf{a - c } \_\_\_ \textsf{ b - c}\)
Solution: Since we know that a is greater than b this means that no matter if we subtract or add a constant to both sides a would stay greater than b. Therefore, this would give us the expression of a - c > b - c.
Solve each inequality. Show work.
|0.7x + 5| > 6.7
Answers
The inequality can be simplified into a compound inequality:
x > 2.43
x < -16.7
How to solve the inequality?Here we want to solve the following inequality:
|0.7*x + 5| > 6.7
To solve it, we need to isolate the variable x. The first thing we need to do is "break" the absolute value part, then we will get a compound inequality.
0.7*x + 5 > 6.7
0.7*x + 5 < -6.7
Now we can isolate x in both of these inequalities:
0.7*x > 6.7 - 5
0.7*x < -6.7 - 5
0.7*x > 1.7
0.7*x < -11.7
x > 1.7/0.7
x < -11.7/0.7
x > 2.43
x < -16.7
That is the solution of the inequality.
Learn more about inequalities at:
https://brainly.com/question/24372553
#SPJ1
Jess and Oscar have $200,000 to invest in a savings account that pays 2.875% interest compounded quarterly. How many years will it take for the account to earn $30,000 in interest? Round your answer to the nearest tenth of a year.
Answers
Answer:
Principle = 200,000
Rate = 2.875% aka 0.02875
Method of compounded = quarterly aka 4.
Future interest
200,00 - 200,000(1 + 0.02875/4)^4n > 30,000
200,000(compound) represents the future value
-200,000 allows for interest gained
solve using logarithims
Answer:
It would approximately 4.9 years.
if the cost per unit is to produce x units of plywood. find the average cost of producing 400 units.
Answers
To find the average cost of producing 400 units of plywood, we need information about the cost function. If we have the cost function per unit, we can calculate the total cost of producing 400 units and then divide it by 400 to find the average cost per unit.
Let's assume the cost function for producing x units of plywood is given by C(x), where C(x) represents the cost in dollars. We can then calculate the total cost of producing 400 units as follows:
Total Cost = C(x) * Number of Units
Total Cost = C(400) * 400
Once we have the total cost, we can find the average cost per unit by dividing the total cost by the number of units:
Average Cost per Unit = Total Cost / Number of Units
Average Cost per Unit = (C(400) * 400) / 400
Average Cost per Unit = C(400)
Therefore, to find the average cost of producing 400 units, we need to know the cost function C(x) specifically for producing 400 units of plywood. Without that information, we cannot provide an exact value for the average cost.
Learn more about average cost here:
https://brainly.com/question/29509552
#SPJ11
If the cost per unit is to produce x units of plywood. find the average cost of producing 400 units.
What does it mean for data to have variability as it refers to statistical questions? Give an example
Answers
Answer:
The term, "variability" describes how far apart data points lie from each other, and from the center of a distribution.
Along with measures of central tendency, measures of variability give you descriptive statistics that summarize your data.
Variability is also referred to as spread, scatter or dispersion.
Example:
The heights of students at Brainly varied from 4 feet and 6 inches tall to 6 feet and 3 inches tall. It varies 1 foot and 9 inches.
What I’d the first step? How do I find angle A?

Answers
Answer: charity activity trivia señora Sonia de warrior Gloria Delaware 853
Step-by-step explanation:
help being bullied still and cant focus on the question

Answers
Which ordered pairs show the location of the bleachers check all that apply
Answers
\(\hookrightarrow \:
in the figure below, what is the measure of angle x

Answers
Check the picture below.

Gloria enjoys playing Skee ball — an arcade game where players toss a ball so it rolls up a ramp and falls into one of several slots. Each slot is worth a different number of points based on how difficult it is to land the ball in that slot. Gloria only aims at the most difficult slot, which is worth 100100100 points, but she only has a 10\%10%10, percent chance of landing the ball in that slot. If she misses, her ball will certainly land in the 101010 point slot.
The table below displays the probability distribution of XXX, the number of points Gloria scores on a random shot.
X=points, 100 10
P(X), 10%, 90%,
Given μx=19μ calculate σ X
Answers
Answer:
27
Step-by-step explanation:
that's the answer on khan
Study the coordinate plane below. A certain polygon has its vertices at the following points: (1, 1), (1, 8), (4, 7), and (4, 3) What is the best description of this polygon? A. rectangle B. pentagon C. trapezoid D. parallelogram
Answers
Answer:
pentegon
Step-by-step explanation:
The certain polygon has its vertices at the following points: (1, 1), (1, 8), (4, 7), and (4, 3) is a trapezoid option (C) is correct.
What is a regular polygon?A polygon is a geometric figure with a finite number of sides in two dimensions. On the sides or edges of a polygon, straight-line segments are joined end to end to form a closed shape. The vertices, also known as corners, are the points where two line segments meet and form an angle.
We have coordinates of a polygon, the coordinate plane:
points: (1, 1), (1, 8), (4, 7), and (4, 3)
After, connecting the points to each other.
We will see, after joining the points, the polygon will be a trapezoid.
Thus, the certain polygon has its vertices at the following points: (1, 1), (1, 8), (4, 7), and (4, 3) is a trapezoid option (C) is correct.
Learn more about the regular polygon here:
brainly.com/question/11810316
#SPJ2

Enter the correct answer in the box. What is the standard form of function

Answers
Answer:
f(x) = 4x² + 48x + 149
Step-by-step explanation:
Given
f(x) = 4(x + 6)² + 5 ← expand (x + 6)² using FOIL
= 4(x² + 12x + 36) + 5 ← distribute parenthesis by 4
= 4x² + 48x + 144 + 5 ← collect like terms
= 4x² + 48x + 149 ← in standard form
Answer:
\(f(x)=4x^{2} +149\)
Step-by-step explanation:
Start off by writing the equation out as it is given:
\(f(x)=4(x+6)^{2} +5\)
Then, get handle to exponent and distribution of the 4 outside the parenthesis:
\(f(x)=4(x^{2} +36)+5\\f(x)=4x^{2} +144+5\)
Finally, combine any like terms:
\(f(x)=4x^{2} +149\)
Rewrite -cot^2x/ 1+ cscx so its not in fraction form.
Answers
Rewriting the given trigonometric form using trigonometric identities by eliminating fraction form gives \(-cotx.cosx.(sinx+1)\)
What are trigonometric identities?Trigonometric Identities are equality statements that hold true for all values of the variables in the equation and that use trigonometry functions.
There are numerous distinctive trigonometric identities that relate a triangle's side length and angle. Only the right-angle triangle is consistent with the trigonometric identities.
The six trigonometric ratios serve as the foundation for all trigonometric identities. Sine, cosine, tangent, cosecant, secant, and cotangent are some of their names. The adjacent side, opposite side, and hypotenuse side of the right triangle are used to define each of these trigonometric ratios. The six trigonometric ratios are the source of all fundamental trigonometric identities.
Calculation:We know that,
\(cot^{2} x = \frac{cos^{2}x }{sin^{2}x }\)
\(1+cscx = (1+\frac{1}{sinx} ) = \frac{sinx+1}{sinx}\)
Rewriting the given trigonometric form using trigonometric identities by eliminating the fraction form gives\(-cotx.cosx.(sinx+1)\)
To know more about trigonometric identities, visit:
https://brainly.com/question/24496175
#SPJ1
The midpoint of is M(1, 5). One endpoint is P(–4, 9). Which equations can be used to find the coordinate of endpoint Q(x2, y2)?
Answers
If the midpoint is M(1, 5). and One endpoint is P(–4, 9) the other point is solved to be
point Q = (6, 19)How to find the other pointThe formula to determine the center point of a straight line using the coordinates of its endpoints is known as the midpoint formula in coordinate geometry.
The formula is of the form
X= (x₂ + x₁)/2
Y = (y₂ + y₁)/2
solving for Q (x₂, y₂)
1 = (x₂ + -4)/2
2 = x₂ + -4
x₂ = 6
5 = (y₂ + 9)/2
10 = y₂ + 9
y₂ = 19
point Q = (6, 19)
the other point is solved to be Q (6, 19)
Learn more about midpoint here:
https://brainly.com/question/29730021
#SPJ1
Answer: the answer is C
-4+x2/2 = 1, 9+y2/2 =5
Step-by-step explanation:
there are $7$ dots on a circle. what is the maximal number of intersection points outside the circle created by connecting these points with lines?
Answers
The maximal number of intersection points outside the circle that can be created by connecting the 7 dots on the circle with lines is 21.
To calculate this, we use the formula for the maximum number of intersection points formed by connecting n points with lines:
Max intersection points = n * (n - 1) / 2
In this case, n = 7, as there are 7 dots on the circle. Plugging in this value into the formula:
Max intersection points = 7 * (7 - 1) / 2
Max intersection points = 7 * 6 / 2
Max intersection points = 42 / 2
Max intersection points = 21
Therefore, the maximal number of intersection points outside the circle formed by connecting the 7 dots on the circle with lines is 21.
To learn more about circle here:
https://brainly.com/question/29130134
#SPJ4
One acre of Christmas trees produces the daily oxygen requirement for how many people?
Answers
Answer: 18
Step-by-step explanation:
one way to maintain good credit is to:
Answers
Answer: not spend anything
Step-by-step explanation:
not spend anything :) YOUR WELCOME
what is the value of q in the equation 4/5q = 32
Answers
Answer:
40
Step-by-step explanation:
4/5 * q = 32
First you divide 4/5 by itself so you can eliminate it from the equation and then you have to divide 32 by 4/5 n when you do that you get 40. So q = 40.
criangle has sides 45, 4x and 2x – 4. What is the possible range of x?
Answers
Answer:
\(\frac{49}{6} <x<\frac{41}{2}\)
Step-by-step explanation:
The possible ranges of x are listed above. Hope this helps! :)
Write an equation for a polynomial with all the following features a. The degree is 5 b. The polynomial has 4 terms c. The graph of the polynomial crosses the vertical axis at y=12
Answers
Answer:
f(x) = ax^5 + bx^3 + cx^2 + 12.
Step-by-step explanation:
the degree is 5 so we will have a term like:
ax^5
it crosses the vertical axis at y = 12 so we will have a constant term equal to 12
the polynomial has 4 terms, and we already have two so we can make up two more but the exponent must be between 1 and 4
f(x) = ax^5 + bx^3 + cx^2 + 12.
it has 4 terms, f(0) = 12, then it intersects the y-axis at y = 12, and the maximum exponent is 5, then the degree of f(x) is 5
what is the area of the region on the xy-plane which is bounded
from above by the curve y=e^x, from bellow by y=cosX and on the
right by vertical line x=pi ?
Answers
A = ∫[0, π] (e^x - cos(x)) dx. We can approximate the value of the integral to find the area of the region bounded by the curves y = e^x, y = cos(x), and the vertical line x = π.
To find the area of the region bounded by the curves y = e^x, y = cos(x), and the vertical line x = π, we need to evaluate the definite integral of the difference between the upper and lower curves with respect to x. Let's denote the area as A. The upper curve is y = e^x, and the lower curve is y = cos(x). We need to find the x-values where these curves intersect to determine the limits of integration.
Setting e^x = cos(x), we can solve for x numerically. Using numerical methods or graphing, we find that the intersection occurs approximately at x = 0.739085. To calculate the area, we integrate the difference between the upper and lower curves over the interval [0, π]: A = ∫[0, π] (e^x - cos(x)) dx
Evaluating this integral will give us the area of the region bounded by the curves. However, this integral does not have a closed-form solution and requires numerical methods to approximate the value. Using numerical integration techniques, such as the trapezoidal rule or Simpson's rule, we can approximate the value of the integral to find the area of the region bounded by the curves y = e^x, y = cos(x), and the vertical line x = π.
To learn more about Simpson's rule, click here: brainly.com/question/30459578
#SPJ11
The covariance of Security X's returns and Security Y’s returns is 10, and the variance of Security X's returns is 13% and the variance of Security Y's returns is 17%. The correlation coefficient of the security X and Y returns is closest to:
1 1.83
2 0.67
3 0.25
4 0.05
Answers
The correlation coefficient of the security X and Y returns is closest to 0.67, indicating a moderate positive correlation between the two securities.
The correlation coefficient of the security X and Y returns is closest to 0.67. This can be calculated by dividing the covariance of the returns by the square root of the product of the variances of X and Y.
The correlation coefficient measures the strength and direction of the linear relationship between two variables. It ranges between -1 and 1, with values close to 1 indicating a strong positive correlation, values close to -1 indicating a strong negative correlation, and values close to 0 indicating a weak or no correlation.
In this case, the given covariance is 10, the variance of Security X's returns is 13%, and the variance of Security Y's returns is 17%. To calculate the correlation coefficient, we use the formula:
Correlation coefficient = Covariance / (\(\sqrt{}\)(Variance_X) * \(\sqrt\)(Variance_Y))
Plugging in the values, we get:
Correlation coefficient = 10 / (\(\sqrt\)(13%) * \(\sqrt\)(17%)) ≈ 0.67
Therefore, the closest option is 0.67, indicating a moderately strong positive correlation between the returns of Security X and Security Y.
Learn more about correlation here:
https://brainly.com/question/29978658
#SPJ11
2x 1/2 x3 2/3 im goin to be need a lot of helpppp
Answers
Answer:
3.66666666667
or
3.67(rounded to the nearest tenth)
Step-by-step explanation:
2x 1/2 x3 2/3 = 3.66666666667
Ace Carlos
Developer
Answer:
=3 2/3
Step-by-step explanation:
2 x 1/2 x 3 2/3
2 x 1/2 = 2/2 = 1
1 x 3 2/3
=3 2/3
if the area of the square 64 CM find the side of the square also find the perimeter
Answers
Answer:
Step-by-step explanation:
The area of a square is x²
This means it is x² = 64cm²
x= 8cm <------ One side
Since it is a square, all sides are the same length.
8*4 = 32cm
Side of the square: 8 cm
Perimeter: 32cm
algebra ,, will mark brainliest!!

Answers
Answer:
B
Step-by-step explanation:
(1 1/2,-2)
solve for x and get 1 1/2
solve for y and get -2
Divide:
7,448 + 6 =
submit
PO
Answers
Answer:
ffggggggggggytdcuihgr
48. Determine whether the given measurements determine 0, 1, or 2
possible triangles.
a=19.3, c= 21.9, C = 39.4°
Answers
Answer:39.9
Step-by-step explanation: