Answers
The average of the lengths of the wood at the store can be found to be C. 10.344 Inches.
How to find the average ?To find the average length, we need to add up the lengths of the 8 pieces of wood and then divide by 8.
10 and 1/16 = 10.0625
10 and 1/8 = 10.125
10 and 1/4 = 10.25
10 and 3/8 = 10.375
Adding these four lengths together, we get:
10.0625 + 10.125 + 10.25 + 10.375 = 40.8125
Dividing by 4, we get the average length:
40.8125 / 4 = 10.344 Inches
Find out more on averages at https://brainly.com/question/20118982
#SPJ1
Related Questions
Given the equation ay + bx - c = 0, solve for the variable c
Answers
Answer:
Step-by-step explanation:
a= -bx+c divided by y
A tree 6m high casts a shadow 4m long. What angle do the sun's rays make with the ground?
Answers
Answer:
tan x° = 56.31°
Step-by-step explanation:
6/4= 1.5
hence x° = 56.31
Enter your answer as an integer. If there is no horizontal asymptote, enter the word none.

Answers
Given
\(h(x)=\frac{4x^3+4x-3}{2x^4+3x-3}\)Find
Horizontal Asymptotes
Explanation
As we know that there is two possible cases in arational function for there to be a horizontal asymptotes.
both depend on the higher degree of numerator and denominator.
1, if degree of denominator is equal to degree of numerator then there will be a horizontal asymptote at the ratio between the coefficients of the highest degree of the function.
2. if degree of denominator is lower to degree of numerator then there will be a horizontal asymptote at the y=0
here in given function degree of denominator is less than degree of numerator , so horizontal asymptote at y=0
Final Answer
horizontal asymptote at y=0
ASAP!!!
Determine the perimeter of the right triangle shown. Round your final answer to the nearest whole number, if necessary.
5 units
12 units
16 units
25 units

Answers
The perimeter of the triangle ABC is 12 units. Hence, option (B) is correct.
What is a triangle?A triangle is a geometric figure with three edges, three angles and three vertices. It is a basic figure in geometry.
The sum of the angles of a triangle is always 180°
In the given right angle triangle,
The vertices are A(-3, 2), B(-3, -1) and C(1, -1)
The distance between AB = 3,
And the distance between BC = 4
To find the distance of AC, use Pythagoreans theorem,
AC² = AB² + BC²
= 3² + 4²
= 9 + 16
AC² = 25
AC = 5
The perimeter of triangle ABC = AB + BC + AC
= 3 + 4 + 5
= 12
The perimeter of the triangle ABC is 12 unit.
To know more about Triangle on:
https://brainly.com/question/2773823
#SPJ1

find an example of a matrix and a matrix such that, letting and , the composition is a reflection over the line .
Answers
The required matrices are:
\(A= \begin{bmatrix}1 & 1 & 0\\1 & -1 & 0\end{bmatrix}\) and \(B= \begin{bmatrix}1 & 1\\1 & -1\\0 & 1\end{bmatrix}\)
letting T(x)=Ax and U(x)=Bx, the composition T∘U is a reflection over the line y=x.
A matrix is a rectangular array of numbers or other mathematical objects (such as complex numbers, polynomials, or functions) arranged in rows and columns. The size or dimensions of a matrix are given by the number of rows and columns it has, and a matrix with m rows and n columns is said to be an m × n matrix.
Let A be the 2×3 matrix:
\(A= \begin{bmatrix}1 & 1 & 0\\1 & -1 & 0\end{bmatrix}\)
and let B be the 3×2 matrix:
\(B= \begin{bmatrix}1 & 1\\1 & -1\\0 & 1\end{bmatrix}\)
Then, for any vector x in \(R^2\), we have:
\(T(U(x)) = A(Bx) = A(x_1 + x_2, x_1 - x_2, x_3)\)
\(= (x_1 + x_2 + x_1 - x_2, x_1 - x_2 - x_1 - x_2, 0)\)
\(= (2x_1, -2x_2, 0)\)
To show that this is a reflection over the line y=x, we can verify that T(U(x)) is equal to its own inverse. That is, we want to show that:
\(T(U(T(U(x)))) = x\)
Substituting T(U(x)) for y, we have:
\(T(U(T(U(x)))) = T(U(y)) = A(By) = A(2y_1, -2y_2, 0)\)
\(= (2y_1 + (-2y_2), 2y_1 - (-2y_2), 0)\)
\(= (4y_1, 4y_2, 0)\)
To see that this is equal to x, we note that the first and second components have been swapped, which corresponds to a reflection over the line y=x. Thus, T(U) is indeed a reflection over the line y=x.
To know more about matrix click here
brainly.com/question/30389982
#SPJ4
The complete question is:
Find an example of a 2×3 matrix A and a 3×2 matrix B such that, letting T(x)=Ax and U(x)=Bx, the composition T∘U is a reflection over the line y=x.
If £1 = US$1.11316 and A$1 = US$0.8558, how many British pounds will you get for one Australian dollar?
=£
Round to two decimal places
Answers
The correct answer is you will get approximately £1.30 for one Australian dollar.
To find out how many British pounds you will get for one Australian dollar, we need to determine the exchange rate between the British pound and the Australian dollar.
Given that £1 = US$1.11316 and A$1 = US$0.8558, we can calculate the exchange rate between the British pound and the Australian dollar as follows:
£1 / (US$1.11316) = A$1 / (US$0.8558)
To find the value of £1 in Australian dollars, we can rearrange the equation:
£1 = (A$1 / (US$0.8558)) * (US$1.11316)
Calculating this expression, we get:
£1 ≈ (1 / 0.8558) * 1.11316 ≈ 1.2992
Therefore, you will get approximately £1.30 for one Australian dollar.
Learn more about statistics here:
https://brainly.com/question/30915447
#SPJ11
Form a linear equation for the given statement and draw its graph:
Taxi fare for the first km is charged at Rs 20 and Rs 10 is charged for another extra
1 km.
Answers
Step-by-step explanation:
See the image for solution
Hope it helps
Have a great day

What is the first step when adding and subtracting rational expressions?
Answers
The First step in adding and subtracting the rational expression is making the denominator equal .
The Rational Expression is defined as the expression that is written in the p/q form , where q ≠ 0 .
Let us understand adding and subtracting of the rational expression by an Example .
For Example : add the rational expressions 2/3 and 1/4 .
The First Step in adding them is to make the denominator equal ,
to make the denominator equal , we take LCM ,
the LCM of 3 and 4 is 12 ,
the rational expression become ⇒ 8/12 and 3/12 .
On adding , we get ; (8 + 3)/12 = 11/12 .
On subtracting , we get ; (8 - 3)/12 = 5/12 .
Therefore , making the denominator equal is the first step for adding and subtracting rational expressions .
Learn more about Rational Expressions here
https://brainly.com/question/11620748
#SPJ4
It's possible to build a triangle with side lengths of 3, 3, and 9.
• A. True
• B. False

Answers
Answer:
yes
Step-by-step explanation:
Answer: False
Explanation: This wouldn't be possible because 3+3 = 6, which is not more than 9. If it was more than 9, it would be true.
solve differential equation dy/dx=y^2 . 16y(2)=0
Answers
The particular solution corresponding to the initial condition 16y(2) = 0 (which I assume means y(2) = 0), we can plug x = 2 and y = 0 into the equation:
-1/0 = 2 + C
To solve the differential equation dy/dx=y^2, we can separate the variables and integrate both sides.
dy/y^2 = dx
Integrating both sides:
-1/y = x + C
where C is the constant of integration. Solving for y:
y = -1/(x+C)
To solve the second part of the question, 16y(2) = 0, we substitute y(2) into the equation we just found:
y(2) = -1/(2+C)
16y(2) = 16*(-1/(2+C)) = -16/(2+C) = 0
Solving for C:
-16 = 0*(2+C)
Thus, C can be any value since 0 multiplied by any number is 0. Therefore, the solution to the differential equation dy/dx=y^2 and the equation 16y(2)=0 is y = -1/(x+ C), where C is any constant.
Learn more about Equation:
brainly.com/question/29538993
#SPJ11
a group contains n men and n women. how many ways are there to arrange these people in a row if the men and women alternate and the first person in the row is the youngest one?
Answers
If there are n men and n women in the group, we can first arrange the men and women separately in alternate positions. We can treat the men as a single group and arrange them in n! ways, and similarly, we can treat the women as a single group and arrange them in n! ways.
Next, we need to arrange the two groups (men and women) in alternate positions to satisfy the given condition. Since we need to place the youngest person (who could be a man or a woman) at the beginning of the row, we have two cases:
Case 1: The youngest person is a man
In this case, the men must start the arrangement, followed by the women. Since there are n men and n women, there are n! ways to arrange the men and n! ways to arrange the women. We can fix the youngest man at the beginning of the row, so there are (n-1)! ways to arrange the remaining n-1 men. Similarly, there are (n-1)! ways to arrange the n-1 women. Therefore, the total number of arrangements in this case is:
n! * n! * (n-1)! * (n-1)!
Case 2: The youngest person is a woman
In this case, the women must start the arrangement, followed by the men. Since there are n women and n men, there are n! ways to arrange the women and n! ways to arrange the men. We can fix the youngest woman at the beginning of the row, so there are (n-1)! ways to arrange the remaining n-1 women. Similarly, there are (n-1)! ways to arrange the n-1 men. Therefore, the total number of arrangements in this case is:
n! * n! * (n-1)! * (n-1)!
To get the total number of arrangements that satisfy the given condition, we need to add the number of arrangements in Case 1 and Case 2:
n! * n! * (n-1)! * (n-1)! + n! * n! * (n-1)! * (n-1)!
= 2 * n! * n! * (n-1)! * (n-1)!
= 2 * (n!)^2 * (n-1)!
Therefore, the total number of ways to arrange the people in a row with alternating men and women, starting with the youngest person, is 2 * (n!)^2 * (n-1)!.
The diagram shows a straight line ABCD.
A is the point (−260,480) D is the point (620,−180)
The line cuts the y-axis at B and the x-axis at C.

Answers
Answer:
B (0, 285 ) , C (380, 0 )
Step-by-step explanation:
the first step is to obtain the equation of the line in slope- intercept form
y = mx + c ( m is the slope and c the y- intercept )
calculate m using the slope formula
m = \(\frac{y_{2}-y_{1} }{x_{2}-x_{1} }\)
with (x₁, y₁ ) = A(- 260, 480 ) and (x₂, y₂ ) = D (620, - 180 )
m = \(\frac{-180-480}{620-(-260)}\) = \(\frac{-660}{620+260}\) = \(\frac{-660}{880}\) = - \(\frac{66}{88}\) = - 0.75 , then
y = - 0.75x + c ← is the partial equation
to find c substitute either of the 2 points into the partial equatio
using (620, - 180 ) , then
- 180 = - 465 + c ⇒ c = - 180 + 465 = 285
So y- intercept is B (0, 285 )
y = - 0.75x + 285 ← equation of line
to find the x- intercept , let y = 0 in the equation and solve for x
0 = - 0.75 + 285 ( subtract 285 from both sides )
- 285 = - 0.75x ( divide both sides by - 0.75 )
380 = x
x- intercept is C (380, 0 )
Difference:
x values
260 + 620 = 880
y values
480 + 180 = 660
Gradient = change in y/ change in x
Or
gradient = rise/run
= 660/880
= 3/4
= 0.75
We've got a negative slope - gradient must be negative
y = mx + c
y = - 0.75x + c
To find the y-intercept (c), which on the diagram is point B - you substitute one of the coordinates into the equation y = - 0.75x + c.
You use - point A or D - it don't matter which
I'll pick D - (620,−180)
y= -0.75x + c
-180 = - 0.75 x 620 + c
-180 = - 465 + c
+465
285= c
Thus, the equation is:
y = - 0.75x + 285
or
y = - 3/4x + 285
Where point B is (0,285) and C is (380,0)
To find:
B substitute x = 0 into the equation
(y= - 3/4 x 0 + 285)
C substitute y = 0 in the equation
0 = - 3/4x + 285
-285
- 285 = - 0.75x
÷ - 0.75
380 = x
( I converted 3/4 to 0.75 to make things clearer)
Hope this helps!
Can you please help me solve these.

Answers
Answer:
please correct me if I'm wrong but is it 9?
Antonia is purchasing a house for $210,000, with a 15-year fixed-rate
mortgage at 4.75% interest. She has made a 5% down payment. The house is
valued at $205,000, and the local tax rate is 3.5%. Her homeowners insurance
is $480 per year. What are her total monthly payments? (Use the table below
to calculate PMI premiums.)
Base-To-Loan %
95.01% to 97%
90.01% to 95%
85.01% to 90%
85% and Under
OA. $2232.92
B. $2420.09
OC. $2345.76
D. $2894.71
Fixed-Rate Loan
30 yrs. 15 yrs.
0.90% 0.79%
0.78% 0.26%
0.52% 0.23%
0.32% 0.19%
ARM 2% +1 Year Cap
30 yrs.
15 yrs.
na
0.92%
0.65%
0.37%
0.81%
0.54%
0.26%
Answers
Anthonia's total monthly payments equals to $2345.76. The Option C is correct.
How do we get the total monthly payments?To calculate Antonia's total monthly payments, we need to consider the following factors:
The amount of the mortgage:
Antonia is purchasing a house for $210,000, with a 5% down payment. Therefore, her mortgage amount is $199,500 ($210,000 - $10,500).The interest rate:
Antonia's mortgage has a fixed interest rate of 4.75% for 15 years.Private Mortgage Insurance (PMI):
Since Antonia made a down payment of less than 20%, she will need to pay PMI. To calculate the PMI premium, we need to determine the base-to-loan percentage, which is the percentage of the home's value that is being financed.
Since Antonia's home is valued at $205,000 and she made a down payment of $10,500, the base-to-loan percentage is 95.12% ($194,500 ÷ $205,000). Looking at the table provided, we can see that the PMI premium for a 15-year fixed-rate loan with a base-to-loan percentage of 95.01% to 97% is 0.26%.
Property taxes:
The local tax rate is 3.5% of the home's value, so Antonia will need to pay $7,175 ($205,000 x 3.5%) in property taxes per year. This is equivalent to $598 per month ($7,175 ÷ 12).Homeowners insurance:
Antonia's annual homeowners insurance premium is $480, which is equivalent to $40 per month.Using these factors, we can calculate Antonia's total monthly payments:
Data
Mortgage payment = $1,671.05 (calculated using a mortgage calculator)
PMI premium = $42.37 ($194,500 x 0.26% ÷ 12)
Property taxes = $598
Homeowners insurance = $40
Total monthly payments will equals to
= $1,671.05 + $42.37 + $598 + $40
= $2,351.42
Read more about monthly payments
brainly.com/question/28106777
#SPJ1
Answer:
It's A
Step-by-step explanation:
2232.92 - Source: trust me bro
Zora owns an online fashion boutique. She launched a promotion where followers of her social media account who tag a friend are entered into a giveaway. The linear relationship between the hours since launching the promotion and her total number of followers on social media is shown on the graph below. a. Find the rate of change and explain its meaning. mt
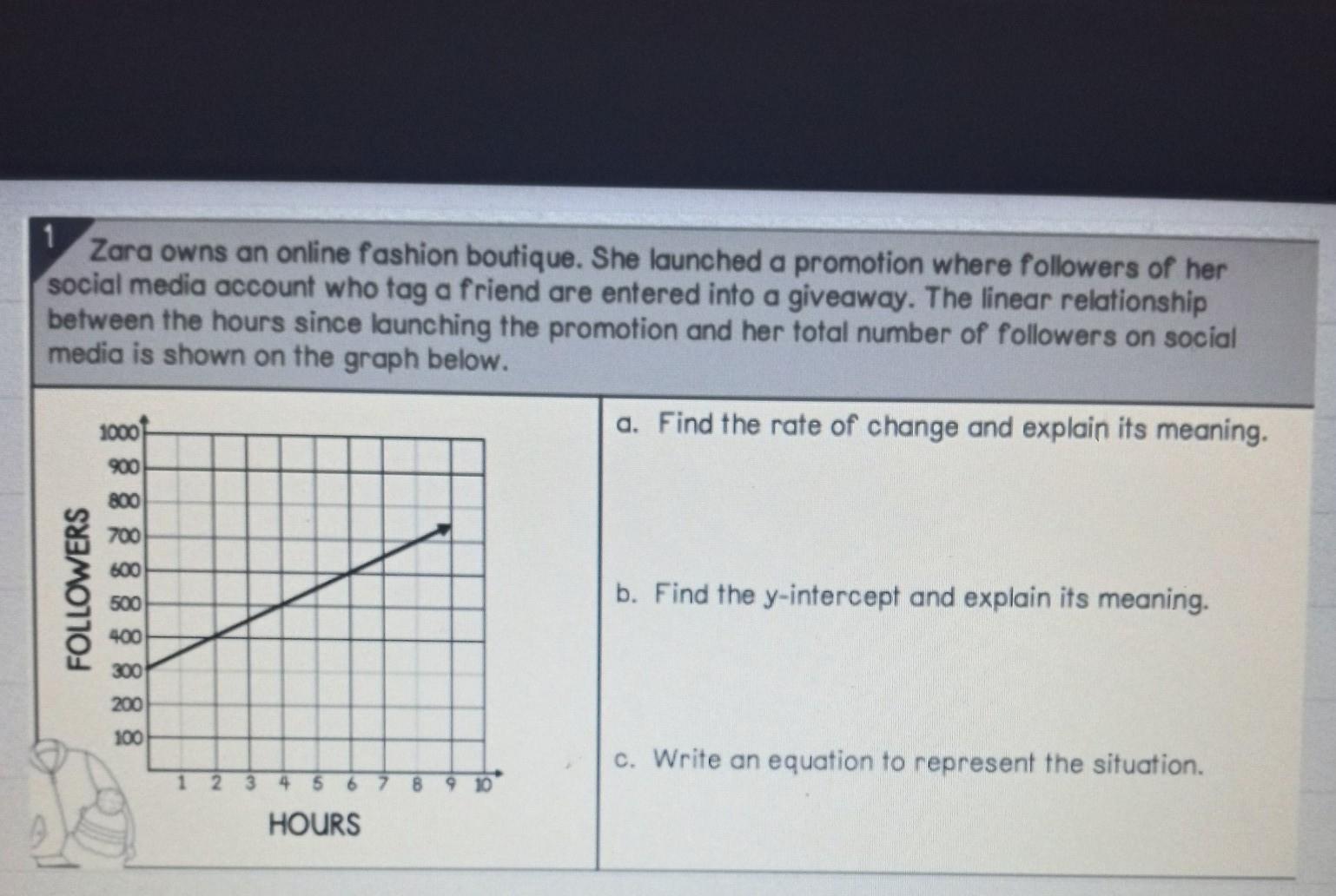
Answers
An equation is formed of two equal expressions. The equation that can be used to represent the graph is y=50x+300.
What is an equation?An equation is formed when two equal expressions are equated together with the help of an equal sign '='.
A.) The rate of change, in this case, signifies the number of followers Zora is gaining per hour. It can be found using any two coordinates of the line.
(x₁ , y₁) = (0,300) = coordinate of point 1,
(x₂ , y₂) = (2,400) = coordinate of point 2,
Now, the rate of change or the slope will be equal to,
\(m = \dfrac{y_2-y_1}{x_2-x_1} = \dfrac{400-300}{2-0} = 50\)
Hence, the rate at which Zora will gain followers is 50 followers/hour.
B.) The y-intercept is the value of y when the value of x is 0. Therefore, the y-intercept of the function is 300.
The y-intercept of the graph is 300, which means at the very initial stage Zora will have 300 followers.
C.) The graph is represented by a line, and the general equation of a line is represented by y=mx+c. To know the value of the constant c, substitute the value of the slope and any coordinate in the equation.
\(y=mx+c\\300=50(0)+c\\c=300\)
Hence, the equation that can be used to represent the graph is y=50x+300.
Learn more about Equation:
https://brainly.com/question/2263981
#SPJ1
rewrite 48+64 gcf using the distribution system
Answers
Answer:
8(6 + 8)
Step-by-step explanation:
48 + 64
GCF or Greatest Common Factor is the greatest factor in the equation. In this case, it is 8, so we factor out 8.
8(6 + 8)
So, the answer is 8(6 + 8)
Solve the open sentence.
-35n+6 57
ons-9 and ns6
n> 9 and ns 7
On-9 and ns1
ons 3 and ns 7

Answers
Answer:
-9 ≤n ≤1
Step-by-step explanation:
-3 ≤ n+6 ≤ 7
Subtract 6 from all sides
-3 -6≤ n+6-6 ≤ 7-6
-9 ≤n ≤1
Use the Simpson's rule to approximate ∫ 2.4 2f(x)dx for the following data
x f(x) f'(x)
2 0.6931 0.5
2.20.7885 0.4545
2.40.8755 0.4167
Answers
To approximate the integral ∫2.4 to 2 f(x) dx using Simpson's rule, we divide the interval [2, 2.4] into subintervals and approximate the integral within each subinterval using quadratic polynomials.
Given the data points (x, f(x)) = (2, 0.6931), (2.2, 0.7885), and (2.4, 0.8755), we can use Simpson's rule to approximate the integral.
Step 1: Determine the step size, h.
Since we have three data points, we can divide the interval [2, 2.4] into two subintervals, giving us a step size of h = (2.4 - 2) / 2 = 0.2.
Step 2: Calculate the approximations within each subinterval.
Using Simpson's rule, the integral within each subinterval is given by:
∫f(x)dx ≈ (h/3) * [f(x₀) + 4f(x₁) + f(x₂)]
where x₀, x₁, and x₂ are the data points within each subinterval.
For the first subinterval [2, 2.2]:
∫f(x)dx ≈ (0.2/3) * [f(2) + 4f(2.1) + f(2.2)]
≈ (0.2/3) * [0.6931 + 4(0.7885) + 0.8755]
For the second subinterval [2.2, 2.4]:
∫f(x)dx ≈ (0.2/3) * [f(2.2) + 4f(2.3) + f(2.4)]
≈ (0.2/3) * [0.7885 + 4(0.4545) + 0.8755]
Step 3: Sum up the approximations.
To obtain the approximation of the total integral, we sum up the approximations within each subinterval.
Approximation ≈ (∫f(x)dx in subinterval 1) + (∫f(x)dx in subinterval 2)
Calculating the values, we get the final approximation of the integral ∫2.4 to 2 f(x) dx using Simpson's rule.
To learn more about Simpson's rule click here:
brainly.com/question/30459578
#SPJ11
HELP WHATS THE ANSWER HUHU
Two computer technicians both charge a fee for a home visit ,plus an hourly rate for their work. Dana charges a $64.95 fee, plus $45/h. Tom charges a $79.95 fee, plus $40/h. For what length of service call do Dana and Tom charge the same amount?
Answers
Answer:
3 hours
Step-by-step explanation:
Step one:
given data
Dana charges
$64.95 fee, plus $45/h.
let the number of hours be x
and the total charges be y
y= 45x+64.95---------1
Tom charges
$79.95 fee, plus $40/h.
let the number of hours be x
and the total charges be y
y= 40x+79.95---------2
Step two:
Equate eqn 1 and 2 to find x
45x+64.95=40x+79.95
collect like terms
45x-40x=79.95-64.95
5x=15
divide by 15
x= 15/5
x= 3 hours
4ny = b
make y subject
Answers
y = b/4n
\(\\\\\)
Step-by-step explanation:
Given Equation : : 4ny = b for making 'y' subject→ y = b/4nJohn has a storage bin in the shape of a rectangular prism the storage bed is still in the and 1/2 ft long 2 ft wide and 2 ft tall Joe will put boxes that measure 1/2 ft on each side of the bed which is the greatest number of boxes drunken put into the bin
Answers
We know, volume of rectangular prism is given by :
\(V = lbh\\\\V=\dfrac{1}{2}\times 2\times 2\ ft^2\\\\V=2\ ft^2\)
Volume of cubic box :
\(v=a^3\\\\v=(\dfrac{1}{2})^3\ ft^3\\\\v=\dfrac{1}{8}\ ft^3\)
Number of cubes can be filled :
\(N=\dfrac{V}{v}\\\\N=\dfrac{2}{\dfrac{1}{8}}\\\\N=16\)
Therefore, 16 cubes can be put in the bin.
Hence, this is the required solution.
Carlos used a coordinate plane to design the patio shown. Each unit on the grid represents 1 meter. To buy materials to build the patio, he needs to know its perimeter. What is the perimeter of the patio?
Answers
A coordinate plane to design the patio the perimeter of the patio is 22 meters.
To calculate the perimeter of the patio, we need to add up the lengths of all the sides.
Given:
The design of the patio on the coordinate plane.
To find the perimeter of the patio, we need to determine the lengths of all its sides and then add them together.
Let's identify the coordinates of the vertices of the patio on the coordinate plane:
A(3, 2)
B(9, 2)
C(9, 7)
D(6, 7)
E(6, 4)
F(3, 4)
To calculate the length of each side, we can use the distance formula:
\(\[ \text{Length of a side} = \sqrt{{(x_2 - x_1)^2 + (y_2 - y_1)^2}} \]\)
Now let's calculate the length of each side:
Side AB: \(\(\sqrt{{(9 - 3)^2 + (2 - 2)^2}} = 6\)\)
Side BC: \(\(\sqrt{{(9 - 9)^2 + (7 - 2)^2}} = 5\)\)
Side CD: \(\(\sqrt{{(6 - 9)^2 + (7 - 7)^2}} = 3\)\)
Side DE: \(\(\sqrt{{(6 - 6)^2 + (4 - 7)^2}} = 3\)\)
Side EF: \(\(\sqrt{{(3 - 6)^2 + (4 - 4)^2}} = 3\)\)
Side FA: \(\(\sqrt{{(3 - 3)^2 + (2 - 4)^2}} = 2\)\)
Now, we can add up the lengths of all the sides to find the perimeter:
Perimeter = AB + BC + CD + DE + EF + FA
Perimeter = 6 + 5 + 3 + 3 + 3 + 2
Perimeter = 22
Therefore, the perimeter of the patio is 22 meters.
To know more about Perimeter visit-
brainly.com/question/30611225
#SPJ11
PLEASE HURRY!!!
what's the answer for 1 and 2?

Answers
Answer:
C and D
Step-by-step explanation:
l faux off Gabba off KK kk haha BB mm hd h
This is a scale drawing of
A ski chalet. The height of
the chalet in the drawing
is 7 inches. The scale that maps
the drawing to the actual chalet is 1 inch to 6.5 feet.

Answers
Answer:
Actual height of the Chalet = 45.5 ft
Step-by-step explanation:
Given:
Height of chalet in drawing = 7 inches
Scale of drawing = 1 inch : 6.5 ft
Required:
Actual height of the Chalet
SOLUTION:
Actual of height of the Chalet can be calculated using proportion as follows,
Let the actual height be x.
Thus,
1 in : 6.5 ft = 7 in : x ft
\( \frac{1}{6.5} = \frac{7}{x} \)
Cross multiply
\( 1*x = 7*6.5 \)
\( x = 45.5 ft \)
Actual height of the Chalet = 45.5 ft
3. The system of equations for two liquid surge tanks in series is
A₁ dh'₁/dt = q'ᵢ - 1/R₁ h'₁, q'₁ = 1/R₁ h'₁
A₂ dh'₂/dt = 1/R₁ h'₁ - 1/R₂ h'₂ q'₂ = 1/R₂ h'₂
Using state-space notation, determine the matrices A,B,C, and D assuming that the level deviations are the state variables: h'₁ and h'₂. The input variable is q'ᵢ , and the output variable is the flow rate deviation, q'₂.
Answers
The surge tank is a vital component of a system in which the flow rate fluctuates significantly. The flow rate entering the tank varies significantly, causing the fluid level in the tank to fluctuate as a result of the compressibility of the liquid. The surge tank is utilized to reduce pressure variations generated by a rapidly fluctspace uating pump flow rate. To determine the matrices A,B,C, and D using state-space notation, here are the steps:State representation is given by:dx/dt = Ax + Bu; y = Cx + DuWhere: x represents the state variablesA represents the state matrixB represents the input matrixC represents the output matrixD represents the direct transmission matrixThe equation can be written asA = [ -1/R₁ 0; 1/R₁ -1/R₂]B = [1/A₁; 0]C = [0 1/R₂]D = 0Thus, the matrices A,B,C and D assuming that the level deviations are the state variables: h'₁ and h'₂. The input variable is q'ᵢ, and the output variable is the flow rate deviation, q'₂ are given by A = [ -1/R₁ 0; 1/R₁ -1/R₂]B = [1/A₁; 0]C = [0 1/R₂]D = 0.Hence, the required matrices are A = [ -1/R₁ 0; 1/R₁ -1/R₂], B = [1/A₁; 0], C = [0 1/R₂], and D = 0 using state-space notation for the given system of equations for two liquid surge tanks.
surge tank: https://brainly.com/question/14143728
#SPJ11
Assume the nth partial sum of a series sigma n =1 to infinity an is given by the following: sn = 7n-5/2n + 5 (a) Find an for n > 1. (b) Find sigma n = 1 to infinity an.
Answers
(a) Using the formula for nth partial sum s2 = a1 + a2, we can find a2, a3, a4 and solving for the next term in the series.
(b) The sum of series is 7.
(a) To find an for n > 1, we can use the formula for the nth partial sum:
sn = 7n-5/2n + 5
Substituting n = 1 gives:
s1 = 7(1) - 5/2(1) + 5 = 6.5
We can then use this value to find a2:
s2 = 7(2) - 5/2(2) + 5 = 10
Using the formula for the nth partial sum, we can write:
s2 = a1 + a2 = 6.5 + a2
Solving for a2 gives:
a2 = s2 - 6.5 = 10 - 6.5 = 3.5
Similarly, we can find a3, a4, and so on by using the formula for the nth partial sum and solving for the next term in the series.
(b) To find the sum of the series sigma n = 1 to infinity an, we can take the limit as n approaches infinity of the nth partial sum:
lim n -> infinity sn = lim n -> infinity (7n-5/2n + 5)
We can use L'Hopital's rule to evaluate this limit:
lim n -> infinity (7n-5/2n + 5) = lim n -> infinity (7 - 5/(n ln 2)) = 7
Therefore, the sum of the series is 7.
Learn more about "series": https://brainly.com/question/24643676
#SPJ11
3) In recent years, a growing array of entertainment options competes for consumer time. By 2004 , cable television and radio surpassed broadcast television, recorded music, and the daily newspaper to become the two entertainment media with the greatest usage (The Wall Street Journal, January 26, 2004). Researchers used a sample of 10 individuals and collected data on the hours per week spent watching cable television and hours per week spent listening to the radio. Use a .05 level of significance and test for a difference between the population mean usage for cable television and radio.
Answers
A study conducted in 2004 examined the usage of cable television and radio among 10 individuals to determine if there was a significant difference in the average hours spent on each medium. Using a significance level of 0.05, statistical analysis was performed to test for a disparity between the population mean usage of cable television and radio.
The researchers collected data on the number of hours per week spent watching cable television and listening to the radio from a sample of 10 individuals. The objective was to determine if there was a significant difference in the average usage between cable television and radio, considering the increasing competition among various entertainment options.
To test for a difference between the population mean usage for cable television and radio, a statistical hypothesis test was conducted. The significance level (α) of 0.05 was chosen, which means that the results would be considered statistically significant if the probability of obtaining such extreme results by chance alone was less than 5%.
The test compared the means of the two samples, namely the average hours spent watching cable television and listening to the radio. By analyzing the data using appropriate statistical techniques, such as a two-sample t-test, the researchers determined whether the observed difference in means was statistically significant or could be attributed to random variation.
After conducting the hypothesis test, if the p-value associated with the test statistic was less than 0.05, it would indicate that there was a significant difference between the population mean usage of cable television and radio. Conversely, if the p-value was greater than 0.05, there would be insufficient evidence to conclude a significant disparity.
To learn more about statistical click here: brainly.com/question/31577270
#SPJ11
0.10(7l + 4s) its like due rn!!
Answers
The solution to the expression 0.10(7l + 4s) is 0.70l + 0.40s.
In mathematics, an expression is a combination of numbers, variables, and mathematical operations (such as addition, subtraction, multiplication, division, and exponentiation) that are combined in a meaningful way.
To solve this expression, we can use the distributive property of multiplication over addition, which states that:
a(b + c) = ab + ac
Using this property, we can rewrite the expression as:
0.10(7l + 4s) = 0.107l + 0.104s
Simplifying the multiplication, we get:
0.70l + 0.40s
Therefore, the solution to the expression 0.10(7l + 4s) is 0.70l + 0.40s.
To know more about an expression follow
https://brainly.com/question/723406
#SPJ1
A dice is taken on which numbers from 6 to 11 are written on its six faces. The HCF of numbers written on any pair of opposite faces is always 1. Based on this information, answer the questions that follow. How many different possible combinations are possible for writing the numbers on six faces?
Answers
There are 120 different possible combinations for writing the numbers on the six faces of the dice, satisfying the given condition.
How to find how many different possible combinations are possible for writing the numbers on six facesTo find the different possible combinations for writing the numbers on the six faces of the dice, we can consider the prime factorization of each number from 6 to 11.
The numbers from 6 to 11 are:
6, 7, 8, 9, 10, 11
Prime factorization of these numbers:
6 = 2 * 3
7 = 7
8 = 2^3
9 = 3^2
10 = 2 * 5
11 = 11
Since the highest common factor (HCF) of numbers written on any pair of opposite faces is always 1, it means that no prime factor is common between the numbers on any pair of opposite faces.
To determine the different combinations, we can count the number of ways we can arrange the prime factors on the six faces of the dice without repeating any factor.
The prime factors are:
2, 3, 5, 7, 11
Considering that each face of the dice can have one prime factor, the number of different combinations is equal to the number of ways we can arrange these prime factors.
Using the concept of permutations, the number of different combinations can be calculated as:
5! (5 factorial) which is equal to 5 * 4 * 3 * 2 * 1 = 120
Therefore, there are 120 different possible combinations for writing the numbers on the six faces of the dice, satisfying the given condition.
Learn more about combinations at https://brainly.com/question/4658834
#SPJ4
Name the Reflection Rule that results in the following transformation
A(1,4), B(2, 7), C(9, 3) to
A'(1,-4), B'(2, -7), C'(9, -3)
