7. Movers, Not Shakers moving company charges $800 plus $16 per hour. Hernia Movers
charges $720 plus $21 per hour.
a. After how many hours, h, would the two moving companies cost the same amount?
b. What would that amount be?
Answers
Answer:
a.16
b.1056
Step-by-step explanation:
a. So we know that Not Shakers charges 800 dollars + 16 per hour, so we can call that 800 + 16h. We also know that Hernia Movers charges 720 dollars + 21 per hour, so we can call that 720 + 21h.
Now in order to find when they will have the same amount, we have to solve for h.
Equation:
800 + 16h= 720+21h
800=720+5h
5h=80
h=16
The two companies will have the same amount after 16 hours.
b. Now we have to find that amount, so all we have to do is replace h with 16. so 720 + 21(16) = 1056.
Therefore, the amount will be 1056 dollars.
Related Questions
the average height of students at uh from an srs of 19 students gave a standard deviation of 3.2 feet. construct a 95% confidence interval for the standard deviation of the height of students at uh. assume normality for the data. a) (1.418, 10.732) b) (1.918, 5.732) c) (2.418, 4.732) d) (6.418, 11.732) e) (5.418, 9.732) f) none of the above
Answers
The 95% confidence interval for the standard deviation of the height of students at UH is (1.918, 5.732), which corresponds to option b.
To construct a 95% confidence interval for the standard deviation of the height of students at UH, we will use the Chi-square distribution. Given the sample standard deviation (s) of 3.2 feet, a sample size (n) of 19 students, and assuming normality for the data, we can find the confidence interval as follows:
1. Determine the degrees of freedom: df = n - 1 = 19 - 1 = 18
2. Identify the Chi-square values for the confidence level (95%): χ²_lower = 7.632, χ²_upper = 32.852 (using a Chi-square table or calculator)
3. Calculate the lower and upper bounds of the confidence interval:
Lower bound = sqrt((n - 1) * s² / χ²_upper) = sqrt(18 * (3.2)² / 32.852) ≈ 1.918
Upper bound = sqrt((n - 1) * s² / χ²_lower) = sqrt(18 * (3.2)² / 7.632) ≈ 5.732
Learn more about standard deviation here:
https://brainly.com/question/13905583
#SPJ11
The 95% confidence interval for the standard deviation of the height of students at UH is approximately (1.918, 5.732), Option B.
Construct a 95% confidence interval for the standard deviation of the height of students at UH, we'll use the given data and the Chi-Square distribution.
Here's a step-by-step explanation:
SRS (simple random sample) of 19 students, which means the degrees of freedom (df) = n - 1 = 19 - 1 = 18.
The sample standard deviation (s) is given as 3.2 feet.
Assume normality for the data.
A 95% confidence interval, we'll use the Chi-Square distribution table to find the critical values.
The two tail probabilities are 0.025 and 0.975, so we'll look up the Chi-Square values for 18 degrees of freedom and these probabilities:
\(- X^2_{0.025} = 30.191 (upper limit)\)
\(- X^2_{0.975} = 8.231 (lower limit)\)
Calculate the confidence interval for the population standard deviation (σ):
\(\((\sqrt((n - 1) \times s^2 / X^2_{upper}), \sqrt((n - 1) \times s^2 / X^2_{lower}))\)\)
Plug in the values:
\(- n = 19\)
\(- s = 3.2\)
\(- df = 18\)
\(\(- X^{2} _{upper} = 30.191\)\)
\(\(- X^2_{lower} = 8.231\)\)
Calculate the confidence interval:
\((√((18 \times 3.2^2) / 30.191), \sqrt((18 \times 3.2^2) / 8.231)) \approx (1.918, 5.732)\)
For similar questions on standard deviation
https://brainly.com/question/12402189
#SPJ11
What degree would be classified as an obtuse angle but is close to being a straight line
120
171
100
110
Answers
Answer: 171
Step-by-step explanation:
An obtuse angle means more than 90 degrees. (90 degrees would be the inside corner of your notebook paper) a straight line would be 180 degrees. 171 is the closest to 180
CAN SOMEONE PLEASE HELP ME
Answers
Answer:
sureeee what's uppp!!!:)
what do you need help with,
A pebble is dropped into a pond, and the resulting ripple travels 3 ft/sec. How fast is the area inside the ripple increasing when the ripple is 8 feet in diameter?
Answers
The answer is 24π square feet per second, which means that the area inside the ripple is increasing at this rate when the ripple is 8 feet in diameter.
To solve this problem, we need to use the formula for the area of a circle, which is A = πr². We know that the diameter of the ripple is 8 feet, so the radius is 4 feet (half of the diameter).
Next, we need to find the rate of change of the area with respect to time. This is given by the formula dA/dt = 2πr(dr/dt), where dr/dt is the rate at which the radius is changing (in this case, the speed of the ripple).
We are given that the speed of the ripple is 3 ft/sec. Therefore, dr/dt = 3 ft/sec.
Substituting the values we have, we get dA/dt = 2π(4)(3) = 24π.
So the area inside the ripple is increasing at a rate of 24π square feet per second when the ripple is 8 feet in diameter.
In more than 100 words, we have used the formula for the area of a circle, as well as the formula for the rate of change of the area with respect to time, to solve this problem. The speed of the ripple, which is given, is used to find the rate at which the radius is changing. Finally, we substitute the values we have into the formula to find the rate of change of the area. The answer is 24π square feet per second, which means that the area inside the ripple is increasing at this rate when the ripple is 8 feet in diameter.
To know more about diameter visit:
https://brainly.com/question/5501950
#SPJ11
From the rate of change in area, the increasing rate of area inside the ripple with diameter 8 feet and ripple rate 3 ft/sec is equals to 24π.
We have a pebble is dropped into a pond. Rate or speed of ripple travelling in pond = 3 ft/sec
We have to determine the rate of the area inside the ripple increasing when the ripple is 8 feet in diameter.
Let area and radius be A and r of the ripple respectively. Both are changing with time, so, \(\frac{ dr}{dt} = 3 ft/sec\). Area of circular ripple is written as,
A = πr² --(1)
Differentiating the above equation with respect to time,t, \(\frac{dA}{dt}= 2πr \frac{dr}{dt } \).
Now, for obtaining the increase rate of area inside the ripple, substitute r = d/2
= 4 feet and \(\frac{ dr}{dt} = 3\)
in above expression
=>\( \frac{dA}{dt} = 2π (4)(3) \)
= 24π
Hence, required value is 24π .
For more information about rate of change in area, visit :
https://brainly.com/question/28520282
#SPJ4
I'm been doing this question about an hour still can't solve so I really need your help! Really appreciate if u do :D

Answers
Explanation:
1 hat = 45 dollars
6 hats = 6*45 = 270 dollars
1 phone = 40 dollars
4 phones = 4*40 = 160 dollars
total = $270 + $160 = $430
Felicia spent a total of $430. Since this amount of money leaves her account, this means we write a negative sign out front to end up with the final answer of -430
In other words, her account balance went down by $430 which is why we use a negative. If someone gave her $430, then the answer would be positive.
Carla corporation issued 1,900 shares of $10 par value common stock conversion of 950 shares of $50
Answers
If Carla Corporation issued 1,900 shares of $10 par value common stock in exchange for the conversion of 950 shares of $50 convertible preferred stock, we can calculate the impact on the financial statements as follows:
Calculation of the Total Par Value of the Common Stock Issued:
The total par value of the common stock issued is equal to the number of shares issued multiplied by the par value per share. In this case, 1,900 shares were issued with a par value of $10 per share, so the total par value of the common stock issued is:
Total Par Value = 1,900 shares x $10/share = $19,000
Calculation of the Conversion Ratio:
The conversion ratio is the number of shares of common stock that can be obtained from one share of preferred stock. In this case, 950 shares of $50 convertible preferred stock were converted into 1,900 shares of common stock, so the conversion ratio is:
Conversion Ratio = 1,900 shares / 950 shares = 2:1
This means that for every share of preferred stock, the holder can receive two shares of common stock.
Calculation of the Value of the Preferred Stock Converted:
To determine the value of the preferred stock that was converted, we need to multiply the number of shares converted by the conversion price. The conversion price is the price at which the preferred stock can be converted into common stock. In this case, the conversion price is not given, so it is not possible to calculate the value of the preferred stock converted.
Impact on the Financial Statements:
The issuance of the 1,900 shares of common stock will increase the equity section of the balance sheet. The total par value of the common stock issued ($19,000) will be recorded as an increase in the common stock account, which is a component of the stockholders' equity section of the balance sheet.
If the preferred stock had any accumulated dividends or other preferences, these would need to be taken into account in the conversion process. Additionally, any difference between the fair value of the preferred stock and the par value of the common stock issued would need to be recorded as an adjustment to the additional paid-in capital account.
Without more information about the conversion price and any other terms of the conversion, it is not possible to provide a more specific analysis of the impact on the financial statements of Carla Corporation.
Learn more about " financial statements " :
https://brainly.com/question/26240841
#SPJ11
Ryan invested \$4,800$4,800 in an account in the year 1990, and the value has been growing exponentially at a constant rate. The value of the account reached \$6,300$6,300 in the year 1998. Determine the value of the account, to the nearest dollar, in the year 2007.
Answers
well, from 1990 to 1998 is 8 years, and we know the amount went from $4800 to $6300, let's check for the rate of growth.
\(\qquad \textit{Amount for Exponential Growth} \\\\ A=P(1 + r)^t\qquad \begin{cases} A=\textit{accumulated amount}\dotfill & \$6300\\ P=\textit{initial amount}\dotfill &\$4800\\ r=rate\to r\%\to \frac{r}{100}\\ t=\textit{years}\dotfill &8\\ \end{cases} \\\\\\ 6300=4800(1 + \frac{r}{100})^{8} \implies \cfrac{6300}{4800}=(1 + \frac{r}{100})^8\implies \cfrac{21}{16}=(1 + \frac{r}{100})^8\)
\(\sqrt[8]{\cfrac{21}{16}}=1 + \cfrac{r}{100}\implies \sqrt[8]{\cfrac{21}{16}}=\cfrac{100+r}{100} \\\\\\ 100\sqrt[8]{\cfrac{21}{16}}=100+r\implies 100\sqrt[8]{\cfrac{21}{16}}-100=r\implies \stackrel{\%}{3.46}\approx r\)
now, with an initial amount of $4800, up to 2007, namely 17 years later, how much will that be with a 3.46% rate?
\(\qquad \textit{Amount for Exponential Growth} \\\\ A=P(1 + r)^t\qquad \begin{cases} A=\textit{accumulated amount}\\ P=\textit{initial amount}\dotfill &4800\\ r=rate\to 3.46\%\to \frac{3.46}{100}\dotfill &0.0346\\ t=years\dotfill &17\\ \end{cases} \\\\\\ A=4800(1 + 0.0346)^{17} \implies A=4800(1.0346)^{17}\implies A \approx 8558.02\)
The perimeter of a triangle is 26 feet. The second side is twice as long as the first. the third side is 1 foot longer than the second side. What is the length of the 3 sides?
Answers
Answer:
5, 10 and 11 feet.
Step-by-step explanation:
Let length of first side be x feet then
second side = 2x feet
third side = 2x + 1 feet.
So we have the equation:
x + 2x + 2x + 1 = 26
5x + 1 = 26
5x = 25
x = 5 feet.
So first side = = 5 feet, second = 10 and third = 11 feet.
what's the slope (3,-1) and (-2,5)
Answers
Answer:
slope = - \(\frac{6}{5}\)
Step-by-step explanation:
Calculate the slope m using the slope formula
m = \(\frac{y_{2}-y_{1} }{x_{2}-x_{1} }\)
with (x₁, y₁ ) = (3, - 1 ) and (x₂, y₂ ) = (- 2, 5 )
m = \(\frac{5-(-1)}{-2-3}\) = \(\frac{5+1}{-5}\) = \(\frac{6}{-5}\) = - \(\frac{6}{5}\)
The second part of the journey took 25 minutes longer than the first part of the journey. find the value of x
Answers
The value of x will be equal to 5/12 for the given equation.
What is speed?Speed is defined as the ratio of the time distance travelled by the body to the time taken by the body to cover the distance.
From the given data we will form an equation
Ayshab walked x miles at 4 mph. She then walked 2x miles at 3 mph. The second part of the journey took 25 minutes longer than the first part of the journey
2x/3 = x/4 + 5/12
2x/ 3 = 3x/12 + 5/12
2x/3 = 3x + 5/2
24x = 9x + 5
15x = 15
X = 1
25 minutes/60 = 5/12
Therefore for the given equation, the value of x will be equal to 5/12.
To know more about Speed follow
brainly.com/question/6504879
#SPJ11
The complete question is:
Ayshab walked x miles at 4 mph. She then walked 2x miles at 3 mph. The second part of the journey took 25 minutes longer than the first part of the journey. Find the value of x
The fruit fly is often used to study genetic mechanisms,
because it reproduces rapidly scientists can observe the
effects of experiments on several generations.
(A) mechanisms, because it reproduces rapidly
(B) mechanisms, since it reproduces rapidly,
(C) mechanisms, since, with its rapid reproduction,
(D) mechanisms; because it reproduces rapidly,
(E) mechanisms; then rapid reproduction allows
Answers
(D) mechanisms; because it reproduces rapidly
The degree of comparison in an adjective expresses how important one thing is in connection to another in a different phrase of the sentence. The superlative degree of an adjective is utilized to contrast the quality with several or all others, whereas the comparative degree is used to contrast the quality against another of its kind.
A semicolon is a type of punctuation that joins two separate clauses in a sentence. They are not the same as colons, that are utilized to start lists, descriptions, or quotations. A semicolon may make a statement simpler to read and understand.
Here's another question with an answer similar about degree of comparison:
https://brainly.com/question/28233004
#SPJ4
Use long or synthetic division to find the quotient. (x^3 + x^2 – x – 1) ÷ (x - 1)
Answers
The quotient of (x³ + x² – x – 1) ÷ (x - 1) by long division or synthetic division is x² + 2x + 1.
To find the quotient using long or synthetic division, we divide the polynomial (x³ + x² – x – 1) by (x - 1).
Using long division:
x² + 2x + 1
__________________
x - 1 | x³ + x² - x - 1
- (x³ - x²)
___________
2x² - x - 1
- (2x² - 2x)
___________
x - 1
- (x - 1)
_________
0
Therefore, the quotient is x² + 2x + 1.
Alternatively, we can used synthetic division:
1 | 1 1 -1 -1
-1 0 -1
__________________
1 0 -1 -2
The last row of the synthetic division represents the coefficients of the quotient polynomial, so we have x² + 2x + 1 as the quotient.
In both methods, we obtain the same result: the quotient of (x³ + x² – x – 1) ÷ (x - 1) is x² + 2x + 1.
Learn more about long division here:
https://brainly.com/question/28824872
#SPJ11
is it bad for the dependent variable y to be correlated with the error term e if the independent variable x is not?
Answers
It is generally considered bad for the dependent variable y to be correlated with the error term e, even if the independent variable x is not.
This is because the presence of such correlation indicates that there may be omitted variables or measurement errors that are affecting both the dependent variable and the error term. In turn, this can lead to biased and inefficient estimates of the parameters in the regression model, as well as invalid hypothesis testing and confidence intervals.
To address this issue, it is recommended to carefully examine the data and model assumptions, consider alternative specifications or estimation methods, and possibly include additional variables or controls that may help explain the relationship between y and e.
This correlation between Y and E violates one of the key assumptions of the classical linear regression model, which states that the error term should be uncorrelated with both the dependent and independent variables. This violation can lead to biased and inconsistent estimators, ultimately affecting the reliability of the regression results.
To learn more about variables, click here:
https://brainly.com/question/17344045
#SPJ11
The perimeter of the square kitchen floor measures 48 feet. What is the area of the kitchen floor?
32 feet
96 square feet
144 square feet
144 cubic feet
Answers
Answer:
144 square feet
Step-by-step explanation:
a square has 4 equal sides + perimeter is the addition of n sides in an n sided shape. 48/4 = 12 so there is 12 feet per side. Area is length times height so 12 x 12 or 144. Two terms multiplied create a square feet so answer is c
Answer:
Step-by-step explanation −2=x
how many k-lists (x1, x2, . . . , xk) are possible, such that each xi is a positive integer and 1 ≤ x1 ≤ x2 ≤ · · · ≤ xk ≤ n? prove your answer.
Answers
The number of k-lists of positive integers such that 1 ≤ x1 ≤ x2 ≤ · · · ≤ xk ≤ n is (k + n - 1) C (k - 1).
The number of k-lists (x1, x2, . . . , xk) of positive integers such that 1 ≤ x1 ≤ x2 ≤ · · · ≤ xk ≤ n is given by the number of solutions to the following equation:
x1 + x2 + · · · + xk = S, where S is a positive integer such that S ≤ k × n.
To see why this is the case, note that each k-list can be uniquely associated with a solution to this equation by letting xi be the number of times i appears in the k-list.
Conversely, each solution to the equation corresponds to a unique k-list by letting xi be the number of times i appears in the k-list.
The number of solutions to this equation is given by the stars and bars formula, which states that the number of solutions to x1 + x2 + · · · + xk = S, where xi are non-negative integers, is equal to the number of ways to arrange S stars and k − 1 bars, where the bars separate the stars into k groups.
The number of stars is S, and the number of bars is k − 1, so the number of arrangements is (S + k − 2) choose (k − 1), or
(S + k - 2) C (k - 1) = (S + k - 2)! / [(k - 1)! (S - 1)!].
Therefore, the number of k-lists of positive integers such that 1 ≤ x1 ≤ x2 ≤ · · · ≤ xk ≤ n is (k + n - 1) C (k - 1), or equivalently, (k + n - 1) choose n.
Note that this result can also be proven combinatorically by observing that we can choose the k numbers from {1, 2, ..., n} that appear in the k-list, and then arrange them in increasing order. The number of ways to choose k numbers from {1, 2, ..., n} is n choose k, and the number of ways to arrange them in increasing order is 1. Thus, the total number of k-lists is n choose k.
To learn more about integers here:
https://brainly.com/question/15276410
#SPJ4
twice a number, increased by three, is between negative one and nine. Find all the numbers.
Answers
The solution set for the obtained inequality is {.......-5, -4, -3, -2, -1, 0, 1, 2}.
Given that, twice a number, increased by three, is between negative one and nine.
What is inequality?The relation between two expressions that are not equal, employing a sign such as ≠ ‘not equal to’, > ‘greater than’, or < ‘less than’.
Let the unknown number be x.
Now, twice a number, increased by three = 2x+3
The given expression is between negative one and nine
-1 < 2x+3 < 9
-1 < 2x+3
Subtract 3 to both the side of the inequality
-1-3<2x+3-3
⇒ -4 < 2x
Divide 2 to both the side of the inequality
-2 < x
Now, 2x+3 < 9
Subtract 3 to both the side of the inequality
2x+3-3<9-3
⇒ 2x<6
Divide 2 to both the side of the inequality
x < 3
So, -2<x<3
Solution set = {.......-5, -4, -3, -2, -1, 0, 1, 2}
Therefore, the solution set for the obtained inequality is {.......-5, -4, -3, -2, -1, 0, 1, 2}.
To learn more about a inequality visit:
https://brainly.com/question/20383699.
#SPJ1
andy can run 20 miles in 6 hours. how much miles can he run in 1 hour.
Answers
I see that this is a rate of change time of problem
If andy can run 20 miles in 6 hours, than our goal is to find out how much he runs in a hour
So, we just have to divide 20 by 6.
It's sorta hard to explain
Questions?
Anyhow, the answer is around 3.3 miles per hour
Answer:
3.33 miles
Step-by-step explanation:
We Know
Andy can run 20 miles in 6 hours.
How many miles can he run in 1 hour?
We Take
20 / 6 ≈ 3.33 miles
So, Andy can run about 3.33 miles in 1 hour.
A corporate bond has a face value of p dollars. The interest each year is 6% of the face value. After received each year. The payout is the sum of the face value and the total interest. years the total interest is the product of the number of years, 1, and the interest (a) Express the total interest /, in dollars, as a function of the age f, in years, of the bond. Note that "I is already provided. Do not include this in your submitted response to this question. I .06t+p Edit (b) Express the payout P, in dollars, as a function of f. Note that "P is already provided. Do not include this in your submitted response to this question. P= Edit
Answers
A corporate bond has a face value of p dollars. The interest each year is 6% of the face value. the total interest /, in dollars, as a function of the age f, in years, of the bond is
a)I(f) = 0.06 * f * p
b) P(f) = I(f) + p
What is the function?Generally, (a) The total interest, in dollars, as a function of the age f, in years, of the bond can be expressed as:
I(f) = 0.06 * f * p
This represents the total interest paid over f years, which is calculated by multiplying the annual interest rate (0.06), the number of years (f), and the face value of the bond (p).
(b) The payout P, in dollars, as a function of f can be expressed as:
P(f) = I(f) + p
This represents the total payout over f years, which is calculated by adding the total interest paid (I(f)) and the face value of the bond (p).
Read more about bond
https://brainly.com/question/10777799
#SPJ1
The table below shows how many gumballs of each color there are in a gumball machine.
Juan is going to buy one gumball, and the gumball is selected at random. What is the
approximate probability that Juan gets a yellow gumball?
A) 0.05
B) 0.06
C) 0.17
D) 0.20
Please explain how you get the answer so I could learn because yt videos don’t help and I don’t want to be a burden to my parents by asking them to help
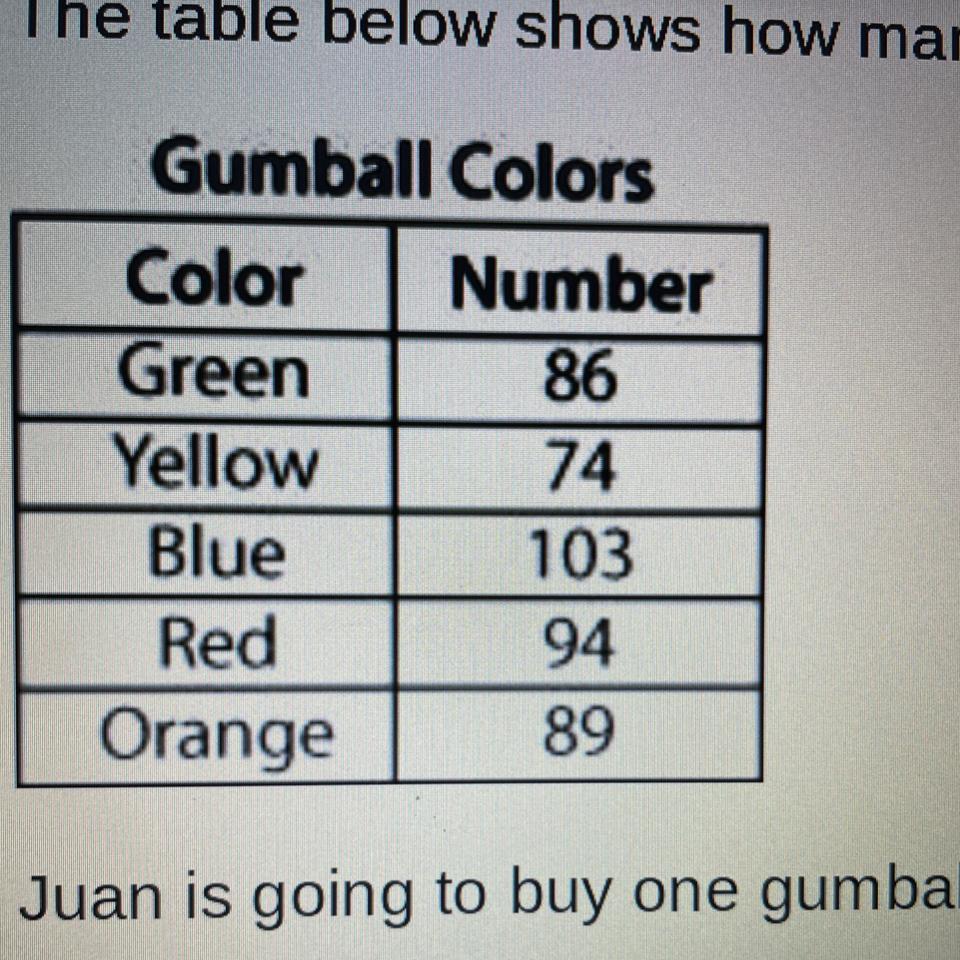
Answers
By taking the quotient between the number of yellow gumballs and the total number of gumballs, we will see that the probability is:
p = 0.17, so the correct option is C.
How to get the probability?
If we assume that all the gumballs have the same probability of being selected by the machine, then the probability of getting a yellow gumball is given by the quotient between the number of yellow gumballs and the total number of gumballs.
There are 74 yellow gumballs.There are 86 + 74 + 103+ 94 +89 = 446 gumballs in the machine.So the probability of getting a yellow one is:
p = 74/446 = 0.17
The correct option is C.
If you want to learn more about probability, you can read:
https://brainly.com/question/251701
Calculate the area of triangle CDE with altitude EF, given C (3, −2), D (−1, 2), E (2, 3), and F (0, 1).
Answers
Altitude EF=√((0-2)²+(1-3)²)
=√(4+4)=√8=2√2
area=1/2 ×4√2×2√2=8 square units
Maureen withdrew $20 from her bank account to go to lunch with friends. Later, she
deposited $25 that she made babysitting.
What is the total change in Maureen's bank account relative to where it started?
Answers
Answer:
+5
Step-by-step explanation:
The total change in Maureen's bank account relative to where it started is +5.
Given that, Maureen withdrew $20 from her bank account to go to lunch with friends. Later, she deposited $25 that she made babysitting.
What is the withdrawal of money?A withdrawal involves removing funds from a bank account, savings plan, pension, or trust.
Now, withdrawal of $20=-20 and deposit of $25=+25
Total change in bank account=-20+25=
Therefore, the total change in Maureen's bank account relative to where it started is +5.
To learn more about withdrawal of money visit:
https://brainly.com/question/3975666.
#SPJ6
Is this relation a function? Justify your answer.
O A. Yes, because the number of x-values is the same as the number of
y-values.
B. Yes, because every x-value has a single y-value.
C. Yes, because every x- and y-value is positive.
D. No, because two points with the same y-value have different x-
values.

Answers
Answer:
B.
Step-by-step explanation:
This relation passes the vertical line test and is a function.
Answer: B.
WILL MARK BRAINLIEST.

Answers
Answer:
option d
Step-by-step explanation:
The cross section of the rectangular prism is parallel to the base.
So, the length and width is congruent to the base. So, same length and width
URGENT HELP PLEASE WITH THESES FOUR QUESTIONS THE FIRST WILL BE REWARDED BRAINLIEST AND 5 stars

Answers
Answer:
Step-by-step explanation:
x=intercept is when the line intersect with the x axis when y=0
6: x intercept (1,0)
y intercept is when the line intersect with y axis at certain point or when x=0
y intercept (0.-24)
5: (0,-4) the y intercept
( 25,0) is the x intercept
21: (0,20) y intercept
(6,0) x intercept
22: (8,0) x intercept
(0 , 4) the y-intercept
Given f(x) = 3. + 1, solve for when f(3) = 7.
Answers
Answer:
The question is incomplete
Is (1, -2) a solution of the graphed inequality?
Answers
Answer:
No, it is not it is a coordinate point
A graphed inequality x<8 that example
Step-by-step explanation:
Answer:
Please attach the graph, and I will edit the answer.
Step-by-step explanation:
Thanks.
what method statistically finds the most defects? group of answer choices code reviews system tests unit tests only a combination makes sense.
Answers
Code reviews are most likely to find the most defects. So, the correct answer is A).
The statistical method that finds the most defects in software development depends on various factors. Code reviews can identify defects early on in the development process, while system tests can find issues with the overall functionality of the software.
Unit tests can catch defects at a lower level and ensure that individual components of the software are working as expected. However, it is often a combination of these methods that provides the best results.
By utilizing a variety of testing and review techniques, software developers can ensure that defects are caught early and that the software meets the necessary requirements and quality standards. so, the correct answer is Code review and option is A).
To know more about code reviews:
https://brainly.com/question/10585841
#SPJ4
Determine whether the statement is true or false. If the statement is false, explain why. The midpoint of the segment joining (0,0) and (38,38) is 19.
Answers
The midpoint has coordinates (19,19) as per the midpoint formula.
The statement is false.
The statement is false. The midpoint of the segment joining two points is determined by taking the average of their x-coordinates and the average of their y-coordinates. In this case, the two given points are (0,0) and (38,38).
To find the x-coordinate of the midpoint, we take the average of the x-coordinates of the two points:
(x1 + x2) / 2
= (0 + 38) / 2
= 38 / 2
= 19
Therefore, the x-coordinate of the midpoint is 19, which matches the statement. However, to determine if the statement is true or false, we also need to check the y-coordinate.
To find the y-coordinate of the midpoint, we take the average of the y-coordinates of the two points:
(y1 + y2) / 2
= (0 + 38) / 2
= 38 / 2
= 19
The y-coordinate of the midpoint is also 19. Therefore, the coordinates of the midpoint are (19,19), not 19 as stated in the statement. Since the midpoint has coordinates (19,19), the statement is false.
To learn more about the midpoint;
https://brainly.com/question/28224145
#SPJ4
A 15 foot ladder is leaning against a wall of a house. The base of the ladder is pulled away from the wall at a rate of 2ft/sec. Find the rate at which the area of the triangle is changing when the base of the ladder is 9ft from the wall
Answers
The rate at which the area of the triangle is changing when the base of the ladder is 9 ft from the wall will be the negative 8/3.
What is differentiation?The rate of change of a function with respect to the variable is called differentiation. It can be increasing or decreasing.
A 15-foot ladder is leaning against a wall of a house.
The base of the ladder is pulled away from the wall at a rate of 2ft/sec.
Then the equation will be
x² + y² = 15²
x² + y² = 225
Differentiate with respect to time. Then we have
2x (2) + 2yy' = 0
y' = -2x / y
Then the rate at which the area of the triangle is changing when the base of the ladder is 9 ft from the wall will be
Then the value of x will be
x² + 9² = 15²
x² + 81 = 225
x² = 144
x = 12 ft
Then the rate will be
y' = -2x / y
y' = – 2 × 12 / 9
y' = – 8/3
More about the differentiation link is given below.
https://brainly.com/question/24062595
#SPJ1
2000000000+2000000000
Answers
Answer:
its 4000000000
Step-by-step explanation:
add numbers
Answer:
2000000000+2000000000= 4000000000