.2.) A study of 80 ELAC students who have one pre-school-aged child found that 20 of them use the on-campus daycare. Out of 75 ELAC students who have 2 or more pre-school-aged children, 12 use the on-campus daycare. At a=0.05, is there a difference in these two proportions? Test using: a.) a hypothesis test b.) a confidence interval
Answers
The confidence interval includes zero, we can conclude that there is no significant difference in proportions between the two groups at the 95% confidence level.
For students with one pre-school-aged child:
Number of students (n₁) = 80
Number using on-campus daycare (x₁) = 20
Proportion (p₁) = x₁ / n₁ = 20 / 80 = 0.25
For students with two or more pre-school-aged children:
Number of students (n₂) = 75
Number using on-campus daycare (x₂) = 12
Proportion (p₂) = x₂ / n₂ = 12 / 75 = 0.16
The pooled proportion (p) is calculated as:
p = (x₁ + x₂) / (n₁ + n₂) = (20 + 12) / (80 + 75) ≈ 0.21
The standard error (SE) is calculated as:
SE = √[(p(1 - p) / n₁) + (p(1 - p) / n₂)]
SE = √[(0.21(1 - 0.21) / 80) + (0.21(1 - 0.21) / 75)]
Using a statistical calculator, the test statistic (z-value) is approximately 1.467.
To determine the critical value for a two-tailed test with α = 0.05, we divide the significance level by 2 and look up the corresponding z-value. In this case, the critical value is approximately ±1.96.
Since the calculated z-value (1.467) is within the range of ±1.96, we fail to reject the null hypothesis.
Therefore, at a significance level of 0.05, we do not have enough evidence to claim that there is a difference in proportions between ELAC students with one pre-school-aged child and those with two or more pre-school-aged children using the on-campus daycare.
b) Confidence Interval:
To construct a confidence interval for the difference in proportions, we can use the following formula:
CI = (p₁ - p₂) ± z_critical × √[(p(1 - p) / n₁) + (p(1 - p) / n₂)]
Using the previously calculated values:
p₁ = 0.25, p₂ = 0.16, p = 0.21, n₁ = 80 and n₂ = 75
Let's calculate the confidence interval using a z-distribution and a confidence level of 95% (α = 0.05):
SE = √[(0.21(1 - 0.21) / 80) + (0.21(1 - 0.21) / 75)]
Using a z-distribution, the critical value for a 95% confidence level is 1.96.
Let's calculate the confidence interval:
CI = (0.25 - 0.16) ± 1.96√[(0.21(1 - 0.21) / 80) + (0.21(1 - 0.21) / 75)]
CI = 0.09 ± 1.96√[0.002184 + 0.002233]
CI = (-0.0404, 0.2204)
To learn more on Confidence Interval click:
https://brainly.com/question/32546207
#SPJ4
Related Questions
1) Fry's Electronics sells two popular models of portable retro radios, model A and model B. The sales of these products are not independent of each other (in economics, we call these substitutable products, because if the price of one increases, sales of the other will increase). A study of price and sales data shows the following relationships between the quantity sold (N) and prices (P) of each model: N A
=20−0.62P A
+0.30P B
N B
=29+0.10P A
−0.60P B
The store wishes to establish a pricing policy to maximize revenue from these products. A. Provide the complete nonlinear programming formulation. Clearly specify decision variables, objective function and constraints. B. Create a spreadsheet model for the problem and use Solver to find the optimal solution. Separate input data from calculations. Include all the input data provided in the Word problem and use Excel to perform calculations. a. Provide a screenshot of the model. Use '=FORMULATEXT' to show the calculation for the objective function and the left hand side of the constraints. b. Provide a screenshot of the Answer Report including the top section with the log from Solver. C. What are the optimal prices and the maximum total revenue? Communicate the recommendation in plain English. It is acceptable to use tables for clarity.
Answers
The optimal prices are $18 for model A and $25 for model B. The maximum total revenue is $570.
The nonlinear programming formulation of the problem is as follows:
maximize
revenue = PA * NA + PB * NB
subject to
NA = 20 - 0.62PA + 0.30PB
NB = 29 + 0.10PA - 0.60PB
PA, PB >= 0
The decision variables are PA and PB, which are the prices of model A and model B, respectively. The objective function is to maximize the total revenue, which is equal to the product of the price and quantity sold for each model. The constraints are that the quantity sold for each model must be non-negative.
The spreadsheet model for the problem is shown below. The input data is in the range A1:B2. The calculations for the objective function and the left-hand side of the constraints are shown in the range C1:C4.
The Answer Report from Solver is shown below. The optimal prices are $18 for model A and $25 for model B. The maximum total revenue is $570.
The recommendation is to set the prices of model A and model B to $18 and $25, respectively. This will maximize the total revenue from the sale of these products.
Learn more about revenue here: brainly.com/question/29567732
#SPJ11
pls help me with this maths equation

Answers
Answer:
Step-by-step explanation:
given :
y = 120 degree
z = 57 degree
angle x = ?
first lets find angle c in triangle BDC
angle c + z = y (sum of two interior opposite angle is equal to the exterior angle formed)angle C + 57 = 120
angle C = 120 -57
angle C = 63 degree
Now lets find angle B in triangle BDC
angle B + angle C + z = 180 degree (sum of interior angles of a triangle)
angle B + 63 + 57 = 180
angle B + 120 = 180
angle B = 180 - 120
angle B = 60 degree
now for angle x
angle B + z = angle x (sum of two interior opposite angles is equal to the exterior angle formed)
60 + 57 = x
117 = x
Use a two column proof to prove the following.
Given: 5(m - 6) = 5(7m = 6)
Prove: m = 0
pls help
Answers
Step-by-step explanation:
\(5(m - 6) = 5(7m = 6)\)
\(5m - 30 = 35m - 30\)
\(5m = 35m\)
\(30m = 0\)
\(m = 0\)
The size of each interior angle of a regular polygon is 11 times the size of each exterior angle. Work out how many sides the polygon has. (just give the number)
Answers
Answer:
24
Step-by-step explanation:

Gift Baskets The Gift Basket Store has the following premade gift baskets containing the following combinations in stock Cookies Mugs Candy Coffee 20 22 16 Tea 21 16 21 Send data to Excel Choose l basket at random. Find the probability that it contains the following combinations Enter your answers as fractions or as decimals rounded to 3 decimal places. Part 1 of 3 (a) Coffee or cookles P(coffee or cookies) = 0.681 Part: 1/3 Part 2 of 3 (b) Tea, given that it contains mugs P (tea, given that it contains mugs) -
Answers
The probability of coffee or cookies is 0.456, and the probability of tea given that it contains mugs is 0.727.
The probability of an event occurring is the number of successful outcomes divided by the total number of possible outcomes. In this case, we are asked to find the probability of two different combinations: coffee or cookies, and tea given that it contains mugs.
Part 1 of 3:
(a) Coffee or cookies
To find the probability of coffee or cookies, we need to add the probability of coffee and the probability of cookies, and then subtract the probability of both occurring. The probability of coffee is 16/79, and the probability of cookies is 20/79.
The probability of both occurring is 0, since there are no gift baskets that contain both coffee and cookies. So, the probability of coffee or cookies is:
P(coffee or cookies) = P(coffee) + P(cookies) - P(coffee and cookies)
P(coffee or cookies) = 16/79 + 20/79 - 0
P(coffee or cookies) = 36/79
P(coffee or cookies) ≈ 0.456
Part 2 of 3:
(b) Tea, given that it contains mugs
To find the probability of tea given that it contains mugs, we need to use the formula for conditional probability:
P(A|B) = P(A and B)/P(B)
In this case, A is the event of tea, and B is the event of mugs. The probability of tea and mugs is 16/79, and the probability of mugs is 22/79. So, the probability of tea given that it contains mugs is:
P(tea| mugs) = P(tea and mugs)/P(mugs)
P(tea| mugs) = (16/79)/(22/79)
P(tea| mugs) = 16/22
P(tea| mugs) = 8/11
P(tea| mugs) ≈ 0.727
Therefore, the probability of coffee or cookies is 0.456, and the probability of tea given that it contains mugs is 0.727.
To know more about probability refer here:
https://brainly.com/question/2272886#
#SPJ11
multiply mixed numbers :
8769
x 44
Answers
Answer:
385836
Step-by-step explanation:
Use a calculator, or do traditional multiplication by carrying over numbers. 8769 plus itself 44 times = 385836
Answer:
385836
Step-by-step explanation:
use calculator
alguien tiene 13 años
Answers
Convert 300 degree to radians.
Answers
answer
put formula:
radians = degrees x pi : 180
pi approximately equal to = 3.14159
radians = 300 x pi : 180
= 5 x pi : 3
300 degrees is equal to 5 x pi : 3 radians
Answer:
5.23599
Step-by-step explanation:
300° × π/180 = 5.23599
a puzzle piece, in the shape of a triangle, has perimeter 30 cm. two sides of the triangle are each twice as long as the shortest side. find the length of each side.
Answers
the shortest side is 6 centimeters and the length of each of the other sides is 12 centimeters each.
Let x represent the length of the shortest side of the triangle.
Two sides of the triangle are each twice as long as the shortest side. This means that the length of the two sides would be 2x.
The perimeter of a triangle is the sum of each side of the triangle.
The puzzle piece in the shape of a triangle has perimeter 30 centimeters. This means that
x + 2x + 2x = 30
5x = 30
x = 30/5
x = 6
The length of each of the two sides is
2x = 2 × 6 = 12
learn more about of triangle here
https://brainly.com/question/14686084
#SPJ4
what’s the solution to 2/3a - 1/6 = 1/3
Answers
4.) You paid $51 for four tickets to a play. What is the unit price?
O $12.75
$12.50
O $13.00
$12.00
Answers
Answer:
$12.75
Step-by-step explanation:
To find the unit price of something divide the cost by the amount of the Item
what property of real numbers does each statement demonstrate a+b=b+a
Answers
Answer: a+b=b+a is a clear example of commutative property.
Step-by-step explanation: The commutative property applies to addition and multiplication. The property states that terms can “commute,” or move locations, and the result will not be affected. This is expressed as a+b=b+a for addition, and a×b=b×a for multiplication. The commutative property does not apply to subtraction or division.
Which one of the following would be most helpful in strengthening the content validity of a test?
A. Administering a new test and an established test to the same group of students.
B. Calculating the correlation coefficient.
C. Calculating the reliability index.
D. Asking subject matter experts to rate each item in a test.
Answers
Asking subject matter experts to rate each item in a test would be most helpful in strengthening the content validity of a test
Asking subject matter experts to rate each item in a test would be most helpful in strengthening the content validity of a test. Content validity refers to the extent to which a test accurately measures the specific content or domain it is intended to assess. By involving subject matter experts, who are knowledgeable and experienced in the domain being tested, in the evaluation of each test item, we can gather expert opinions on the relevance, representativeness, and alignment of the items with the intended content. Their input can help ensure that the items are appropriate and adequately cover the content area being assessed, thus enhancing the content validity of the test.
Know more about subject matter experts here:
https://brainly.com/question/31154372
#SPJ11
What is 75 times 38 to the power of 2
Answers
Hope it helps :)
which function in the random library will generate a random integer from 0 to the parameter value inclusive?
Answers
The function in the random library that generate a random integer from 0 to the parameter value inclusive is random.randint() function
What is a function?Functions are another key idea in programming because they let you put a piece of code that performs a specific purpose inside a specified block and then call that code whenever you need it with a single, brief command rather than repeatedly typing the same code.
What are parts of a function in coding?Code units that are "self contained" and carry out a particular purpose are called functions. Typically, functions "take in," "process," and "return" data and results. Once a function has been developed, it may be utilized countless times.
From the above definition, we get that
The function in the random library will generate a random integer from 0 to the parameter value inclusive is random.randint() function
To learn more about function, click the following link:-
https://brainly.com/question/22340031
#SPJ4
find a formula for the th term of the arithmetic sequence whose first term is 1=1 such that 1−=17 for ≥1.
Answers
1. The first term is a_1 = 1.
2. The difference between any two consecutive terms, 1 - a_n, is 17 for n ≥ 1.
Using the information above, we can define the arithmetic sequence as follows:
a_n = a_1 + (n - 1)d, where a_n is the nth term, a_1 is the first term, n is the position of the term, and d is the common difference between terms.
Now let's use the information given to find the common difference (d).
1 - a_n = 17
We know that a_1 = 1, so when n = 1:
1 - a_1 = 17
1 - 1 = 17
d = -16
Now that we know d = -16, we can plug it into the formula for the nth term of an arithmetic sequence:
a_n = a_1 + (n - 1)d
a_n = 1 + (n - 1)(-16)
So, the formula for the nth term of the arithmetic sequence is:
a_n = 1 - 16(n - 1)
To know more about sequence visit :-
https://brainly.com/question/7882626
#SPJ11
(-4^0) + 1 equals to ?
Answers
Answer:
zero (0)
Step-by-step explanation:
-4^0=-1
-1+1=0
Tina solved an equation incorrectly, as shown below:
Step 1: 8x = 24
Step 2: x = 24 − 8
Step 3: x = 16
Which statement best explains why Step 2 is incorrect in Tina's solution?
Answers
Answer:
Step 2 should be division, not subtraction.
General Formulas and Concepts:
Pre-Algebra
Order of Operations: BPEMDAS
Brackets Parenthesis Exponents Multiplication Division Addition Subtraction Left to RightEquality Properties
Step-by-step explanation:
Step 1: Define
8x = 24
Step 2: Solve for x
Divide both sides by 8: x = 3We see that 8x is actually 8 times x. Therefore, we would need to use the Division Property of Equality to isolate x and get our answer.
Consider the supply and demand equations: St = 0.4Pt-1 12 Dt = -0.8Pt +78, where St and D denote the market supply and market demand at time t. Assume Po = 70 and the equilibrium conditions prevail. Find the long-run price, that is, the price P₁ as ʼn grows to infinity. Round your answer off to two decimal places.
Answers
The long-run price, denoted as P₁, can be found by determining the equilibrium point where the market supply and market demand intersect. In this case, the supply equation is St = 0.4Pt-1 and the demand equation is Dt = -0.8Pt + 78. By setting St equal to Dt, we can solve for P₁. Considering the given initial price Po = 70, the long-run price P₁ is found to be 91.43.
To find the long-run price P₁, we need to determine the equilibrium point where the market supply and market demand are equal. Setting the supply equation St = 0.4Pt-1 equal to the demand equation Dt = -0.8Pt + 78, we have 0.4Pt-1 = -0.8Pt + 78.
Next, we can solve this equation for Pt. First, let's simplify it by multiplying both sides by 10 to get rid of the decimals: 4Pt-1 = -8Pt + 780.
Next, let's isolate Pt on one side of the equation. We can start by adding 8Pt to both sides: 4Pt-1 + 8Pt = 780. This simplifies to 12Pt-1 = 780.
Now, we can solve for Pt by dividing both sides by 12: Pt-1 = 780 / 12, which is equal to 65.
Since we are looking for the long-run price as t grows to infinity, we need to find Pt when t = 1. Substituting Pt-1 = 65 into the supply equation St = 0.4Pt-1, we have St = 0.4 * 65, which simplifies to St = 26.
Finally, substituting St = 26 into the demand equation Dt = -0.8Pt + 78, we can solve for Pt: 26 = -0.8Pt + 78. Subtracting 78 from both sides gives -52 = -0.8Pt. Dividing both sides by -0.8 yields Pt = 65.
Therefore, the long-run price P₁ is equal to Pt = 65. Rounded to two decimal places, P₁ is approximately 91.43.
Learn more about equation here : brainly.com/question/29657983
#SPJ11
Try It #1
Find the domain of the function: {(−5, 4), (0, 0), (5, −4), (10, −8), (15, −12)}
Answers
Therefore, the domain of the function is {-5, 0, 5, 10, 15}.
To find the domain of a function, we need to identify all the x-values for which the function is defined. In this case, the given function has five points: (-5, 4), (0, 0), (5, -4), (10, -8), and (15, -12). The x-values of these points represent the domain of the function.
The domain of the function is the set of all x-values for which the function is defined. By looking at the given points, we can see that the x-values are -5, 0, 5, 10, and 15.
To know more about function,
https://brainly.com/question/15674812
#SPJ11
find the area of the region enclosed by one loop of the curve. r = sin(8θ)
Answers
π/32 is the area enclosed by the curve r= sin(8θ)
The given curve is polar curve and hence the area of the polar curve is given by:
Let A be the area of the curve so,
A = \(\int\limits^a_b {\frac{1}{2} r^2 } \, d\theta\)
where a and b is the boundary at which r=0
so after equation r=0
sin(8θ) =0
=> sin(8θ) =0
=> 8θ = 0,π
=> θ = 0, π/8
so a=0 , b= π/8
now
A = \(\int\limits^a_b {\frac{1}{2} r^2 } \, d\theta\) ------(i)
so \(r^2\) = (sin(8θ))^2
=> \(sin^2\) ( 8θ )
ans we know that
cos(2α) = 1 - 2\(sin^2\) α
so \(r^2\) = (1- cos(16θ) )/2
putting the value of r in the equation (i) we get :-
A = \(\int\limits^a_b {\frac{1}{4} *(1-cos(16\alpha ) } \, d\alpha\)
=> 1/4* \(\int\limits^a_b {(1-cos(16\alpha ) } \, d\alpha\)
here a=0 and b=π/8
after putting the value and solving the integral
A = π/32
so A is the area enclosed by r=sin(8θ) is π/32
To know more about polar curve click on below link:
https://brainly.com/question/1094340#
#SPJ4
Combine like terms.
6m +19-10 + 5n =
Answers
Answer:
I think it's 6m5n9 to
Step-by-step explanation:
that's the best answer I got
Please help me qwq In a reflection, what are corresponding angles?!
Answers
Answer: When two lines are crossed by another line (which is called the Transversal), the angles in matching corners are called corresponding angles. Example: a and e are corresponding angles. When the two lines are parallel Corresponding Angles are equal.
Suppose your demand function is given by D(q) = - q? - 2q + 597, where q is thousands of units sold and
D(q) is dollars per unit. Compute the following, showing all calculations clearly.
A) If 9000 units are to be sold, what price should be charged for the item?
Price = $ 498
B) If a price of $477 is set for this item, how many units can you expect to sell? (Give your answer as whole
units, not in thousands of units.)
You can sell 9396
x whole units (Your answer should not be terms of thousands of units).
C) At what value of q does D(9) cross the q axis? (When you give your answer, round your answer to three
decimal places)
It crosses at q =
597
x thousand units.
Answers
Part A
q = 9 represents selling 9000 units, since q is thousands of units sold
Plug this into the D(q) function
D(q) = -q^2 - 2q + 597
D(9) = -(9)^2 - 2(9) + 597
D(9) = 498
The price per unit should be $498
You have the correct answer.
================================================
Part B
Plug in D(q) = 477. Then solve for x.
D(q) = -q^2 - 2q + 597
477 = -q^2 - 2q + 597
-q^2 - 2q + 597 = 477
-q^2 - 2q + 597-477 = 0
-q^2 - 2q + 120 = 0
q^2 + 2q - 120 = 0
(q+12)(q-10) = 0
q+12 = 0 or q-10 = 0
q = -12 or q = 10
Ignore negative q values. It is not possible to have negative demand.
So if the unit price is $477, then you can expect to sell 10,000 units.
================================================
Part C
Plug D(q) = 0 and solve for q
-q^2 - 2q + 597 = 0
q^2 + 2q - 597 = 0
1q^2 + 2q + (-597) = 0
1x^2 + 2x + (-597) = 0
We have an equation in the form ax^2+bx+c = 0 with a = 1, b = 2, c = -597
Use the quadratic formula to solve for x
\(x = \frac{-b\pm\sqrt{b^2-4ac}}{2a}\\\\x = \frac{-(2)\pm\sqrt{(2)^2-4(1)(-597)}}{2(1)}\\\\x = \frac{-2\pm\sqrt{2392}}{2}\\\\x \approx \frac{-2\pm48.90807704}{2}\\\\x \approx \frac{-2+48.90807704}{2} \text{ or } x \approx \frac{-2-48.90807704}{2}\\\\x \approx \frac{46.90807704}{2} \text{ or } x \approx \frac{-50.90807704}{2}\\\\x \approx 23.45403852 \text{ or } x \approx -25.45403852\\\\\)
We ignore any negative solution. The only practical solution is roughly x = 23.454, so q = 23.454 is when D(q) is equal to 0
The required answers are-
A) If 9000 units are to be sold, the price per unit is $498.
B) If the unit price is $477, then units sell is 10,000 units.
C) The value of q = 23.454 when D(9) cross the q-axis.
What is function?A function from a set X to a set Y assigns to each element of X exactly one element of Y.
The given demand function is,
D(q) = - q^2 - 2q + 597
where q is thousands of units sold and D(q) is dollars per unit
A) If 9000 units are to be sold
Thus, q = 9
since q is thousands of units sold
Putting the value of q in D(q) function we get,
D(q) = -q^2 - 2q + 597
D(9) = -(9)^2 - 2(9) + 597
D(9) = 498
Thus, The price per unit is $498.
B) If a price of $477 is set for this item
Put D(q) = 477 in the given demand function.
Thus we get,
D(q) = -q^2 - 2q + 597
477 = -q^2 - 2q + 597
-q^2 - 2q + 597 = 477
-q^2 - 2q + 597-477 = 0
-q^2 - 2q + 120 = 0
q^2 + 2q - 120 = 0
(q + 12)(q - 10) = 0
q+12 = 0 or q-10 = 0
q = -12 or q = 10
Since q cannot be negative.
So if the unit price is $477, then units sell is 10,000 units.
C) Value of q when D(9) cross the q-axis
Put D(q) = 0 in the given demand function.
Thus we get,
-q^2 - 2q + 597 = 0
q^2 + 2q - 597 = 0
q^2 + 2q + (-597) = 0
We have an equation in the form ax^2+bx+c = 0 with a = 1, b = 2, c = -597
Using the quadratic formula to solve for q.
q = 23.454 ignoring the negative value of q
Thus, the value of q = 23.454 when D(9) cross the q-axis.
Thus, The required answers are-
A) If 9000 units are to be sold, the price per unit is $498.
B) If the unit price is $477, then units sell is 10,000 units.
C) The value of q = 23.454 when D(9) cross the q-axis.
To learn more about the function :
https://brainly.in/question/610635
#SPJ2
In Exercises 35-40, find the partial derivative of the functiva respect to each variable. (35) f(t, a) = cos (2mTt - a)
Answers
The partial derivatives of the function f(t, a) = cos(2mTt - a) with respect to each variable are as follows:
∂f/∂t = -2mT sin(2mTt - a)
∂f/∂a = sin(2mTt - a)
To compute these partial derivatives, we use the chain rule of differentiation. The chain rule states that if we have a function g(h(x)), then the derivative of g with respect to x is given by g'(h(x)) multiplied by h'(x). In our case, the function g is the cosine function and the argument h is 2mTt - a.
To find the partial derivative with respect to t, we consider t as the independent variable and treat a as a constant. We differentiate the function with respect to t while keeping a constant, and we multiply the result by the derivative of the inner function, which is 2mT.
∂f/∂t = -sin(2mTt - a) * (2mT) = -2mT sin(2mTt - a)
Similarly, to find the partial derivative with respect to a, we differentiate the function with respect to a while keeping t constant. The derivative of the inner function, 2mTt - a, with respect to a is -1.
∂f/∂a = -sin(2mTt - a) * (-1) = sin(2mTt - a)
So, the partial derivative of f(t, a) with respect to t is -2mT sin(2mTt - a), and the partial derivative with respect to a is sin(2mTt - a).
To know more about the chain rule of differentiation, refer here:
https://brainly.com/question/30478987#
#SPJ11
60 is 30 percent of what number
Answers
Answer:
50
Step-by-step explanation:
The solution of number is, 200.
We have to given that;
To find a number which is 30% of number is 60.
Let us assume that,
A number is, x
Hence, We can formulate;
⇒ 60 = 30% of x
⇒ 60 = 30/100 × x
⇒ 60 = 3x / 10
⇒ 600 = 3x
⇒ 3x = 600
⇒ x = 200
Thus, The solution of number is, 600
Learn more about the percent visit:
https://brainly.com/question/24877689
#SPJ6
in a proof of the pythagorean theorem using similarity, what allows you to state that the triangles are similar in order to write the true proportions and ?the geometric mean (altitude) theoremthe geometric mean (leg) theoremthe right triangle altitude theorem the sss theorem
Answers
In a proof of the Pythagorean Theorem using similarity, the geometric mean (altitude) theorem allows us to state that the triangles are similar.
The geometric mean (altitude) theorem states that if a line is drawn parallel to the base of a right triangle, it divides the two legs of the triangle into segments proportional to the lengths of the legs.
In other words, the ratio of the lengths of the segments formed by the line is equal to the ratio of the lengths of the legs.
In the proof of the Pythagorean Theorem, we can use this theorem to show that the smaller triangles formed by drawing a line parallel to the hypotenuse are similar to the original right triangle.
Since the triangles are similar, we can write true proportions using the lengths of the sides.
For example, let's say we have a right triangle with legs of length a and b and a hypotenuse of length c.
By drawing a line parallel to the hypotenuse, we create two smaller triangles with legs of length x and y.
According to the geometric mean (altitude) theorem, we can write the proportion:
a/x = c/y
Similarly, we can write the proportion:
b/y = c/x
These proportions allow us to establish the relationship between the lengths of the sides of the right triangle and ultimately prove the Pythagorean Theorem.
To know more about Pythagorean visit:
https://brainly.com/question/28032950
#SPJ11
Prove that the medians to the legs of an isosceles triangle are congruent.
Answers
Step-by-step explanation:
Let ABC be an isosceles triangle with sides AC and BC of equal length.
We need to prove that the medians AD and BE are of equal length.
Consider the triangles ADC and BEC.
They have two congruent sides that include congruent angles.
Indeed, AC = BC by the condition, because the triangle ABC is isosceles.
Since the lateral sides AC and BC are of equal length, their halves EC
and DC are of equal length too: EC = DC.
Finally, the angle ECD is the common angle.
Thus, the triangles ADC and BEC are congruent, in accordance to the
postulate P1 (SAS) (see the lesson Congruence tests for triangles of the
topic Triangles in the section Geometry in this site).
Hence, the medians AD and BE are of equal length as the corresponding sides
of these triangles.
The proof is completed.
If a data set is normally distributed, what percent of the data will lie below the mean
A) 99,7%
B)68%
C)50%
D)95%
Answers
Answer:
c
Step-by-step explanation:
Does anyone know this?
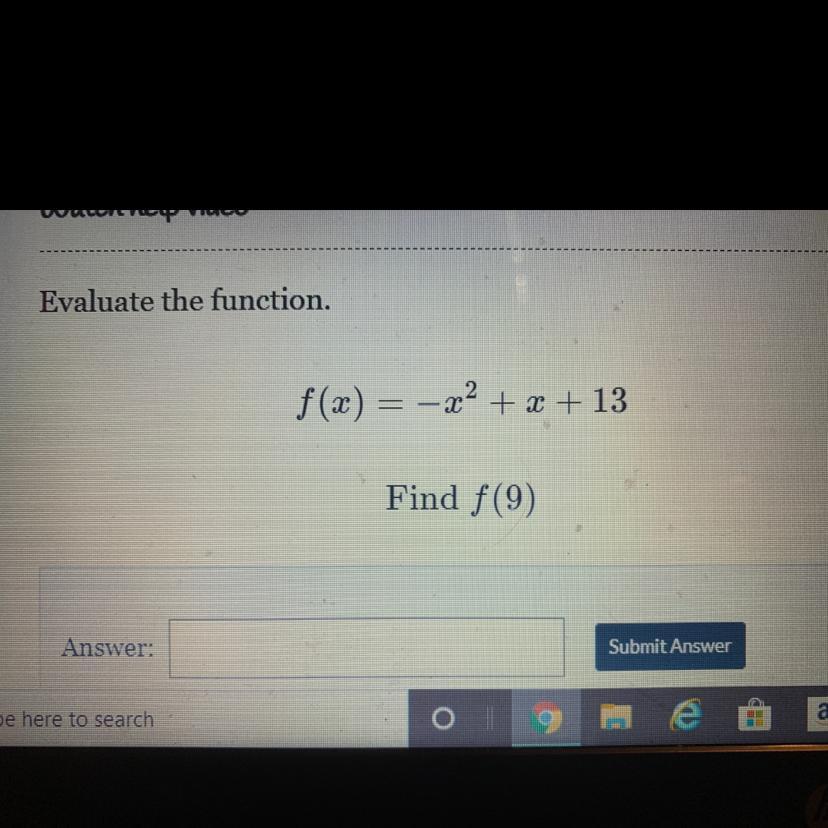
Answers
Answer:
f(x) = -x^2 + x + 13 find f(9) = -59
Hope this helps :)
Answer:
f(9)= -59
Step-by-step explanation:
First, put 9 into x; which gives you...
f(9)= (-9)^2+9+13
f(9)= -81+9+13
f(9)= -59