0.04 Equation Editor
Answers
Answer:
Step-by-step explanation:
Equation 3.0.
Related Questions
How many triangles can be drawn with one 90o angle, a 70° angle, and an included side measuring 3 inches? Explain. Select the correct choice below and, if necessary, fill in the answer box to complete your choice.
Answers
Answer:
2
Step-by-step explanation:
This is because any of the sides can be the one measuring 3 inches. Since it is a triangle, the sum of angles is 180. Let the third angle be x.
So, x + 90° + 70° = 180°
x + 160° = 180°
x = 180° - 160°
x = 20°
So, the third angle is 20°.
If the included side is a side other than the hypotenuse side, we can find it by applying the sine rule.
The included side can be either side other than the hypotenuse.
Also, either of the third angle in each triangle can be 20°
So, we have two sides and two triangles.
If the included side is the hypotenuse side, we use Pythagoras' theorem to find the length of the other two sides (and they must be equal)since the angle between the two sides is a right-angle, that is, 90°.
Since the other two angles are not equal, we cannot have a triangle with two equal sides and an hypotenuse. That is, we cannot have an isosceles triangle. So, no third triangle.
So, the total number of triangles that can be drawn with one 90° angle, a 70° angle and an included side measuring 3 inches is 2.
if a country has a natural increase rate of 2.0 percent, how long will it take to double its population?
Answers
It will take 35 years to double the population
What is Percent ?Percentages are just fractions with a denominator of 100. To show that a number is a percentage, we place the percent sign (%) next to it. For instance, if you properly answered 68 out of 100 questions on a test, you would have received a 68% grade (68/100).
According to the given information
Let \(P(0)\) be initial population
and K be the growth rate
Then Population of the country after t years
\(P = P(0)e^{Kt}\)
Let x be the present population
2x will be the double of present population
And we know
The growth rate K = 0.02%
So
The value of t could be determined as follows
\(xe^{0.02t}=2x\)
\(e^{0.02}=2\)
\(0.02t=ln(2)\)
Multiply both sides by 1000
0.02t × 1000 = ln(2) × 1000
Refine
20t = ln(2) × 1000
Divide both sides by 20
\(\frac{20t}{20}=\frac{ln(2).1000}{20}\)
t = \(ln(2).500\)
t = 0.693×50
t = 34.65 years
t = 35 years
It will take 35 years to double the population
To know more about Percent
https://brainly.com/question/28840349
#SPJ4
A small square frame has an area of 16 square inches. A large square frame has an area of 64 square inches. How much longer is the side length of the large frame than the side length of the small frame
Answers
The side length of the large frame is 4 inches longer than the side length of the small frame.
To find the difference in side lengths between the small square frame and the large square frame, we need to find the length of the sides of each frame.
Let x be the length of the side of the small square frame. Then, we know that the area of the small frame is 16 square inches.
Area of small frame = side length of small frame x side length of small frame = 16
\(x^2 = 16\)
Taking the square root of both sides, we get:
\(x = 4 inches\)
So, the length of the side of the small square frame is 4 inches.
Now, let y be the length of the side of the large square frame. We know that the area of the large frame is 64 square inches.
Area of large frame = side length of large frame x side length of large frame = 64
\(y^2 = 64\)
Taking the square root of both sides, we get:
y = 8 inches
So, the length of the side of the large square frame is 8 inches.
To find the difference in side lengths, we subtract the length of the small frame from the length of the large frame:
\(y - x = 8 - 4 = 4\)
Therefore, the side length of the large frame is 4 inches longer than the side length of the small frame.
To know more about "Square root" refer here:
https://brainly.com/question/3120622#
#SPJ11
Need help due in a few minutes. Plzzzzzz

Answers
Answer:
7 seconds
Step-by-step explanation:
set the equation to 0 because the water is the 0 point, and solve, you will get 7 and -5. It is not possible to have negative seconds so 7
And the cross-section perpendicular to the yaxis is a square. Set up a definite integral expressing the volume of the solid
Answers
The volume of the cross-section perpendicular to the solid is the amount of space in the cross-section
How to set up the integral?The question is incomplete;
So, I will give a general explanation on how to set up a definite integral for volume of a solid
Assume the solid is a cone;
Using the disk method, the integral of the volume is:
\(V = \int\limits^a_b {\pi r(x)^2} \, dx\)
Using the washer method, the integral of the volume is:
\(V = \int\limits^a_b {\pi [R(x)^2 -r(x)^2 ]} \, dx\)
Read more about volume integrals at:
https://brainly.com/question/18371476
what is the answer ?

Answers
Answer:
V = 3 x 14 x 6 1/4 = 3 x 14 x 25/4 = 1050/4 = 525/2 = 262 1/2 ft
=> Option D is correct
Hope this helps!
:)
Answer:
\( 262 \frac{1}{2} \: {ft}^{3}\)
Step-by-step explanation:
\(l = 14 \: ft \: \: \\b = 6 \frac{1}{4} \: ft = \frac{25}{4} \: ft \: \: \\h = 3 \: ft \\ \\ V_{rectangular \: prism} = lbh \\ = 14 \times \frac{25}{4} \times 3 \\ = \frac{1050}{4} \\ = 262 \frac{1}{2} \: {ft}^{3} \)
what is the length of the arc on a circle of radius r of 33cm intercepted by a central angle 1.5 radians
Answers
\(\textit{arc's length}\\\\ s = r\theta ~~ \begin{cases} r=radius\\ \theta =\stackrel{radians}{angle}\\[-0.5em] \hrulefill\\ r=33\\ \theta =1.5 \end{cases}\implies s=(33)(1.5)\implies s=49.5\)
Find the volume of a rectangular prism with length 7 feet,width 6 feet, and height 4 feet.
Answers
Answer:
V=168ft³
Step-by-step explanation:
V=
w-6
h-4
l-7
V = 168ft³
9) Solve and graph each inequality on a number line.g. -6x ≤ -42
Answers
To solve the inequality:
\(\begin{gathered} -6x\leq-42 \\ \text{Divide both sides by -6:} \\ \frac{-6}{-6}x\leq\frac{-42}{-6} \\ x\ge7 \end{gathered}\)Now, the graph of this inequality would be a number line with a shaded region of any number greater or equal than 7, like this:

is -3x+2y=23 a linear equation
Answers
-3x+2y=23
Yes, the above equation is linear equation.
What is AAA similarity rule?
Answers
The AAA (angle-angle-angle) similarity theorem can be used to express it as follows: two triangles have equal corresponding angles if and only if their corresponding sides are proportionate.
The Angle-Angle-Angle (AAA) criterion for the similarity of triangles states that “If in two triangles, corresponding angles are equal, then their corresponding sides are in the same ratio (or proportion) and hence the two triangles are similar”.
Proof:
Consider two triangles ABC and DEF, such that ∠A = ∠D, ∠B = ∠E and ∠C = ∠F as shown in the figure.
Now, cut DP= AB and DQ= AC and join PQ. Hence, we can say that a triangle ABC is congruent to the triangle DPQ.
(i.e) ∆ ABC ≅ ∆ DPQ, which gives ∠B = ∠P = ∠E and also, the line PQ is parallel to EF.
Therefore, by using the basic proportionality theorem, we can write
DP/PE = DQ/QF.
(i.e) AB/DE = AC/DF. …(1)
Similarly,
AB/DE = BC/EF …(2)
From (1) and (2), we can write:
AB/DE = BC/EF = AC/DF.
Therefore, the two triangles ABC and DEF are similar.
Hence, AAA(Angle-angle-angle) is proved.
To know more about Triangle check the below link:
https://brainly.com/question/7620723
#SPJ1
use the trapezoidal rule and simpson's rule to approximate the value of the definite integral for the given value of n. round your answer to four decimal places and compare the results with the exact value of the definite integral. 5 x x2 4 0 dx, n
Answers
Exact value of the definite integral is 320. Comparing the results: Exact value of the definite integral = 320, Trapezoidal Rule approximation (n = 4) = 340, Simpson's Rule approximation (n = 4) ≈ 246.6667.
What is trapezoid?
A trapezoid is a quadrilateral (a polygon with four sides) that has one pair of parallel sides. The parallel sides are called the bases of the trapezoid, while the non-parallel sides are called the legs.
To approximate the value of the definite integral ∫[0, 4] 5x * x^2 dx using the Trapezoidal Rule and Simpson's Rule, we need to specify the value of n, which represents the number of subintervals.
Let's calculate the approximations using n = 4 for both methods:
Trapezoidal Rule:
Using n = 4, we divide the interval [0, 4] into four subintervals of equal width: h = (4 - 0) / 4 = 1.
The approximated value using the Trapezoidal Rule is given by:
\(T_4 = (h/2) * [f(x_0) + 2f(x_1) + 2f(x_2) + 2f(x_3) + f(x_4)]\)
Plugging in the values:
\(T_4 = (1/2) * [f(0) + 2f(1) + 2f(2) + 2f(3) + f(4)]\\\\= (1/2) * [5(0)(0^2) + 2(5)(1)(1^2) + 2(5)(2)(2^2) + 2(5)(3)(3^2) + 5(4)(4^2)]\\\\= (1/2) * [0 + 10 + 80 + 270 + 320]\\\\= (1/2) * 680\\\\= 340\)
Simpson's Rule:
Using n = 4, we divide the interval [0, 4] into four subintervals of equal width: h = (4 - 0) / 4 = 1.
The approximated value using Simpson's Rule is given by:
\(S_4 = (h/3) * [f(x_0) + 4f(x_1) + 2f(x_2) + 4f(x_3) + f(x_4)]\)
Plugging in the values:
\(S_4 = (1/3) * [f(0) + 4f(1) + 2f(2) + 4f(3) + f(4)]\\\\= (1/3) * [5(0)(0^2) + 4(5)(1)(1^2) + 2(5)(2)(2^2) + 4(5)(3)(3^2) + 5(4)(4^2)]\\\\= (1/3) * [0 + 20 + 40 + 360 + 320]\\\\= (1/3) * 740\\\\= 246.6667\)
Exact value of the definite integral:
∫[0, 4] 5x * \(x^2\) dx = [(5/4) * \(x^4\)] evaluated from 0 to 4
\(= (5/4) * 4^4 - (5/4) * 0^4\\\\= (5/4) * 256 - (5/4) * 0\\\\= 320 - 0\\\\= 320\)
Comparing the results:
Exact value of the definite integral = 320
Trapezoidal Rule approximation (n = 4) = 340
Simpson's Rule approximation (n = 4) ≈ 246.6667
As we can see, the Trapezoidal Rule approximation is slightly greater than the exact value, while Simpson's Rule approximation is less than the exact value.
To learn more about trapezoid visit:
https://brainly.com/question/1410008
#SPJ4
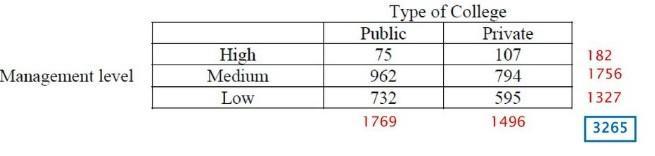
Researchers looking at the relationship between the type of college attended (public or private) and achievement gather the following data on 3265 people who graduated from college in the same year. The variable "management level" describes their job description 20 years after graduating from college.
Type of College
Public Private High 75 107 Medium 962 794 Low 732 595
Management level Calculate the marginal distribution of management level in percents.
Answers
For a data set of relationship between the type of college and management level, the marginal distribution with frequencies of management level high, medium and low in percents are 5.6%, 53.8% and 40.6% respectively..
The marginal relative frequency of a data set is determined by dividing the sum of a row or the sum of a column by the total number of values in a dataset. The relationship between the type of college attended (public or private).
Numbers of people = 3265
See the above table represents the different management levels ( high, medium and low) for different types of colleges. We have to calculate the marginal distribution of management level in percents. See the above figure 2 which contains all total or sum values of each row and columns.
Total frequency in this case = 3265
Number of high management level = 182
Marginal frequency Percent for high management level = \( ( \frac{182}{3265})100\)
= 5.6%
Number of medium management level
= 1756
Marginal frequency Percent for medium management = \( ( \frac{1756}{3265})100\)
= 53.8%
Number of low management level
= 1327
Marginal frequency Percent for low management = \( ( \frac{1327}{3265})100\) = 40.6%
Hence, required value is 40.6%.
For more information about marginal distribution, visit :
https://brainly.com/question/30438396
#SPJ4
Complete question:
The above figure complete the question.
Researchers looking at the relationship between the type of college attended (public or private) and achievement gather the following data on 3265 people who graduated from college in the same year. The variable "management level" describes their job description 20 years after graduating from college. Type of College Public Private High 75 107 Medium 962 794 Low 732 595
Management level Calculate the marginal distribution of management level in percents.

please explain this in steps please friends

Answers
Answer:
See below
Step-by-step explanation:
The LHS and RHS are exactly the same, so the equality is true.
The associate property of addition says that
\(\boxed{a+(b+c)=(a+b)+c}\)
In order to make the addition of fractions, the denominators should be same. So we can write three fractions as one.
\(\dfrac{1}{2} +\left(\dfrac{2}{3}+\dfrac{3}{4} \right )\)
\(\dfrac{1}{2} +\dfrac{2}{3}+\dfrac{3}{4}\)
\(\dfrac{1\cdot 6 }{2\cdot 6} +\dfrac{2 \cdot 4}{3 \cdot 4}+\dfrac{3 \cdot 3 }{4 \cdot 3}\)
\(\dfrac{ 6 }{12} +\dfrac{8}{12}+\dfrac{9 }{12}\)
\(\dfrac{ 23 }{12}\)
Answer:
You gotta give me (us) more to work with homie.
Step-by-step explanation:
If I have more of a picture with it I could possibly help but all there is a barely readable and legible nonsense equation. Sorry :(
what is the answer to - (-8 - 6t)
Answers
Answer:
8 + 6t
Step-by-step explanation:
just distribute the negative signs in front of the parenthesis which is technically just -1
or if its easier, just multiply the numbers inside the parenthesis by -1 and get rid of the parenthesis
The number of students in the yearbook club and the number of students on student council are shown in the Venn diagram. Based on the diagram, find the following:

Answers
Answer:
2
Step-by-step explanation:
Y ∩ S means the intersection of sets Y and S.
The intersection of two sets is the set that contains the element present in both sets.
In this case, 2 students are both in the yearbook club and in the student council.
Answer: 2
Select the correct answer from each drop-down menu.
Let e(x) be the elevation, in meters, of a cable car from the ground, x minutes after it begins descending from the top
e(t) = 840 – 52.51
meters from the ground
minutes after it
Since e(14) = , the elevation of the cable car will be
from the top of the hill,
Answers
Corrected Question
e(x)=840-52.5x
Let e(x) be the elevation, in meters, of a cable car from the ground, x minutes after it begins descending from the top of a hill. Since e(14) = ?, the elevation of the cable car will be meters from the ground minutes after it begins descending from the top of the hill.
Answer:
e(14) =105 meters
Elevation of the cable car 14 minutes after it begins descending from the top of the hill= 105 meters from the ground
Step-by-step explanation:
Given the elevation, e(x) in meters, of a cable car from the ground , x minutes after it begins descending from the top of a hill.
e(x)=840-52.5x
e(14)=840-52.5(14)
e(14)=840-735
e(14)=105 meters
Since e(14) =105 meters, the elevation of the cable car will be 105 meters from the ground 14 minutes after it begins descending from the top of the hill.
A savings account balance is compounded continuously.If the interest rate is 3.1% per year and the current balance is 1077.00 in how many years will the balance reach 1486.73?
Answers
Answer:13.8 is the best answer i could get
Step-by-step explanation:1,077 multiply that × 13.8 =14,862
find the marked angle of

Answers
Answer:
∠ C = 100°
Step-by-step explanation:
since 2 sides of the triangle are congruent then the triangle is isosceles with base angles congruent.
consider the angle inside the triangle to the left of 140°
this angle and 140° are a linear pair and sum to 180°
angle + 140° = 180° ( subtract 140° from both sides )
angle = 40°
then the angle on the left of the triangle = 40° ( base angles congruent )
the sum of the angles in a triangle = 180° , so
∠ C + 40° + 40° = 180°
∠ C + 80° = 180° ( subtract 80° from both sides )
∠ C = 100°
Find the range for the possible measure of the third side of a triangle given two sides?
4 cm, 6 cm
Range:
Answers
Answer:
The possible range of the measure of the third side is 2 < 3rd side < 10
Step-by-step explanation:
In any triangle:
The sum of the lengths of any two sides must be greater than the length of the 3rd sideThe length of any side must greater than the difference of the lengths of the other two sidesThe difference of the other sides < The side < The sum of the other sidesLet us solve the question
∵ The measures of the two sides of a triangle are 4 cm and 6 cm
∵ The difference between their measures = 6 - 4 = 2 cm
∵ The sum of their measures = 6 + 4 = 10 cm
→ By using the rules above
∴ The measure of the third side is between 2 and 10
∴ 2 < the third side < 10
∴ The possible range of the measure of the third side is 2 < 3rd side < 10
Mark ran 12 miles in 132 minutes. Assume Mark maintains a constant speed. How many minutes does it takes Mark to run 1 mile.
Answers
Answer:
11
Step-by-step explanation:
132 divided by 12=11
12x12=144, subtract 12 and you get 12x11=132
BRAINLIEST PLZ???
Mr.Joseph recently received a notice that his average gas and electric bill would increase at the beginning of the year. He went online to see the amount of his last 12 bills. If his average bill over the past 12 month goes up 17%, what can he expect to pay on average next year.
Answers
Answer:
about 2,546
Step-by-step explanation:
2 2.1 Mathematical intro show that there is another form for spherical harmonics: 1 3 3 Y₁ x iy 1/√√2 (²-1) 2πT 2π 1 3 3 z YO 2 2π π r 1 3 x iy Y₁¹ 3 2π - - 12 √ √ 2² (²+²) 2 2π
Answers
Spherical harmonics are an integral part of quantum mechanics. They describe the shape of the orbitals, which electrons occupy in atoms. Moreover, the spherical harmonics provide the angular distribution of a wave in spherical coordinates. In 3D, the spherical harmonics can be written as:
Ylm(θ, φ) = √(2l + 1)/(4π) * √[(l - m)!/(l + m)!] * Plm(cosθ) * e^(imφ)
Here, l and m are known as the angular quantum numbers. They define the shape and orientation of the orbital. Plm(cosθ) represents the associated Legendre polynomial, and e^(imφ) is the exponential function. The spherical harmonics have various forms, including:
Y1,1 = -Y1,-1 = 1/2 √(3/2π) sinθe^(iφ)
Y1,0 = 1/2 √(3/π)cosθ
Y2,2 = 1/4 √(15/2π)sin²θe^(2iφ)
Y2,1 = -Y2,-1 = 1/2 √(15/2π)sinθcosφ
Y2,0 = 1/4 √(5/π)(3cos²θ-1)
Y0,0 = 1/√(4π)
The spherical harmonics have various applications in physics, including quantum mechanics, electrodynamics, and acoustics. They play a crucial role in understanding the symmetry of various systems. Hence, the spherical harmonics are an essential mathematical tool in modern physics. Thus, this is how one can show another form for spherical harmonics.
To know more about Spherical harmonics visit
https://brainly.com/question/23067012
#SPJ11
Why are we here what is the purpose?
Answers
Answer:
We are here because we need help with a particular course or question
Step-by-step explanation:
Our goal is to try our best and explain to others to their understanding (and win titles)
BOOM!!!
A company determines the mean and standard deviation of the employees' salaries in one year. What is the best description of the standard deviation?
Approximately the median distance between the individual salaries of employees and the mean of every employee's salary.
The amount of money separating the highest salary from the lowest salary when considering the middle 50% of the distribution.
The amount of money separating the highest salary from the lowest salary when considering all employees.
The distance between the salary of an employee and the mean salary of all the employees.
Approximately the mean distance between the individual salaries of employees and the mean of all employees' salaries.
Answers
The best description of the standard deviation is "Approximately the mean distance between the individual salaries of employees and the mean of all employees' salaries."
Standard deviation, denoted by σ, is defined as the measure which indicates the average distance of the observations from the mean of the data set. It tells how spread out numbers are. It is equal to the square root of the variance. Variance is the average of the squared differences from the mean.
For the given problem, the standard deviation can be describe as the average(mean) distance of the individual salaries of employees from the mean of all employees' salaries.
Learn more about standard deviation here: https://brainly.com/question/475676
#SPJ4
Find an equation of the plane that is orthogonal to the plane 8 x + 7 z = 2 and contains the line of intersection of the planes 2x - 3y + z = 28 and x + 2y - 3z = 35.
(a) 7 x + y - 8 z = 160 (b) 7 x + y - 8 z = 167 (c) 7 x + y - 8 z = 169 (d) 7 x + y - 8 z = 156 (e) None of the given. (f) 7 x + y - 8 z = 179
Answers
The equation of the plane that is orthogonal to the plane 8x + 7z = 2 and contains the line of intersection of the planes 2x - 3y + z = 28 and x + 2y - 3z = 35 can be found by taking the cross product of the normal vectors of the two given planes. The correct option is (e) None of the given.
The normal vectors of the planes 2x - 3y + z = 28 and x + 2y - 3z = 35 are <2, -3, 1> and <1, 2, -3> respectively. Taking the cross product of these two vectors gives us the normal vector of the plane that contains the line of intersection.
Cross product: <2, -3, 1> x <1, 2, -3> = <7, -8, -7>
Now we have the normal vector of the desired plane, which is <7, -8, -7>. Using this normal vector, we can write the equation of the plane in the form ax + by + cz = d.
Substituting the values, we have 7x - 8y - 7z = d.
To determine the value of d, we can substitute the coordinates of a point on the line of intersection of the given planes into the equation. Let's take the point (2, -7, -1) which lies on the line of intersection.
Substituting the values, we get 7(2) - 8(-7) - 7(-1) = 14 + 56 + 7 = 77.
Therefore, the equation of the plane that is orthogonal to the plane 8x + 7z = 2 and contains the line of intersection of the planes 2x - 3y + z = 28 and x + 2y - 3z = 35 is 7x - 8y - 7z = 77.
The correct option is (e) None of the given.
Know more about line of intersection :brainly.com/question/14217061
#SPJ11
out of 500 people sampled, 375 had kids. assuming the conditions are met, construct a 99% confidence interval for the true population proportion of people with kids.
Answers
99% confidence interval for the true population proportion of people with kids : {0.700 , 0.799}
Given,
n = 500
X = 375
Here,
p = X/n
p = 375/500
p = 3/4
p = 0.75
Confidence interval: 99%
= 1-0.99
= 0.01
Calculate,
α/2
= 0.01/2
= 0.005
\(So,\\\)
\(Z_{\alpha /2}\) = 2.576
99% confidence interval for population proportion,
= p ± \(Z_{\alpha /2}\) √p(1-p)/n
= 0.75 ± 2.576(√0.75(1-0.75))/500
= {0.700 , 0.799}
Know more about confidence interval,
https://brainly.com/question/32546207
#SPJ4
Which of the following are assumptions for the confidence interval for the different between two population means?
Data is Quantitative.
Data is from a Convenience Sample
Random Sample
Both sample sizes are greater than 30 or the data from a Normal Distribution
Data is Categorical.
There are at least 15 successes and 15 failures.
Answers
The valid assumptions for constructing a confidence interval for the difference between two population means are: Data is Quantitative, Random Sample and Both sample sizes are greater than 30 or the data is from a Normal Distribution.
To answer your question, when constructing a confidence interval for the difference between two population means, certain assumptions must be met. These assumptions include:
1. Data is Quantitative: Since we are dealing with population means, the data should be quantitative, meaning it consists of numerical values. This is a correct assumption.
2. Data is from a Convenience Sample: This is not a valid assumption. To ensure the reliability of the confidence interval, data should be collected through random sampling, which ensures that each individual in the population has an equal chance of being included in the sample.
3. Random Sample: This is a correct assumption. A random sample is necessary to ensure the sample's representativeness and accuracy in estimating the population means.
4. Both sample sizes are greater than 30 or the data is from a Normal Distribution: This is a valid assumption. If both sample sizes are greater than 30, the Central Limit Theorem can be applied, which states that the sampling distribution of the sample means will be approximately normal. If the data is already from a normal distribution, the normality assumption is met.
5. Data is Categorical: This assumption is incorrect. As previously mentioned, the data should be quantitative for this analysis.
6. There are at least 15 successes and 15 failures: This assumption is not relevant for confidence intervals for the difference between two population means. This criterion is related to proportions rather than means.
To learn more about confidence interval, refer:-
https://brainly.com/question/24131141
#SPJ11
Someone help me out please?

Answers
1/4=25/100 fraction
0.25=25/100 decimal
25%=25/100 percent
All three are variation of the same value
Maxine spent 15 hours doing her homework last week. This week she spent 18 hours
doing homework. She
says that she spent 120% more time doing homework this week.
Is she correct? Show your work to justify your decision.
Answers
Answer:
It's incorrect. there is no such thing as 120%
Step-by-step explanation:
No step problem